Implicit Differentiation Day 2
TLDRThe video lesson covers implicit differentiation, a key concept for AP calculus free response questions. The instructor explains how to find dy/dx (or y prime) for a given implicit function and demonstrates solving for the derivative. They also discuss finding the equation of the tangent line at a specific point and identifying points where the tangent to the curve is vertical. The lesson includes step-by-step calculations, factoring, and using a calculator for complex algebraic solutions, making it a thorough and practical guide for students.
Takeaways
- 📚 The lesson is focused on practicing implicit differentiation, which is a topic that could appear on an AP test as a free response question.
- 🔍 The instructor introduces an implicit function and explains that it is not a function in the traditional sense, as it does not pass the vertical line test.
- 📝 The notation Y' is used to represent the derivative of Y with respect to X, alongside the traditional dy/dx notation, to emphasize their equivalence.
- 🧐 The derivative of the given implicit function is derived by differentiating each term with respect to X and rearranging to solve for Y'.
- 🔢 The derivative is simplified to Y' = 2x / (3y^2 + 2y - 5), which is a key step in finding the slope of the tangent line at any point on the curve.
- 📈 The equation of the tangent line at a specific point (1, -3) on the curve is found using the slope derived from the derivative at that point.
- 📌 The slope at the point (1, -3) is calculated to be 1/8, indicating a very gentle incline, almost flat.
- 🔍 The instructor discusses finding points on the curve where the tangent line is vertical, which corresponds to where the derivative (slope) is undefined.
- 🤔 To find vertical tangents, the bottom of the fraction in the derivative (3y^2 + 2y - 5) is set to zero, ensuring the top is not zero, leading to potential vertical tangents.
- 📊 The instructor estimates and then verifies the points where vertical tangents occur, using both algebraic methods and calculator assistance for complex calculations.
- 📝 The final points where vertical tangents occur are (1, 1), (-1, 1), and two additional points calculated with a calculator, approximately (3.23, -5/3) and (-3.23, -5/3).
Q & A
What is the main topic of the video script?
-The main topic of the video script is implicit differentiation, with a focus on finding the derivative of an implicit function and applying it to find the equation of the tangent line and points where the tangent is vertical.
Why can't the given curve be written as a function of x?
-The curve cannot be written as a function of x because the implicit equation does not pass the vertical line test, indicating that there is not a unique y value for each x value.
What are the two notations used to represent the derivative of y with respect to x in the script?
-The two notations used are dy/dx and y' (y prime), both representing the derivative of y with respect to x.
What is the process for finding the derivative of the given implicit function?
-The process involves differentiating each term of the implicit equation with respect to x, factoring out y' (or dy/dx), and then solving for y' to express it in terms of x and y.
How is the equation of the tangent line to the curve at a specific point found?
-The equation of the tangent line is found by using the point-slope form y - y1 = m(x - x1), where m is the slope (y') at the point (x1, y1).
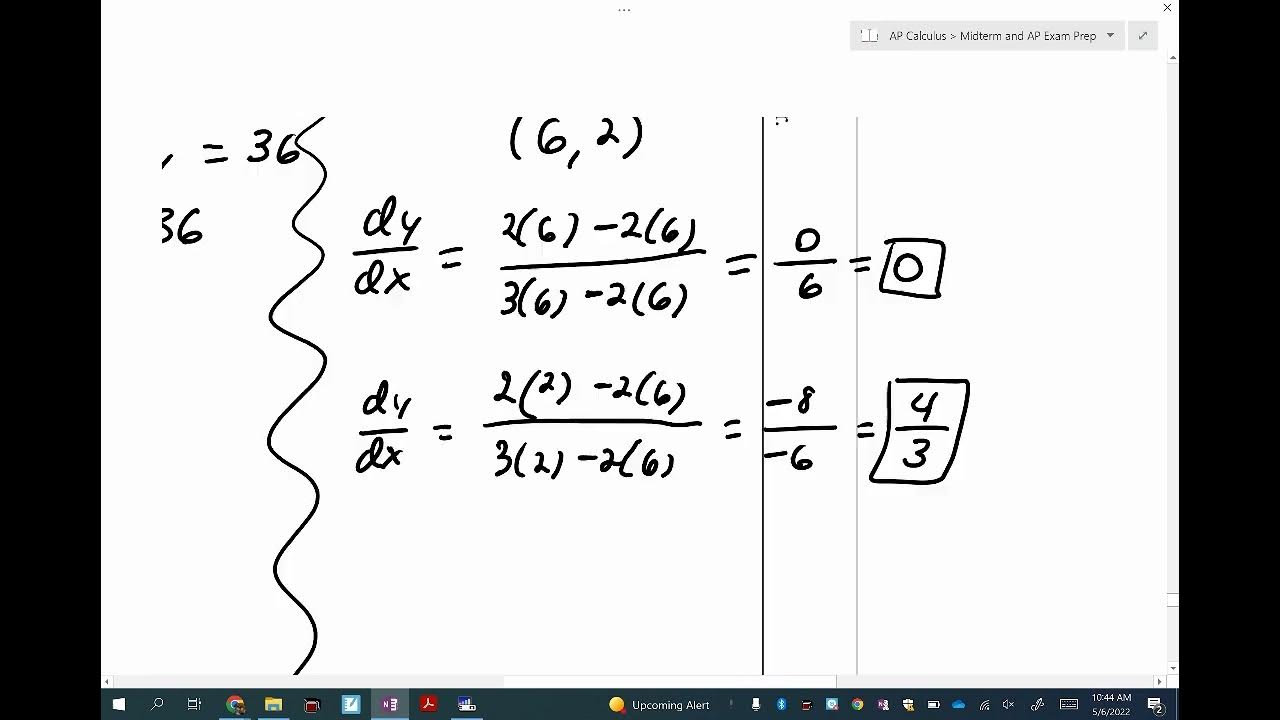
What is the slope of the tangent line at the point (1, -3)?
-The slope of the tangent line at the point (1, -3) is calculated to be 1/8, found by substituting x = 1 and y = -3 into the derivative y'.
What does it mean for a tangent line to be vertical?
-A tangent line is vertical when the slope is undefined, which occurs when the denominator of the derivative expression equals zero while the numerator does not.
How many vertical tangent lines are there on the graph according to the script?
-According to the script, there are four vertical tangent lines on the graph.
How are the coordinates of the points with vertical tangent lines determined?
-The coordinates are determined by setting the expression in the denominator of the derivative (3y^2 + 2y - 5) equal to zero and solving for y, then using the original implicit equation to find the corresponding x values.
What is the significance of using both dy/dx and y' notations in the script?
-Using both notations helps to clarify that they represent the same concept and allows for a comparison of the different ways in which derivatives can be expressed in mathematical literature and textbooks.
Outlines
📘 Introduction to Implicit Differentiation
The instructor greets the students and introduces the topic of implicit differentiation, emphasizing its importance for the AP test. The instructor demonstrates finding dy/dx for an implicit function using both dy/dx and y' notations, and explains the significance of X being the independent variable. The process involves differentiating and solving for y', and includes factoring out y' and simplifying the expression.
📝 Finding the Equation of the Tangent Line
The instructor explains how to find the equation of the tangent line to the curve at a specific point (1, -3). Using the point-slope form of the equation, the instructor demonstrates how to calculate the slope by substituting the given point into the derivative. After verifying the slope calculation, the final equation of the tangent line is presented as y + 3 = 1/8(x - 1).
📈 Identifying Points with Vertical Tangents
The instructor discusses finding points on the curve where the tangent line is vertical. This involves determining where the derivative is undefined by setting the denominator of the slope fraction to zero while ensuring the numerator is not zero. Through solving the quadratic equation 3y^2 + 2y - 5 = 0, two y-values (1 and -5/3) are found. The instructor plugs these y-values back into the original equation to find the corresponding x-values, resulting in points (1, 1), (-1, 1), and two additional points involving square roots.
🔢 Utilizing Calculators for Complex Solutions
The instructor acknowledges the difficulty of solving for x when y = -5/3 algebraically and resorts to using a calculator (TI-89) to find the exact values. The calculator provides solutions involving square roots, which are then verified against the graph. The instructor concludes that such problems might be intended as calculator questions and informs students about an upcoming worksheet for further practice.
Mindmap
Keywords
💡Implicit differentiation
💡Free response question
💡Vertical line test
💡Notation
💡Independent variable
💡Tangent line
💡Slope
💡Undefined slope
💡Factoring
💡Calculator
Highlights
Introduction to implicit differentiation with a focus on AP test preparation.
The importance of recognizing the independent variable in differentiation.
Using both dy/dx and y' notations for derivatives to provide clarity.
Differentiating an implicit function to find dy/dx or y'.
Factoring out y' to solve for the derivative in terms of x and y.
Deriving the formula for the slope of the tangent line at a given point on the curve.
Calculating the slope of the tangent line at a specific point using y'.
Writing the equation of the tangent line using the point-slope form.
Identifying the coordinates of the point where the tangent line is vertical.
Understanding that a vertical tangent line implies an undefined derivative.
Setting up the equation to find points with a vertical tangent by making the denominator zero.
Solving the equation for y to find potential points of vertical tangency.
Using the original implicit equation to find corresponding x-coordinates.
Estimating and verifying the existence of vertical tangent lines on the curve.
The practical use of a calculator to solve complex equations for x.
The importance of verifying calculator results with the original equation.
The conclusion of the lesson with a reminder of the upcoming practice worksheet.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: