Improper Integrals - Discontinuity in the Interval

turksvids
8 Jun 201405:45
EducationalLearning
32 Likes 10 Comments

TLDRThis video script delves into improper integrals, focusing on the second type where the function is discontinuous between the limits of integration. The instructor demonstrates how to handle discontinuities by splitting the integral into two parts and approaching the point of discontinuity from both sides. Two examples illustrate the process: the first integral diverges to negative infinity, while the second converges to zero, highlighting the importance of checking convergence for each part before summing the results.

Takeaways
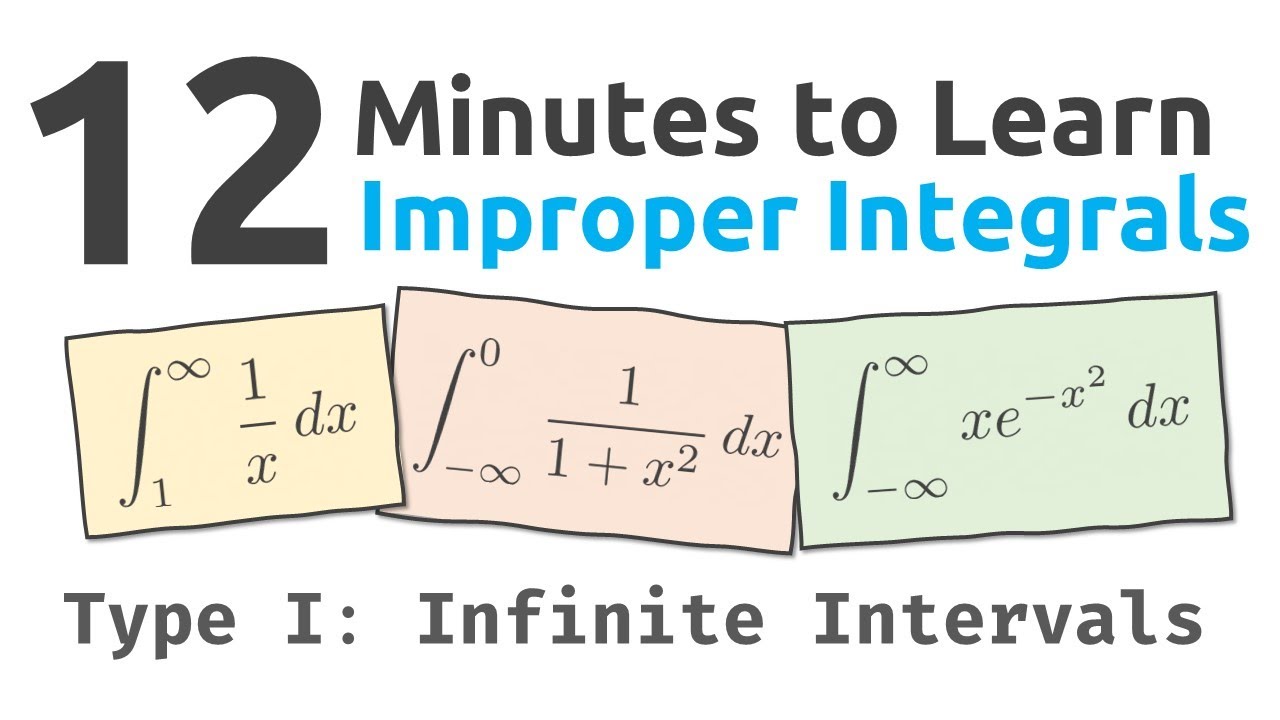
  • 📚 There are three types of improper integrals: those with a discontinuity at the limits, those with a discontinuity within the bounds, those with infinite limits, and a combination of the second and third types.
  • 🔍 The second type of improper integral, which the video focuses on, involves a function that is discontinuous somewhere between the limits of integration.
  • 📉 When dealing with a discontinuity within the bounds, the integral is split into two parts, approaching the point of discontinuity from the left and the right.
  • ⚠️ It's important to identify the discontinuity to understand how to handle the integral properly.
  • 🔄 The function 1/x^3 is discontinuous at x=0, and the integral from -2 to 2 of this function is used as an example to demonstrate the process.
  • 💥 If the first integral diverges to negative infinity, the second integral does not need to be calculated, as the overall integral is already divergent.
  • 📌 The integral's convergence is determined by the finiteness of both split integrals; if one diverges, the entire integral diverges.
  • 📐 The integral from 1 to 3 of 1/(x-2)^(1/3) is used as another example, with a discontinuity at x=2.
  • 🔢 For the second example, the integral converges to zero after evaluating both parts from the left and the right of the discontinuity.
  • 📝 The process involves taking limits and using the fundamental theorem of calculus to evaluate the integrals.
  • 🔄 The video script provides a step-by-step guide on how to approach and solve improper integrals with a discontinuity within the bounds.
Q & A

  • What are the three types of improper integrals discussed in the video?

    -The three types of improper integrals discussed are: 1) Integrals with a discontinuity at either A or B, 2) Integrals where the function is discontinuous somewhere between A and B, and 3) Integrals where A or B, or both, could be infinite.

  • What is the fourth case mentioned in the video regarding improper integrals?

    -The fourth case is a combination of the second and third types, where there is a discontinuity within the bounds and one or both of the limits of integration are infinite.

  • How does the video handle the integral of 1/x^3 from -2 to 2?

    -The integral is handled by breaking it into two integrals, one from -2 to a value C approaching 0 from the left, and the other from C approaching 0 from the right to 2. The function is discontinuous at 0, so this method is used to approach the discontinuity from both sides.

  • Why does the first integral of 1/x^3 from -2 to a value approaching 0 from the left diverge?

    -The first integral diverges because as C approaches 0 from the left, the value of 1/(-C)^3 becomes increasingly negative and large, effectively going to negative infinity, indicating divergence.

  • What is the significance of the statement 'as soon as you don't get a finite answer to the first integral, you stop'?

    -This statement emphasizes that if one part of an improper integral does not converge to a finite number, the entire integral is considered to diverge, and there is no need to calculate the second part, as the overall result will not be finite.

  • How is the integral from 1 to 3 of (1/(X-2)^(1/3)) handled in the video?

    -The integral is split into two parts, one from 1 to a value C approaching 2 from the left, and the other from C approaching 2 from the right to 3, due to the discontinuity at X=2.

  • What is the result of the improper integral from 1 to 3 of (1/(X-2)^(1/3))?

    -The result of the improper integral is 0, as the first part converges to -3/2 and the second part converges to 3/2, and their sum equals zero.

  • Why is it important to evaluate the limits when dealing with improper integrals?

    -Evaluating the limits is crucial because it allows you to determine whether the integral converges or diverges, which is necessary for understanding the behavior of the function at the points of discontinuity or at infinity.

  • What does the video suggest about the relationship between the two parts of an improper integral?

    -The video suggests that for an improper integral to have a finite value, both parts resulting from the split must converge to finite numbers. The sum of these finite numbers gives the value of the integral.

  • What is the fundamental theorem of calculus used for in the context of this video?

    -The fundamental theorem of calculus is used to evaluate the integrals by finding the antiderivative of the function and then applying the limits of integration to determine the value of the integral.

  • Why might the video mention 'symmetric infinities' in the context of improper integrals?

    -The mention of 'symmetric infinities' refers to a misconception that positive and negative infinities might cancel each other out. The video clarifies that this is not the case; both integrals must converge to finite numbers for the overall integral to have a finite value.

Outlines
00:00
📚 Understanding Improper Integrals with Discontinuities

This paragraph introduces the concept of improper integrals, focusing on the second type where the function is discontinuous between the bounds of integration. The speaker explains how to handle such integrals by breaking them into two parts, approaching the point of discontinuity from both the left and the right. An example is given where the integral of 1/X^3 from -2 to 2 is discussed, highlighting the divergence of the integral as it approaches zero from the left, leading to the conclusion that the integral diverges without needing to calculate the right-sided approach.

05:00
🔍 Calculating Improper Integrals with Finite Results

The second paragraph delves into another example of an improper integral, this time with a discontinuity at X = 2 for the integral from 1 to 3 of 1/(X - 2)^(1/3). The integral is split into two parts, with the first part calculated as C approaches 2 from the left, yielding a finite value. The second part involves a limit as C approaches 2 from the right, which also results in a finite value. The paragraph concludes by summing the results of both integrals, showing that the overall improper integral converges to zero. The explanation emphasizes the importance of obtaining finite values for both parts to determine the convergence of the integral.

Mindmap
Keywords
💡Improper Integrals
Improper integrals are a type of integral where the integral is not well-defined due to one or more of the limits being infinite or the function being undefined (discontinuous) within the interval. In the video, the focus is on the second type, where the function is discontinuous within the bounds of integration. This is a key concept as it sets the stage for the methods used to evaluate such integrals.
💡Discontinuity
Discontinuity refers to a point in a function where the function is not continuous, meaning there is a break or gap in the graph of the function. In the context of the video, discontinuity is a critical issue that needs to be addressed when dealing with improper integrals, as it affects how the integral is evaluated.
💡Integral Bounds
Integral bounds are the limits of integration, typically denoted as 'a' and 'b' in the integral from 'a' to 'b'. In the video, the script discusses how to handle cases where the integral bounds include points of discontinuity, which is a central theme in understanding how to approach improper integrals.
💡Limit
In the context of improper integrals, the term 'limit' is used to describe the process of approaching a point of discontinuity from one side or the other. The video script uses limits to break down the integral into parts that can be evaluated separately, which is a common technique in dealing with discontinuities.
💡Convergence
Convergence in the context of improper integrals refers to whether the integral yields a finite value. The video emphasizes that for an improper integral to have a meaningful value, both parts of the integral (when split due to discontinuity) must converge to finite numbers. This is a crucial concept in determining the validity of the integral.
💡Diverges
Diverges is the opposite of converges. When an integral diverges, it means that it does not yield a finite value. In the video, the script explains that if one part of an improper integral diverges, the entire integral is considered to diverge, and further calculations are unnecessary.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a key mathematical principle that relates the process of integration to the concept of the derivative. In the video, the theorem is used to evaluate the integrals by finding antiderivatives and then applying the limits of integration.
💡Reciprocal
The reciprocal of a number is 1 divided by that number. In the video script, the reciprocal is used in the context of the integrals being discussed, particularly when dealing with functions like 1/x^3 or 1/(x-2)^(1/3), where the reciprocal appears in the integrand.
💡Symmetric Infinities
Symmetric infinities refer to the idea that if one part of an improper integral diverges to negative infinity and the other part diverges to positive infinity, they do not cancel each other out to yield a finite value. The video script warns against this misconception, clarifying that only finite values are meaningful in the context of improper integrals.
💡Type 2 Improper Integrals
Type 2 improper integrals are those where the function being integrated is discontinuous somewhere between the bounds of integration. The video script specifically focuses on this type, explaining how to handle the discontinuity by breaking the integral into parts and evaluating them separately.
💡Type 3 Improper Integrals
Type 3 improper integrals involve one or both of the bounds of integration being infinite. Although not the main focus of the video script provided, the script mentions that Type 3 will be discussed in a future video, indicating that it is another category of improper integrals that requires special handling.
Highlights

Introduction to improper integrals and their three types.

Type 1 improper integrals have discontinuities at either A or B.

Type 2 improper integrals have discontinuities somewhere between A and B.

Type 3 improper integrals involve infinite limits of integration.

Type 4 improper integrals combine the issues of types 2 and 3.

Example of handling type 2 improper integrals by breaking them into two integrals.

Discontinuity at zero in the integral from negative 2 to 2 of 1 over X cubed DX.

Approaching discontinuity from the left and right to evaluate the integral.

First integral diverges to negative infinity as C approaches zero from the left.

If one integral diverges, the improper integral is considered divergent.

Example of the integral from 1 to 3 of DX over the quantity X minus 2 to the 1/3.

Discontinuity at 2 in the integral, handled by breaking it into two parts.

First integral converges to a finite number as C approaches 2 from the left.

Second integral also converges to a finite number as C approaches 2 from the right.

Both integrals converging to finite numbers allows for the sum to be the value of the improper integral.

Final value of the improper integral is the sum of the values of the two convergent integrals.

Explanation of why symmetric infinities do not cancel out in improper integrals.

Upcoming discussion on type 3 improper integrals in a future video.

Transcripts

Browse More Related Video

Improper Integrals of Type I (Infinite Intervals) in 12 Minutes

Improper Integrals - Infinite Bounds

Improper Integrals - Convergent or Divergent (Made Easy)

Improper Integrals BC Calc

AP Calculus BC Lesson 6.13

Integral Test | MIT 18.01SC Single Variable Calculus, Fall 2010

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Improper IntegralsMathematicsDiscontinuityCalculusIntegralsEducationalTutorialVideoLimitsConvergence