Improper Integrals of Type I (Infinite Intervals) in 12 Minutes
TLDRIn this informative video, Wilson explains the process of evaluating three types of improper integrals. He begins by defining improper integrals and demonstrates how to convert them into limits of definite integrals. Wilson then works through the first integral, which diverges to infinity. The second integral involves the arctangent function and converges to pi/2. The third integral is split into two parts, with the anti-derivative found through a u-substitution. Wilson shows that both parts converge, leading to a final sum of zero. The video is a comprehensive guide to understanding improper integrals and their convergence, providing clear examples and explanations.
Takeaways
- 📖 Evaluating improper integrals involves using limits as one of the boundaries approaches infinity.
- 🔨 The process begins by expressing the improper integral as the limit of a definite integral from a finite boundary to a variable that approaches infinity.
- 📚 For the integral ∫1∞ (1/x dx), the anti-derivative is ln|x|, and the limit as the upper bound approaches infinity leads to the conclusion that the integral is divergent.
- 📝 When the lower limit is negative infinity, such as in ∫-∞0 (1/(1+x²) dx), the integral is evaluated by finding the limit of the anti-derivative (arctan(x)) as the lower limit approaches negative infinity.
- 📱 The tangent inverse function's behavior helps determine the limit, using its horizontal asymptotes at π/2 and -π/2.
- 🔍 For the integral with both limits at infinity, the integral is split into two parts at a convenient point, often zero, and evaluated separately.
- 📊 Substitution is a useful technique for finding the anti-derivative, especially in integrals involving e^(-x²), by setting u = -x².
- 📈 If either part of the integral from the previous step diverges, then the entire integral diverges; otherwise, the integral is convergent if both parts converge.
- 💰 The evaluation of each part involves applying the fundamental theorem of calculus and then taking the limit as the boundary approaches infinity or negative infinity.
- 🚀 The final value of the original integral is determined by summing the results of the two convergent parts, demonstrating whether the entire integral converges or diverges.
Q & A
What is the first type of improper integral discussed in the video?
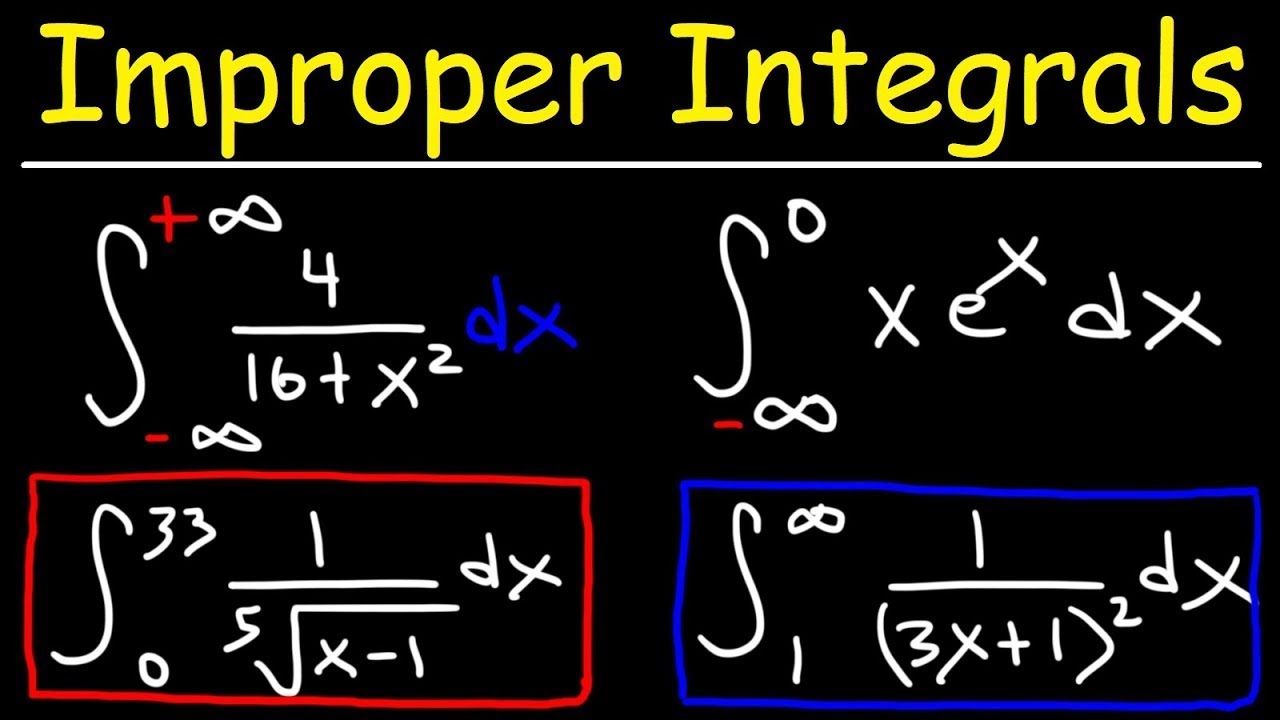
-The first type of improper integral discussed is one with an infinite interval, specifically from 1 to infinity.
How is an improper integral defined as a limit?
-An improper integral is defined as the limit of a definite integral. It is written as the limit of the definite integral from 1 to b as b approaches infinity.
What is the antiderivative of 1/x?
-The antiderivative of 1/x is the natural logarithm of the absolute value of x, denoted as ln|x|.
What is the result of the first improper integral example in the video?
-The result of the first improper integral example is infinity, indicating that the integral is divergent.
How does the second improper integral example differ from the first?
-The second improper integral example differs in that it has a lower limit of negative infinity and an upper limit of 0, and the integrand is 1/(1+x^2) instead of 1/x.
What is the antiderivative of 1/(1+x^2)?
-The antiderivative of 1/(1+x^2) is the arctangent of x, denoted as arctan(x).
What is the result of the second improper integral example?
-The result of the second improper integral example is pi/2, indicating that the integral is convergent.
How does the third improper integral example separate into two parts?
-The third improper integral example is separated into two parts: one from negative infinity to 0 with the integrand x*e^(-x^2), and the other from 0 to positive infinity with the same integrand but with x starting from 0.
What is the method used to find the antiderivative of x*e^(-x^2)?
-The method used is substitution, specifically u-substitution, where u is set to -x^2 and du is -2x dx, which simplifies the integral for further evaluation.
What are the results of the two parts of the third improper integral example?
-The first part evaluates to -1/2 and the second part evaluates to +1/2. When added together, the sum is zero, indicating that the original improper integral is convergent.
What is the final result of the third improper integral example?
-The final result of the third improper integral example is zero, confirming that the integral is convergent.
Outlines
📚 Introduction to Improper Integrals
The video begins with the host, Wilson, introducing the topic of improper integrals of the first type, which involve infinite intervals such as from 1 to infinity. Wilson explains that the approach to solving these integrals is to use the definition of the improper integral as a limit of a definite integral. The example provided involves the integral of 1/x from 1 to infinity. Wilson demonstrates the process of evaluating the definite integral, taking the limit as the upper limit approaches infinity, and using the fundamental theorem of calculus to find that the value of the improper integral is infinity, indicating divergence.
📈 Evaluating Improper Integrals with Limits
In this segment, Wilson continues the discussion on improper integrals by presenting another example with a lower limit of negative infinity and an upper limit of zero. The integrand is 1/(1+x^2), and Wilson uses the definition of the improper integral to express it as a limit. He integrates the function to obtain the arctangent function and evaluates it from a to 0, noting that the arctangent of 0 is 0. Wilson then discusses the limit as a approaches negative infinity, using a sketch of the arctangent function to illustrate the behavior of the function and determine that the limit is pi/2. This indicates that the improper integral is convergent.
🔢 Convergence and Divergence of Combined Improper Integrals
The final part of the video script involves a more complex improper integral with limits from negative infinity to positive infinity and the integrand xe^(-x^2). Wilson breaks down the integral into two parts for easier computation, choosing zero as a convenient point to separate the integral. He explains the process of finding the antiderivative of the integrand and emphasizes that if both integrals converge, the original improper integral converges, but if one diverges, so does the original. Wilson uses substitution to find the antiderivative and then evaluates the definite integrals for both parts, ultimately finding that both are convergent. He concludes by adding the values of the two integrals to find the value of the original improper integral, which is zero, confirming its convergence.
Mindmap
Keywords
💡Improper Integrals
💡Definite Integral
💡Limit
💡Anti-Derivative
💡Fundamental Theorem of Calculus
💡Convergence
💡Divergence
💡Arctangent Function
💡Asymptotes
💡Separation of Integrals
💡Integration by Substitution
Highlights
Introduction to evaluating improper integrals of type one with infinite intervals.
Using the definition of improper integrals as limits of definite integrals to approach infinity.
Integration of 1/x to find the anti-derivative and application of the fundamental theorem of calculus.
Determination that the improper integral of 1/x from 1 to infinity diverges, resulting in infinity.
Second problem with lower limit as negative infinity and upper limit as zero, using the same definition approach.
Integration of 1/(1+x^2) resulting in the arctangent function and evaluation of its limit as x approaches zero.
Calculation of the limit for the improper integral with lower limit as negative infinity, yielding pi/2.
Third problem with limits from negative infinity to positive infinity, splitting the integral into two parts for easier computation.
Anti-derivative found for xe^(-x^2) and its application in evaluating both improper integrals.
First part of the third integral evaluates to negative 1/2 as x approaches negative infinity.
Second part of the third integral also converges to 1/2 as x approaches infinity.
Summation of the results from both parts of the third integral, showing the original improper integral converges to zero.
Explanation of the process for determining convergence or divergence of improper integrals with infinite intervals.
Use of u-substitution in integration to simplify the process and find the anti-derivative.
Visual aid suggestion through sketching the tangent inverse function to better understand the limit behavior.
Final summary stating that all three discussed improper integrals are convergent, with the third integral summing to zero.
Encouragement for viewers to subscribe for more content on evaluating improper integrals and related mathematical concepts.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: