Tests for Convergence
TLDRThis educational video explores various tests to determine the convergence or divergence of infinite series, focusing on the harmonic series and its divergence. The presenter uses direct comparison, the integral test, and the p-series test to demonstrate divergence, comparing series to known convergent or divergent ones. Additionally, the limit comparison test is introduced, showing how series with the same behavior can be identified through the ratio of their terms. The video aims to provide a conceptual understanding of these tests, with examples to solidify the learning.
Takeaways
- 📚 The harmonic series, which is the sum of 1/n, diverges. This is a fundamental concept explained in the video.
- 🔍 Two methods are used to prove the divergence of the harmonic series: direct reasoning and comparison with another series (R).
- 📉 The series R is constructed to show that it diverges and is used as a comparison to demonstrate that the harmonic series (S) diverges as well.
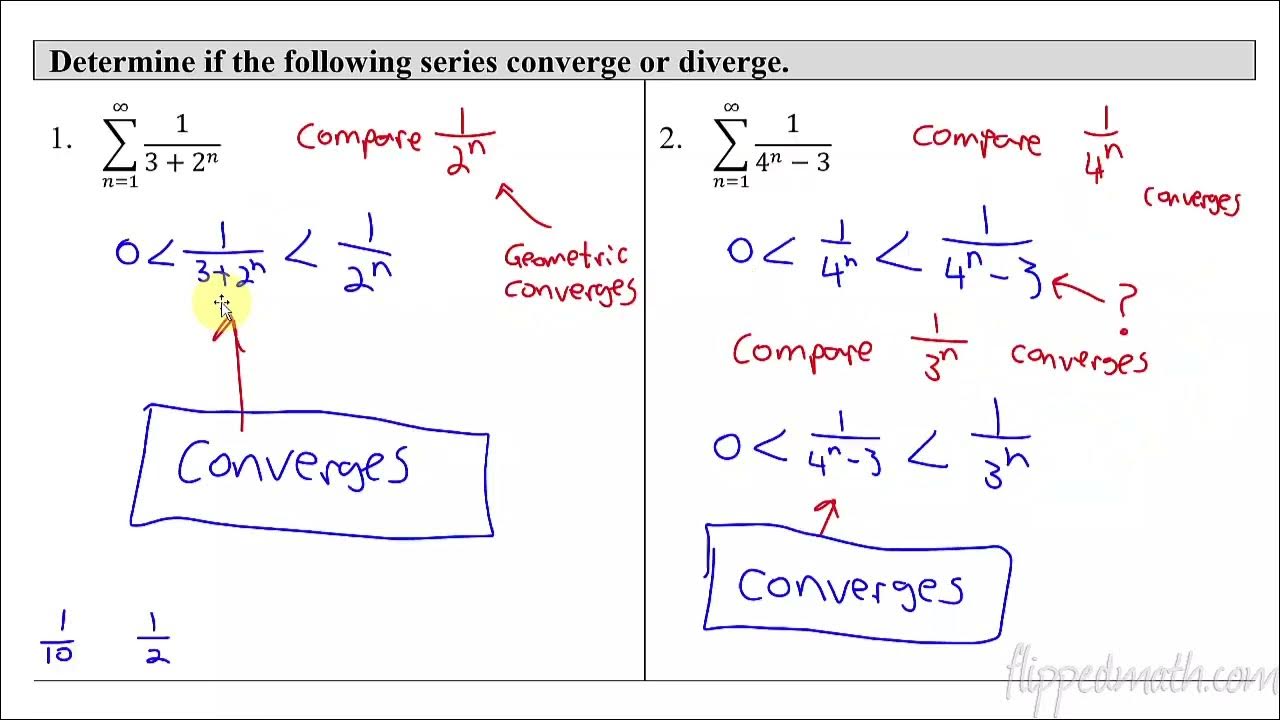
- 🌐 The direct comparison test states that if a series has terms smaller than another convergent series, it also converges; if terms are larger than a divergent series, it also diverges.
- 📊 The video demonstrates using the direct comparison test to show that the sum of 1/√n diverges by comparing it to the known divergent harmonic series.
- 🔢 The integral test is introduced as a method to determine convergence by comparing a series to its corresponding improper integral.
- 📚 The integral test is applicable to series with positive, decreasing terms and a continuous defining function, and it's used to show the divergence of the harmonic series.
- 📈 The p-series test is explained, which determines convergence based on the power of n in the denominator being greater than 1.
- 🔄 The limit comparison test is presented, which compares the ratio of two series to determine if they share the same convergence behavior, provided the limit of the ratio is positive and finite.
- 📘 Examples are given to apply the p-series and limit comparison tests to determine the convergence or divergence of various series.
- 🚫 The script emphasizes that the limit comparison test cannot be used to infer opposite behaviors if the limit is not positive and finite.
Q & A
What is the harmonic series and why does it diverge?
-The harmonic series is the sum of the reciprocals of the natural numbers, represented as S = 1 + 1/2 + 1/3 + 1/4 + ... The script explains that it diverges because it can be shown to be larger than another series (R) which also diverges, and by the principle of direct comparison, if a series is larger than a divergent series, it must also diverge.
What is the direct comparison test and how is it used to determine convergence or divergence of a series?
-The direct comparison test is a method used to determine whether a series converges or diverges by comparing it to another series whose behavior is already known. If the terms of the series in question are smaller than the terms of a convergent series, then it also converges. Conversely, if the terms are larger than a divergent series, then it also diverges.
How does the script use the series R to show the divergence of the harmonic series?
-The script introduces series R, which is constructed in such a way that it is clearly divergent. Then, by comparing the terms of the harmonic series (S) with R and showing that each term of S is greater than the corresponding term of R, it concludes that since R diverges and S is larger, S must also diverge.
What is the integral test and how does it relate to the convergence of a series?
-The integral test is a method to determine the convergence of a series with positive, decreasing terms. It states that the series converges if and only if the corresponding improper integral converges. The test connects the behavior of the series to the behavior of the integral of the function that defines the terms of the series.
Can you provide an example of using the integral test as demonstrated in the script?
-The script uses the integral test on the harmonic series. It compares the sum of 1/n to the improper integral from 1 to infinity of 1/x. Since the integral of 1/x diverges (as the natural logarithm of x approaches infinity), the harmonic series also diverges according to the integral test.
What is a geometric series and how does the script show that a certain series converges by comparing it to a geometric series?
-A geometric series is a series where each term is a constant ratio times the previous term. The script shows that the sum of 1/(2^n + n) converges by comparing it to the geometric series 1/2^n, which is known to converge. Since the terms of the given series are less than the corresponding terms of the geometric series, it also converges.
What is the p-series test and how does it determine the convergence of a series?
-The p-series test applies to series of the form 1/n^p. It states that the series converges if the power p is greater than 1, and diverges if p is less than or equal to 1. The script uses this test to quickly determine the convergence or divergence of series based on the value of p.
How does the script use the limit comparison test to determine the convergence or divergence of a series?
-The limit comparison test involves taking the limit of the ratio of the terms of two series. If this limit is positive and finite, and one series is known to converge or diverge, then the other series behaves the same way. The script uses this test to compare unknown series with known series to determine their behavior.
What is the difference between the direct comparison test and the limit comparison test?
-The direct comparison test compares the terms of two series directly, while the limit comparison test compares the limit of the ratio of corresponding terms as n approaches infinity. The direct comparison test can be used when one series is known to be larger or smaller than another, whereas the limit comparison test requires a known series for comparison and a positive, finite limit of the ratio.
Can you explain the concept of an improper integral and how it is used in the integral test?
-An improper integral is an integral that has an infinite limit of integration, an integrand that is unbounded, or both. In the integral test, the improper integral of the function defining the terms of the series is compared to the series itself. If the integral converges, so does the series, and if it diverges, the series does as well.
What are some series that the script suggests for the viewer to try on their own using the integral test?
-The script suggests trying the integral test on series such as 1/n^(1/2) and 1/n^(3/2). The viewer is encouraged to compute the corresponding improper integrals and determine whether they converge or diverge to understand the convergence of the series.
Outlines
📚 Introduction to Series Convergence Tests
This paragraph introduces the topic of the video, which is to demonstrate various tests for determining whether an infinite series converges or diverges. The presenter begins with a discussion on the harmonic series, a well-known divergent series, and promises to provide two distinct proofs of its divergence. The first method involves a direct comparison with another series, 'r', which is constructed to show that the harmonic series is greater and thus also diverges. The second method is the direct comparison test, which states that if a series has terms larger than a known convergent series, it must also diverge.
🔍 Direct Comparison Test and Series Convergence
In this paragraph, the presenter explains the direct comparison test in more detail, providing the mathematical theorem and its implications for series with positive terms. The test is used to show that a series 's', which is greater than a known divergent series 'h', must also diverge. The presenter then applies the direct comparison principle to demonstrate that the series of 1 over the square root of 'n' diverges by comparing it to the already established divergent harmonic series. The paragraph concludes with an example of using the direct comparison test to show convergence, using the sum of 1 over 2 to the power of 'n' as a reference.
📈 The Integral Test for Series Convergence
The presenter introduces the integral test, which is applicable to series with positive and decreasing terms. The test connects the convergence of a series to the convergence of the corresponding improper integral. The presenter does not provide a proof but instead offers to show it in a separate video. Examples are given to illustrate the use of the integral test, including an application to the harmonic series, where the corresponding integral is shown to diverge, confirming the series' divergence. The paragraph also includes a challenge for the viewer to apply the integral test to determine the convergence of other series.
📉 The p-Series Test and Limit Comparison Test
This paragraph discusses two additional tests for series convergence: the p-series test and the limit comparison test. The p-series test states that a series of the form 1 over 'n' to the power of 'p' converges if 'p' is greater than 1. The presenter provides examples to demonstrate the application of this test. The limit comparison test is then introduced, which compares the ratio of the terms of two series to determine their convergence behavior. The test is applied to examples, showing how a positive and finite limit indicates similar convergence properties between the series being compared. The paragraph concludes with a note on the limitations of the limit comparison test and a prompt for the viewer to try additional examples.
🏁 Conclusion and Further Practice
In the final paragraph, the presenter wraps up the video by encouraging viewers to practice the tests on their own with additional examples provided. The focus is on understanding the differences in the powers of the terms in the series and applying the appropriate tests to determine convergence or divergence. The presenter thanks the viewers for watching and provides a final set of examples for further practice.
Mindmap
Keywords
💡Infinite Series
💡Harmonic Series
💡Convergence
💡Divergence
💡Direct Comparison Test
💡Integral Test
💡P-Series Test
💡Limit Comparison Test
💡Geometric Series
💡Improper Integral
Highlights
Introduction to the harmonic series and its divergence.
Explanation of the harmonic series as the sum of one over n.
Introduction of series R to demonstrate divergence of the harmonic series.
Comparison of series S and R to show that S is larger than R.
Conclusion that the harmonic series diverges using direct comparison.
Introduction to the direct comparison theorem in series analysis.
Application of direct comparison to show divergence of the sum of 1/√n.
Explanation of the principle that smaller terms in a series lead to convergence.
Demonstration of using a known convergent series to prove convergence of another series.
Introduction to the integral test for series convergence.
Explanation of the integral test and its connection to the series.
Use of the integral test to demonstrate divergence of the harmonic series.
Introduction to the p-series test and its criteria for convergence.
Examples of applying the p-series test to determine series convergence.
Introduction to the limit comparison test for series analysis.
Explanation of how the limit comparison test works and its limitations.
Examples of using the limit comparison test to determine series convergence or divergence.
Summary of the various tests for series convergence and divergence discussed in the video.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: