Alternating Series Error Bound

RH Mathematics
18 Feb 202412:51
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video delves into the concept of alternating series error bounds, focusing on how to approximate values such as the natural logarithm of two using power series. It explains the process of determining if a series converges using the alternating series test and how to find the error in approximations. The video illustrates the error bound theorem, showing that the error is less than or equal to the absolute value of the next term in the series. It also provides examples and strategies for applying these concepts in AP Calculus, including multiple-choice and free-response questions, to solidify understanding.

Takeaways
  • πŸ“š The video discusses the concept of 'error' in the context of alternating series and how it relates to the approximation of values like the natural log of two.
  • πŸ” It explains the process of applying the alternating series test to determine if a series converges and whether it is absolutely or conditionally convergent.
  • πŸ“ˆ The script uses a visual approach to demonstrate how the partial sums of an alternating series converge to a specific value, using the natural log of two as an example.
  • πŸ“‰ The concept of 'error' is illustrated through the difference between the partial sums and the actual value of the series, with the error decreasing as more terms are added.
  • πŸ“ The video introduces the theorem for the alternating series error bound, which states that the absolute value of the difference between the series sum and a partial sum is no more than the absolute value of the next term.
  • πŸ”’ The importance of verifying the convergence of a series before applying the error bound is emphasized, as it is a prerequisite for using the error bound.
  • πŸ“Š The script provides examples of how to calculate the error bound for a given series and how to interpret the results, including a multiple-choice question to test understanding.
  • πŸ€” The video encourages viewers to approach problems carefully, especially when determining the number of terms needed to achieve a specific error threshold.
  • πŸ“š It also reviews recent pre-response problems from AP Calculus exams, showing how to apply the alternating series error bound in different scenarios.
  • πŸ“ The video concludes with a reminder of the importance of understanding the alternating series test, absolute and conditional convergence, and the error bound for effective problem-solving in calculus.
  • πŸ‘ The presenter thanks the viewers for watching and encourages them to practice applying these concepts to become proficient.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is the investigation of alternating series, specifically focusing on the concept of the alternating series error bound.

  • What is an alternating series test?

    -The alternating series test is a method used to determine if an alternating series converges. It checks if the absolute values of the terms of the series decrease and approach zero, and if the series is alternating.

  • How can we determine if a series is absolutely or conditionally convergent?

    -We can determine if a series is absolutely or conditionally convergent by applying the alternating series test and other convergence tests, and by examining the behavior of the series' terms.

  • What is the natural log of 2 approximated by?

    -The natural log of 2 is approximated by the alternating harmonic series, which is the sum of the series from 1 to infinity of (-1)^(n+1) * (1/n).

  • What is the concept of 'error' in the context of this video?

    -In this context, 'error' refers to the difference between the approximated value of a series using a finite number of terms and the actual value that the series converges to.

  • What is the alternating series error bound theorem?

    -The alternating series error bound theorem states that for an alternating series that converges to S, the absolute value of the difference between S and a partial sum is no more than the absolute value of the next term in the series.

  • How can we use the alternating series error bound in AP Calculus?

    -In AP Calculus, the alternating series error bound can be used to estimate how close an approximation of a series is to the actual value by comparing it to the absolute value of the next term in the series.

  • What is the significance of the movement between the black points in the video?

    -The movement between the black points signifies the change in the partial sum of the series, indicating how much the approximation changes with the addition of each term.

  • How does the error change as more terms are added to the series?

    -As more terms are added to the series, the error generally decreases, getting closer to the actual value that the series converges to.

  • What is the strategy for determining how many terms are needed to achieve a specific error threshold?

    -The strategy involves calculating the absolute value of the terms of the series sequentially until the term's absolute value is less than the desired error threshold, indicating that using that many terms will result in an error below the threshold.

  • Can you provide an example of how to apply the alternating series error bound to a problem?

    -An example would be to use the first nine terms of the alternating harmonic series to approximate the natural log of 2. The error bound would be the absolute value of the tenth term, which is 1/10, indicating the approximation is within an error of 0.1.

Outlines
00:00
πŸ“š Introduction to Alternating Series Error Bound

This paragraph introduces the concept of error in the context of alternating series. It discusses the ability to determine convergence and the type of convergence (absolute or conditional) using the alternating series test. The natural log of two is used as an example to illustrate how the series can be approximated by partial sums, and how the error of these approximations decreases as more terms are included. The paragraph emphasizes the importance of understanding the movement between partial sums and the true value, setting the stage for the discussion of the alternating series error bound.

05:01
πŸ” Applying the Alternating Series Error Bound

The second paragraph delves into the application of the alternating series error bound. It uses the alternating harmonic series as an example to demonstrate how to calculate the error bound after approximating a series with a finite number of terms. The paragraph explains that the error is no more than the absolute value of the next term not included in the partial sum. It also addresses a multiple-choice question about determining the smallest number M that guarantees the error is less than M when using only three terms of the series, concluding with the correct answer of 1/19.

10:02
πŸ“‰ Error Analysis and Series Convergence Verification

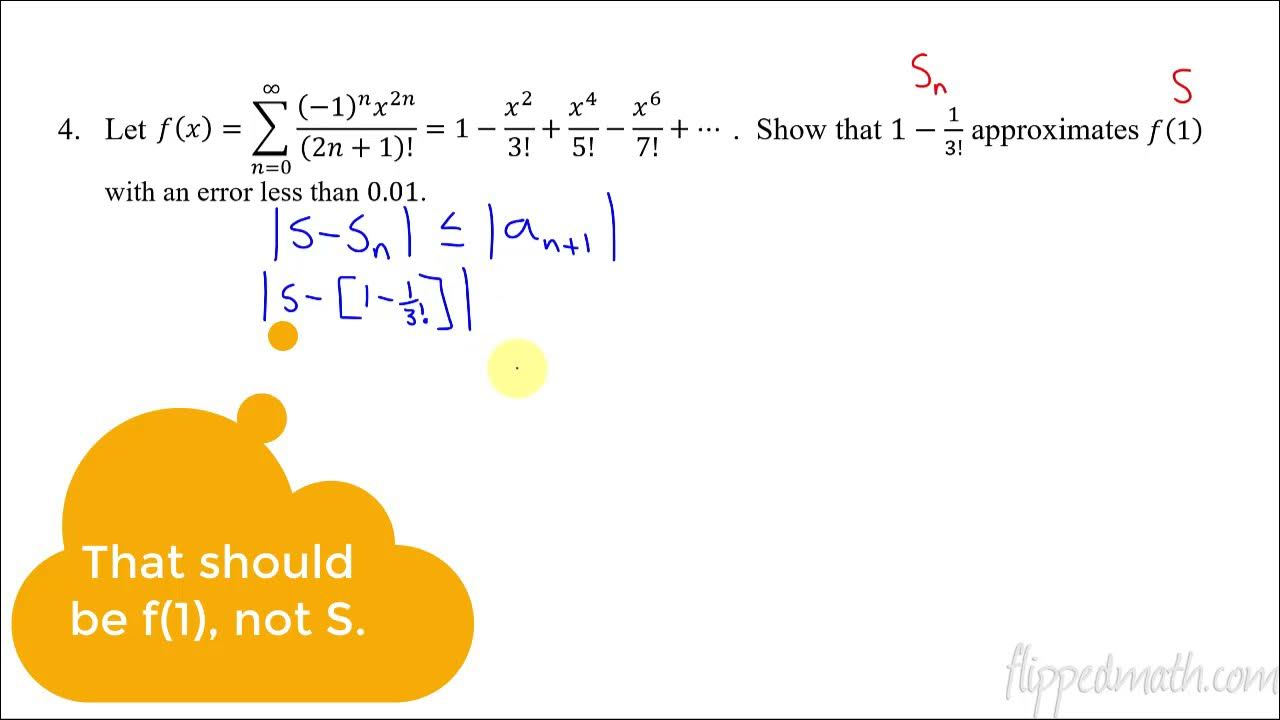
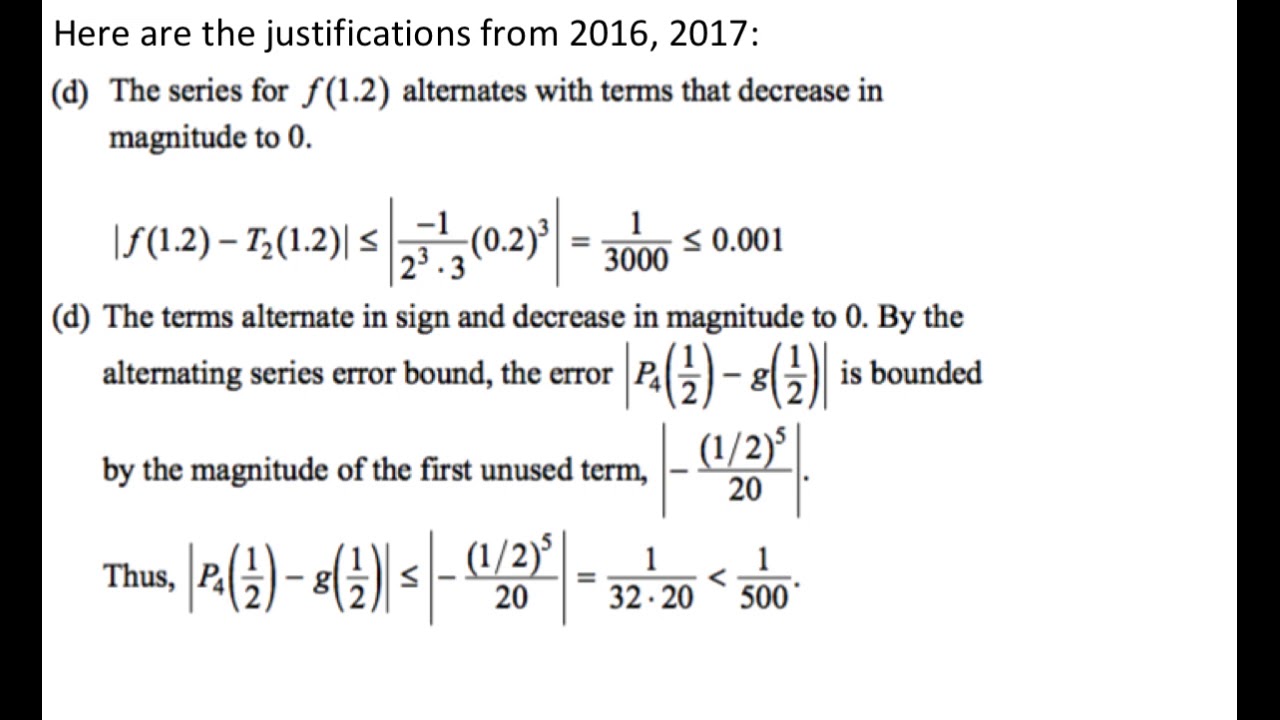
This paragraph focuses on the analysis of error in series approximation and the importance of verifying series convergence before applying the alternating series error bound. It provides a step-by-step explanation of how to determine the number of terms needed to achieve a specific error threshold, using the alternating series as an example. The paragraph also includes a discussion of a pre-response problem from 2022, which tests the understanding of the error bound in the context of a power series, and demonstrates the process of showing that the absolute value of the difference between the function evaluated at a certain point and its approximation is less than a given value.

πŸ“˜ Examples and Conclusion on Alternating Series Error Bound

The final paragraph presents examples to further illustrate the application of the alternating series error bound, including a free-response problem that requires verification of series convergence and calculation of the error bound using the first two terms of a series. It emphasizes the need to verify the convergence of the series and to understand the decreasing magnitude of terms towards zero. The paragraph concludes with a summary of the key points discussed in the video, highlighting the importance of the alternating series test, absolute and conditional convergence, and the error bound in the study of calculus.

Mindmap
Keywords
πŸ’‘Alternating Series
An alternating series is a series where the terms alternate in sign, typically represented as a sum of (-1)^n times a sequence of terms. In the video, the concept is central to the discussion of convergence and error bounds. For instance, the natural log of two is approximated using an alternating harmonic series, which is a specific type of alternating series with terms that involve the harmonic progression.
πŸ’‘Error Bound
In the context of the video, an error bound is a value that provides an upper limit on the error when approximating the sum of an infinite series with a finite number of its terms. The script discusses the concept in relation to alternating series, stating that the error in approximation is less than or equal to the absolute value of the next term in the series.
πŸ’‘Convergence
Convergence refers to the property of a series where the sum of its terms approaches a finite limit as more terms are added. The video script mentions that to apply the alternating series error bound, one must first verify the convergence of the series using the alternating series test, which is a method to determine if an alternating series converges.
πŸ’‘Alternating Series Test
The alternating series test is a specific test used to determine the convergence of an alternating series. The script explains that for a series to pass this test, its terms must decrease in magnitude and approach zero as the number of terms increases, which is demonstrated with the alternating harmonic series.
πŸ’‘Partial Sums
Partial sums are the sums of the first n terms of a series. The video script uses the concept of partial sums to illustrate how the approximation of the natural log of two improves as more terms of the alternating series are included, with each additional term reducing the error of the approximation.
πŸ’‘Natural Logarithm
The natural logarithm, often denoted as ln(x), is the logarithm of a number to the base e, where e is an irrational constant approximately equal to 2.71828. In the video, the natural log of two is approximated using a power series, which is a specific application of the concept in the context of alternating series.
πŸ’‘Power Series
A power series is an infinite series of the form Ξ£a_n * (x - c)^n, where a_n are the coefficients and c is a constant. The script discusses a specific power series for the natural logarithm of (1 + x), which when x is substituted with 1, becomes an alternating harmonic series converging to the natural log of two.
πŸ’‘Absolute Value
The absolute value of a number is its distance from zero on the number line, disregarding its sign. In the script, the absolute value is used when discussing error bounds, ensuring that the magnitude of the error is considered, regardless of whether it is positive or negative.
πŸ’‘Free Response
Free response refers to open-ended questions that require a more elaborate answer, often found in exams like the AP Calculus exam. The script includes examples of free response problems that test the understanding of alternating series, convergence, and error bounds.
πŸ’‘AP Calculus
AP Calculus is a high school course and exam that covers advanced topics in calculus, including series and convergence. The video script provides examples and explanations relevant to students preparing for the AP Calculus exam, particularly in the context of understanding and applying the concepts of alternating series and error bounds.
Highlights

Introduction to the concept of 'error' in the context of alternating series.

Explanation of how the alternating series test can determine convergence and absolute or conditional convergence of a series.

Demonstration of approximating the natural log of two using a power series.

Illustration of partial sums and their convergence to the natural log of two.

Introduction of the alternating series error bound theorem.

The error bound theorem states that the absolute value of the difference between the series sum and a partial sum is no more than the absolute value of the next term.

Verification of series convergence is necessary before applying the alternating series error bound.

Example of using the first nine terms of an alternating series to approximate a value and determine the error bound.

Explanation of how the error bound is determined by the absolute value of the term not included in the partial sum.

A multiple-choice question example involving determining the smallest number M for an error bound using three terms of a series.

Discussion on how many terms are needed to achieve an error less than one over 500.

Advice on being careful with calculations and not making off-by-one errors.

Example from a 2022 problem involving showing the absolute value difference between F of a half and a half is less than a 10.

Explanation of how to use the alternating series error bound in a free-response scenario.

Another example of using the first two terms of a series to approximate a function and determine an upper bound on the error.

Emphasis on verifying the convergence of the series before applying the alternating series error bound.

Final thoughts on the importance of breaking down complex topics into manageable chunks for better understanding and practice.

Transcripts

Browse More Related Video

AP Calculus BC Lesson 10.10

Alternating Series Day 2

Alternating series remainder | Series | AP Calculus BC | Khan Academy

Calculus BC – 10.10 Alternating Series Error Bound

Calculus Chapter 5 Lecture 56 Approximation & Error

Review of Lagrange Error Bound and Alternating Series Error for the BC Calculus Exam

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Alternating SeriesError BoundsCalculusConvergenceLogarithm ApproximationSeries TestNatural LogHarmonic SeriesPartial SumsAP Calculus