Review of Lagrange Error Bound and Alternating Series Error for the BC Calculus Exam

turksvids
13 Apr 201915:09
EducationalLearning
32 Likes 10 Comments

TLDRThis video tutorial reviews the handling of errors in power series, specifically Taylor and Maclaurin series, which are crucial for the AP Calculus BC exam. It covers the two main types of error: alternating series error and the Lagrange error bound, explaining how each appears on the exam and how to approach them. The video highlights the importance of recognizing patterns in exam questions and provides detailed examples and justifications for calculating errors, ensuring viewers are well-prepared for this topic.

Takeaways
  • 📚 Alternating series error and Lagrange error bound are essential topics for the AP Calculus BC exam.
  • 📈 Alternating series error is more common in the released forms of the exam, while Lagrange error appears frequently in the secure, non-released forms.
  • 📝 When dealing with Lagrange error, look out for information about derivatives as it will guide you on how to approach the problem.
  • 🔍 Alternating series error often requires you to use the first few nonzero terms of the series and check for properties ensuring the approximation's accuracy.
  • 📉 To justify alternating series error, ensure the series is convergent and use the absolute value of the next term as the error bound.
  • 📊 The Lagrange error formula involves the maximum absolute value of the next derivative over a specific interval, centered around the point of approximation.
  • 🔢 Exam questions often provide information about the derivatives, which you will use to identify the maximum value needed for the Lagrange error formula.
  • ✍️ Using absolute value expressions is a consistent method for writing justifications for both types of error approximations.
  • 📋 The steps for handling alternating series error include showing convergence, identifying the term left off, and using absolute value expressions.
  • 🧮 For Lagrange error, ensure you understand the notation and process for finding the maximum value of the necessary derivative over the given interval.
Q & A

  • What are the two types of errors discussed in the video script related to series approximations?

    -The two types of errors discussed are the alternating series error and the L'Hôpital's error bound, also known as the Lagrange error bound.

  • Which type of error is more common on the released AP Calculus BC exams?

    -The alternating series error is more common on the released AP Calculus BC exams.

  • What is the significance of the absolute value expression in the context of series approximations?

    -The absolute value expression is used to describe the error of an approximation, showing the difference between the actual function value and the polynomial approximation.

  • How does the video script suggest determining whether to use the alternating series error or the L'Hôpital's error bound in a problem?

    -If the problem explicitly mentions an alternating series or provides information about a random derivative, it's likely to be an L'Hôpital's error bound problem. Otherwise, it's probably an alternating series error.

  • What is the formula for the error in approximating a convergent alternating series with its first n terms?

    -The error is the magnitude or absolute value of its (n + 1)th term.

  • How is the L'Hôpital's error bound formula structured?

    -The L'Hôpital's error bound formula is structured as M times the absolute value of (a - C) to the power of (n + 1), all divided by (n + 1) factorial, where M is the maximum of the absolute value of the (n + 1)th derivative on the interval from a to C or C to a.

  • What is the importance of recognizing the absolute value expression in exam questions?

    -Recognizing the absolute value expression is important because it shows up in exam questions and is a good way to write justifications when asked to find the error of an approximation.

  • What does the video script suggest for justifying the error in an alternating series approximation?

    -The script suggests establishing that the series is convergent, identifying the first term that was left off (which is the maximum possible error), and using the absolute value expression to show the error is less than a certain value.

  • Can the maximum value of the nth derivative (M in the L'Hôpital's error bound formula) be assumed or must it be given in the problem?

    -The maximum value of the nth derivative (M) is typically given in the problem because it is necessary for solving the problem and cannot be reasonably assumed.

  • How does the video script illustrate the process of using the L'Hôpital's error bound in exam questions?

    -The script provides examples from past exams, showing how the maximum value of the nth derivative is given and how to plug it into the L'Hôpital's error bound formula to find the error of an approximation.

  • What is the advice given in the video script for students preparing for the AP Calculus BC exam regarding the topic of series approximation errors?

    -The advice is to thoroughly understand both the alternating series error and the L'Hôpital's error bound, as they are frequently tested on the AP Calculus BC exam and are crucial for success.

Outlines
00:00
📚 Introduction to Error in Series Approximations

This paragraph introduces the topic of error handling in power or Taylor/Maclaurin series, specifically in the context of the AP Calculus BC exam. The speaker discusses two types of errors: alternating series error and L'Orange error bound. The paragraph highlights the frequency of these topics on released exams since 2012 and emphasizes the importance of understanding these concepts for exam success. It also previews the types of questions that might be asked, such as using L'Orange bounds to show certain properties or dealing with alternating series errors to estimate values within specific margins of error.

05:01
🔍 Exploring L'Orange Error Bound and Alternating Series Error

The speaker delves into the specifics of L'Orange error bound, providing examples of how it has been tested on past exams and the kind of information that might be provided to solve related problems. The paragraph also explains how to identify when to use L'Orange error as opposed to alternating series error, based on the information given in the exam questions. The explanation includes how to work with absolute value expressions to estimate errors and justify answers, with references to specific past exam questions and their solutions.

10:02
📘 Understanding and Calculating Series Errors

This paragraph focuses on the mechanics of calculating errors in series approximations. It explains the process for determining the error in an alternating series, which involves using the absolute value of the first term omitted from the series. The speaker provides a step-by-step approach to justifying the error, including establishing convergence, identifying the term that represents the maximum possible error, and using absolute value expressions in explanations. Examples from past exams are given to illustrate how these concepts are applied in solving problems.

15:02
📘 Further Insight into L'Orange Error and Practical Examples

The speaker continues the discussion on L'Orange error, explaining its formula and the components involved, such as the center of the series, the value being approximated, and the maximum of the absolute value of the nth derivative. The paragraph provides practical examples from past exams, demonstrating how the maximum value (M) is identified and used in the error formula. It also shows how to write justifications for the error using proper notation and how to plug in values to estimate the error in function approximations.

🎓 Conclusion and Encouragement for Exam Preparation

In the final paragraph, the speaker wraps up the discussion on series errors, expressing hope that the detailed explanation was helpful for exam preparation. The paragraph serves as a conclusion, summarizing the importance of understanding error calculations in series approximations for the AP Calculus BC exam and wishing the viewers good luck in their studies and exam.

Mindmap
Keywords
💡Error
In the context of the video, 'error' refers to the discrepancy between the value of an approximation made by a power, Taylor, or Maclaurin series and the actual value of the function being approximated. The video discusses two types of errors: the alternating series error and the L'Hôpital's rule error bound, which are crucial for understanding the theme of the video as it prepares students for the AP Calculus BC exam.
💡Alternating Series Error
The 'alternating series error' is the error associated with approximating a convergent alternating series using its first few terms. The video explains that the error is the absolute value of the first term that was not included in the approximation. This concept is vital for the video's theme as it is a common topic on the AP Calculus BC exam, and the script provides examples from various exam years where this concept is tested.
💡L'Hôpital's Rule Error Bound
The 'L'Hôpital's rule error bound' is used when dealing with Taylor or Maclaurin series. It provides an upper limit for the error when using a polynomial of a certain degree to approximate a function. The video script mentions that this error bound is less common on released exams but still important, as it has appeared in exams such as those from 2004, 2008, and 2011.
💡AP Calculus BC Exam
The 'AP Calculus BC exam' is a standardized test that the video is preparing viewers for. It is an advanced placement exam offered by the College Board, and the video focuses on specific topics within calculus that are likely to appear on this exam, such as error analysis in series approximations.
💡Taylor Series
A 'Taylor series' is a representation of a function as an infinite sum of terms calculated from the values of the function's derivatives at a single point. The video discusses how errors in approximations using Taylor series can be analyzed and is a key concept as it is a fundamental tool in calculus for understanding functions and their approximations.
💡Maclaurin Series
A 'Maclaurin series' is a special case of a Taylor series where the point about which the function is expanded is zero. The video mentions this as part of the broader discussion on series approximations and errors, indicating its relevance to the exam content.
💡Absolute Value
The 'absolute value' is used in the video to describe the magnitude of the error without considering its sign. It is a mathematical operation that is crucial in defining both the alternating series error and the L'Hôpital's rule error bound, as it helps to quantify the maximum possible error in the approximation.
💡Convergent
'Convergent' refers to a series that approaches a finite sum as more terms are added. In the video, establishing that a series is convergent is a necessary step before discussing the error of its approximation. The script provides examples where the convergence of a series is assumed or proven to justify the use of certain approximation techniques.
💡Derivative
A 'derivative' in calculus represents the rate at which a function changes with respect to its variable. The video discusses the importance of derivatives in constructing Taylor and Maclaurin series and in calculating the error bounds for these approximations. The script mentions specific derivatives, such as the fourth or fifth, in the context of error analysis.
💡Polynomial Approximation
In the video, 'polynomial approximation' refers to the use of polynomials, which are expressions involving a finite number of terms with non-negative integer exponents, to approximate more complex functions. The script explains how the error of these approximations can be calculated and bounded, which is a central theme of the video.
Highlights

The video discusses handling errors in power or Taylor/Maclaurin series, particularly for the AP Calculus BC exam.

Two types of errors are covered: alternating series error and L'Orange error bound.

Alternating series error is more common on released exams, while L'Orange error is prevalent in secure exams.

L'Orange error bound has appeared in exams from 2004, 2008, 2011, and others.

Alternating series error is frequently tested in exams from 2006 to 2018.

Errors are important in both free response and multiple-choice sections of the exam.

The video explains how to identify when to use the Lagrangian or alternating series based on exam questions.

L'Orange error involves using the nth derivative to construct a polynomial and estimating the error.

Alternating series error is calculated by the magnitude of the (n+1)th term of a convergent series.

Justification for alternating series error involves proving convergence and identifying the first omitted term.

L'Orange error requires finding the maximum of the absolute value of the (n+1)th derivative on the interval.

Exam questions often provide the maximum value needed for L'Orange error calculations.

The video provides examples of how to justify and calculate errors for both types of series.

Absolute value expressions are crucial for writing justifications in error calculations.

The video demonstrates step-by-step solutions for error calculations using past exam questions.

Understanding the error calculation process is essential for success in the AP Calculus BC exam.

The video concludes with a summary of error handling on the exam and encouragement for exam preparation.

Transcripts

Browse More Related Video

7 | FRQ (No Calculator) | Practice Sessions | AP Calculus BC

AP Calculus BC 2023 Taylor Series FRQs: What to Look For?

Alternating Series Error Bound

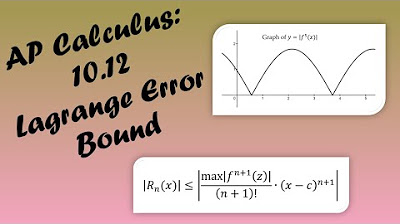
AP Calculus BC Lesson 10.12

AP Calculus BC Multiple Choice Practice Test (2016 AP CED Problems)

Avon High School - AP Calculus BC - Topic 10.12 - Example 3

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
AP CalculusError BoundsTaylor SeriesMaclaurin SeriesLagrange ErrorAlternating SeriesMath Exam PrepCalculus TipsAP ExamSeries Convergence