2.4 Units and Conversions | High School Chemistry
TLDRThis chemistry lesson focuses on units and conversions, essential for scientific calculations. It covers the metric system, SI units, and the importance of using consistent units to avoid errors, exemplified by the Mars rover mishap. The instructor explains the process of dimensional analysis for converting units, including length, volume, area, and temperature, and emphasizes the significance of prefixes and memorization in mastering conversions. Practical examples and step-by-step calculations are provided for clarity, with a special note on density calculations for substances, highlighting the concept of intensive properties.
Takeaways
- 📚 The lesson focuses on units and conversions, including metric conversions and dimensional analysis, which are essential in chemistry.
- 🔍 The metric system is based on powers of ten, making calculations easier compared to other systems like the US customary units.
- 👑 The speaker humorously points out that not using the metric system can lead to confusion and errors, referencing the Mars rover incident as an example.
- 🌟 SI units are the standard in science, with the meter for length, kilogram for mass, second for time, and kelvin for temperature being the fundamental units.
- 🔢 The script explains the conversion between Celsius and Kelvin, emphasizing the simplicity of adding 273.15 to Celsius to get Kelvin.
- 📉 The importance of significant figures in scientific calculations is highlighted, with a guide on how to maintain the correct number of significant figures during conversions.
- 🔄 Detailed steps are provided for converting between different metric units, including the use of prefixes like kilo, centi, and milli.
- 📏 The script clarifies the difference between SI units for volume, such as cubic meters, and more commonly used units like liters.
- 📐 Special attention is given to conversions involving area and volume, where squaring or cubing the conversion factors is necessary to maintain unit consistency.
- 🚀 The lesson touches on the concept of density, explaining it as an intensive property that remains constant regardless of the size of the sample.
- 🧊 A practical example is given to calculate the density of a substance and how to find the mass of a larger cube of the same substance using the previously calculated density.
Q & A
What is the main topic of the final lesson in the measurement chapter of the high school chemistry playlist?
-The main topic of the final lesson is units and conversions, including metric conversions and dimensional analysis.
Why is the metric system considered more convenient for calculations?
-The metric system is considered more convenient for calculations because it is based on powers of ten, making it easier to convert between units and perform calculations.
What is the SI unit for length?
-The SI unit for length is the meter, represented by the lowercase letter 'm'.
Why is the conversion between Celsius and Kelvin considered simple?
-The conversion between Celsius and Kelvin is simple because it involves adding 273.15 to the Celsius temperature to get the Kelvin temperature, or subtracting 273.15 to convert from Kelvin to Celsius.
What is the significance of the Mars rover incident mentioned in the script?
-The Mars rover incident is used as an example of the importance of using consistent units in scientific calculations, as the confusion between metric and imperial units led to the failure of the mission.
What is the SI unit for temperature?
-The SI unit for temperature is Kelvin.
What is the difference between the SI unit for volume and the commonly used unit in chemistry?
-The SI unit for volume is the cubic meter (m³), while in chemistry, liters are more commonly used.
What is the purpose of the metric prefixes in the metric system?
-The purpose of the metric prefixes is to create multiples and submultiples of the base units, allowing for a wide range of measurements to be expressed conveniently.
How should one approach converting units within the metric system?
-When converting units within the metric system, one should use the appropriate conversion factor, placing the unit with the prefix over the unit without the prefix and multiplying by the corresponding power of ten.
What is the proper way to express the answer in significant figures after performing a conversion?
-The proper way to express the answer in significant figures is to consider only the significant figures of the original number before the conversion, ignoring the conversion factor for significant figures.
Why is it necessary to square or cube conversion factors when dealing with area or volume conversions?
-It is necessary to square or cube conversion factors when dealing with area or volume conversions because the units of measurement for these dimensions are squared or cubed (e.g., square meters for area, cubic meters for volume), and the conversion factor must reflect this to ensure proper unit cancellation.
What is the concept of density and how is it calculated?
-Density is a measure of mass per unit volume of a substance. It is calculated using the formula density = mass/volume. If given the mass and volume, one can solve for density, and if given the density and one of the other two variables, one can solve for the third.
How does the density of a substance remain the same regardless of the size of the sample?
-Density remains the same regardless of the size of the sample because it is an intensive property, meaning it is independent of the amount of substance present. Whether you have a small or large sample of the same substance, the mass per unit volume will be consistent.
What is the significance of the conversion factor 2.54 cm/inch in the context of the script?
-The conversion factor 2.54 cm/inch is significant because it is used to convert between imperial (U.S. customary) units and metric units. It is typically provided and not memorized, and it is used in the script to convert feet to centimeters.
Outlines
📚 Introduction to Units and Conversions
This paragraph introduces the topic of units and conversions in the context of a high school chemistry playlist. The speaker discusses the importance of understanding metric conversions, dimensional analysis, and the significance of the metric system. They highlight the convenience of the metric system, which is based on powers of ten, and contrast it with the less intuitive British Imperial system. The speaker also mentions a historical mishap involving a Mars rover due to unit conversion errors, emphasizing the importance of accurate unit conversions in scientific work.
🌐 Understanding the Metric System and SI Units
The speaker delves into the details of the metric system, explaining its foundation on prefixes that modify the base units by powers of ten. They introduce the seven fundamental SI units, including the meter for length, kilogram for mass, second for time, kelvin for temperature, mole for the amount of substance, ampere for electric current, and candela for luminous intensity. The paragraph also covers derived units like volume (cubic meter), energy (joule), and pressure (pascal), noting the differences in commonly used units in chemistry versus physics.
🔍 Metric System Prefixes and Their Powers of Ten
This paragraph focuses on the prefixes of the metric system and how they scale units. The speaker lists prefixes from peta (10^15) to atto (10^-18), explaining how they can be applied to base units to create new units. They discuss the practicality of using different prefixes for various measurement contexts, such as using kilometers for long distances and millimeters for small lengths. The speaker also emphasizes the need to memorize these prefixes and their corresponding powers of ten for effective unit conversion.
⚖️ Converting Units: From Inches to Feet and Beyond
The speaker demonstrates how to convert units, starting with a simple example of converting inches to feet. They explain the concept of dimensional analysis, where units are placed in the denominator to cancel out in the calculation. The paragraph also covers the importance of significant figures in conversions, ensuring that the result reflects the precision of the original measurement. Examples include converting 84 inches to feet and 2.5 feet to inches, highlighting the use of scientific notation for expressing results with the correct number of significant figures.
📏 Advanced Metric Conversions: Meters, Kilometers, and Beyond
This paragraph continues the discussion on unit conversions, focusing on the metric system. The speaker shows how to convert meters to kilometers and vice versa, emphasizing the correct use of prefixes and powers of ten. They also discuss the potential confusion in choosing between two methods of conversion and decide on a consistent approach based on the definition of prefixes. Examples include converting 1500 meters to kilometers and 32.1 kilometers to meters, illustrating the process of dimensional analysis and the importance of significant figures.
📏📏 Complex Metric Conversions: Area and Volume
The speaker addresses the more complex conversions involving area and volume. They explain the need to square or cube conversion factors when dealing with units of area (e.g., square centimeters to square meters) and volume (e.g., cubic centimeters to cubic meters). The paragraph highlights the common mistake of not squaring the conversion factor, leading to incorrect results. Examples include converting 1200 square centimeters to square meters and discussing the conversion of volume units.
🔗 Cross-System Conversions: US to Metric
This paragraph introduces conversions between the US customary units and the metric system. The speaker explains the need for 'crossover conversion factors' that link units from different systems, such as inches to centimeters. They demonstrate how to convert feet to centimeters using a two-step process involving feet to inches and then inches to centimeters. The paragraph also discusses the importance of treating conversion factors as exact, despite their actual values being approximations.
🏃♂️ Velocity Conversions: Kilometers per Hour to Meters per Second
The speaker tackles the conversion of velocity from kilometers per hour to meters per second. They emphasize that this involves multiple metric conversions, first converting kilometers to meters and then hours to seconds. The paragraph demonstrates the process of dimensional analysis in a multi-step conversion, highlighting the need for consistency in using conversion factors. The example provided calculates the velocity as 15 meters per second, ensuring the result has the correct number of significant figures.
📦 Density Calculations and Mass Determination
The final paragraph introduces the concept of density and its role in determining the mass of objects. The speaker explains the formula for density (mass divided by volume) and uses it to calculate the density of a cube with 2.00 cm edges. They then apply this density to determine the mass of a larger cube with 5.00 cm edges, emphasizing that density is an intensive property that remains constant regardless of the size of the sample. The paragraph concludes with the correct calculation of mass, ensuring the result has the appropriate number of significant figures.
Mindmap
Keywords
💡Metric System
💡Dimensional Analysis
💡Significant Figures
💡Scientific Notation
💡SI Units
💡Conversion Factors
💡Prefixes
💡Density
💡Volume
💡Temperature Conversion
Highlights
The final lesson in the measurement chapter focuses on units and conversions, including metric conversions and dimensional analysis.
The metric system is based on powers of ten, making calculations easier compared to other systems like the British Imperial system.
The United States and the UK are not fully adopting the metric system, which can cause issues in calculations and measurements.
The SI unit for length is the meter, for mass is the kilogram, and for temperature is Kelvin.
Conversion between Celsius and Kelvin is straightforward, involving adding or subtracting 273.15.
The metric system uses prefixes like kilo, centi, and milli to denote different magnitudes, which is crucial for conversions.
Dimensional analysis is a method used for converting units by ensuring the units cancel out appropriately.
When converting units, it's important to consider significant figures and express the answer with the correct number of significant figures.
The SI unit for volume is the cubic meter, but liters are more commonly used in everyday measurements.
Energy work is measured in joules, and pressure in pascals, though other units like atmospheres are more commonly used in chemistry.
Conversions within the metric system require memorizing prefixes and their corresponding powers of ten.
Area and volume conversions require squaring or cubing the conversion factors, respectively, to ensure units cancel out correctly.
Cross-system conversions, such as from US units to metric, require knowing specific conversion factors like 2.54 centimeters in an inch.
Multiple conversions in a single problem require careful attention to ensure each step is correctly converted.
Density is defined as mass per unit volume and can be calculated using the formula density = mass/volume.
The density of a substance is an intensive property, meaning it remains constant regardless of the size of the sample.
Calculating the mass of a larger cube of the same substance involves using the previously calculated density and the new volume.
Transcripts
Browse More Related Video

Learn Metric Units & Unit Conversions (Meters, Liters, Grams, & more) - [5-8-1]
Introduction to Pharmacy Calculations
How to Convert Units in Chemistry

02 - Learn Unit Conversions, Metric System & Scientific Notation in Chemistry & Physics

College Physics 1: Lecture 4 - Units and Unit Conversions
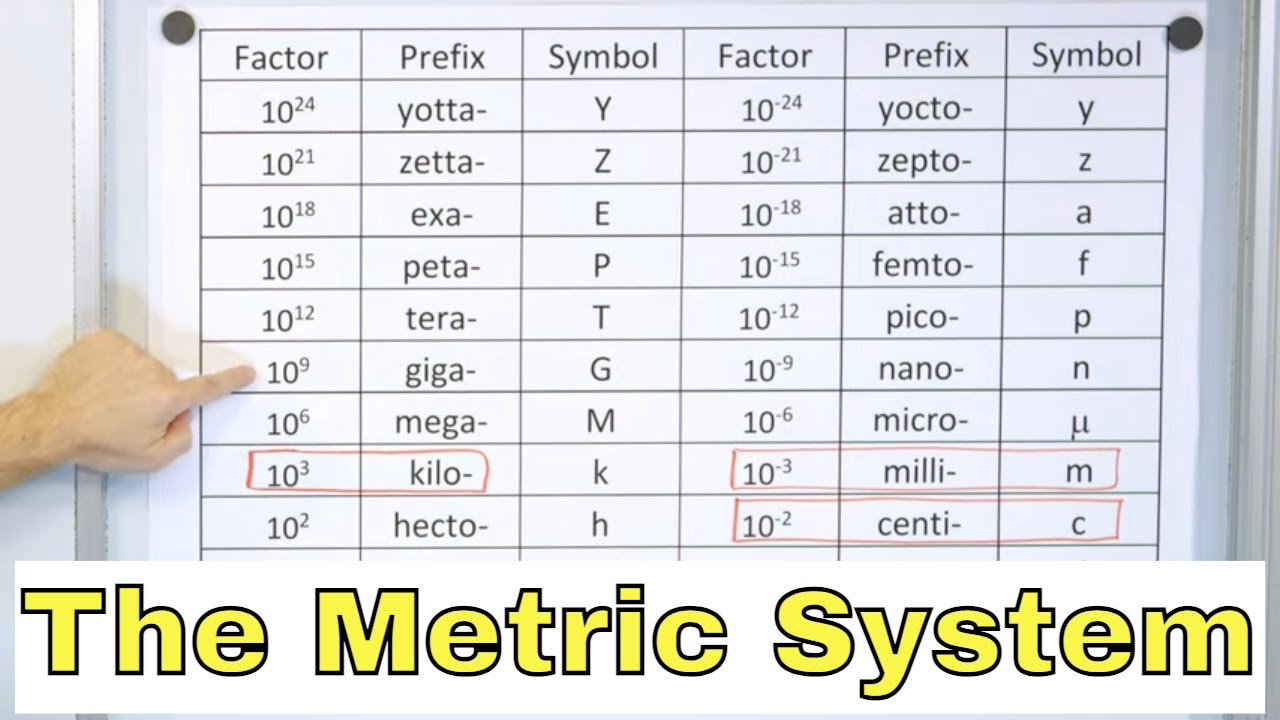
The Metric System and SI Units in Physics - [1-1-3]
5.0 / 5 (0 votes)
Thanks for rating: