What is Descriptive Statistics ... [Examples and Concept - Mean Median Mode]
TLDRThis video script from 'Statistics for Beginners' by Learning Fury introduces practical applications of statistical measures like range, mean, median, and mode. It uses real-life examples to explain the importance of choosing the right measure for data analysis. The script covers how mean reflects the average response, median provides a more accurate middle value in skewed data, and mode identifies the most frequent occurrence. It emphasizes the role of the statistician in selecting the appropriate measure for better decision-making.
Takeaways
- 📊 The video discusses practical applications of statistical concepts like range, mean, median, and mode.
- 📚 Statistics is categorized into descriptive and inferential statistics, with the video focusing on descriptive statistics.
- 🔍 Descriptive statistics help gauge the central tendency of data, such as average or middle responses.
- 🕒 The example given involves measuring feelings on a scale of 1 to 5 throughout a day, with data collected every 1-2 hours.
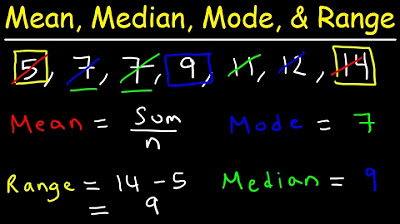
- 📈 The range of data is the difference between the maximum and minimum values, which in this case is 5 - 1.
- 🧮 The arithmetic mean (or simply mean) is calculated by averaging the responses, which in the example yields a neutral day.
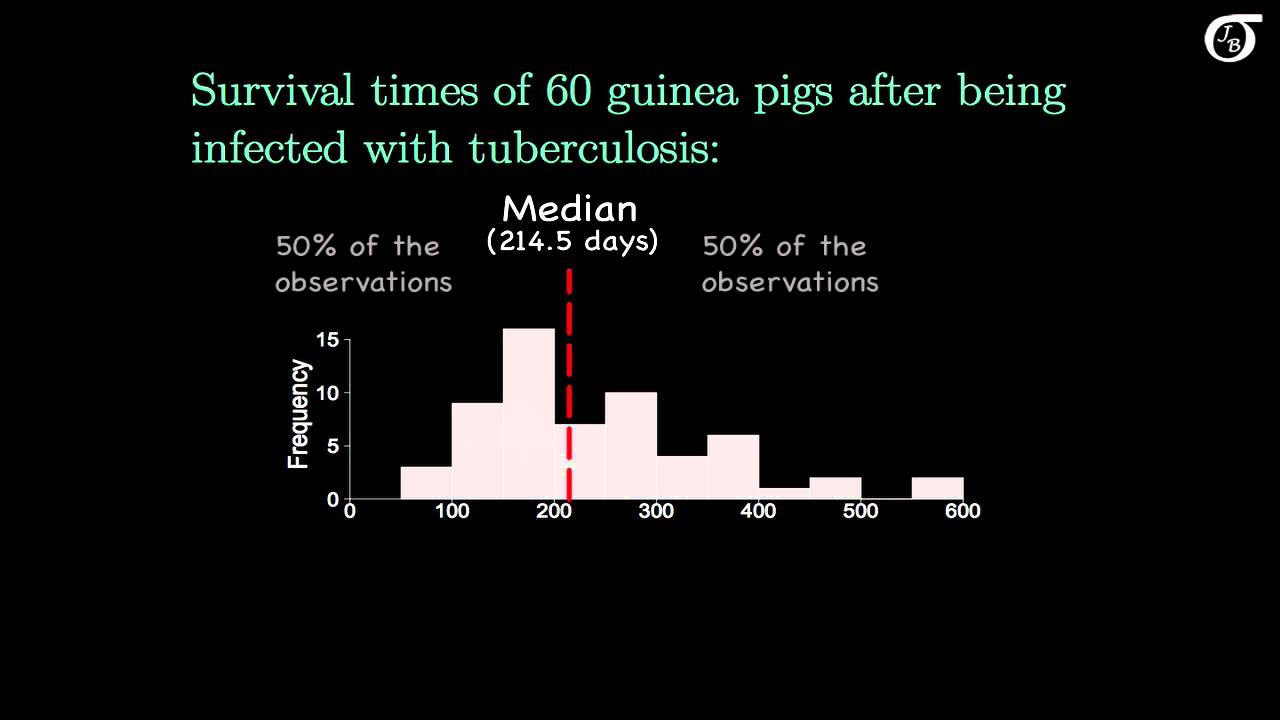
- 💼 The median is used when responses vary widely, as it provides a better representation of the middle value by finding the middle response in an ordered list.
- 👟 The mode is useful for identifying the most frequently occurring value, as seen in a retailer's decision-making about stocking shoe sizes.
- 🔑 Multiple modes can exist, indicating more than one value that occurs most frequently, which can be crucial for business decisions.
- 📉 Extreme values can skew the mean, making the median a more reliable measure of central tendency in certain scenarios.
- 📚 The choice of statistical measure (mean, median, or mode) depends on the data and the specific context, emphasizing the importance of careful data observation.
Q & A
What are the two main types of statistical analysis mentioned in the video?
-The two main types of statistical analysis mentioned are descriptive statistics and inferential statistics.
What is the purpose of descriptive statistics?
-Descriptive statistics are used to summarize and organize the characteristics of a set of data, providing measures of central tendency like mean, median, and mode.
What is the range in the context of the video's example about feeling during the day?
-The range in this context is the difference between the maximum value (5, very lively) and the minimum value (1, very tired) of the predefined scale used to measure feelings throughout the day.
How is the arithmetic mean calculated in the video's example?
-The arithmetic mean is calculated by summing all the responses and then dividing by the number of responses, which in the video's example results in a mean of 3, indicating a neutral day.
Why might the arithmetic mean not be the best measure of central tendency in the case of income during a population census?
-The arithmetic mean might not be the best measure because extreme values, such as very high incomes, can skew the average, making it less representative of the majority of the population.
What is the median and how is it calculated?
-The median is the middle value of a data set when it is ordered from least to greatest. If the number of observations is odd, the median is the middle number; if even, it is the average of the two middle numbers.
Why is the median a better measure of central tendency for the income data in the video's example?
-The median is a better measure for the income data because it is less affected by extreme values and provides a more accurate representation of the middle income level.
What is the mode and how is it used in the video's example of a shoe retailer?
-The mode is the value that appears most frequently in a data set. In the shoe retailer example, the mode helps identify the most frequently sold shoe sizes, which can inform stocking decisions.
What is the scenario where multiple modes might be useful for a retailer?
-Multiple modes are useful when a retailer wants to identify all the most popular items or sizes, not just one, to make informed decisions about inventory and sales.
Why might a retailer choose to stock more of sizes 7, 8, and 9 based on the mode?
-A retailer might choose to stock more of sizes 7, 8, and 9 because these sizes have the highest sales, indicating they are the most popular and in demand.
What is the importance of choosing the right measure for statistical analysis?
-Choosing the right measure is crucial for accurate decision-making. It ensures that the analysis reflects the true characteristics of the data set and provides meaningful insights.
Outlines
📊 Introduction to Statistical Measures
The script introduces the application of statistical concepts such as range, mean, median, and mode in real-life scenarios. It sets the stage for a discussion on how these measures can be utilized to understand and interpret numerical data. The video is aimed at beginners in statistics, providing an overview of descriptive statistics and the importance of central tendency in data analysis. An example is given where a person's mood throughout the day is measured on a scale, and the mean is used to determine the overall mood for the day. The script also touches on the limitations of the mean in scenarios with a wide range of values, such as income during a population census.
📈 Exploring Median and Mode in Statistical Analysis
This paragraph delves deeper into the use of median and mode as statistical measures, especially in cases where the mean might not accurately represent the data. The median is introduced as a measure that can provide a better representation of the middle value when there is a wide range of responses, as seen in population income data. The mode is presented as a useful measure for identifying the most frequently occurring values, such as the most popular shoe sizes in a retailer's inventory. The script also discusses the potential for multiple modes and how they can inform business decisions, such as stock management. The importance of choosing the right statistical measure based on the data at hand is emphasized, and the video promises a follow-up to demonstrate the calculation of these measures.
Mindmap
Keywords
💡Descriptive Statistics
💡Inferential Statistics
💡Central Tendency
💡Range
💡Arithmetic Mean
💡Median
💡Mode
💡Multiple Modes
💡Summary Statistics
💡Statistical Measures
💡Data Analysis
Highlights
Introduction to practical applications of statistical concepts like range, mean, median, and mode.
Descriptive statistics and inferential statistics are two types of statistical analysis.
Descriptive statistics focuses on the central tendency of data.
Example of measuring feelings on a scale from 1 to 5 throughout the day.
Range is the difference between the maximum and minimum values in the data.
Arithmetic mean is calculated to provide an average response.
Mean is useful in describing a batsman's strike rate in cricket.
Median is used when there is a wide spectrum of responses, like in a population census.
Median provides a better representation of the mid-value in data sets with extreme values.
Mode is useful in identifying the most frequently occurring value in a data set.
Mode can be used in business scenarios like determining which shoe sizes to stock.
Multiple modes can occur, indicating more than one frequently sold item.
Mean, median, and mode are summary statistics that provide a summary of a data set.
The choice of statistical measure depends on the data and the decision-making context.
Statistician or analyst must choose the right measure for better decision-making.
Upcoming video will demonstrate how to calculate mean, median, and mode.
Encouragement to like, subscribe, and enable notifications for more videos.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: