Calculus Chapter 5 Lecture 52 Convergence Tests 2
TLDRIn this calculus lecture, Professor Gist introduces two convergence tests for infinite series: the Root Test and the Ratio Test. The Root Test involves calculating the limit of the nth root of the sequence's nth term, determining convergence if the limit is less than one. The Ratio Test compares the ratio of consecutive terms, also converging if the limit is less than one. These tests are applied to various series, including geometric and p-series, demonstrating their utility and limitations. The lecture emphasizes the importance of choosing the right test for different series to determine convergence or divergence effectively.
Takeaways
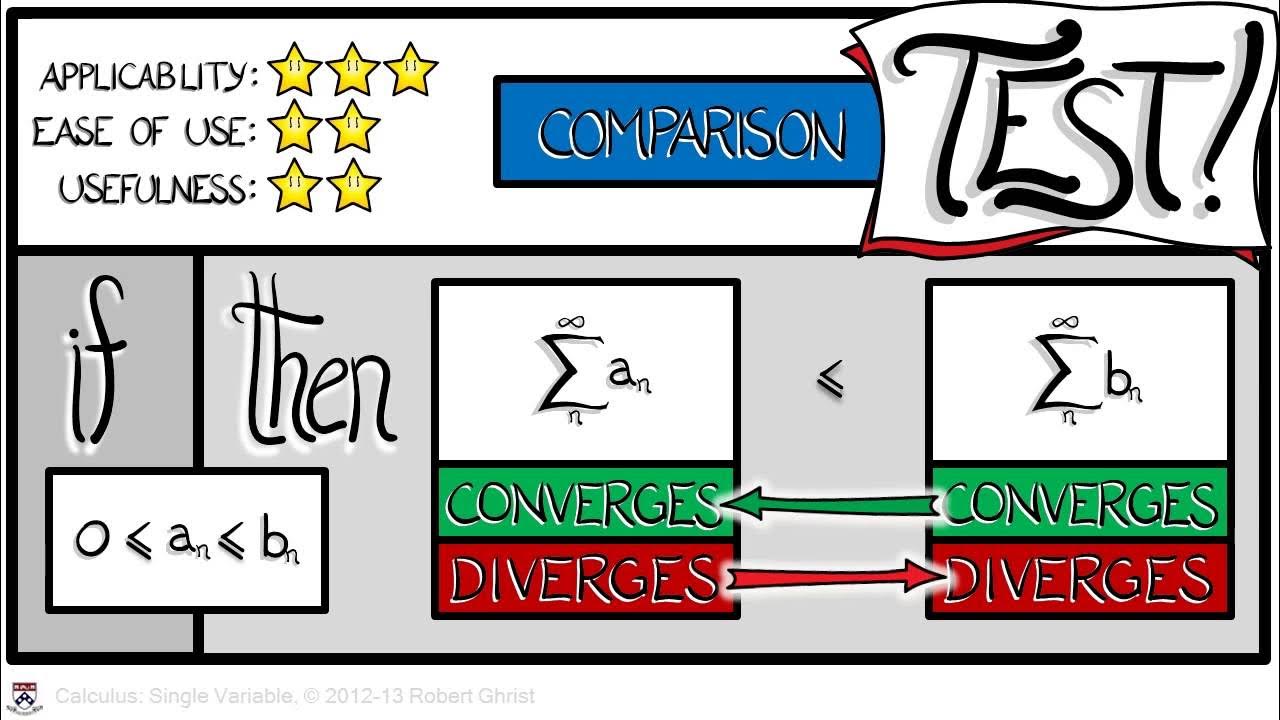
- 📚 The lecture introduces two additional tests for series convergence, the root test and the ratio test, building on the understanding of the geometric series.
- 🔍 The root test involves taking the limit of the nth root of the term 'a_subn' as n approaches infinity. If the limit is less than 1, the series converges; if greater than 1, it diverges; if equal to 1, the test is inconclusive.
- 📉 The ratio test assesses the limit of the ratio of consecutive terms 'a_subn+1/a_subn' as n approaches infinity. Similar to the root test, if the limit is less than 1, the series converges; if greater than 1, it diverges.
- 🤔 The root test can be difficult to apply due to the complexity of computing the nth root and the limit, which may limit its usefulness.
- 🔄 The ratio test is highlighted as a very general and easy-to-use test, often being the first choice for most series convergence checks.
- 🌰 An example is given where the root test is applied to a series involving logarithmic and trigonometric functions, demonstrating its effectiveness in cases where other tests might be cumbersome.
- 📉 The limitations of the root test are shown with the p-series, where the test fails to provide a conclusion, as the limit equals 1 regardless of the value of 'p'.
- 📈 The ratio test is illustrated with examples of factorial series, showing how it can easily determine convergence or divergence through simplification and cancellation of terms.
- 🤷♂️ The script emphasizes the importance of choosing the right test for a given series, as some tests may be more suitable depending on the series' characteristics.
- 🧠 The lecture encourages students to practice with various convergence tests to develop an intuition for which test is best suited for different types of series.
- 🔑 The conclusion reminds students that there are multiple tests available for determining the convergence or divergence of infinite series, and they should be used strategically.
Q & A
What are the two convergence tests introduced in the lecture?
-The two convergence tests introduced in the lecture are the Root Test and the Ratio Test.
How is the Root Test applied to determine the convergence of a series?
-In the Root Test, you compute the quantity \( \rho \) defined as the limit as \( n \) goes to infinity of the nth root of \( a_n \). The series converges if \( \rho \) is less than 1 and diverges if \( \rho \) is greater than 1. If \( \rho \) equals 1, the test is inconclusive.
Why might the Root Test be considered difficult to apply?
-The Root Test can be difficult to apply because computing the nth root and taking a limit as \( n \) goes to infinity can be challenging, which limits the usefulness of the test in some cases.
Can you provide an example where the Root Test is effective?
-An example where the Root Test is effective is the series \( \sum_{n=1}^{\infty} \left(\log(1 + \cos(\frac{1}{n}))\right)^{12n} \). The Root Test shows that this series converges since the computed \( \rho \) is the square root of the log of 2, which is less than 1.
What is a limitation of the Root Test as demonstrated by the P-series example?
-The Root Test fails for the P-series \( \sum_{n=1}^{\infty} \frac{1}{n^p} \) because the computed limit \( \rho \) equals 1, which makes the test inconclusive.
How is the Ratio Test defined?
-The Ratio Test involves computing \( \rho \) as the limit as \( n \) goes to infinity of the ratio \( \frac{a_{n+1}}{a_n} \). The series converges if \( \rho \) is less than 1 and diverges if \( \rho \) is greater than 1.
Why is the Ratio Test often preferred over the Root Test?
-The Ratio Test is often preferred because it is easier to use and tends to be more applicable in a wider variety of series, making it the first choice for many convergence problems.
Can you give an example where the Ratio Test is effectively used?
-An effective use of the Ratio Test is for the series \( \sum_{n=0}^{\infty} \frac{n!^3}{(3n)!} \). The test shows that the series converges with a limit of \( \frac{1}{27} \), which is less than 1.
What is an example of a series where the Ratio Test indicates divergence?
-An example is the series \( \sum_{n=0}^{\infty} \frac{n^n}{n!} \). The Ratio Test reveals that the series diverges because the limit \( \rho \) equals \( e \), which is greater than 1.
What advice is given regarding the use of convergence tests?
-The advice given is to understand and apply various convergence tests as a collection of tools, selecting the appropriate test based on the structure of the series. Practicing with different series will help in gaining intuition for which test is most suitable in a given situation.
Outlines
📘 Introduction to Convergence Tests
In this lecture, Professor Gist discusses the geometric series and introduces two additional tests for series convergence: the root test and the ratio test. The root test involves computing the limit of the nth root of a sequence and determining convergence based on whether the limit is less than one. The ratio test involves computing the limit of the ratio of subsequent terms in a sequence. Examples are provided to illustrate how these tests are applied.
🔍 Root Test Details and Example
The root test is explained in detail, highlighting its general applicability despite some computational challenges. An example is given where the root test is used to determine the convergence of a complex series involving logarithms and cosine functions. The test successfully shows that the series converges. Another example with the p-series demonstrates the limitations of the root test when the limit equals one.
🔗 Ratio Test Introduction and Example
The ratio test is introduced as an alternative to the root test, often easier to use and highly effective for most series. An example is provided with a series involving factorials, where the ratio test successfully demonstrates convergence. Another example with an exponential series shows how the ratio test can explicitly confirm divergence.
🔄 Further Applications and Comparisons
Additional examples are given to show the application of the ratio test, including a series with products of odd and even numbers and another with factorials and exponents. The ratio test effectively demonstrates convergence in these cases. The importance of selecting the appropriate test for different types of series is emphasized, along with a reminder to consider the form of the series before choosing a test.
📝 Conclusion and Further Learning
The lecture concludes with advice on mastering convergence tests through practice. The importance of understanding which test works best for different series is highlighted, likening it to using the right key for a lock. The next lesson will introduce a new class of infinite series and refine concepts of convergence.
Mindmap
Keywords
💡Convergence Tests
💡Geometric Series
💡Root Test
💡Ratio Test
💡nth Root
💡Limit
💡P-Series
💡Factorials
💡Divergence
💡Logarithm
💡Euler's Number (e)
Highlights
Introduction to Lecture 52 on convergence tests, leveraging the understanding of the geometric series.
Explanation of the root test for series convergence, including its conditions and limitations.
Root test applicability to general series and its ease of use, with potential difficulties in computing limits.
Example of applying the root test to a series involving logarithmic and trigonometric functions.
Demonstration of the root test's effectiveness in determining convergence when the limit is less than one.
Discussion on the root test's failure to provide information when the limit equals one.
Introduction of the ratio test as an alternative method for determining series convergence.
Description of the ratio test's ease of use and its applicability as a first choice for most series.
Example using the ratio test on a series with factorial terms and its simplification process.
Illustration of the ratio test's ability to quickly determine the convergence of a series with factorial growth.
Comparison between the root and ratio tests, emphasizing the latter's practicality and effectiveness.
Analysis of a series with odd and even numbers, using the ratio test to show its convergence.
Explanation of why the ratio test is preferred when dealing with series that have factorial terms.
Application of the ratio test to a series involving powers of pi and exponential functions, demonstrating its versatility.
Discussion on the importance of choosing the right test for different types of series based on their characteristics.
Emphasis on the need for practice in applying various convergence tests to gain intuition about their effectiveness.
Conclusion of the lecture with a reminder to explore different tests and their suitability for different series.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: