Tukey Kramer Multiple Comparison Procedure and ANOVA with Excel
TLDRThis educational video demonstrates the Tukey Kramer procedure for post-hoc analysis following a one-way ANOVA test. It explains the rejection of the null hypothesis, indicating at least one group mean differs from others. The script guides viewers through setting up a comparison table, calculating absolute differences, and using the studentized range (q) table to determine critical ranges. The procedure identifies which group means are significantly different, providing a deeper understanding of group variances beyond the initial ANOVA results.
Takeaways
- 🔍 The Tookie Kramer procedure is used for one-way ANOVA to make pairwise comparisons after rejecting the null hypothesis.
- 🧐 The null hypothesis in one-way ANOVA states that all group means are equal (μA = μB = μC = μD), while the alternative hypothesis suggests at least one mean is different.
- 📊 Rejecting the null hypothesis indicates that at least one group mean differs from the others, but it doesn't specify which ones.
- 🤔 After rejecting the null hypothesis, it's useful to identify which specific group means are different from each other through pairwise comparisons.
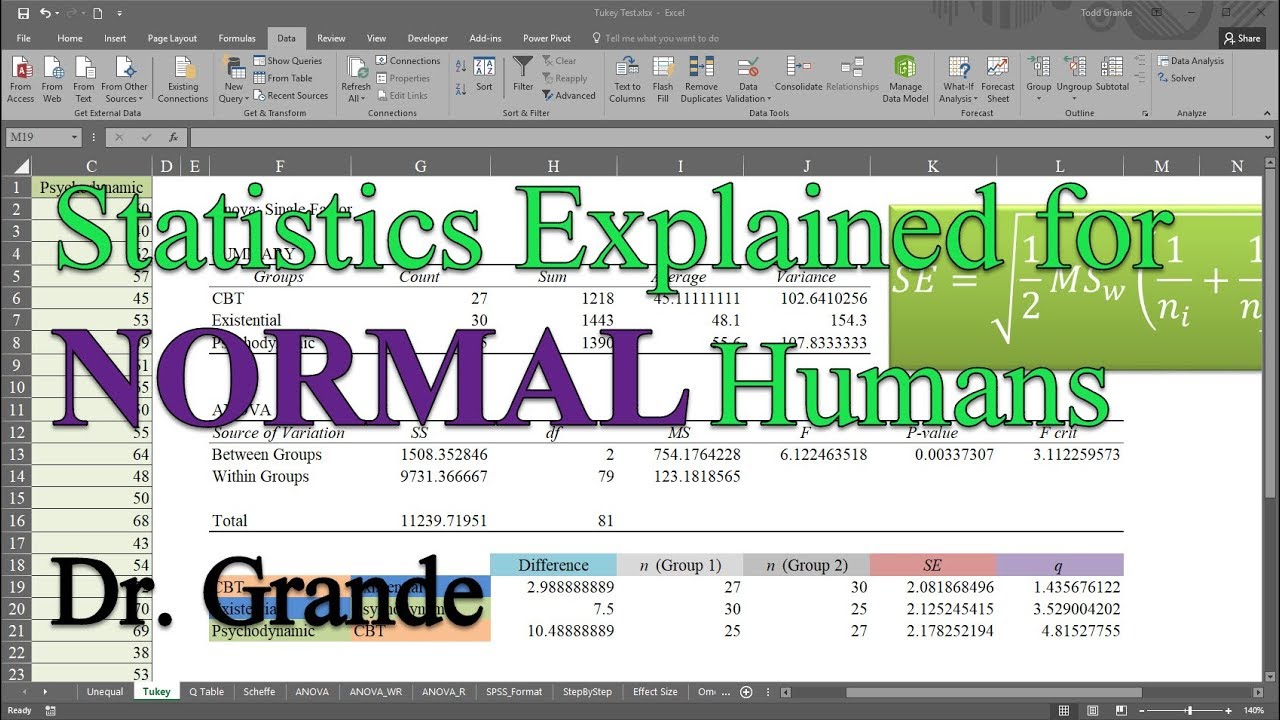
- 📝 The number of pairwise comparisons is calculated as C(C-1)/2, where C is the number of groups, resulting in 6 comparisons for 4 groups.
- 📈 The absolute difference between group means is calculated using the ABS function in Excel for each pairwise comparison.
- 📚 A Studentized Range (Q) table is necessary to determine the critical range values for the comparisons.
- 📋 The critical range formula is Q value * sqrt(Pooled Variance / number of observations in one group).
- 🔢 The degrees of freedom for the comparison are the number of groups for the numerator and total observations minus the number of groups for the denominator.
- ✅ If the absolute difference between two group means is greater than the critical range, the means are significantly different.
- 📉 Conversely, if the absolute difference is less than or equal to the critical range, the means are not significantly different.
Q & A
What is the Tookie Kramer procedure used for in the context of ANOVA?
-The Tookie Kramer procedure is used for post-hoc pairwise comparisons after rejecting the null hypothesis in a one-way ANOVA test, to determine which specific group means are significantly different from each other.
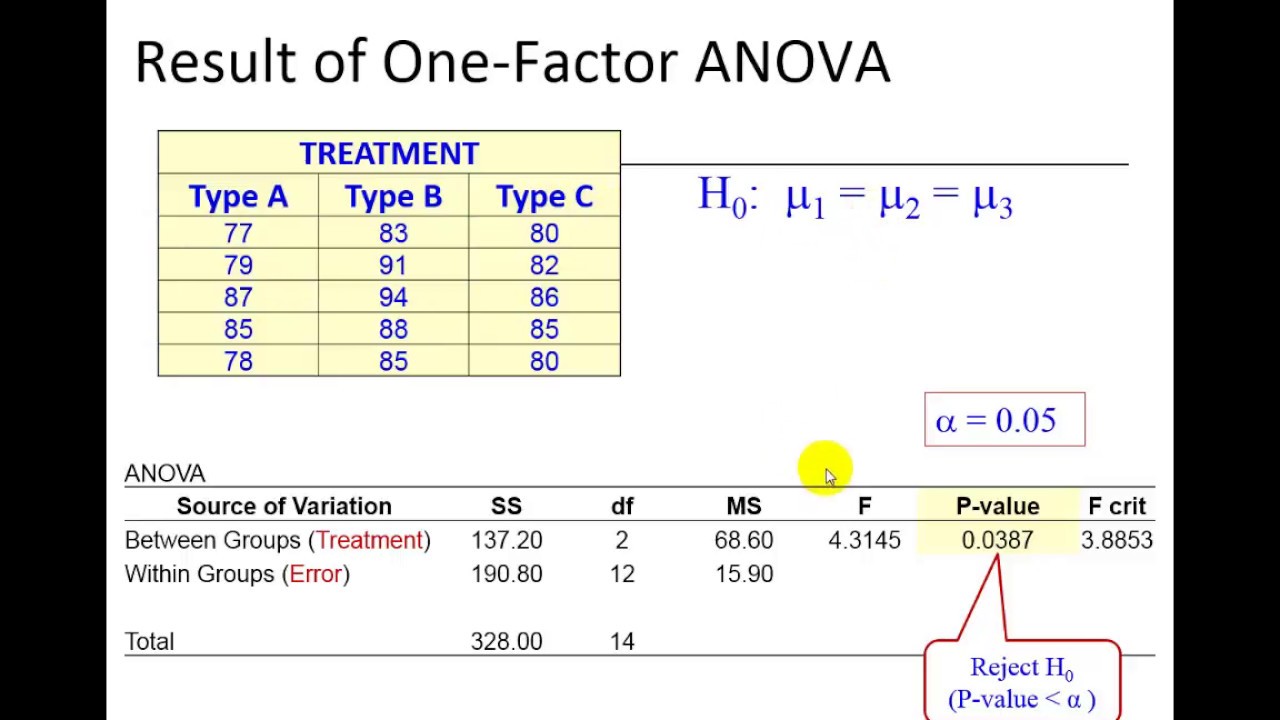
What is the null hypothesis in a one-way ANOVA test?
-The null hypothesis in a one-way ANOVA test is that all group means are equal, represented as μA = μB = μC = μD for four groups.
What is the alternative hypothesis if the null hypothesis is rejected in a one-way ANOVA test?
-The alternative hypothesis is that at least one group mean is different from the others, indicating some form of inequality among the group means.
How many pairwise comparisons are possible with four groups?
-With four groups, there are six possible pairwise comparisons: A vs. B, A vs. C, A vs. D, B vs. C, B vs. D, and C vs. D.
What is the formula for calculating the critical range in the Tookie Kramer procedure?
-The critical range is calculated using the formula Q * sqrt(S²_pooled / n_dot), where Q is the critical value from the studentized range q table, S²_pooled is the pooled variance, and n_dot is the number of observations in each group.
How do you determine the degrees of freedom for the numerator in the Tookie Kramer procedure?
-The numerator degrees of freedom is equal to the number of groups or levels in the ANOVA, which is C for C groups.
What is the formula for calculating the denominator degrees of freedom in the Tookie Kramer procedure?
-The denominator degrees of freedom is calculated as the total number of observations (n) across all groups minus the number of groups (C), which is n - C.
What is the purpose of the studentized range q table in the Tookie Kramer procedure?
-The studentized range q table provides critical values needed to determine the critical range for each pairwise comparison, which helps in identifying if the difference between group means is statistically significant.
How does the Tookie Kramer procedure help in interpreting the results of a one-way ANOVA test?
-The Tookie Kramer procedure helps in identifying which specific pairs of group means are significantly different after the overall ANOVA test has indicated that at least one group mean is different from the others.
What should you do if the absolute difference between two group means is greater than the critical range?
-If the absolute difference between two group means is greater than the critical range, it indicates that the two groups have significantly different means.
Outlines
📊 Introduction to Tookie Kramer Procedure for ANOVA
This paragraph introduces the Tookie Kramer procedure used for one-way or one-factor ANOVA, which is applied after rejecting the null hypothesis of equal means among groups. The null hypothesis (H0) states that all group means are equal (μA = μB = μC = μD), while the alternative hypothesis (H1) suggests at least one mean is different. The paragraph explains the implications of rejecting H0 and the necessity to identify which group means differ, setting the stage for multiple comparison tests.
🔍 Setting Up for Multiple Comparisons with Tookie Kramer
The speaker outlines the process of setting up for multiple comparisons using the Tookie Kramer procedure. It involves listing all possible pairwise comparisons among the groups, calculating the absolute differences between group means, and using the ABS function in Excel to ensure positive values. The paragraph also explains the need for a studentized range (q) table to determine critical values for comparisons, and how to calculate degrees of freedom for the numerator and denominator.
📐 Calculating the Critical Range for Comparisons
This section details the calculation of the critical range, which is essential for determining if the differences between group means are statistically significant. The critical range formula involves the q value from the studentized range table, the square root of the pooled variance, and the number of observations per group. The paragraph demonstrates how to perform these calculations in Excel and emphasizes the importance of using absolute references in the formula.
📉 Interpreting Results of Tookie Kramer Multiple Comparisons
The final paragraph discusses the interpretation of results from the Tookie Kramer procedure. It explains how to compare the absolute differences between group means with the critical range to determine if the means are significantly different. The paragraph concludes by summarizing the findings from the example, where not all pairwise comparisons showed significant differences, highlighting the importance of this procedure in identifying specific group differences after an initial rejection of the ANOVA null hypothesis.
Mindmap
Keywords
💡One-way ANOVA
💡Null Hypothesis
💡Alternative Hypothesis
💡Tukey's Honest Significant Difference (HSD)
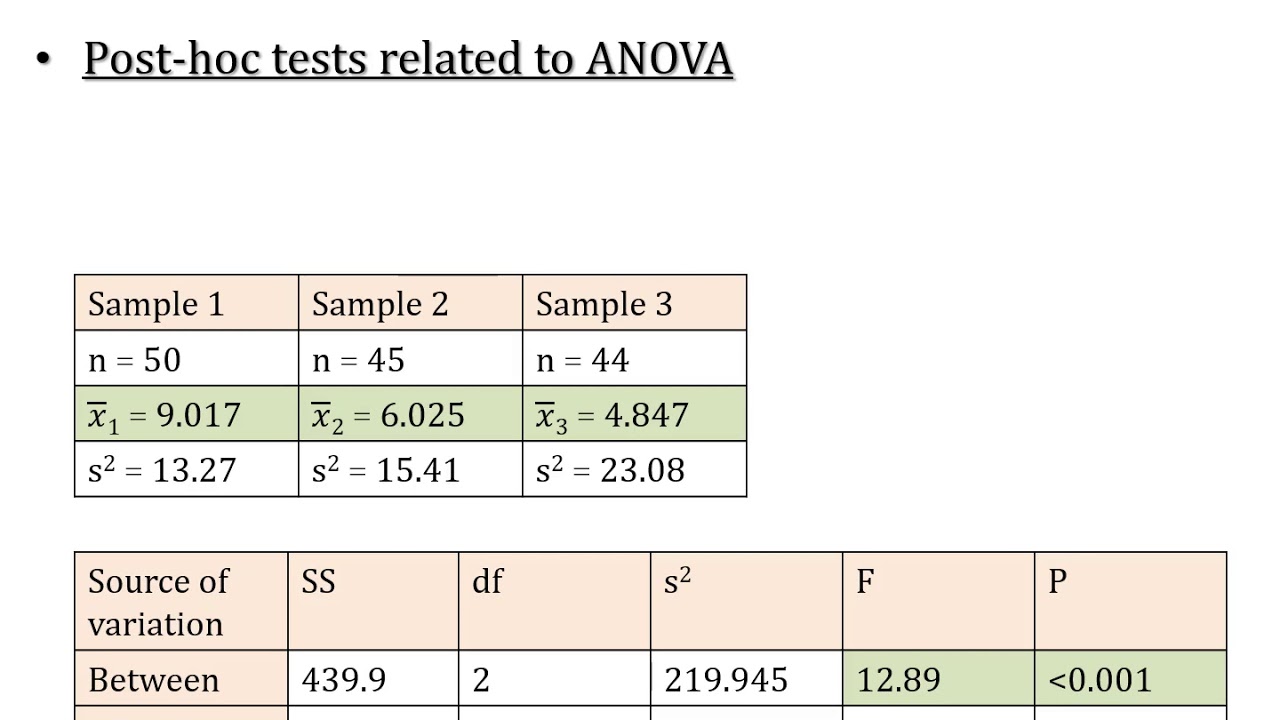
💡Post-hoc Test
💡Degrees of Freedom
💡Studentized Range (q) Table
💡Pooled Variance
💡Critical Range
💡Pairwise Comparison
💡Significant Difference
Highlights
Introduction to the Tookie Kramer procedure for one-way ANOVA.
Explanation of when to use the Tookie Kramer procedure: after rejecting the null hypothesis in one-way ANOVA.
Clarification of the one-way ANOVA null hypothesis: equal means across all groups.
Description of the alternative hypothesis: at least one group mean is different.
Importance of identifying which groups differ after rejecting the null hypothesis.
Demonstration of setting up a table for pairwise comparisons in the Tookie Kramer procedure.
Calculation of absolute differences between group means for comparisons.
Introduction to the studentized range (Q) table for critical value determination.
Method for determining degrees of freedom for the numerator and denominator.
Use of the Q value from the studentized range table in the critical range calculation.
Formula for calculating the critical range with equal group sizes.
Explanation of how to interpret results using the critical range and absolute differences.
Example of applying the Tookie Kramer procedure to determine significant differences between group means.
Discussion on the implications of the ANOVA results and the Tookie Kramer procedure.
Highlighting the practical use of the Tookie Kramer procedure for multiple comparisons.
Advice on using the studentized range Q table for accurate results.
Encouragement to subscribe for more statistical analysis tutorials.
Transcripts
Browse More Related Video
Tukey-Kramer Post Hoc Test after One-Way ANOVA in Excel
Tukey Method - One-Way ANOVA
LSD; Least Significant Difference; Post Hoc Test of ANOVA; Comparison of Means (Part A)
Fisher's LSD Explained

SPSS (9): Mean Comparison Tests | T-tests, ANOVA & Post-Hoc tests

One Way ANOVA (Analysis of Variance): Introduction | Statistics Tutorial #25 | MarinStatsLectures
5.0 / 5 (0 votes)
Thanks for rating: