Prove the equation has at least one real root (KristaKingMath)
TLDRThis video script discusses the application of the Intermediate Value Theorem to prove the existence of at least one real root for the function cos(x) - x^3. It guides viewers through analyzing the domain and range of each term, demonstrating that for values greater than one, the function is positive, and for values less than or equal to -1, it is negative. The script concludes by applying the theorem between -1 and 1, showing the function must cross the x-axis, thus confirming a real root exists. The explanation ensures the function's continuity, validating the theorem's application.
Takeaways
- 📚 The video discusses the application of the Intermediate Value Theorem to prove the existence of at least one real root for a given function.
- 🔍 The function in question is cos(x) - x^3, and the goal is to show that there is at least one point where the function crosses the x-axis (f(x) = 0).
- 📉 The video explains that normally, an interval is given to demonstrate the existence of a root, but in this case, an interval is not specified.
- 📌 The approach involves collecting all terms to one side of the equation to analyze the function's behavior over its domain.
- 📐 The domain of x^3 is all real numbers, with no restrictions on its range, which can vary from negative infinity to positive infinity.
- 🌐 The range of cos(x) is limited between -1 and 1, which fluctuates between these values.
- 🔑 The video suggests picking a value outside the range of cos(x), such as x = 2, to demonstrate that f(x) will be positive for any x > 1.
- 📈 For values of x less than -1, the function f(x) is shown to be negative, indicating that the function must cross the x-axis between -1 and 1.
- 📊 The Intermediate Value Theorem is applied to conclude that since f(-1) < 0 and f(1) > 0, there must be a root between -1 and 1.
- 🔍 The video emphasizes the importance of ensuring the function's continuity over the range where the root is sought.
- 📝 The function cos(x) - x^3 is proven to be continuous because both x^3 and cos(x) are continuous functions, satisfying the conditions of the theorem.
Q & A
What is the main topic of the video script?
-The main topic of the video script is the use of the Intermediate Value Theorem to prove that a function has at least one real root.
What function is the focus of the problem in the script?
-The function in focus is f(x) = x^3 - cos(x).
Why is the Intermediate Value Theorem applicable in this context?
-The Intermediate Value Theorem is applicable because it states that if a function is continuous on a closed interval and changes sign over that interval, then it has at least one root in that interval.
What does the script suggest as the first step to analyze the function f(x) = x^3 - cos(x)?
-The first step is to collect all terms onto one side to form an equation, which is done by subtracting cosine of x from both sides, resulting in f(x) = x^3 - cos(x).
What is the domain and range of x^3 in the script's context?
-The domain of x^3 is all real numbers, and its range is also all real numbers, from negative infinity to positive infinity.
What is the range of the cosine function as discussed in the script?
-The range of the cosine function is between -1 and 1, as it fluctuates between these two values.
How does the script suggest to find a point where f(x) is positive?
-The script suggests picking a value of x that is greater than 1, such as 2, and showing that f(x) will be positive for any value greater than 1.
What values of x are considered to show that f(x) is negative?
-The script considers values of x that are less than or equal to -1, such as -2, to show that f(x) will be negative for any value less than or equal to -1.
What conclusion does the script draw about the continuity of the function f(x) = x^3 - cos(x)?
-The script concludes that the function is continuous because both x^3 and cos(x) are continuous functions, and the composition of continuous functions is also continuous.
What does the script suggest as the final step to prove that the equation has at least one real root?
-The final step is to apply the Intermediate Value Theorem by showing that f(x) changes sign between -1 and 1, which implies that there is a root in that interval.
What is the script's stance on the necessity of continuity for applying the Intermediate Value Theorem?
-The script emphasizes that the necessity of continuity is crucial for applying the theorem, and it justifies this by stating that since all terms in the function are continuous, the function as a whole is continuous.
Outlines
📚 Applying the Intermediate Value Theorem to Prove Real Roots
This paragraph introduces the concept of using the intermediate value theorem to demonstrate the existence of at least one real root for a given function. The focus is on the function cos(x) - x^3, and the task is to show that there is at least one point where this function crosses the x-axis, indicating a real root. The speaker explains the process of moving all terms to one side to form a new function, F(x) = x^3 - cos(x), and discusses the domain and range of each term. It is highlighted that x^3 can take any real number value, while cos(x) is restricted between -1 and 1. The paragraph concludes by illustrating that for values of x greater than 1, F(x) will be positive, setting the stage for applying the intermediate value theorem.
📉 Analyzing the Function's Behavior at Extreme Values
In this paragraph, the speaker delves deeper into the behavior of the function F(x) = x^3 - cos(x) by examining its values at the extremes of the cosine function's range. By choosing x values such as -2 and 2, which are outside the range of cos(x), the speaker demonstrates that F(x) yields negative values, indicating that the function is below the x-axis. The analysis extends to values just outside the range of the cosine function, such as 1.1, reinforcing the conclusion that F(x) is negative for values near -1 and positive for values near 1. The intermediate value theorem is then invoked to assert that since F(x) is negative at -1 and positive at values greater than 1, there must be at least one point where the function crosses the x-axis between these points, confirming the existence of a real root. The speaker also assures the continuity of the function, which is a prerequisite for applying the intermediate value theorem, by confirming the continuity of both x^3 and cos(x). The paragraph ends with a summary of the proof and an invitation for viewers to engage with the content.
Mindmap
Keywords
💡Intermediate Value Theorem
💡Function
💡Real Root
💡Domain
💡Range
💡Graph
💡Cosine Function
💡X-Cubed
💡Continuous Function
💡Sign Change
💡Inequality
Highlights
Introduction to using the Intermediate Value Theorem to prove the existence of at least one real root for a function.
Explanation of the process to show that the function crosses the x-axis by examining the range of each term.
Task to prove the existence of a real root for the function cos(x) * x^3 without a specified interval.
Method to collect terms and form the equation F(x) = x^3 - cos(x).
Analysis of the domain and range of x^3, which is all real numbers.
Understanding the range of the cosine function, which fluctuates between -1 and 1.
Investigation of the function's behavior by choosing a value outside the range of the cosine function.
Demonstration that F(x) is positive for x values greater than 1.
Explanation of the function's value being positive for values just outside the cosine range.
Analysis of the function's behavior at the extreme values of the cosine function, showing it is negative.
Application of the Intermediate Value Theorem to prove the existence of a real root between -1 and 1.
Necessity to ensure the graph's continuity for the application of the theorem.
Proof of the function's continuity based on the continuity of its components.
Conclusion that the equation cos(x) * x^3 has at least one real root in its domain.
Encouragement for viewers to like and subscribe for future educational content.
Transcripts
Browse More Related Video
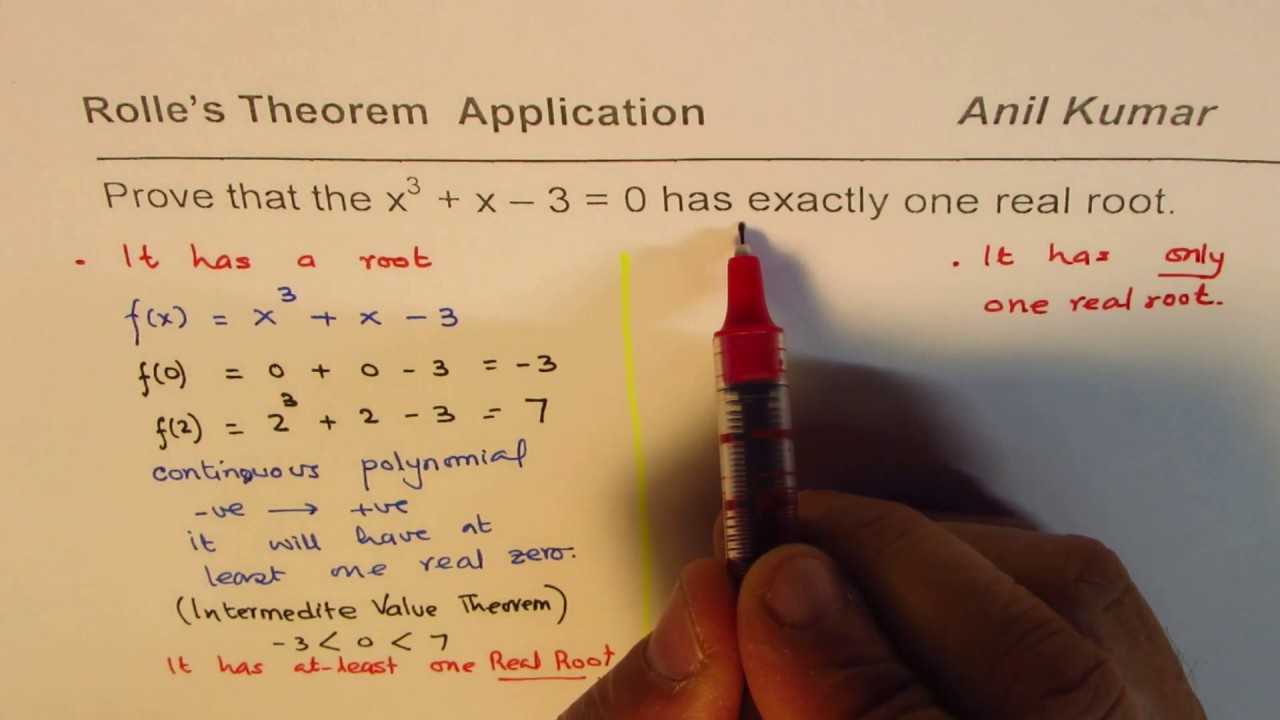
Rolle's Theorem to Prove Exactly one root for Cubic Function AP Calculus
Intermediate value theorem to prove a root in an interval (KristaKingMath)

Intermediate Value Theorem: show function has at least one solution -- CALCULUS
Intermediate Value Theorem, calculus 1 tutorial, showing a root of a function on an interval

Mean Value Theorem | MIT 18.01SC Single Variable Calculus, Fall 2010

❖ The Mean Value Theorem ❖
5.0 / 5 (0 votes)
Thanks for rating: