Kinetic Molecular Theory of Gases - Practice Problems
TLDRThis video script delves into the kinetic molecular theory of ideal gases, outlining five key assumptions that define their behavior, such as negligible particle volume and constant random motion. It contrasts ideal gas behavior with real gases, which deviate due to factors like particle size and intermolecular forces. The script explores gas laws, including Boyle's, Charles's, Avogadro's, and Gay-Lussac's, explaining how temperature, pressure, and volume interplay. It also discusses factors affecting gas pressure and velocity, using examples and diagrams for clarity, and provides practice problems to reinforce understanding.
Takeaways
- 🌌 The kinetic molecular theory of gases is a model that describes the behavior of an ideal gas, which assumes negligible volume of gas particles, constant random motion, elastic collisions, no intermolecular forces, and direct proportionality between average kinetic energy and Kelvin temperature.
- 🔍 Real gases may deviate from the ideal gas behavior due to factors such as significant particle volume, intermolecular forces, and non-elastic collisions.
- 📉 According to Boyle's Law, for an ideal gas, doubling the volume results in halving the pressure, but real gases may deviate from this due to their particle volume.
- 🏃 Gas particles are in perpetual random motion due to collisions with other particles and the container walls, which appears random due to the high number of particles.
- 🔁 Elastic collisions in gases mean kinetic energy is conserved, with no loss of energy during collisions.
- 🧲 Intermolecular forces in real gases, such as dipole interactions and hydrogen bonds, cause deviations from ideal gas behavior, especially in polar gases.
- ⚖️ Non-polar gases, which only have weak London dispersion forces, tend to behave more ideally than polar gases.
- ↔️ The average kinetic energy of a gas is directly proportional to its Kelvin temperature, indicating that gases at higher temperatures have more kinetic energy.
- 🏋️ The average velocity of gas molecules is determined by the root mean square velocity formula, which depends on temperature and molar mass, with lighter gases moving faster.
- 📉 Under high temperature and low pressure conditions, real gases behave more like ideal gases due to reduced intermolecular forces and increased particle motion.
- 📊 The behavior of gases can be understood through various laws, including Boyle's Law (pressure-volume relationship), Charles's Law (volume-temperature relationship), and Avogadro's Law (volume-moles relationship), each explaining different aspects of gas behavior.
Q & A
What is the kinetic molecular theory of gases?
-The kinetic molecular theory of gases is a model that attempts to describe the behavior of an ideal gas, based on certain assumptions such as negligible volume of gas particles, constant random motion of particles, elastic collisions, no intermolecular forces, and direct proportionality between average kinetic energy and temperature.
How do real gases deviate from the behavior of an ideal gas?
-Real gases deviate from ideal gas behavior due to factors such as significant volume of gas particles, non-negligible intermolecular forces, and non-elastic collisions, which are not accounted for in the ideal gas model.
Why does the pressure of a real gas not decrease exactly by a factor of two when its volume is doubled, according to Boyle's Law?
-The pressure of a real gas may not decrease exactly by a factor of two when its volume is doubled because real gas particles take up space and can exert intermolecular forces, causing deviations from the ideal gas behavior.
What is the significance of gas particles being in constant random motion?
-The constant random motion of gas particles is significant because it leads to frequent collisions with the container walls, which is the primary factor contributing to the pressure exerted by the gas.
Why are collisions between gas particles considered elastic in the kinetic molecular theory?
-Collisions between gas particles are considered elastic because kinetic energy is conserved during these collisions, meaning there is no loss of energy in the system.
How do polar gases differ from non-polar gases in terms of their behavior as an ideal gas?
-Polar gases tend to deviate more from ideal gas behavior due to the presence of intermolecular forces such as dipole interactions and hydrogen bonds, whereas non-polar gases only exhibit weak London dispersion forces and thus behave more ideally.
What is the relationship between the average kinetic energy of a gas and its temperature?
-The average kinetic energy of a gas is directly proportional to its Kelvin temperature, meaning that as the temperature increases, so does the average kinetic energy of the gas.
Why does the average velocity of gas molecules increase with temperature?
-The average velocity of gas molecules increases with temperature because the kinetic energy, which is a function of the temperature, is proportional to the square of the velocity, leading to faster-moving particles at higher temperatures.
Under what conditions will a real gas behave more like an ideal gas?
-A real gas will behave more like an ideal gas under conditions of low pressure and high temperature, where the effects of intermolecular forces and particle volume are minimized.
Which gas would best approach the behavior of an ideal gas among methane, argon, and helium?
-Helium would best approach the behavior of an ideal gas due to its non-polar nature and lowest molar mass, resulting in the least intermolecular forces and smallest particle volume.
How does the pressure exerted by a gas change with its molar mass?
-The pressure exerted by a gas is independent of its molar mass. While heavier gas molecules may exert more force due to greater mass, they also move slower, balancing out the effect so that the average pressure is the same regardless of molar mass.
What is the explanation behind Boyle's Law stating that decreasing the volume of a container increases the pressure?
-According to Boyle's Law, when the volume of a container is decreased, the gas particles collide more frequently with the container walls due to less space, leading to an increase in pressure.
How does Avogadro's Law relate to the increase in volume when the number of moles of gas is increased?
-Avogadro's Law states that when the number of moles of gas in a container is increased, the volume of the container increases to accommodate the additional gas particles, assuming the temperature and pressure are constant.
What is the effect of temperature on the pressure of a gas according to Gay-Lussac's Law?
-According to Gay-Lussac's Law, when the temperature of a gas in a fixed volume container is increased, the pressure also increases due to the increased average speed and collision frequency of the gas particles with the container walls.
How does Charles's Law explain the relationship between temperature and volume of a gas?
-Charles's Law states that when the temperature of a gas in a flexible container increases, the volume also increases. This is because the increased temperature leads to higher pressure, which in turn causes the flexible container to expand, accommodating the increased volume.
Outlines
🌌 Ideal Gas Behavior and Deviations
This paragraph introduces the kinetic molecular theory of gases, which is a model describing the behavior of an ideal gas. It explains that real gases may deviate from the ideal behavior due to factors such as particle volume, which is considered negligible in an ideal gas. The paragraph also discusses Boyle's law in the context of ideal versus real gases, highlighting how real gases can exhibit different pressure changes with volume adjustments compared to the predictable behavior of ideal gases.
🔁 Constant Random Motion of Gas Particles
The paragraph delves into the second assumption of the kinetic molecular theory, which is that gas particles are in perpetual random motion due to thermal energy, even at absolute zero. It uses the analogy of a crowded pool table to illustrate how gas particles collide with each other and the container walls in a seemingly random fashion, emphasizing the constant and straight-line nature of their movement before collisions occur.
🚫 No Intermolecular Forces in Ideal Gases
This section addresses the fourth assumption of the kinetic molecular theory, stating that particles in an ideal gas do not attract or repel each other, in contrast to real gases which can exhibit intermolecular forces. It provides the example of polar gases like ammonia, which have partial charges leading to attractive and repulsive forces between molecules, causing deviations from ideal gas behavior. The paragraph also explains that non-polar gases, lacking such forces, tend to behave more ideally.
⚖️ Average Kinetic Energy and Temperature
The paragraph discusses the relationship between the average kinetic energy of a gas and its temperature, as described by the kinetic molecular theory. It states that the average kinetic energy is directly proportional to the Kelvin temperature, meaning that gases at higher temperatures have greater average kinetic energy. The summary also touches on the concept of average kinetic energy in the context of a collection of gas particles, rather than individual particles.
❓ Kinetic Molecular Theory Practice Problems
This segment presents multiple-choice practice problems related to the kinetic molecular theory of gases. It covers questions about which gas would have the highest average kinetic energy at a given temperature and pressure, the effect of molar mass on the average velocity of gases, and which gases would behave most ideally. The paragraph uses the root mean square velocity formula to explain the relationship between molar mass, temperature, and velocity.
📉 Conditions for Real Gases to Behave Ideally
The paragraph explores the conditions under which real gases can approximate the behavior of ideal gases. It explains that real gases are more likely to behave ideally at low pressures and high temperatures, using a phase diagram to illustrate the relationship between temperature, pressure, and the state of matter. It also contrasts the behavior of polar and non-polar gases, noting that non-polar gases with lower molar mass and fewer intermolecular forces will behave more ideally.
📊 Boyle's Law and Pressure-Volume Relationship
This section focuses on Boyle's law, which describes the inverse relationship between the pressure and volume of a gas when temperature and the amount of gas are held constant. It discusses the implications of Boyle's law, including how an increase in volume leads to a decrease in pressure, and vice versa. The paragraph also differentiates between the graphical representations of Boyle's law and other gas laws, such as Charles's and Gay-Lussac's laws.
🌡️ Temperature Effects on Gas Density and Velocity
The paragraph examines how temperature affects gas density and the average velocity of gas molecules. It explains that gas density is inversely proportional to temperature, as an increase in temperature leads to an increase in volume and a decrease in density. Conversely, the average velocity of gas molecules increases with temperature. The summary also addresses the misconception that heavy gas molecules exert more pressure on container walls than lighter ones, clarifying that pressure is independent of molar mass due to the balancing effects of mass and velocity.
🏺 Expanding on Gas Laws with Avogadro's and Charles's Law
This final paragraph synthesizes the concepts of Avogadro's and Charles's laws, explaining how an increase in temperature leads to an increase in volume for a gas in a flexible container. It uses the example of a balloon to illustrate how an initial increase in pressure due to temperature rise results in the expansion of the balloon, followed by a decrease in pressure as the volume increases, ultimately returning to equilibrium. The paragraph emphasizes the net effect of these changes, which is an increase in volume without a change in pressure.
Mindmap
Keywords
💡Kinetic Molecular Theory
💡Ideal Gas
💡Boyle's Law
💡Elastic Collision
💡Intermolecular Forces
💡Polar and Non-Polar Gases
💡Average Kinetic Energy
💡Root Mean Square Velocity
💡Phase Diagram
💡Charles's Law
💡Gay-Lussac's Law
Highlights
The kinetic molecular theory of gases is a model that describes the behavior of an ideal gas, with deviations possible in real gases.
The first assumption of the kinetic molecular theory is that the volume of individual gas particles is negligible compared to the distance between them.
Real gases may deviate from ideal gas behavior due to the significant volume of their particles.
Boyle's law states that for an ideal gas, doubling the volume results in half the pressure, but real gases may deviate from this relationship.
Gas particles are in constant random motion due to temperature being above absolute zero.
The motion of gas particles appears random due to frequent collisions with other particles and the container walls.
Elastic collisions in gases mean kinetic energy is conserved, unlike inelastic collisions where energy is lost.
The fourth assumption is that gas particles do not attract or repel each other, exerting no forces, which differs in real gases.
Polar gases like ammonia exhibit deviations from ideal gas behavior due to intermolecular forces like dipole interactions.
Non-polar gases behave more ideally than polar gases due to weaker London dispersion forces compared to dipole interactions or hydrogen bonds.
The average kinetic energy of a gas is directly proportional to its Kelvin temperature, as per the kinetic molecular theory.
The average velocity of gas particles is determined by the root mean square velocity formula, which depends on temperature and molar mass.
Lighter gases have higher average velocities due to lower molar mass, despite having the same average kinetic energy as heavier gases.
The pressure of a gas is not dependent on its molar mass, contradicting a common misconception.
A real gas will behave more like an ideal gas under conditions of low pressure and high temperature, aligning with the kinetic molecular theory.
Helium, with the lowest molar mass, exhibits behavior closest to an ideal gas due to minimal intermolecular forces.
The relationship between pressure and volume for an ideal gas follows Boyle's law, depicted as an inverse relationship in a graph.
Gases with more moles in a container exert a greater pressure due to increased molecular collisions with the container walls.
Density of a gas is inversely proportional to the Kelvin temperature and directly related to pressure and molar mass.
The average velocity of gas molecules increases with temperature, as derived from the root mean square velocity formula.
The pressure of a gas is dependent on the collision frequency of gas particles with the container walls, contrary to a false statement.
Transcripts
Browse More Related Video

9.1 Pressure and Kinetic Molecular Theory of Gases | High School Chemistry
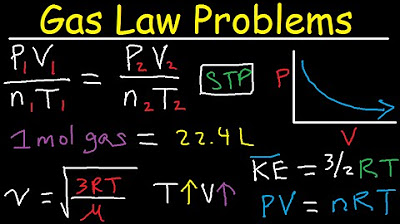
Gas Law Problems Combined & Ideal - Density, Molar Mass, Mole Fraction, Partial Pressure, Effusion
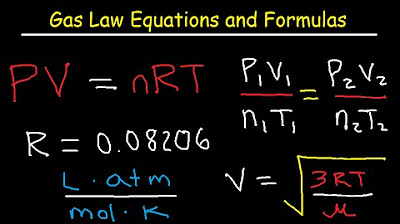
Gas Laws - Equations and Formulas
Ideal Gas Problems: Crash Course Chemistry #13
Ideal gas equation: PV = nRT | Chemistry | Khan Academy
Kinetic Theory of Gases - A-level Physics
5.0 / 5 (0 votes)
Thanks for rating: