Reaction Kinetics Concepts
TLDRThis video script offers an in-depth crash course on reaction kinetics, exploring the concept of reaction speed and how it's measured. It explains the definitions of instantaneous and initial rates, and contrasts them with average rates. The script delves into experimental methods for measuring reaction rates, the dependence of reaction rates on concentration, temperature, and catalysts, and introduces rate equations. It also covers different reaction orders, the significance of half-life in first-order reactions, and the transformation of second-order reactions into pseudo first-order reactions under certain conditions. The script concludes with the macroscopic basis of reaction kinetics, including the Arrhenius equation and the Maxwell-Boltzmann distribution, and touches on the relationship between reaction rate equations and mechanisms.
Takeaways
- 🔍 Reaction kinetics is the study of the speed at which a chemical reaction occurs.
- 📉 The rate of a reaction can be defined based on the change in concentration of reactants or products over time.
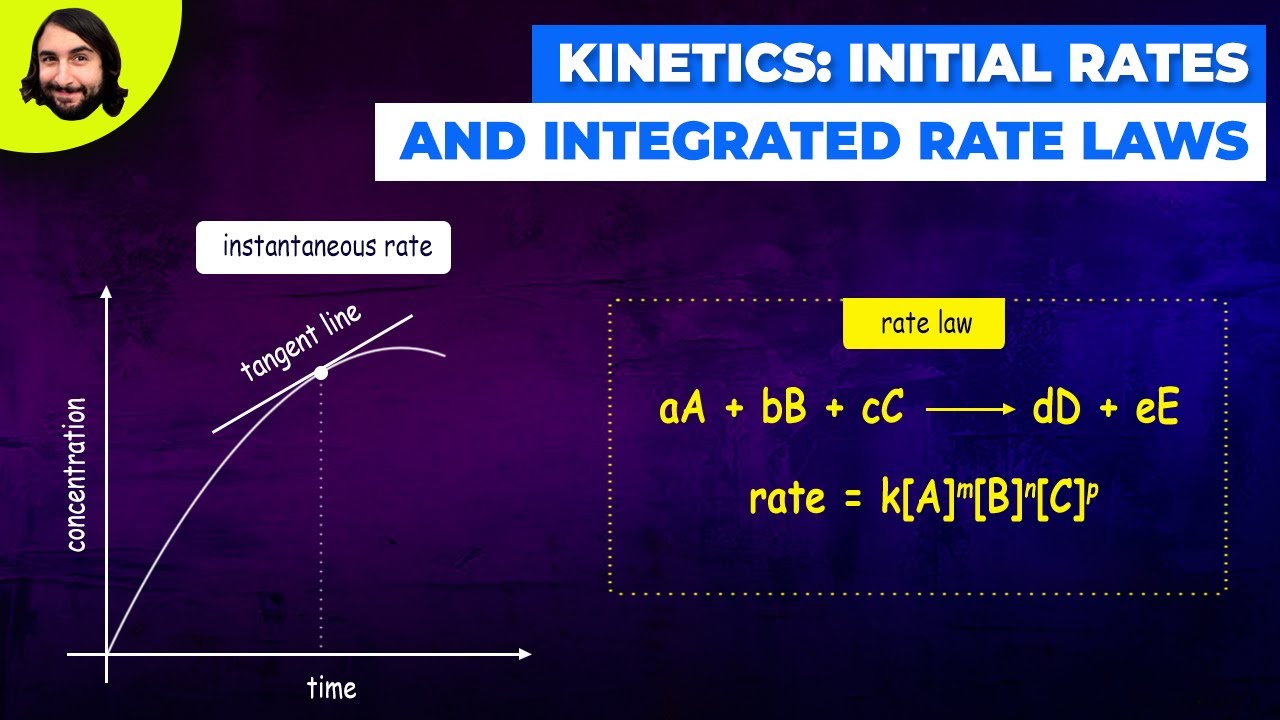
- 📈 Instantaneous rate is the derivative of concentration with respect to time, while initial rate is the instantaneous rate at time equals zero.
- 🔬 Average rate is an approximation of the instantaneous rate, calculated as the change in concentration over a time interval.
- 🧪 Experimental methods to measure reaction rates include continuous monitoring and clock-wise methods observing visual changes.
- 🌡 The reaction rate depends on factors such as temperature, presence of a catalyst, and the concentration of reactants.
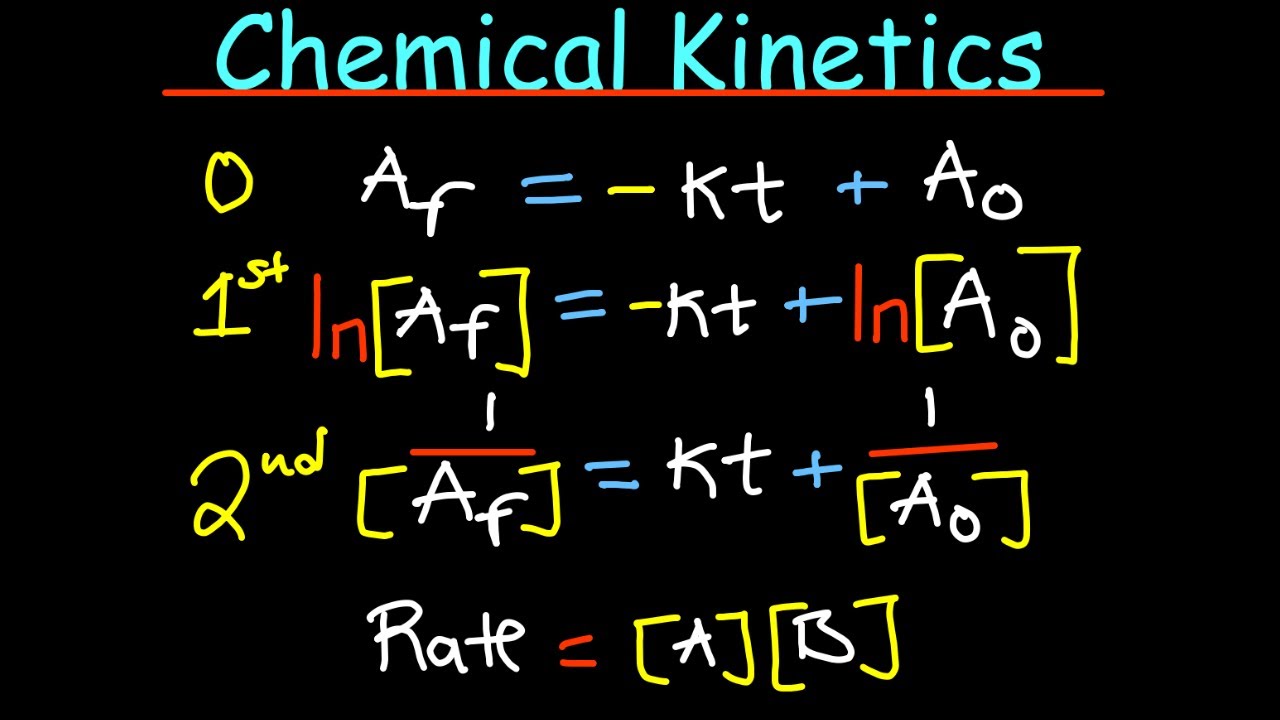
- ⚗ The rate equation expresses how the rate of a reaction depends on the concentration of reactants, with the rate constant K being temperature and activation energy dependent.
- 📊 First-order reactions have a constant half-life, which can be determined from the rate constant and is independent of the initial concentration.
- 🔄 Second-order reactions can be dependent on the concentration of one or two reactants, with different forms of rate equations.
- 🔄 Pseudo-first-order reactions occur when the concentration of one reactant is much larger than the other, simplifying the rate equation.
- 🌡 The Arrhenius equation relates the rate constant to temperature and activation energy, explaining how temperature affects reaction rates.
- 📚 The Maxwell-Boltzmann distribution illustrates the effect of temperature and catalysts on the number of particles with sufficient energy for a reaction.
Q & A
What is reaction kinetics?
-Reaction kinetics is the study of the speed or rate at which a chemical reaction occurs. It involves understanding the factors that influence how quickly reactants are converted into products.
How is the rate of a reaction defined in terms of concentration changes?
-The rate of a reaction can be defined based on the change in concentration of a reactant or product with respect to time. It is typically represented as the derivative of the concentration with respect to time, considering the stoichiometric coefficients.
What is the difference between instantaneous rate and initial rate?
-The instantaneous rate is the rate of the reaction at a specific point in time, derived from the slope of the concentration-time graph at that point. The initial rate is the instantaneous rate at the very start of the reaction, essentially the slope of the tangent to the concentration-time graph at time equals zero.
How is the average rate of a reaction determined?
-The average rate of a reaction is an approximation of the instantaneous rate and is calculated by taking the difference in concentration of a reactant or product over a certain time period and dividing it by the time elapsed between two different time points.
What are the two common experimental methods for measuring the rate of a reaction?
-The two common experimental methods are the continuous method, which tracks the concentration of reactants or products over time, and the clock method, which measures the time taken for a prominent visual change corresponding to a certain change in concentration.
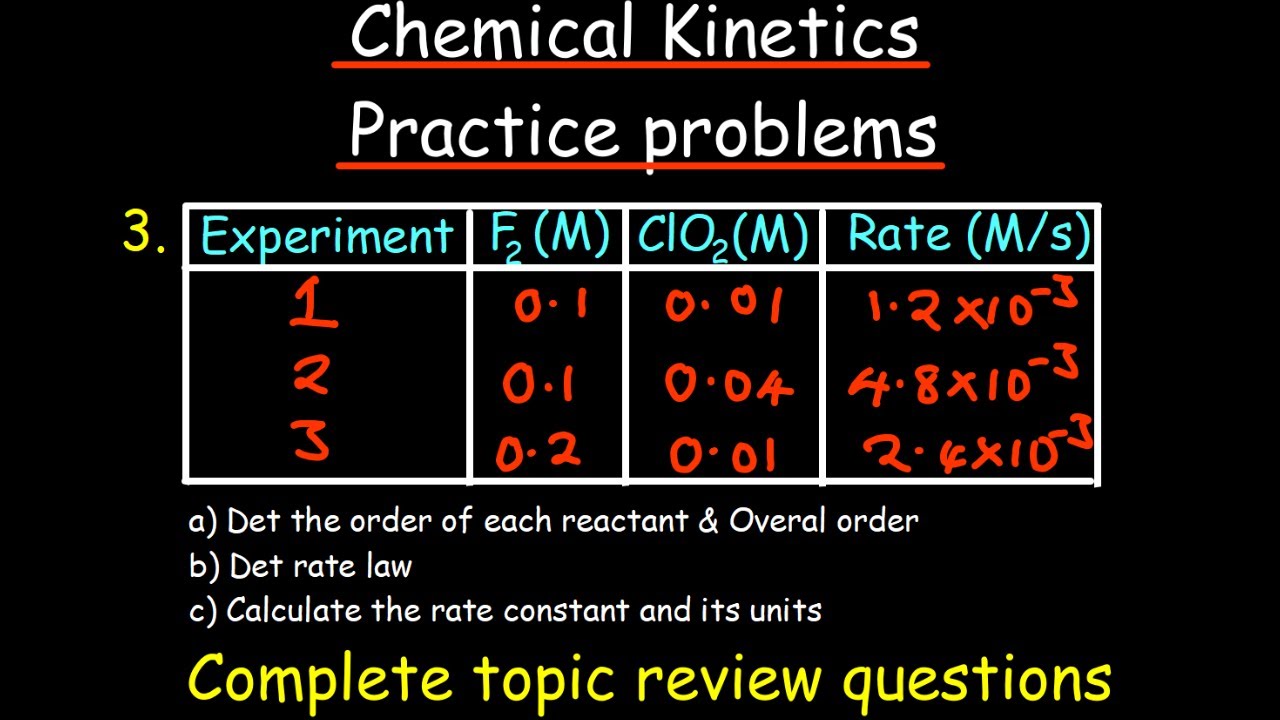
How does the rate of a reaction depend on the concentration of reactants?
-The rate of a reaction can be expressed as a function of the concentration of reactants through the rate equation, which typically includes terms raised to powers that represent the reaction orders with respect to each reactant.
What is the significance of the rate constant (k) in the rate equation?
-The rate constant (k) in the rate equation is a proportionality constant that accounts for the temperature and activation energy of the reaction. It increases with temperature and is affected by the presence of a catalyst, which can lower the activation energy and increase k.
Can you explain the concept of half-life in the context of first-order reactions?
-In first-order reactions, the half-life is the time it takes for the concentration of a reactant to decrease to half its initial value. It is a constant value at a given temperature and activation energy and is independent of the initial concentration of the reactant.
What is the difference between a zero-order and a pseudo-zero-order reaction?
-A zero-order reaction is one where the rate is independent of the concentration of the reactants, with the rate constant at a given temperature. A pseudo-zero-order reaction appears to be zero-order because the concentration of one reactant is much larger than the other, making the change in its concentration negligible over the course of the reaction.
How does the presence of a catalyst affect the rate of a reaction?
-A catalyst affects the rate of a reaction by providing an alternative pathway with a lower activation energy. This allows more particles to have sufficient energy to react, thus increasing the rate of the reaction without being consumed in the process.
What is the relationship between the reaction rate equation and the reaction mechanism?
-The reaction rate equation is derived from the reaction mechanism, which is a step-by-step description of how a reaction proceeds. The slowest step in the mechanism determines the overall rate of the reaction, and the rate equation reflects the concentrations of reactants involved in this slow step.
Outlines
🔍 Introduction to Reaction Kinetics
This paragraph introduces the fundamental concept of reaction kinetics, which is the study of the speed at which chemical reactions occur. It explains the rate of a reaction in terms of the change in concentration of reactants and products over time, and how this rate can be defined using the stoichiometric coefficients. The paragraph distinguishes between instantaneous rate, which is the derivative of concentration with respect to time at a specific point, and initial rate, which is the instantaneous rate at the start of the reaction. It also covers average rate, which is an approximation of the instantaneous rate, calculated as the change in concentration over a time interval. The paragraph concludes with a discussion on experimental methods to measure reaction rates, such as the continuous method and the clock method, which observe changes in concentration over time or measure the time for a significant visual change, respectively.
🌡 Factors Influencing Reaction Rates
This paragraph delves into the factors that affect the rate of a chemical reaction, such as the concentration of reactants, temperature, the presence of a catalyst, and the volume of the reaction mixture. It emphasizes that the rate of reaction is directly related to the concentration of the reactants, and this relationship can be expressed through a rate equation. The paragraph introduces the concept of reaction orders, where the rate depends on the concentration raised to a power, and the rate constant, which is influenced by temperature and activation energy. It also discusses different types of reactions based on their order, such as first-order reactions with a constant half-life, and second-order reactions, which can be dependent on one or two reactants raised to the power of one or two, respectively.
📚 Pseudo-First Order Reactions and Catalysts
The paragraph explores pseudo-first order reactions, where the concentration of one reactant is significantly larger than the other, making the percentage change in its concentration negligible compared to the change in the other reactant. This allows the reaction to be treated as if it were a first-order reaction, simplifying the analysis of the reaction rate. It also discusses the role of catalysts in reactions, which are not consumed in the overall reaction but help lower the activation energy, thus increasing the rate constant and the rate of the reaction. The paragraph provides an example of a reaction where the concentration of a reactant is effectively constant, allowing for a simplified rate equation and half-life calculation.
🔬 Macroscopic Basis of Reaction Kinetics
This paragraph provides a deeper understanding of the macroscopic basis of reaction kinetics, focusing on the activation energy and its effect on the rate constant. It introduces the Arrhenius equation, which relates activation energy, temperature, and the rate constant. The paragraph explains how a decrease in activation energy, such as through the use of a catalyst, increases the rate constant and thus the reaction rate. It also discusses the Maxwell-Boltzmann distribution, illustrating how a catalyst or an increase in temperature can increase the proportion of particles with sufficient energy to undergo a productive collision, leading to a faster reaction rate.
🔄 Relationship Between Reaction Rate Equation and Mechanism
The paragraph examines the relationship between the reaction rate equation and the reaction mechanism, which is a series of steps that describe how a reaction proceeds. It explains that the slowest step in the mechanism determines the overall rate of the reaction, and this step's rate equation must be consistent with experimental observations. The paragraph uses an example to illustrate how the rate equation can be derived from a proposed mechanism and how it can be used to validate or refute different mechanisms based on experimental data. It concludes by emphasizing the importance of understanding both the conceptual and application aspects of reaction kinetics, including the ability to manipulate mathematical equations.
Mindmap
Keywords
💡Reaction Kinetics
💡Rate of Reaction
💡Stoichiometric Coefficient
💡Instantaneous Rate
💡Initial Rate
💡Average Rate
💡Continuous Method
💡Clockwise Method
💡Rate Equation
💡Activation Energy
💡Catalyst
💡Arrhenius Equation
💡Maxwell-Boltzmann Distribution
💡Reaction Mechanism
💡Pseudo-First-Order Reaction
Highlights
Reaction kinetics is the study of the speed at which a reaction occurs.
The rate of a reaction can be defined based on the concentration change of reactants or products over time.
A unified definition of reaction rate involves dividing the rate of change by the stoichiometric coefficient.
Instantaneous rate is defined as the derivative of concentration at a specific time.
Initial rate is the instantaneous rate at time equals zero.
Average rate is an approximation of instantaneous rate and is calculated over a time interval.
Continuous methods track concentration changes over time to measure reaction rates.
Clock method measures the time for a prominent visual change in the reaction, corresponding to concentration changes.
Reaction rate depends on the concentration of reactants, temperature, presence of catalysts, and activation energy.
The rate equation expresses how the rate of a reaction depends on the concentration of reactants.
Reaction orders (x and y) and the rate constant (K) are determined experimentally.
First-order reactions have a constant half-life, independent of the initial concentration.
Second-order reactions can depend on the concentration of one or two reactants raised to the power of one.
Zero-order reactions have a constant rate that is independent of reactant concentrations.
Pseudo first-order reactions occur when the concentration of one reactant is much larger than the other, simplifying the rate equation.
Catalysts lower the activation energy and increase the rate constant, thus speeding up reactions.
The Arrhenius equation relates activation energy, temperature, and the rate constant.
Maxwell-Boltzmann distribution curves illustrate the effect of temperature and catalysts on the energy of particles in a reaction.
The relationship between reaction rate equations and reaction mechanisms is crucial for understanding the steps of a reaction.
Experimental data can be used to deduce the most likely reaction mechanism based on the observed rate equation.
Conceptual understanding and application are key to mastering the concepts of reaction kinetics.
Additional topics such as planning kinetics experiments and understanding catalysts are important but not covered in this transcript.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: