Lec 36 | MIT 18.01 Single Variable Calculus, Fall 2007
TLDRThis MIT OpenCourseWare lecture delves into the concept of dealing with infinity in calculus, focusing on L'Hopital's Rule for indeterminate forms and the comparison of growth rates of functions. The professor introduces notation to denote functions that grow much more slowly than others and discusses improper integrals, providing a clear definition and exploring their convergence or divergence. Key examples include integrals involving exponential decay and polynomial functions, highlighting their applications in physics and probability. The lecture also covers limit comparison techniques and the importance of understanding tails in probability and financial mathematics, offering both theoretical insights and practical applications.
Takeaways
- 📚 The lecture continues with Unit 5, focusing on 'Dealing With Infinity', particularly in relation to limits and integrals.
- 🏥 L'Hopital's Rule is reviewed, specifically for the case of indeterminate forms of infinity (∞/∞), with the hypothesis that both functions tend to infinity and their derivatives' ratio has a limit L.
- 🔍 The professor introduces a notation to compare the growth rates of functions asymptotically, using 'f(x) is a lot less than g(x)' to indicate that the limit of f(x)/g(x) as x approaches infinity is 0.
- 📈 Emphasis is placed on understanding rates of growth and decay of functions, with examples provided to illustrate the concepts, such as ln(x) growing slower than x to a power.
- ∫ The concept of improper integrals is introduced, with a focus on determining whether they converge (have a finite value) or diverge (do not have a finite value).
- 📉 The integral from 'a' to infinity of f(x) dx is defined as the limit as n approaches infinity of the integral from 'a' to 'n' of f(x) dx, with convergence depending on the existence of this limit.
- 🌟 A fundamental improper integral example is given: the integral from 0 to infinity of e^(-kx) dx, which converges to 1/k for a positive constant k.
- 📊 The physical interpretation of certain integrals is discussed, such as the integral representing the total number of particles in a radioactive substance as time approaches infinity.
- 🧩 The importance of understanding the 'tail' of a distribution is highlighted, as it is crucial for determining the negligibility of unlikely events in various fields like financial mathematics.
- 🔢 The borderline case of integrals involving powers of x is explored, with the integral from 1 to infinity of dx/x^p diverging if p <= 1 and converging to 1/(p-1) if p > 1.
- 📚 Limit comparison technique is introduced as a method to determine the convergence or divergence of an integral by comparing it to another integral with known convergence properties.
Q & A
What is the informal title of Unit 5 that the professor is discussing?
-The informal title of Unit 5 is 'Dealing With Infinity', which focuses on topics like limits and integrals with an emphasis on infinity.
What are the three hypotheses for applying L'Hopital's Rule as stated in the lecture?
-The three hypotheses for applying L'Hopital's Rule are: 1) f(x) tends to infinity, 2) g(x) tends to infinity, and 3) the derivative of f(x) divided by the derivative of g(x) tends to a limit L, all as x tends to some limit a.
Can L'Hopital's Rule be applied when the limit L or the number a is infinite?
-Yes, L'Hopital's Rule can be applied when the limit L and the number a are infinite, as long as the conditions for the rule are met.
What does the notation 'f(x) is a lot less than g(x)' signify in the context of the lecture?
-The notation 'f(x) is a lot less than g(x)' signifies that the limit of f(x)/g(x) as x approaches infinity tends to 0, indicating that f(x) grows at a much slower rate than g(x) asymptotically.
How does the professor describe the rates of growth for functions like ln(x), x^n, and e^x?
-The professor describes the rates of growth as follows: ln(x) grows a lot more slowly than x to a power, which in turn grows more slowly than e^x, and e^x^2 grows the fastest of all, tending to infinity at different rates.
What is the definition of an improper integral from a to infinity of f(x) dx?
-The definition of an improper integral from a to infinity of f(x) dx is the limit as n goes to infinity of the ordinary definite integral from a to n of f(x) dx.
What does it mean for an integral to converge or diverge?
-An integral converges if the limit exists and the total area under the curve is finite. It diverges if the limit does not exist or the total area is infinite.
Can you provide an example of an improper integral and its evaluation?
-An example of an improper integral is the integral from 0 to infinity of e^-kx dx, where k is a positive constant. The evaluation involves finding the antiderivative and taking the limit as the upper limit of integration goes to infinity, which results in a finite value of 1/k.
What is the physical interpretation of the integral from 0 to infinity of e^-kx dx in the context of radioactive decay?
-In the context of radioactive decay, the integral from 0 to infinity of e^-kx dx represents the total number of particles that decay in a radioactive substance over an infinite amount of time, which is an idealization used for theoretical calculations.
What is the significance of the integral from minus infinity to infinity of e^-x^2 dx in probability and statistics?
-The integral from minus infinity to infinity of e^-x^2 dx is significant in probability and statistics because it evaluates to the square root of pi. This constant is key to understanding standard deviation and other concepts in probability, as well as for calculating confidence intervals in polling and insurance mathematics.
What is the concept of limit comparison in the context of improper integrals?
-Limit comparison is a method used to determine the convergence or divergence of an improper integral by comparing it to another integral whose convergence properties are already known. If two functions are asymptotically similar as x approaches infinity, their integrals from some point to infinity will either both converge or both diverge.
How does the integral from 1 to infinity of dx/x^p behave with respect to the value of p?
-The integral from 1 to infinity of dx/x^p diverges if p <= 1 and converges if p > 1. The convergence for p > 1 can be evaluated to a finite value of 1/(p-1).
What is an improper integral of the second type and how does it differ from the first type?
-An improper integral of the second type involves an integral with an integrand that has a singularity, typically at one of the limits of integration, such as 0 or infinity. This differs from the first type, which involves limits of integration that extend to infinity. The second type requires careful consideration of the singularity and may not always yield a meaningful value without additional context or methods.
Outlines
📚 Introduction to Dealing with Infinity in Mathematics
This paragraph introduces the topic of dealing with infinity in mathematical contexts, specifically within the realms of limits and integrals. The professor begins by discussing the importance of MIT OpenCourseWare and its reliance on donations to provide free educational resources. The main focus then shifts to L'Hopital's Rule, which is a method for finding limits, particularly in cases where the limit results in infinity over infinity. The professor clarifies the hypotheses and the conclusion of the rule, emphasizing that it applies when both functions involved tend towards infinity and the derivative of the ratio tends to a limit L. An interesting point is made regarding the rule's applicability even when the limit L and the variable a are infinite. The paragraph concludes with an introduction to the concept of comparing growth rates of functions, introducing a notation to denote when one function grows significantly slower than another in the limit as x approaches infinity.
📈 Rates of Growth and Decay in Functions
The second paragraph delves into the comparison of rates of growth and decay of functions. The professor discusses how different functions approach infinity at varying rates, using the natural logarithm, exponential functions, and powers of x as examples. A similar approach is taken for rates of decay, which are the speeds at which functions approach zero. The reciprocals of these functions are used to illustrate decay rates, with the professor emphasizing the importance of understanding these rates in relation to improper integrals. The concept of improper integrals is introduced, with a focus on integrals that extend to infinity and the conditions under which they converge or diverge. The professor also touches on the geometric interpretation of convergence, relating it to the finiteness of the area under the curve. The paragraph concludes with a discussion of the integral from a to infinity of f(x) dx, highlighting the importance of determining whether this integral converges to a finite value or diverges to infinity.
🧩 Exploring Improper Integrals and Their Convergence
In this paragraph, the professor provides a detailed exploration of improper integrals, focusing on their convergence properties. The integral from 0 to infinity of e^(-kx) dx is examined, where k is a positive constant. The antiderivative of this function is calculated, and the limit as n approaches infinity is taken to determine the convergence of the integral. The professor uses this example to illustrate the concept of convergence and divergence in the context of improper integrals. A shorthand method for calculating such integrals is introduced, emphasizing the importance of paying attention to the signs and the behavior of the function as the limit is approached. The paragraph also addresses a common question about the use of infinity as a limit and the conditions under which the integral converges to a finite value. The professor encourages students to practice these techniques, as they are applicable to various problems in mathematics.
🔬 Physical Interpretation and Radioactive Decay
The fourth paragraph discusses a physical interpretation of the integrals covered in the lecture, specifically relating to radioactive decay. The professor explains how the integral from 0 to infinity of e^(-kx) dx can be used to model the average number of particles that decay in a radioactive substance over time. The limit as the time T approaches infinity is considered, and it is shown that this integral equals the total number of particles in the substance. The professor emphasizes that while waiting for an infinite amount of time is not realistic, the theoretical concept is useful for normalization and understanding the total quantity of a substance. The simplicity of the resulting formula is highlighted, showing that the limit process can lead to more elegant expressions, which is a recurring theme in mathematics.
📉 The Significance of Tails in Probability and Finance
This paragraph highlights the importance of understanding the tails of probability distributions, particularly in the context of probability and financial mathematics. The professor discusses how the tails represent the likelihood of unlikely events, which can have significant impacts, as seen in financial scandals like the mortgage crisis. The focus is on the need for mathematicians to determine which parts of a distribution can be considered negligible in finite calculations and which require theoretical reasoning. The concept of 'fat tails' is introduced, referring to events with low probability but high impact. The professor emphasizes the importance of getting the mathematics right in these areas, as they can have serious real-world consequences.
🔍 Analyzing Borderline Cases of Convergence and Divergence
The sixth paragraph focuses on analyzing borderline cases of convergence and divergence in improper integrals. The integral from 1 to infinity of 1/x^p dx is examined, with p being a variable power. The professor demonstrates that when p equals 1, the integral diverges, serving as a borderline case between convergence and divergence. A systematic approach is taken to evaluate the integral for different powers p, showing that the integral diverges if p is less than or equal to 1 and converges if p is greater than 1. The antiderivative is used to determine the behavior of the integral, and the professor emphasizes the importance of careful calculation and understanding the mathematical properties at play.
📐 Limit Comparison Test for Improper Integrals
In this paragraph, the professor introduces the limit comparison test, a method for determining the convergence of improper integrals when the integral itself cannot be easily evaluated. The test is based on comparing the given integral with another whose convergence properties are already known. If the ratio of the two functions tends to 1 as x approaches infinity, then both integrals converge or diverge simultaneously. The professor provides examples to illustrate the application of the limit comparison test, showing how it can be used to quickly determine the convergence of an integral without needing to compute it. The examples demonstrate the power of this method in analyzing the behavior of integrals at infinity.
📘 Convergence Assessment Using Comparison and Limit Comparison
The eighth paragraph continues the discussion on assessing the convergence of improper integrals, focusing on the use of comparison and limit comparison methods. The professor presents an example involving the integral from 0 to infinity of dx/(sqrt(x^2+10)), which cannot be easily evaluated. By comparing this integral to a known convergent integral, the professor demonstrates how to determine its convergence properties. The importance of avoiding the origin when making these comparisons is emphasized, as the behavior of the functions at the origin can be misleading. The professor also addresses a student's question about the choice of changing the limits of integration and explains the rationale behind it.
🚫 The Pitfalls of Improper Integrals with Singularities
The final paragraph of the script addresses the challenges associated with improper integrals that have singularities, a second type of improper integral. The professor warns of the potential for incorrect results when calculating these integrals without considering their convergence. An example is given where the integral from -1 to 1 of dx/x^2 results in a seemingly nonsensical negative value, highlighting the importance of understanding the nature of the integral before attempting to calculate it. The professor emphasizes the need to be cautious and to consider the entire behavior of the function, especially near points of discontinuity or infinity, to avoid falling into such traps.
Mindmap
Keywords
💡Creative Commons license
💡L'Hopital's Rule
💡Infinity
💡Improper Integrals
💡Convergence
💡Divergence
💡Asymptotic Behavior
💡Rates of Growth
💡Rates of Decay
💡Limit Comparison
💡Singularity
Highlights
Introduction to Unit 5: Dealing With Infinity, focusing on limits and integrals.
Explanation of L'Hopital's Rule, specifically the infinity/infinity case.
Three hypotheses of L'Hopital's Rule: f(x) tends to infinity, g(x) tends to infinity, and f'(x)/g'(x) tends to a limit L.
Introduction of new notation for comparing functions: f(x) is a lot less than g(x).
Discussion of rates of growth and decay, using examples like ln(x), x^p, e^x, and e^x^2.
Introduction to improper integrals and their significance in mathematical analysis.
Definition and distinction between convergent and divergent integrals.
Example calculation: integral from 0 to infinity of e^-kx dx, demonstrating convergence to 1/k.
Explanation of why improper integrals are used in theoretical calculations, even if not physically realistic.
Historical context: calculation of the integral from minus infinity to infinity of e^-x^2 dx equals the square root of pi.
Introduction to the concept of limit comparison for integrals.
Example of limit comparison: integral from 1 to infinity of 1/sqrt(x^3 + 3) dx, showing convergence.
Discussion of improper integrals of the second type, focusing on singularities and their impact.
Cautionary example of incorrect integral calculation: integral from -1 to 1 of 1/x^2 dx, demonstrating divergence.
Summary of the importance of understanding convergence and divergence in practical applications like financial mathematics.
Transcripts
Browse More Related Video

Lec 37 | MIT 18.01 Single Variable Calculus, Fall 2007

Using L'Hopital's Rule to show that exponentials dominate polynomials
Calculus Chapter 1 Lecture 9 BONUS

Unit VI: Lec 4 | MIT Calculus Revisited: Single Variable Calculus

Unit VII: Lec 2 | MIT Calculus Revisited: Single Variable Calculus
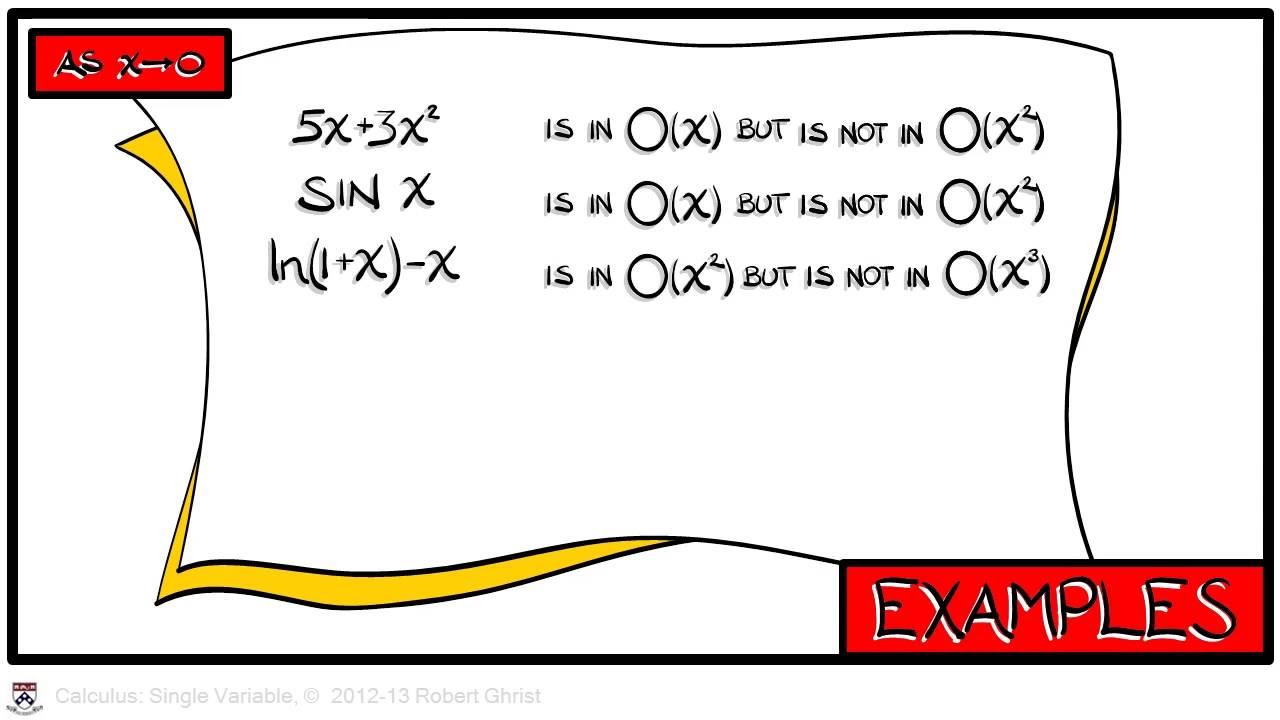
Calculus Chapter 1 Lecture 9 Orders of Growth
5.0 / 5 (0 votes)
Thanks for rating: