Calculus 1: Shell Method Examples
TLDRThis educational video script explains the shell method for calculating volumes of solids formed by revolving shapes around axes. It illustrates the process with examples, starting with a thin cylindrical shell created by revolving a rectangle around the y-axis. The script guides through setting up the integral for the volume, using the formula for the volume of a shell and integrating from 0 to 2. It then changes the problem to involve more complex functions and suggests using integration by parts, a technique not covered in the script. Finally, it demonstrates finding the volume of a solid created by revolving a region around the x-axis, involving solving for x and integrating with respect to y. The summary concludes with a call to action, directing viewers to the speaker's website for more calculus examples and solutions.
Takeaways
- 📚 The video discusses the shell method for calculating volumes of solids, an alternative to the washer method.
- 🔄 The shell method involves revolving a shape around an axis to create a solid, and then finding its volume by integrating the 'shells' formed.
- 📏 A representative rectangle is used to represent each shell, with its dimensions determined by the shape's geometry and the axis of rotation.
- 📐 The volume of each shell is calculated by multiplying the thickness (dx or dy), the height (a function of x or y), and the circumference of the circle formed by the revolution.
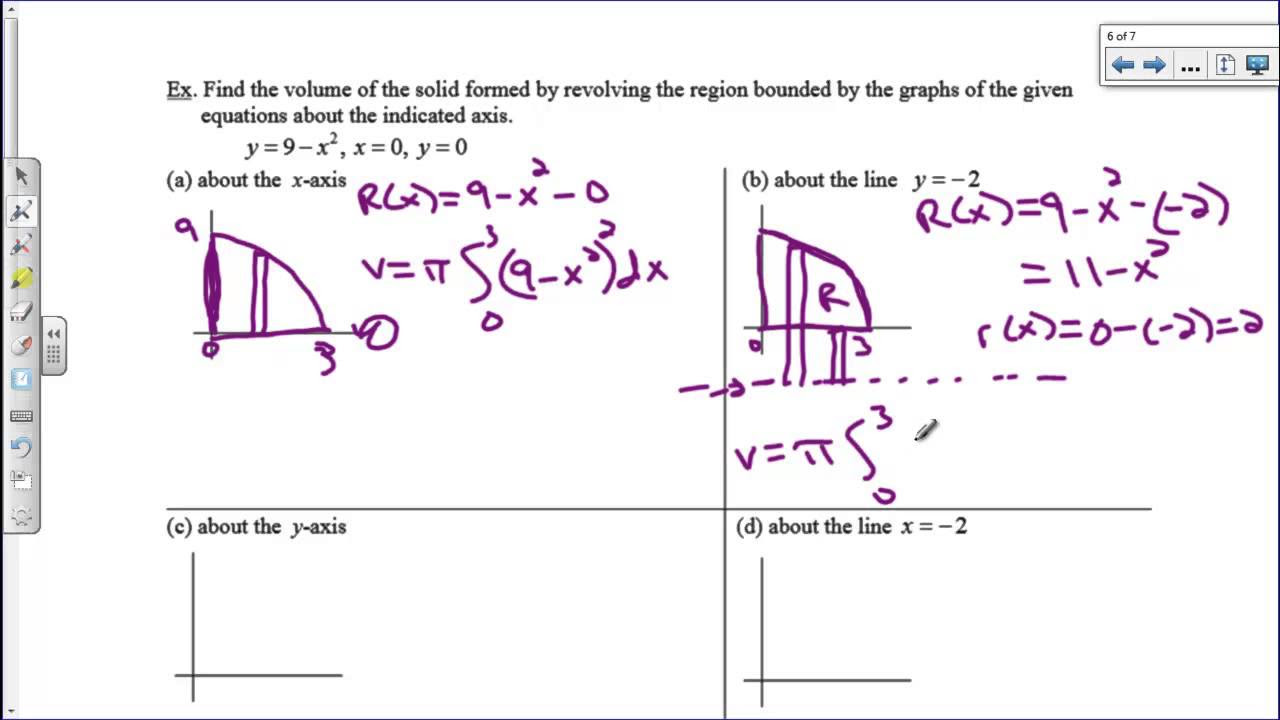
- 📉 The first example involves revolving a rectangle around the y-axis, creating a cylindrical shell with a volume formula involving (3 - x^2), 2πx, and dx.
- 🧩 The integration for the first example is performed over a range from 0 to 2, avoiding double-counting by not extending to negative values.
- 🤔 The second example involves revolving a rectangle around the y-axis with a height of cos(x), but the integral is not computed due to the complexity of the function.
- 🔄 The third example uses the shell method to find the volume when revolving around the x-axis, with the height determined by the difference in x-values and the radius as the y-value.
- ✂️ The integral for the third example involves a substitution to simplify the expression and requires careful handling of the bounds due to the negative sign.
- 📝 The final calculation for the third example results in a volume expressed in terms of sqrt(3), demonstrating the use of integration to find the volume of a complex solid.
- 🔗 The video concludes with an invitation to visit the speaker's website for more examples and step-by-step solutions to calculus problems.
Q & A
What is the shell method used for in the context of finding volumes?
-The shell method is used to find the volume of a solid created by revolving a shape around an axis. It involves creating a representative rectangle and revolving it around the axis to form a thin cylindrical shell, which can then be integrated to find the volume.
Why might the shell method be easier to use than the washer method for certain volumes?
-The shell method might be easier to use because it can simplify the process of finding volumes for shapes that naturally lend themselves to being 'unrolled' into a flat rectangle, making the integration process more straightforward.
How is the representative rectangle used in the shell method?
-The representative rectangle is used to visualize the shape that would be created if the shell were unrolled. This helps in determining the dimensions needed for the volume calculation, such as the height and the length (which becomes the circumference times the radius after unrolling).
What is the formula for the volume of a cylindrical shell created by revolving a representative rectangle around the y-axis?
-The formula for the volume of a cylindrical shell is the thickness (dx) times the height (y-value of the curve) times the circumference of the circle (2π times the radius), which in this case is 2π times the x-value, resulting in the volume formula: (3 - x^2) * 2πx * dx.
What is the range of integration for the volume calculation in the example provided?
-The range of integration for the volume calculation is from 0 to 2, as this range encompasses all the rectangles created from revolving the shape around the y-axis without double-counting.
How does the thickness of the cylindrical shell relate to the variable dx?
-The thickness of the cylindrical shell is represented by dx, which signifies an infinitesimally small change in the x-direction as the shape is revolved around the axis.
What is the significance of the y-value in the volume formula for a cylindrical shell?
-The y-value in the volume formula represents the height of the representative rectangle after it has been unrolled. It is crucial for determining the volume of the shell as it contributes to the area that will be multiplied by the circumference of the circle.
Can you provide an example of a setup for an integral using the shell method that involves revolving around the x-axis?
-An example setup for an integral using the shell method that involves revolving around the x-axis is when the height of the shell is given by the x-value minus another x-value, and the radius is the y-value. The integral would then be set up as an integration over the difference in x-values times the circumference times the radius, with the bounds determined by the limits of the y-value.
What technique is suggested for solving the integral involving cos(x)/x?
-Integration by parts is suggested for solving the integral involving cos(x)/x, as it is a technique that can handle the product of two functions where one function is the derivative of the other.
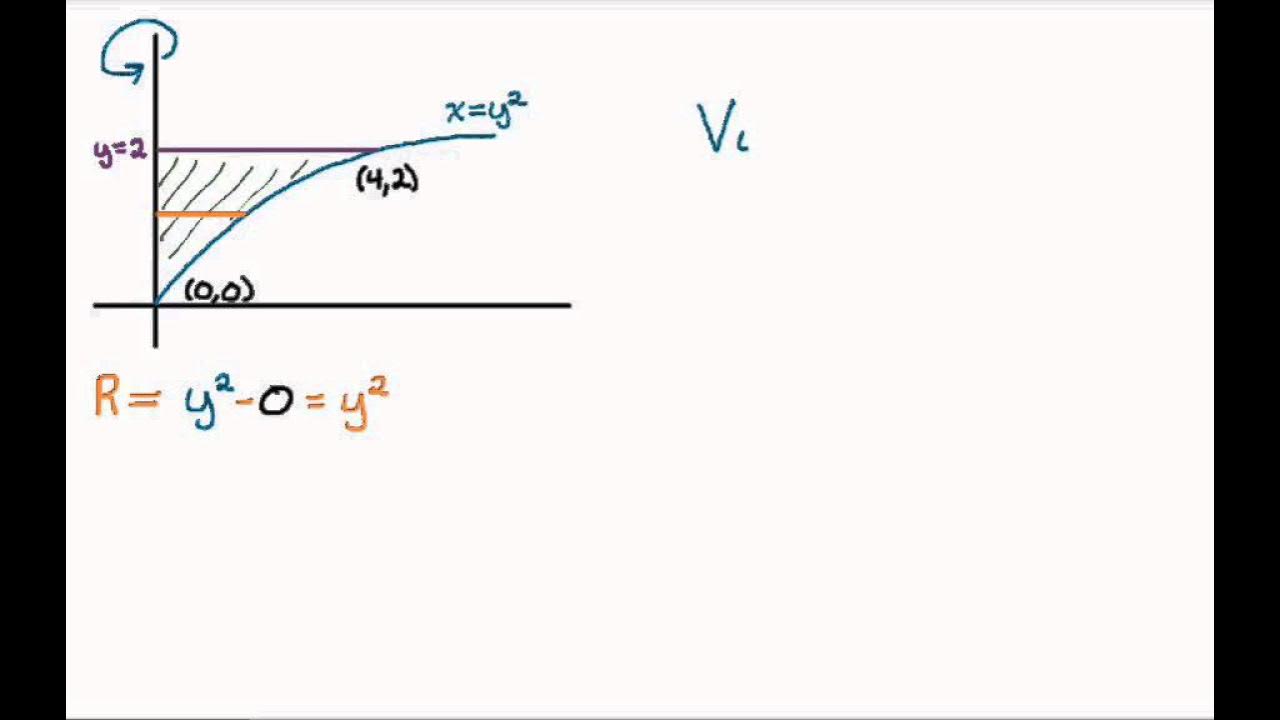
How does the setup for the integral change when the solid is revolved around the x-axis instead of the y-axis?
-When the solid is revolved around the x-axis, the setup for the integral changes to consider the thickness in the y-direction (dy), the height determined by the difference in x-values, and the circumference of the circle being 2π times the y-value. The integral is then set up accordingly with the appropriate bounds for y.
What substitution is used to simplify the integral involving 2y√(3-y) dy?
-A u-substitution is used to simplify the integral involving 2y√(3-y) dy, where y is replaced with 3-u, allowing the integral to be expressed in terms of u and making it easier to integrate.
How is the final volume calculated after performing the integral?
-The final volume is calculated by evaluating the integral over the specified bounds and then simplifying the resulting expression to obtain the total volume of the solid formed by revolving the shape around the axis.
Outlines
📚 Introduction to the Shell Method for Volume Calculations
This paragraph introduces the shell method, an alternative technique to the washer method, for calculating volumes of solids formed by revolving shapes around an axis. The focus is on creating a representative rectangle and revolving it around the y-axis to form a cylindrical shell. The volume of this shape is determined by considering the thickness (dx), height (y-value of the curve, 3 - x^2), and the circumference of the circle (2π times the radius, which is the x-value). The integral of the product of these dimensions from 0 to 2 gives the total volume, avoiding double-counting by not extending to negative values. The integral simplifies to 2π times (3/2 * x^2 - 1/4 * x^4) evaluated from 0 to 2, resulting in a volume of 4π. The paragraph also touches on revolving a rectangle around the y-axis with thickness dx, height as cosine x, and radius as x, leading to an integral of 2π * x * cosine x * dx, but acknowledges the complexity of solving this integral without certain techniques.
🔍 Advanced Shell Method Application and Integration Techniques
The second paragraph delves into more complex applications of the shell method, specifically revolving around the x-axis. It discusses setting up the shells to calculate the volume, considering the thickness (dy), height as the difference in x-values (2 * sqrt(3 - y)), and radius as the y-value. The integral to solve involves 2y * sqrt(3 - y) dy, with the limits from 0 to 3. The paragraph explains the necessity of a u-substitution for solving the integral, where y is substituted with 3 - u, leading to a new integral in terms of u. After performing the substitution and adjusting the bounds, the integral simplifies to 2 * (3^(3/2) - 2/5 * 3^(5/2)). The final calculation results in a volume of 24/5 * sqrt(3). The paragraph concludes with a resource for further learning, directing viewers to a website with over 400 calculus problems solved step-by-step.
Mindmap
Keywords
💡Shell Method
💡Volume
💡Representative Rectangle
💡Revolve
💡Thickness
💡Y-Axis
💡Circumference
💡Radius
💡Integration
💡U-Substitution
Highlights
Introduction to the shell method for finding volumes.
Comparison between the washer method and shell method.
Explanation of creating a representative rectangle and revolving it around the y-axis.
Visualizing the shape as a thin cylindrical shell.
Unrolling the cylindrical shell into a flat piece to understand its volume.
Formula for the volume involving the thickness (dx), height (3 - x^2), and circumference (2πx).
Integrating the volume from x = 0 to x = 2 to avoid double-counting.
Simplification of the integral to 2π times the integral of (3x - x^3) dx.
Evaluation of the integral resulting in the final volume calculation.
Second example involving cosine function and revolving around the y-axis.
Setup of the integral involving 2πx cos(x) dx.
Adjusting the problem to make it solvable with current techniques.
Use of u-substitution to solve integrals involving square root functions.
Detailed steps in converting and solving the integral with u-substitution.
Final volume calculation using the shell method for the given problem.
Invitation to visit the website for more examples and free access to calculus questions.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: