Calculus 1: Area Between Curves Examples

Allen Tsao The STEM Coach

5 Aug 201906:31

EducationalLearning

32 Likes 10 Comments

TLDRThis video script delves into the concept of using integrals to calculate the area between curves. It emphasizes the importance of understanding the process rather than just memorizing formulas. The instructor illustrates the method by breaking down the area into small rectangles, where the integral notation represents the summation of these rectangles' areas over a given interval. The script provides step-by-step examples, including simplifying the integrand and integrating from specific limits, to find the area under different functions. The examples range from simple rectangles to more complex functions like secant squared and sine. The video concludes with an invitation to visit the instructor's website for additional calculus problems and solutions.

Takeaways

📚 Integrals are used to calculate the area between curves by summing up the areas of small rectangles under the curve.
📐 The process involves setting up integrals to find the area by considering the height as the difference between the upper and lower function values (top - bottom).
📈 The integral notation represents the summation of the areas of these rectangles over a given interval, using the integral sign which resembles a 'sum'.
📉 To find the area under a curve, the height of each rectangle is determined by the value of the function at that point (f(x)) and the width is typically Δx or dx.
📏 The video script provides a step-by-step approach to setting up and solving integrals for areas between curves, emphasizing understanding over memorization.
🔍 The script illustrates the process with an example, showing how to find the height of a representative rectangle by subtracting the y-values of the top and bottom curves.
📝 The integral of the simplified integrand is then calculated over the specified interval, and the antiderivative is found to determine the area.
📉 The example provided in the script involves integrating from 0 to 2π, simplifying the integrand, and evaluating the antiderivative at the bounds of the interval.
📊 The final result of the integral gives the area between the curves, with the example yielding an area of π² + 4π.
📚 The script also briefly touches on the concept of integrating a constant value over an interval, which simply results in the constant times the length of the interval.
🔗 The video transcript ends with a call to action, inviting viewers to visit the speaker's website for more examples and step-by-step solutions to calculus problems.

Q & A

What is the fundamental concept of integrals as described in the script?
-The fundamental concept of integrals is to split an area into little rectangles, where the height of each rectangle is represented by the function value at a point (f(x)), and the width is represented by a small change in x (dx). The integral sums up the areas of all these rectangles over a given interval.
How does the script suggest to set up the integral for finding the area between two curves?
-The script suggests setting up the integral by first drawing a representative rectangle to visualize the problem. The height of the rectangle is the difference between the y-values of the top and bottom functions, and the width is dx. The integral is then the sum of the areas of these rectangles over the interval of interest.
What is the integral notation for the area of each rectangle under a curve?
-The integral notation for the area of each rectangle under a curve is f(x)dx, where f(x) represents the y-value (height) of the rectangle and dx represents the width.
What does the integral sign represent?
-The integral sign represents the sum of an infinite number of small areas (or rectangles) over a given interval. It is a stylized 'S' which stands for 'sum'.
How does the script explain the process of finding the area between two curves using integrals?
-The script explains that to find the area between two curves, one should consider the upper function minus the lower function to get the height of the rectangle, multiply it by the width dx, and then integrate over the interval from x=0 to x=2π.
What is the simplified integrand for the example given in the script?
-The simplified integrand for the example is 1/2x + 2 - 1/2cos(x), which is obtained by combining the terms 1/2x + 3 - 1/2cos(x) - 1.
What are the antiderivative functions for the simplified integrand in the example?
-The antiderivative functions for the simplified integrand are 1/4x^2 + 2x for the linear terms and -1/2sin(x) for the cosine term.
How does the script handle the evaluation of the antiderivative at the bounds of integration?
-The script evaluates the antiderivative at the upper bound (2π) and subtracts the evaluation at the lower bound (0) to find the definite integral, which gives the area between the curves over the interval [0, 2π].
What is the final result of the integral for the example given in the script?
-The final result of the integral for the example is π^2 + 4π, obtained by evaluating the antiderivative at the bounds and simplifying the expression.
How does the script approach the integral of a rectangle with a constant height?
-For a rectangle with a constant height, the script suggests integrating x from 0 to π, since the width is dx and the height is constant (1 in the example), resulting in an integral of x which evaluates to π.
What is the antiderivative of secant squared x as mentioned in the script?
-The antiderivative of secant squared x is tan(x), and for sine 4x, it is 1/4 cosine(4x) due to the chain rule.

Outlines

00:00

📚 Calculating Area Between Curves Using Integrals

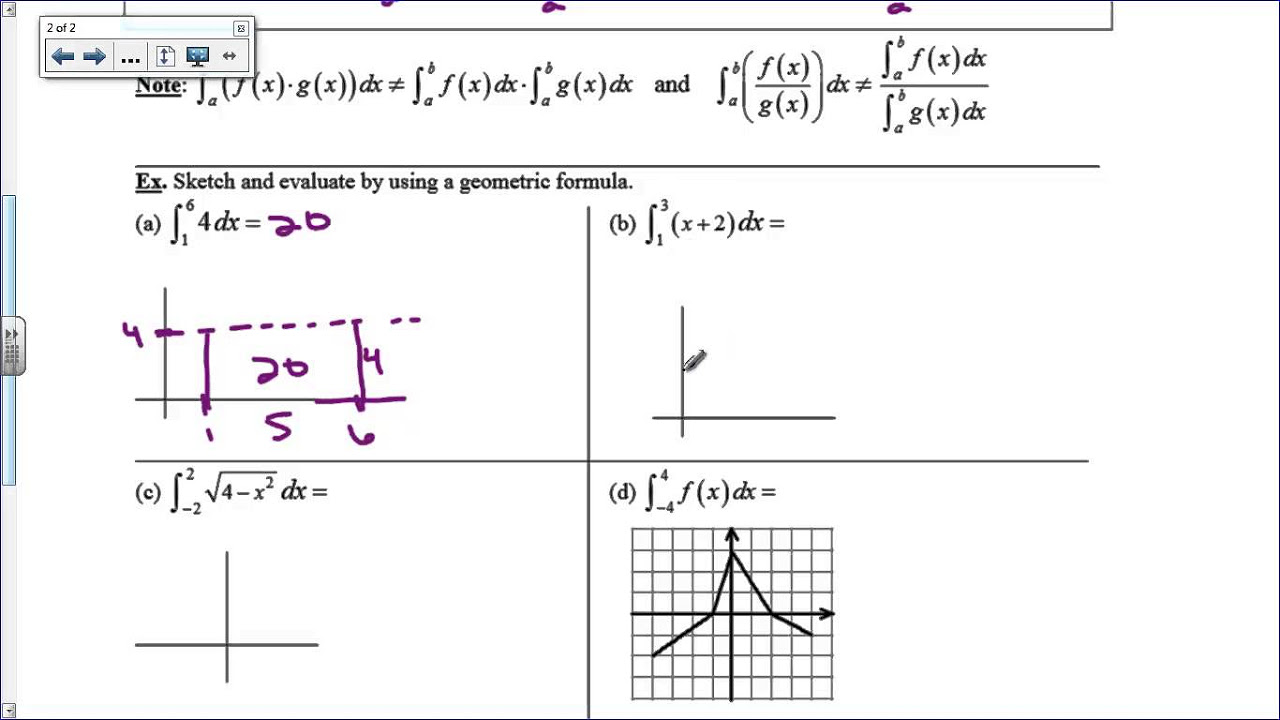
This paragraph introduces the concept of using integrals to calculate the area between two curves. The speaker emphasizes the importance of understanding the process rather than just memorizing formulas for later applications, such as volume problems. The fundamental idea of integrals is explained as summing up the areas of small rectangles under a curve. The height of each rectangle is determined by the y-value of the curve (f(x)) and the width is represented by Δx (written as dx). The integral notation is explained as summing the areas of these rectangles over a given interval. The speaker then demonstrates how to set up and simplify the integral for a specific example, integrating from x=0 to x=2π, and evaluates it to find the area. The process involves subtracting the lower function from the upper function to find the height of the rectangle and then integrating this difference over the specified interval.

05:01

📈 Applying Integrals to Find Area with Trigonometric Functions

In this paragraph, the speaker continues the discussion on integrals by focusing on calculating areas with trigonometric functions. The approach involves setting up integrals to find the area between the curves defined by secant squared and sine functions. The speaker explains how to find the height of the rectangle by subtracting the bottom y-value from the top y-value, resulting in the expression secant squared x minus sine 4x. The width is represented by dx, and the integration is performed over the interval from x=0 to x=π/4. The antiderivative of secant squared is tan x, and for sine 4x, it's 1/4 cosine 4x, with adjustments made for the chain rule. The final step involves evaluating the integral at the bounds of the interval, which yields a result of 1/2 after simplification. The speaker also invites viewers to visit their website for more examples and step-by-step solutions to over 400 calculus problems.

Mindmap

Keywords

💡Integrals

Integrals are a fundamental concept in calculus that represent the process of finding the area under a curve by summing up infinitely small rectangles. In the video, integrals are used to calculate the area between two curves, which is a common application. The script mentions integrals as a way to split an area into little rectangles and sum their areas, which is the core of the process being explained.

💡Area between curves

The area between curves is a specific application of integrals where the goal is to find the enclosed area between two curves on a graph. The video script discusses this concept by showing how to set up integrals to calculate this area, emphasizing the importance of understanding the process rather than just memorizing formulas.

💡Fundamental theorem of calculus

Although not explicitly mentioned in the script, the fundamental theorem of calculus is implicitly referenced as it provides the framework for calculating areas using integrals. The theorem connects differentiation and integration, allowing for the calculation of areas by integrating functions over an interval.

💡Representative rectangle

A representative rectangle is a visual tool used in the script to illustrate how integrals approximate areas under curves. The script describes how to draw a rectangle that represents the area under a curve and how the height of this rectangle is determined by the difference in y-values of the functions involved.

💡Height and width of rectangle

In the context of calculating areas using integrals, the height and width of a rectangle are critical components. The script explains that the height is determined by the y-value of the top function minus the y-value of the bottom function, while the width is represented by dx, indicating an infinitesimally small change in x.

💡Summation

Summation, represented by the integral sign in calculus, is the process of adding up a series of values. The script uses the term to describe the process of adding up the areas of all the small rectangles to find the total area under the curve, which is the essence of integration.

💡Antiderivative

An antiderivative is a function that represents the reverse process of differentiation. In the script, finding the antiderivative is part of the process of evaluating integrals. The antiderivative is used to find the accumulated area as x changes from one value to another.

💡Chain rule

The chain rule is a fundamental principle in calculus for differentiating composite functions. Although the script does not delve into the chain rule's details, it mentions it in the context of finding the antiderivative of a function involving a composite, such as cosine(4x), where the rule is implicitly used.

💡Secant squared and tangent

Secant squared and tangent are trigonometric functions used in the script to illustrate the process of finding the area between curves involving trigonometric expressions. The script shows how to set up and evaluate integrals involving these functions to find the area between the curves y = secant squared(x) and y = sine(4x).

💡Evaluation of integrals

Evaluating integrals involves finding the antiderivative and then applying the limits of integration to determine the area. The script demonstrates this process with examples, showing how to calculate the area by evaluating the antiderivative at the given limits of integration, such as from 0 to 2π or from 0 to π/4.

💡Trigonometric identities

Trigonometric identities are equations that relate different trigonometric functions and their values. The script uses these identities implicitly when simplifying expressions and evaluating integrals involving trigonometric functions, such as when simplifying sine and cosine functions.

Highlights

Introduction to the application of integrals for calculating the area between curves.

Explanation of the integral process as summing up the areas of little rectangles.

The integral notation represents the sum of the areas of rectangles over an interval.

Drawing a representative rectangle to visualize the problem-solving process.

Determining the height of the rectangle as the difference between the upper and lower y-values.

The width of the rectangle is represented by dx, integrating over the x-direction.

Summing up rectangles from x=0 to x=2π to find the total area.

Simplification of the integrand to prepare for integration.

Integration from 0 to 2π and the application of fundamental calculus rules.

Evaluating the integral at the bounds 0 and 2π to find the area.

Calculating the area as a rectangle with height 1 and width π.

Integration of secant squared and sine 4x to find the area between the curves.

Use of the chain rule in integration to handle the derivative of sine 4x.

Evaluating the integral from x=0 to x=π/4 to find the area.

Final calculation of the area using the values of tangent and cosine at the given bounds.

Conclusion with the final area calculation result.

Invitation to the speaker's website for more examples and step-by-step solutions.

Transcripts

Browse More Related Video

Calculus 1: Washer and Disk Method Examples

Riemann Sums and Definite Integrals

Calculus 1 Lecture 4.3: Area Under a Curve, Limit Approach, Riemann Sums

AP Calculus AB: Lesson 6.2 Part 2 (Limit Definition of Definite Integral)

Definite Integrals from Graphs (Part 1 of 3)

Math 1325 Lecture 13 2

Calculus 1: Area Between Curves Examples

Takeaways

Q & A

What is the fundamental concept of integrals as described in the script?

How does the script suggest to set up the integral for finding the area between two curves?

What is the integral notation for the area of each rectangle under a curve?

What does the integral sign represent?

How does the script explain the process of finding the area between two curves using integrals?

What is the simplified integrand for the example given in the script?

What are the antiderivative functions for the simplified integrand in the example?

How does the script handle the evaluation of the antiderivative at the bounds of integration?

What is the final result of the integral for the example given in the script?

How does the script approach the integral of a rectangle with a constant height?

What is the antiderivative of secant squared x as mentioned in the script?