Calculus 1: Related Rates Examples
TLDRThe video script discusses related rates problems, a common topic in AP Physics and calculus courses. The presenter illustrates how to approach these problems by using the example of a circular puddle forming from water flow and the rate of change of its radius. They assign variables, use volume equations, and apply differentiation to find the rate at which the radius grows. Another example involves calculating the rate at which an anti-aircraft gun must adjust its angle to track an F-22 aircraft flying at a certain velocity. The presenter uses trigonometric functions and differentiation to solve for the rate of change of the angle. The script concludes with an invitation to visit the presenter's website for more calculus examples and solutions.
Takeaways
- 📚 The video discusses related rates problems, a common topic in AP Physics and Calculus courses.
- 🔍 The key to solving related rates problems is to identify a variable that changes with respect to time and to set up an equation involving its derivative.
- 💧 An example problem involves water flowing into a flat surface to form a circular puddle, with the task of finding the rate at which the radius grows given certain conditions.
- 📐 The volume of the circular puddle is related to its radius and height, and the volume equation is used to find the derivative with respect to time.
- 🔢 Assigning variables and understanding their relationships is crucial for solving the problem, such as relating the radius and volume of the puddle.
- ⏱ The derivative of the volume equation with respect to time involves implicit differentiation and the chain rule.
- 🛫 Another example involves an F-22 aircraft flying at a certain velocity and the need to determine how fast an anti-aircraft gun can track it when the plane is one mile away.
- 📐 The relationship between the plane's position (X) and the angle of the gun's aim (theta) is established using the tangent function.
- 🔄 The derivative of the tangent function with respect to time is found using the chain rule and involves the secant squared function.
- 📏 The problem-solving process requires finding the value of theta when the plane is one mile away and then using it to calculate the rate of change of theta (d theta/dt).
- 🔢 Units conversion is necessary to ensure consistency, such as converting miles per hour to feet per second for the aircraft's velocity.
Q & A
What is a related rates problem?
-A related rates problem involves finding the rate at which one variable changes with respect to time by relating it to other variables that also change with respect to time.
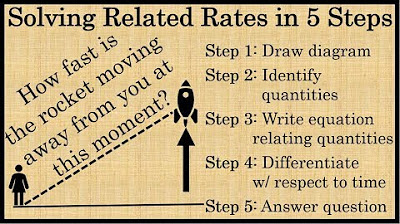
What is the general approach to solving related rates problems?
-The general approach involves identifying the variables that change with respect to time, finding an equation that relates these variables, and then taking the derivative of the equation with respect to time.
In the given example, what is the rate at which water flows into the flat surface?
-The rate at which water flows into the flat surface is 5 cubic centimeters per second.
How deep is the circular puddle mentioned in the problem?
-The circular puddle is 10 millimeters deep.
What is the goal in the first related rates problem involving the circular puddle?
-The goal is to find how fast the radius of the circular puddle is growing when the radius is 10 centimeters.
What equation relates the volume and radius of the circular puddle?
-The volume V of the puddle, modeled as a cylinder, is related to the radius r by the equation V = πr^2 * h, where h is the height. Given h = 1 centimeter, the equation simplifies to V = πr^2.
How do you find the derivative of the volume equation with respect to time?
-To find the derivative of the volume equation V = πr^2 with respect to time, you apply the chain rule: dV/dt = π * 2r * dr/dt.
What is the value of dr/dt when the radius is 10 centimeters?
-When the radius is 10 centimeters, dr/dt is 1/(4π) centimeters per second.
In the second related rates problem, what is the velocity of the aircraft?
-The aircraft is flying at 500 miles per hour.
What equation relates the angle θ and the distance x in the second problem?
-The equation relating the angle θ and the distance x is tan(θ) = 10,000/x.
How do you find the derivative of the angle equation with respect to time?
-To find the derivative of tan(θ) = 10,000/x with respect to time, you apply the chain rule: sec^2(θ) * dθ/dt = -10,000/x^2 * dx/dt.
How do you convert the aircraft's velocity from miles per hour to feet per second?
-To convert 500 miles per hour to feet per second, use the conversion factors: 1 mile = 5280 feet and 1 hour = 3600 seconds. The conversion gives 500 * 5280 / 3600 = 733.33 feet per second.
What is the final value of dθ/dt when the aircraft is one mile away?
-The final value of dθ/dt when the aircraft is one mile (5280 feet) away is approximately -0.057 radians per second.
Outlines
💧 Calculating the Rate of Growth of a Circular Puddle
This paragraph discusses a related rates problem involving a circular puddle of water. The problem states that water flows onto a flat surface at a rate of 5 cubic centimeters per second, forming a circular puddle that is 10 millimeters deep. The objective is to determine how fast the radius of the puddle is growing when the radius is 10 centimeters. The solution involves assigning variables to the given numbers and understanding the relationship between the radius and the volume of the puddle. The volume of a cylinder is used as the basis for the equation, which is then differentiated with respect to time. The resulting equation relates the rate of change of volume (dV/dt) to the radius and its rate of change (dr/dt). By substituting the given values and solving, the rate at which the radius is increasing is found to be 1/(4π) centimeters per second.
🚀 Tracking an Aircraft with an Anti-Aircraft Gun
The second paragraph presents a related rates problem concerning an F-22 aircraft flying at 500 miles per hour and an anti-aircraft gun that needs to track the plane. The scenario describes the plane's velocity and its distance from the gun, with the goal of finding out how fast the gun must adjust its angle to track the aircraft when it is one mile away. The solution involves relating the rate of change of the horizontal position (dx/dt) to the angle (theta) between the gun and the plane. Using the tangent function, the derivative of the tangent is taken to find d(theta)/dt. By substituting the known values, including the distance of one mile and the aircraft's velocity, the rate of change of the angle is calculated. The final step involves converting the aircraft's speed from miles per hour to feet per second and using trigonometric functions to find the angular velocity in radians per second, which is approximately -0.057 radians per second.
Mindmap
Keywords
💡Related Rates Problems
💡Variable
💡Derivative
💡Cylindrical Volume
💡Implicit Differentiation
💡Chain Rule
💡Rate of Change
💡Tangent Function
💡Secant Function
💡Trigonometric Functions
💡Dimensional Analysis
Highlights
Introduction to related rates problems commonly found in AP Physics and Calculus courses.
Key concept of related rates problems is identifying a variable changing with respect to time and formulating an equation.
Problem statement: Water flows into a flat surface forming a circular puddle with a rate of 5 cubic centimeters per second and a depth of 10 millimeters.
Objective: Determine the rate at which the radius of the puddle is growing when the radius is 10 centimeters.
Assigning variables and understanding the relationship between the depth, radius, and volume of the puddle.
Visualizing the problem in three dimensions to represent the circular puddle as a cylinder.
Volume of the cylinder is represented as π times the radius squared times the height.
Differentiating the volume equation with respect to time to find dV/dt.
Using the chain rule to differentiate the radius squared term with respect to time.
Solving for dr/dt, the rate of change of the radius with respect to time.
Calculating dr/dt to be 1/(4π) centimeters per second using the given volume rate and radius.
Problem statement: An F-22 aircraft flying at 500 miles per hour at an elevation of 10,000 feet.
Objective: Determine how fast an anti-aircraft gun can accurately track the aircraft when it is one mile away.
Relating the distance (X) and the angle (theta) using the tangent function.
Differentiating the tangent function with respect to time to find dtheta/dt.
Using the chain rule to relate dx/dt, x, and dtheta/dt in the derivative.
Calculating dtheta/dt using the given values and converting units from miles per hour to feet per second.
Final calculation of dtheta/dt resulting in a negative value, indicating the direction of the rate of change.
Invitation to visit the instructor's website for more examples and step-by-step solutions to calculus problems.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: