How to solve problem in mathematics with calculator (calculator tricks) part1

FUNAI TUTORIAL

18 Oct 202210:40

EducationalLearning

32 Likes 10 Comments

TLDRThis tutorial video offers essential calculator tricks to enhance math performance, particularly for SSCE and AF900 level exams on MTH101. The instructor demonstrates how to clear the calculator, solve equations with fractions and two unknowns, simplify logarithmic expressions, and evaluate logarithmic functions with different bases. The video also covers solving quadratic equations and finding specific values for variables in given equations. It emphasizes the importance of following the correct order of operations and understanding the calculator's functions for accurate results. Viewers are encouraged to subscribe and stay tuned for part two of the tutorial series.

Takeaways

🧐 Clear your calculator before starting calculations to avoid errors by pressing 'Shift', then 'Clear', and follow the prompts.
🔢 To solve equations with fractions, input the numerator and denominator correctly and use the 'Alpha' key to denote variables.
📚 For equations with two unknowns, use the 'Mode' and 'Equation' options on the calculator, ensuring equations are input in the correct order.
🔍 When simplifying logarithms, use the fraction button and ensure brackets are closed before inputting values to prevent errors.
📈 Understand that logarithms without a base are in base 10, and use the calculator's log functions accordingly.
📉 In solving equations involving square roots, use the square root button and ensure to press 'Default' to exit the square root mode.
🎯 For quadratic equations, use the 'Mode', 'Equation', and then 'Quadratic' options, inputting coefficients a, b, and c correctly.
🤔 Always compare the solutions of quadratic equations to determine the smaller value, as it may have more significant implications.
📑 When dealing with fractions in equations, replace variables with 'X' if necessary to maintain the correct order of operations on the calculator.
📘 In complex equations involving multiple variables, replace non-variable terms with 'X' to simplify input on the calculator.
🔔 Stay tuned for the second part of the tutorial by subscribing, turning on notifications, and engaging with the content for more tips.

Q & A

What is the first step to prepare a calculator for solving mathematical problems as described in the tutorial?
-The first step is to clear the calculator to delete every unnecessary file. This can be done by pressing the 'shift' button, then selecting the 'clear' option, and either choosing 'setup to memory' or 'reset all to set', or by pressing the 'AC' button for a reset.
How do you input a fraction into the calculator according to the tutorial?
-To input a fraction, you press the 'fraction' button, then input the numerator, press the 'forward' button to switch to the denominator, and input the denominator. After that, press the 'forward' button again to proceed with the rest of the equation.
What does the tutorial suggest to do when encountering an equation with variables in the form of a fraction?
-The tutorial suggests using the 'Alpha' key to input variables in place of fractions. For example, if the equation involves '2x/3', you would input '2', press 'forward', input '3', and then use 'Alpha' to represent 'x'.
How does the tutorial handle solving equations with two unknowns using the calculator?
-The tutorial instructs to use the 'mode' function, select option five for equations, and then choose option one for equations with two unknowns. The calculator will guide you through inputting the coefficients and constants of the variables X and Y.
What is the correct order of inputting equations with two unknowns in the calculator as per the tutorial?
-The equations should be inputted in the same order as they appear in the problem statement. For instance, if the first equation is '3x - 2 = 7' and the second is 'x + 2y = -3', they should be entered maintaining this order in the calculator.
How does the tutorial describe simplifying logarithmic expressions using the calculator?
-The tutorial suggests using the 'fraction' button for logarithmic expressions that involve fractions. It also emphasizes closing brackets before inputting values to avoid errors and using the 'log' function with the appropriate base or assuming base 10 when no base is specified.
What is the process for solving a quadratic equation using the calculator as outlined in the tutorial?
-The process involves going to the 'mode' function, selecting option five for equations, then choosing option three for a quadratic equation. Input the coefficients a, b, and c for the equation ax^2 + bx + c = 0, and the calculator will provide the roots x1 and x2.
How does the tutorial recommend finding the smaller value of x for a quadratic equation?
-The tutorial suggests that after solving the quadratic equation, the smaller value of x is represented by x2. It compares it to owing money, where owing more is worse, hence the smaller value should be chosen.
What is the approach to solving equations involving variables other than x in the calculator, as per the tutorial?
-The tutorial advises using x in place of other variables when solving equations in the calculator, as it is the initial or non-variable that the calculator recognizes. For example, replace 'y' with 'x' when solving the equation.
How does the tutorial explain finding the value of a variable in an equation involving fractions and multiple variables?
-The tutorial demonstrates solving such equations by using the 'fraction' button and replacing variables with 'x' to ensure the calculator can process the equation correctly. It also emphasizes the importance of inputting the equation in the correct format.

Outlines

00:00

📚 Mathematics Calculator Tricks

This paragraph introduces a tutorial focused on calculator tricks to enhance performance in mathematics exams, specifically using past questions from SSCE and JAMB exams. The speaker demonstrates how to clear the calculator, solve equations involving fractions and algebraic expressions, and emphasizes the importance of resetting the calculator to avoid errors. The tutorial covers solving linear equations with two unknowns, simplifying logarithmic expressions, and evaluating logarithmic functions without a specified base (defaulting to base 10).

05:01

🔍 Advanced Calculator Techniques for Equations and Logarithms

The second paragraph delves into more advanced calculator techniques, including solving equations with multiple variables and simplifying complex logarithmic expressions. The speaker illustrates how to use the calculator to solve for X in a quadratic equation, find values that satisfy a given equation, and simplify expressions involving square roots and logarithms. The emphasis is on the correct input order and the importance of understanding the calculator's functions to arrive at the correct solutions.

10:05

📢 Conclusion and Invitation to Future Tutorials

The final paragraph serves as a conclusion to the first part of the video tutorial, summarizing the content covered and encouraging viewers to subscribe and turn on notifications for the release of part two. The speaker also prompts viewers to like the video and leave comments, highlighting the importance of the calculator in solving general and further mathematics exam questions and reminding viewers that success in exams is not solely about intelligence but also about smart study strategies.

Mindmap

Keywords

💡Calculator Tricks

Calculator tricks refer to the efficient and often shortcut methods used to solve mathematical problems using a calculator. In the video, these tricks are essential for solving complex equations and simplifying calculations quickly. For example, the script mentions using the 'fraction' button to input fractions and the 'Alpha' key to solve for variables in equations.

💡SSCE Past Questions

SSCE stands for Senior Secondary School Certificate Examination, which is a common educational qualification in some African countries. In the context of the video, the presenter is using past questions from this examination to demonstrate how to apply calculator tricks in solving mathematics problems, thus preparing students for their exams.

💡AF900 Level Exam Paper

The AF900 Level Exam Paper refers to a specific set of examination materials, likely from a university or advanced educational institution. The video uses this as a source of mathematical problems to illustrate the application of calculator tricks, indicating the relevance of these skills beyond basic education.

💡MTH101

MTH101 typically refers to a course code for an introductory mathematics course at a university level. The video mentions this code, suggesting that the calculator tricks being discussed are applicable to a wide range of mathematical topics, including those encountered in a foundational university math course.

💡Clear Calculator

Clearing a calculator involves resetting it to its initial state, often to remove any stored data or unwanted calculations. In the script, the presenter instructs viewers to clear their calculators to ensure accurate and uncluttered results, which is a fundamental step before starting any new calculation.

💡Fraction

A fraction in mathematics is a numerical expression that represents part of a whole, expressed as one quantity divided by another. The video script describes how to input fractions into a calculator, which is crucial for solving equations involving fractional components, such as the equation '2/3 * (2x - 1) = 1/4 * (5x - 3)'.

💡Equation Solver

An equation solver is a function on a calculator that can find the values of variables that make an equation true. The video demonstrates using the calculator's equation solver to find 'x' and 'y' in systems of linear equations, showcasing the practical application of this feature in solving mathematical problems.

💡Quadratic Equation

A quadratic equation is a polynomial equation of degree two, typically in the form of ax^2 + bx + c = 0. The script mentions using the calculator's mode to solve quadratic equations, such as 'x^2 + 7x + 10 = 0', which is a common type of problem in algebra.

💡Logarithm

A logarithm is the inverse operation to exponentiation, used to solve equations involving powers and roots. The video script includes an example of simplifying a logarithmic expression, demonstrating the calculator's utility in handling logarithmic calculations.

💡Root

In mathematics, a root of an equation is a value that, when substituted into the equation, makes the equation true. The script refers to square roots and cube roots, illustrating how to use a calculator to find roots of numbers, such as in the expression '√72 + √32 - 3√18'.

💡Mode

In the context of a calculator, mode refers to the different operational states or functions that a calculator can perform. The video script mentions changing the calculator's mode to access specific functions, such as solving equations or simplifying expressions, which is necessary for tackling different types of mathematical problems.

💡Subscription Button

The subscription button is a feature on video platforms that allows users to subscribe to a channel to receive notifications about new content. The script encourages viewers to subscribe and turn on notifications to stay updated with the tutorial series, highlighting the interactive aspect of online learning.

Highlights

Clearing the calculator to prepare for calculations by pressing shift, clear, and then reset or AC button.

Solving for x in a fractional equation using the fraction button and solving for x with the first attraction at zero.

Using the equation mode to solve for two unknowns, X and Y, and ensuring the equations are in the correct order.

Simplifying logarithmic expressions by using the fraction button and correctly inputting the values.

Evaluating logarithmic expressions with different bases, and understanding the default base of 10.

Finding the value of X in an equation involving square roots by using the default button.

Solving a quadratic equation using the equation mode and identifying the smaller value of x.

Solving a fractional equation by substituting variables and using the correct calculator functions.

Calculating values in an equation with variables by using the correct order and operations.

Understanding the importance of resetting the calculator to avoid errors in calculations.

Using the calculator's equation mode to solve for two variables systematically.

The significance of inputting equations in the correct format for the calculator to solve.

The process of simplifying complex logarithmic expressions using the calculator.

Identifying the correct option for the solution of an equation based on the calculator's output.

The method to find the smaller root of a quadratic equation using the calculator.

The importance of using the correct variable substitution when solving equations on the calculator.

The strategy to solve for a variable in an equation involving multiplication and addition of variables.

Transcripts

Browse More Related Video

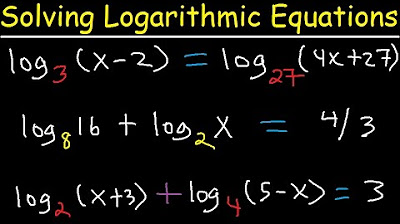
Solving Logarithmic Equations With Different Bases - Algebra 2 & Precalculus

ACT Calculator Strategies TI-84+

Calculator Techniques FOR BOARD EXAM - Evaluating Functions and Simplifying Expressions 10 EXAMPLES

SAT® Calculator Hacks: TI-84 Tips & Tricks

Business Calculus -- Math 1329 -- Section 4.2 -- Logarithmic Functions

Factorizing Using Casio 570MS Calculator