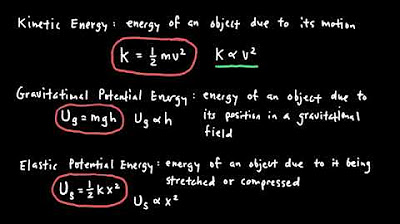College Physics 1: Lecture 30 - Conservation of Energy

Spahn's Science Lectures

3 Dec 202225:17

EducationalLearning

32 Likes 10 Comments

TLDRThis lecture from the College Physics 1 series focuses on the conservation of energy, a fundamental principle stating that energy in an isolated system remains constant. The instructor introduces the work-energy equation, explaining how work done on a system equates to changes in various forms of energy, including kinetic, gravitational potential, elastic potential, and thermal energy. The lecture outlines a problem-solving strategy for energy conservation problems, emphasizing the importance of writing out the full conservation equation and eliminating irrelevant terms. Two examples are worked through, illustrating the process of setting up and solving equations to determine minimum velocities and initial speeds in scenarios involving hills and aircraft landings on an aircraft carrier. The lecture aims to equip students with the tools to approach and solve conservation of energy problems methodically.

Takeaways

📚 The lecture focuses on the concept of conservation of energy, building upon previous discussions on work and different forms of energy.
🔧 The work-energy equation introduced states that work (W) is equal to the change in energy (ΔE), which includes kinetic, gravitational potential, elastic potential, and thermal energy.
🚫 For isolated systems, external forces do not add or remove energy, ensuring that the total energy of the system is conserved.
✍️ A problem-solving strategy is presented for conservation of energy problems, emphasizing the importance of writing out the full conservation equation and eliminating irrelevant terms.
🧩 The strategy involves writing out the full equation, eliminating unnecessary terms, identifying terms equal to zero, solving the simplified equation, and ensuring the answer makes sense and answers the question.
🚀 An example problem is solved involving a puck sliding on frictionless ice towards a hill, illustrating the process of determining if the puck has enough kinetic energy to reach the top.
🛫 Another example problem involves a jet landing on an aircraft carrier and being stopped by a spring, demonstrating how to calculate the initial velocity of the plane.
🔄 The concept of mass cancellation is highlighted, where the mass on both sides of the equation can often be eliminated, simplifying the problem-solving process.
🔢 The lecture provides step-by-step solutions to the example problems, emphasizing the importance of unit consistency and significant figures in the final answer.
❗ The instructor reminds students to ensure their final answers address the specific question asked, avoiding the common mistake of stopping prematurely.
👋 The lecture concludes with the instructor expressing gratitude to the students and viewers, and mentioning future plans to expand the lecture series.

Q & A

What is the fundamental principle of the conservation of energy?
-The fundamental principle of conservation of energy is that the total energy of an isolated system remains constant. It states that energy cannot be created or destroyed, only transformed from one form to another.
What does the work-energy equation represent?
-The work-energy equation represents the relationship that the work done on an object is equal to the change in its energy. In the context of the script, it is expressed as the change in the sum of all possible types of energy in a system.
What are the different forms of energy considered in the work-energy equation discussed in the lecture?
-The different forms of energy considered in the work-energy equation are kinetic energy, gravitational potential energy, elastic potential energy, and thermal energy.
How does the concept of an isolated system relate to the conservation of energy?
-An isolated system is one where no external forces add or remove energy. In such systems, the total energy remains constant, which is the essence of the conservation of energy principle.
What is the significance of writing out the full conservation equation when solving energy problems?
-Writing out the full conservation equation is important as it lays out all possible energy terms, reducing the chance of forgetting one. It helps in identifying which terms are relevant to the problem and which can be eliminated.
Why is it suggested to eliminate terms equal to zero early in the problem-solving process?
-Eliminating terms equal to zero simplifies the equation and reduces the complexity of the problem. It helps in focusing on the relevant energy terms and speeds up the problem-solving process.
What is the minimum initial velocity a puck needs to reach the top of a hill in the given example?
-The minimum initial velocity the puck needs to reach the top of the hill is 4.5 meters per second, as calculated using the conservation of energy principles.
Why is thermal energy not considered in the problem involving the puck on frictionless ice?
-Thermal energy is not considered because the ice is frictionless, meaning there is no friction to generate heat, and thus no conversion of energy into thermal form.
How does the spring constant affect the energy problem involving the jet landing on an aircraft carrier?
-The spring constant is a measure of the stiffness of the spring. In the problem, a larger spring constant indicates a stiffer spring, which is necessary to absorb more energy and stop the jet quickly.
What was the initial velocity of the plane as it landed on the aircraft carrier, according to the example provided?
-The initial velocity of the plane as it landed on the aircraft carrier was approximately 60 meters per second, as determined by the conservation of energy and the given spring properties.

Outlines

00:00

📚 Introduction to Conservation of Energy

The lecture begins by introducing the concept of energy conservation in the context of college physics. The instructor emphasizes the importance of understanding work and various forms of energy, such as kinetic, gravitational potential, elastic potential, and thermal energy. The work-energy equation is discussed, highlighting that in an isolated system, the total energy remains constant unless external forces are at play. The lecture aims to integrate these concepts to solve problems related to energy conservation, focusing on the work-energy equation in its expanded form, which accounts for all the different types of energy changes.

05:01

🔍 Problem-Solving Strategy for Energy Conservation

The instructor outlines a personal problem-solving strategy for energy conservation problems. The strategy involves writing out the full conservation equation, identifying and eliminating irrelevant energy terms based on the context of the problem, and simplifying the equation by considering terms that equal zero. The goal is to simplify the equation to a form that can be easily solved using algebra. The strategy is illustrated with an example of a puck sliding on frictionless ice towards a hill, where the challenge is to determine if the puck has enough kinetic energy to reach the top of the hill.

10:06

🚀 Calculating Minimum Initial Velocity

This section delves into the specifics of calculating the minimum initial velocity required for an object to reach the top of a hill. The problem involves a puck that slides on frictionless ice towards a one-meter high hill, starting at a speed of four meters per second. By applying the conservation of energy principle and the problem-solving strategy, the instructor demonstrates how to set up and simplify the equation to find the minimum initial velocity needed for the puck to just reach the top of the hill, which turns out to be 4.5 meters per second, indicating that the puck will not make it to the top at its given speed.

15:10

✈️ Solving for the Jet's Landing Speed

The fourth paragraph presents a problem involving a jet landing on an aircraft carrier and being brought to rest by a spring. The instructor applies the previously discussed problem-solving strategy to determine the jet's initial velocity upon landing. The problem involves considering the kinetic and elastic potential energies, with the spring's compression and the jet's mass being key factors. The solution process involves writing out the relevant equations, simplifying them by canceling out terms, and solving for the initial velocity, which is found to be approximately 60 meters per second.

20:10

🔧 Addressing the Absence of Friction and Gravitational Changes

In this paragraph, the instructor clarifies the conditions under which gravitational potential energy and frictional thermal energy are considered in the problem-solving process. It is emphasized that for the jet landing problem, changes in height are not relevant since the focus is on the plane's motion from the moment it lands until it comes to a stop. Similarly, friction is not considered due to the assumption of an ideal scenario without friction. This clarification helps to simplify the problem and maintain focus on the relevant energy changes.

25:11

🎉 Conclusion and Final Remarks

The lecture concludes with the instructor expressing gratitude to the students and viewers for their attention and engagement. They reflect on the journey through the physics course, highlighting the importance of the problem-solving strategies covered. The instructor also hints at future plans to expand the lectures to include more topics and specialized subjects. The closing remarks are a heartfelt thank you, encouraging students to continue learning and appreciating their efforts in understanding the complex concepts of physics.

Mindmap

Keywords

💡Conservation of Energy

Conservation of Energy is a fundamental principle in physics stating that the total energy of an isolated system remains constant over time. In the context of the video, it is the central theme around which the lecture is built. The script discusses how energy in various forms—kinetic, gravitational potential, elastic potential, and thermal—is conserved in an isolated system where no external forces add or remove energy. The principle is applied to solve problems involving different types of energy transformations.

💡Work-Energy Equation

The Work-Energy Equation is a fundamental concept in physics that relates the work done on an object to the change in its energy. In the video, the equation is introduced as W = ΔE, where W is work and ΔE is the change in energy. This equation is expanded to include various forms of energy, such as kinetic, gravitational potential, elastic potential, and thermal energy. It is a key tool used in solving problems related to energy conservation.

💡Kinetic Energy

Kinetic Energy is the energy possessed by an object due to its motion, defined as \( \frac{1}{2}mv^2 \), where m is the mass and v is the velocity of the object. In the video, kinetic energy is one of the energy types considered in the work-energy equation and is essential in problems where objects are moving, such as the example of the puck sliding on frictionless ice.

💡Gravitational Potential Energy

Gravitational Potential Energy is the energy an object possesses due to its position in a gravitational field, calculated as mgh, where m is mass, g is the acceleration due to gravity, and h is the height above a reference point. The script uses this concept in the context of objects changing height, such as a puck moving up a hill.

💡Elastic Potential Energy

Elastic Potential Energy is stored energy in an elastic object, such as a spring, when it is stretched or compressed. It is defined as \( \frac{1}{2}kx^2 \), where k is the spring constant and x is the displacement from the equilibrium position. In the video, elastic potential energy is discussed in the context of a spring stopping a jet on an aircraft carrier.

💡Thermal Energy

Thermal Energy, in the context of this video, refers to the energy associated with the random motion of particles in a substance due to heat. It is mentioned in the script as being relevant when friction is involved in a problem, such as converting kinetic energy into heat due to friction.

💡Isolated Systems

An Isolated System is a system that does not exchange matter or energy with its surroundings. In the video, the concept of isolated systems is crucial for the conservation of energy principle. The script emphasizes that for such systems, the total energy remains constant, which simplifies the analysis of energy transformations.

💡Problem-Solving Strategy

The Problem-Solving Strategy outlined in the video is a step-by-step approach to tackle physics problems involving conservation of energy. It includes writing out the full conservation equation, eliminating unnecessary terms, identifying terms equal to zero, and solving the simplified equation. This strategy is exemplified through various problems in the script to help students understand and apply the conservation of energy.

💡Frictionless

Frictionless, as used in the video, refers to a condition where there is no resistance to the motion of an object on a surface. This is important because it implies that there is no conversion of kinetic energy into thermal energy due to friction. The script uses the term in the context of a puck sliding on ice, indicating no thermal energy is considered in the problem.

💡Spring Constant

Spring Constant, denoted as k in the video, is a measure of the stiffness of a spring. It is the force needed to stretch or compress the spring by one unit of displacement. The script mentions a spring constant of 60,000 Newtons per meter when discussing how a spring is used to stop a jet on an aircraft carrier, indicating a very strong spring.

Highlights

Introduction to lecture 30 on conservation of energy and its foundational concepts.

Explanation of the work-energy equation and its relation to the conservation of energy.

The significance of work being equal to the change in energy for isolated systems.

Detailed breakdown of the work-energy equation including kinetic, gravitational, elastic potential, and thermal energy.

Clarification on the use of the work-energy equation for isolated systems where no external work is done.

The importance of writing out the full conservation equation for problem-solving.

Strategy for solving conservation of energy problems by eliminating irrelevant energy terms.

Guidance on identifying terms equal to zero and simplifying the equation accordingly.

Emphasis on ensuring the final answer makes sense with respect to the problem context.

Illustration of the problem-solving strategy with an example involving a puck on frictionless ice.

Conceptualization of whether the puck has enough kinetic energy to reach the top of a hill.

Calculation of the minimum initial velocity required for the puck to reach the top of the hill.

Determination of the puck's inability to reach the top of the hill based on its initial velocity.

Application of the problem-solving strategy to a scenario involving a jet landing on an aircraft carrier.

Use of a spring's properties to determine the initial velocity of a landing plane.

Final calculation revealing the plane's landing speed and the conclusion of the lecture.

Transcripts

Browse More Related Video

College Physics 1: Lecture 28 - Work and Energy

College Physics 1: Lecture 29 - Forms of Energy

2022 Live Review 4 | AP Physics 1 | Understanding Work and Energy

AP Physics 1 Energy Review

AP Physics 1: Work, Energy and Power Review

AP Physics 1 review of Energy and Work | Physics | Khan Academy

Related Tags

Physics Education Energy Conservation Work-Energy Equation Problem Solving Kinetic Energy Potential Energy Elastic Potential Thermal Energy College Physics Lecture Series