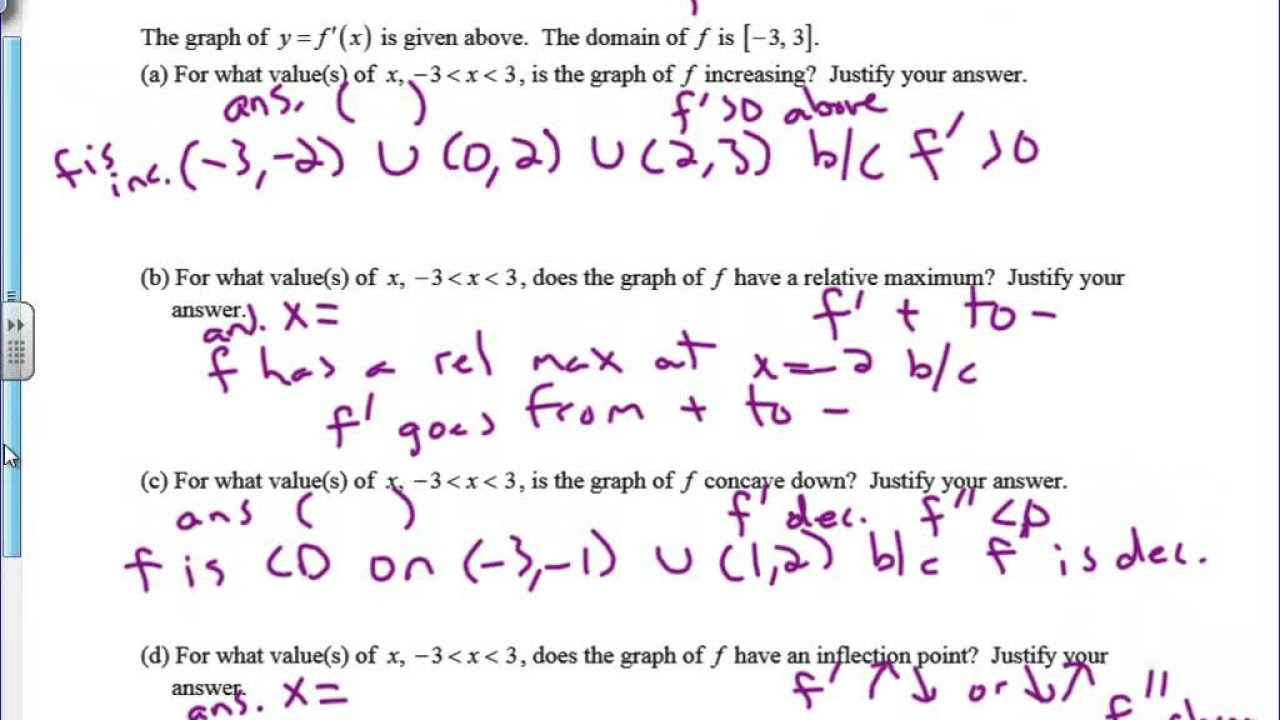
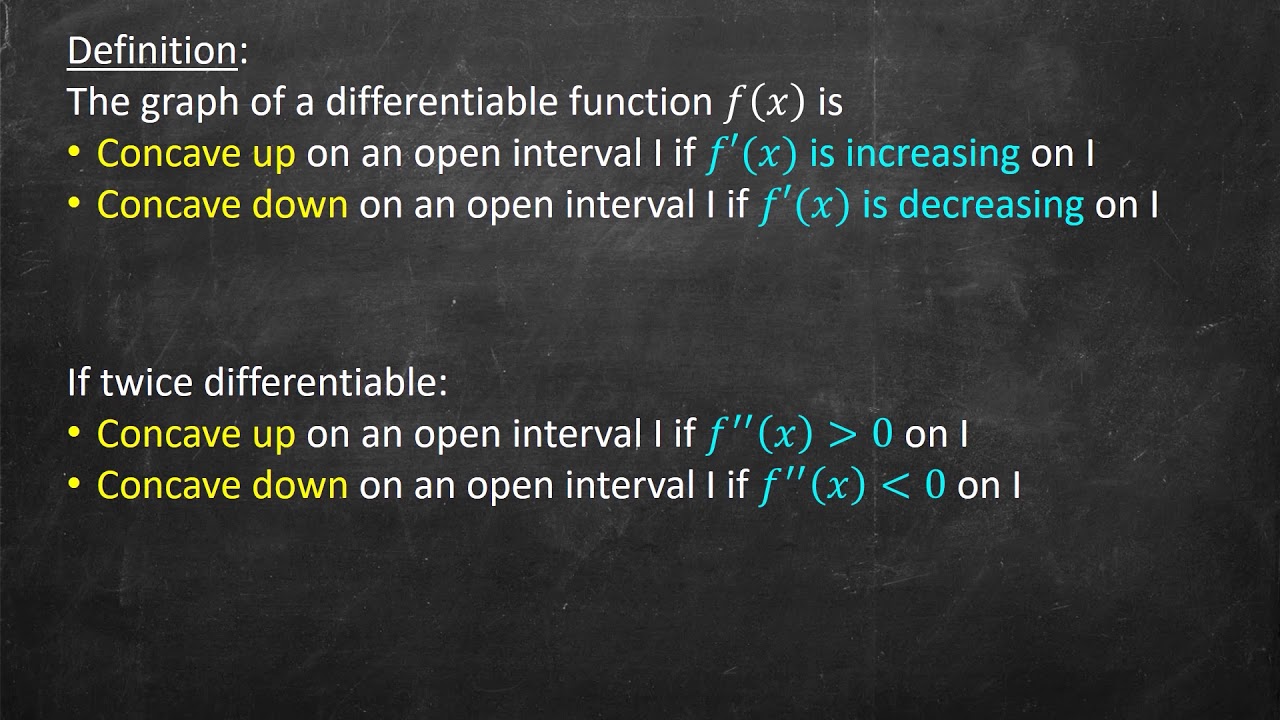
Connecting f, f', and f'' graphically | AP Calculus AB | Khan Academy
TLDRIn the video, the instructor presents a challenge to identify three graphs representing a function f, its first derivative f', and its second derivative f''. The analysis begins by observing the slope changes in the orange graph, which suggests a positive slope that decreases to zero and then becomes negative. This pattern leads to the conclusion that the magenta graph, showing an intersect with the x-axis and a positive trend, is likely the derivative of the orange graph. The blue graph's trend is opposite, eliminating it as the derivative of the orange graph. The instructor then sketches a possible derivative for the blue graph, which matches the brown graph, indicating the blue graph as the original function f and the brown graph as its first derivative f'. Finally, the instructor suggests that the derivative of the magenta graph would resemble an upward opening U, which is not present among the graphs, confirming the initial hypothesis. The conclusion identifies the middle graph as f, the left graph as f', and the right graph as the second derivative f''.
Takeaways
- 📈 The video script discusses a method to identify which of three given graphs represent a function 'f', its first derivative 'f prime', and its second derivative 'f prime prime'.
- 🔍 The instructor suggests pausing the video to attempt the problem before continuing, encouraging active learning.
- 🟠 The first graph (orange) is analyzed for its slope, which starts positive, decreases to zero, and then becomes increasingly negative.
- 📊 The instructor sketches a possible derivative graph based on the slope changes of the orange graph, indicating a trend from positive to negative crossing zero.
- 🔵 The blue graph is ruled out as the derivative of the orange graph due to its opposite trend from negative to positive.
- 💗 The magenta graph is considered a good candidate for the derivative of the orange graph, as it has the right trend and intersects the x-axis at the correct point.
- 🟢 The instructor explains that derivatives can have more extreme points than the original function, which might cause confusion but is possible if the entire function isn't visible.
- 🟢 The second graph (blue) is analyzed, with its slope starting negative, decreasing to zero, and then becoming increasingly positive.
- 🏾 The brown graph is identified as the likely derivative of the blue graph, matching the expected trend of the derivative.
- 🔄 The instructor suggests sketching the derivative of the brown graph to confirm its identity as the second derivative, which would resemble an upward opening U shape.
- 📚 The final conclusion is that the middle graph is 'f', the left graph is 'f prime', and the right graph is the second derivative 'f prime prime'.
Q & A
What is the objective of the video script?
-The objective of the video script is to help viewers determine which of the three given graphs represents the function f, its first derivative, and its second derivative.
What is the first step suggested by the instructor to approach the problem?
-The first step suggested by the instructor is to pause the video and attempt to solve the problem independently before proceeding to work through it together.
How does the instructor begin to analyze the graphs?
-The instructor begins by sketching what the derivatives of each graph might look like, based on the observed slopes and trends of the original graphs.
What observation about the orange graph leads the instructor to conclude its derivative's behavior?
-The instructor notes that the orange graph has a positive slope that decreases until it reaches zero, then becomes increasingly negative, which suggests that its derivative will start positive, cross zero, and become increasingly negative.
Why does the instructor rule out the blue graph as the derivative of the orange graph?
-The instructor rules out the blue graph because its trend is opposite to that of the orange graph; it goes from negative to positive, whereas the orange graph's derivative should go from positive to negative.
What characteristic of the magenta graph makes the instructor consider it as a potential derivative of the orange graph?
-The magenta graph has a trend that matches the expected behavior of the orange graph's derivative, including intersecting the x-axis at the correct point and being positive over the observed interval.
Why might the instructor's initial assumption about the magenta graph being the derivative be questioned?
-The initial assumption might be questioned because it's unusual for a derivative to have more extreme points (minima and maxima) than the original function, although the instructor suggests this could be due to not seeing the entire original function.
What does the instructor suggest about the relationship between the magenta and orange graphs?
-The instructor suggests that the magenta graph is a good candidate for being the derivative of the orange graph, indicating that the orange graph could be the function f and the magenta graph its first derivative, f prime.
How does the instructor analyze the blue graph's derivative?
-The instructor analyzes the blue graph's derivative by noting that its slope starts negative, becomes less negative until it reaches zero, and then becomes increasingly positive, suggesting a behavior that matches the brown graph.
What conclusion does the instructor reach about the brown graph in relation to the blue graph?
-The instructor concludes that the brown graph likely represents the derivative of the blue graph, with the blue graph being the function f, the brown graph its first derivative, f prime, and the second derivative being the one not depicted.
What additional step does the instructor recommend to confirm the assignments of the graphs?
-The instructor recommends sketching out the derivative of the assigned second derivative graph (the brown graph) as an additional step to confirm the assignments, ensuring that it matches the expected behavior of an upward opening U shape.
Outlines
📈 Identifying Functions and Their Derivatives
The instructor introduces a problem involving three graphs representing a function f, its first derivative f', and its second derivative f''. The task is to correctly match each graph to its corresponding function. The instructor suggests pausing the video to attempt the problem independently before proceeding. The approach taken is to sketch the derivatives based on the given graphs' slopes. The first graph in orange shows a positive slope that decreases to zero and then becomes negative, suggesting a derivative that crosses zero and becomes negative. The instructor rules out the blue graph as the derivative of the orange graph due to the opposite trend. The magenta graph is considered a good candidate for the derivative of the orange graph, aligning with the expected trend and x-axis intersection.
🔍 Analyzing the Derivatives and Matching the Second Derivative
Continuing the analysis, the instructor examines the blue graph's slope, which goes from negative to positive, and hypothesizes its derivative, which would intersect the x-axis where the slope is zero and change from negative to positive. The brown graph is identified as a likely match for the blue graph's derivative based on the sketched trend. The instructor then considers the magenta graph's derivative, which should resemble an upward opening U shape, indicating that the second derivative is not depicted in the provided graphs. Concluding the analysis, the instructor assigns the middle graph as f, the left graph as f', and the right graph as the second derivative, suggesting that the magenta graph's derivative is not shown.
Mindmap
Keywords
💡Graphs
💡Function 'f'
💡Derivative
💡First Derivative
💡Second Derivative
💡Slope
💡Tangent Line
💡Intersection with x-axis
💡Positive and Negative
💡Minima and Maxima
💡Upward Opening U
Highlights
The task involves identifying three functions where one is the original function f, another is its first derivative, and the third is its second derivative.
The approach is to sketch the derivatives based on the given graphs to deduce which function is which.
Orange graph's slope starts positive, decreases to zero, and then becomes increasingly negative.
The first derivative of the orange graph should start positive, cross zero, and become increasingly negative.
Blue graph's trend is opposite to the orange graph, ruling it out as the derivative.
Magenta graph has the right trend and intersects the x-axis at the correct point, suggesting it could be the first derivative.
The possibility of the derivative having more extreme points than the original function is discussed.
The magenta graph is identified as a good candidate for being the derivative of the orange graph.
Blue graph's slope changes from negative to less negative until it reaches zero, suggesting its derivative would cross the x-axis and become positive.
The brown graph resembles the expected derivative of the blue graph, indicating it could be the first derivative.
The second derivative, f prime prime, is expected to show changes in the rate at which the first derivative is increasing or decreasing.
The brown graph is confirmed as the first derivative of the blue graph, leading to the identification of the original function f.
The derivative of the magenta graph is sketched to resemble an upward opening U, which is not present in the provided graphs.
The absence of the upward opening U shape in the provided graphs confirms that the magenta graph's derivative is not depicted.
The final identification is the middle graph as f, the left graph as f prime, and the right graph as the second derivative.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: