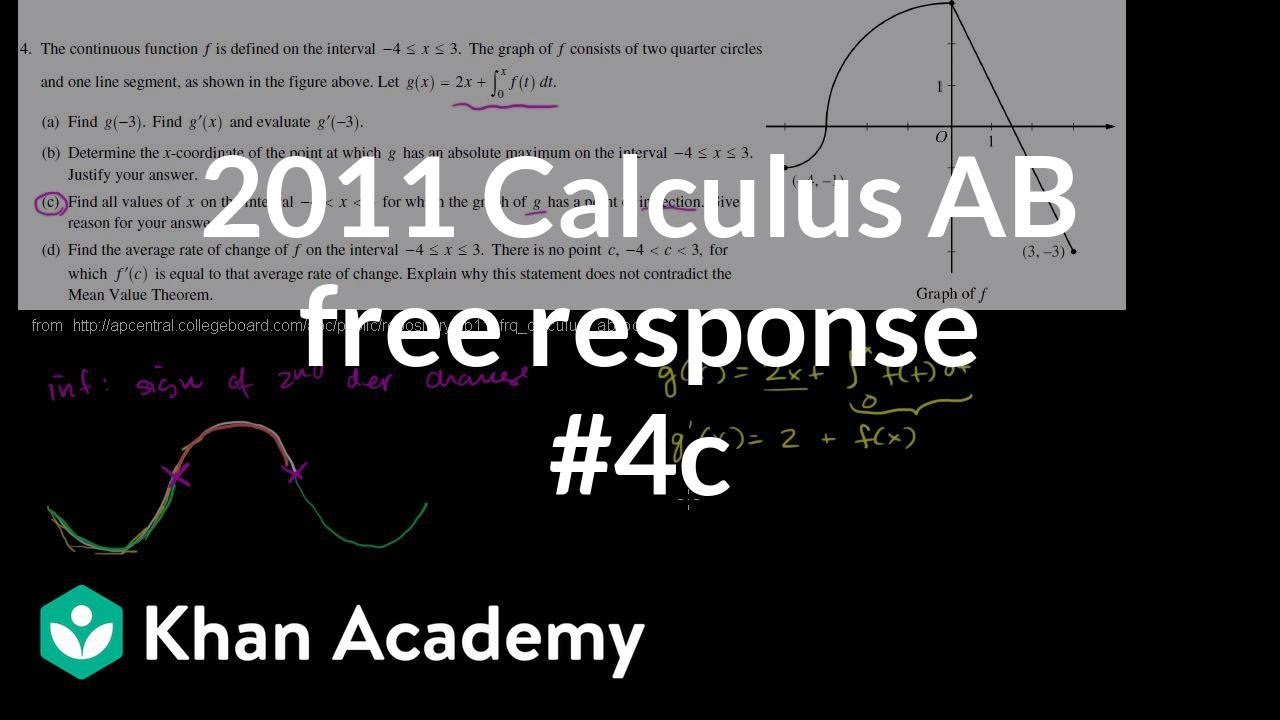
AP CALCULUS AB 2022 Exam Full Solution FRQ#3(a,b)
TLDRThe video script discusses a calculus problem involving a differentiable function f, with an initial condition f(4) = 3, and its derivative f' represented by a graph consisting of a semicircle and two line segments. The focus is on calculating the values of f at x=0 and x=5 by integrating the derivative function from x=4 to the desired x values. The process involves understanding the concept of displacement and the direction of integration. Additionally, the video addresses finding points of inflection on the graph of f between 0 and 7. This is done by examining the slope of f', which corresponds to the second derivative f'', and looking for changes in direction that indicate a change in concavity. The analysis concludes with the identification of two points of inflection at x=2 and x=6, based on the observed changes in the slope of the derivative function.
Takeaways
- 📈 The script discusses a free response question involving a derivative function, f prime, which is given as a graph consisting of a semicircle and two line segments.
- 📌 The initial condition for the function f is that f(4) equals 3, which is a fixed starting point for calculating the function's values at other points.
- 🔍 To find the value of f at a given x, one must integrate from x=4 to that x, considering the displacement represented by the area under the curve of f prime.
- ⛓ When integrating from right to left, the displacement is the opposite, which is important for calculating the function's values.
- 🔢 For calculating f(0), the area under the curve from x=4 to x=0, which is a semicircle with radius 2, equals 2π. However, since it's under the x-axis, it contributes a positive displacement.
- 📐 The value of f(0) is calculated to be 3 plus 2π, which results from the positive displacement from the semicircle's area.
- 📊 For f(5), the area to be considered is from x=4 to x=5, and it is calculated to be one-half, leading to f(5) being 3 plus 0.5 or 7/2.
- 🔍 Part B of the question asks for the x-coordinates of all points of inflection on the graph of f from 0 to 7.
- 📉 The slope of f prime represents the second derivative, f double prime, which indicates the concavity of the function.
- 🔄 A change in the direction of the slope of f prime indicates a change in concavity, which corresponds to an inflection point.
- 📍 Inflection points occur where the second derivative (slope of f prime) changes from positive to negative or vice versa, which are found at x=2 and x=6 in this case.
Q & A
What is the initial condition given for the function f in the script?
-The initial condition given for the function f is that f(4) equals 3.
What is the significance of the area under the curve of f'?
-The area under the curve of f' (the derivative of f) represents the displacement of the function f over the given interval.
How is the value of f(0) calculated in the script?
-The value of f(0) is calculated by starting at the fixed point (x=4, y=3) and integrating the area under the f' graph from x=4 to x=0. The area is a semicircle with radius 2, which results in a displacement of 2π, leading to f(0) being 3 + 2π.
How is the value of f(5) determined from the script?
-The value of f(5) is found by starting at the initial condition (y=3) and adding the displacement from x=4 to x=5, which is the area above the curve of f' from x=4 to x=5. This area is calculated to be 0.5, so f(5) equals 3 + 0.5, or 3.5.
What is the role of the second derivative (f'') in determining points of inflection?
-The second derivative (f'') indicates the concavity of the function. A change in the sign of the second derivative, from positive to negative or vice versa, indicates a point of inflection.
At what x-coordinates does the script identify points of inflection for the function f?
-The script identifies points of inflection at x=2 and x=6, where there are changes in the direction of the slope of the f' graph.
What is the geometric interpretation of the second derivative being zero?
-When the second derivative is zero, it means that the function has a horizontal tangent at that point, indicating neither positive nor negative concavity.
How does the direction of integration affect the calculated displacement in the script?
-The direction of integration affects the sign of the calculated displacement. Integrating from right to left results in the opposite displacement, which is considered when calculating the function values.
What is the significance of the semicircle in the graph of f'?
-The semicircle in the graph of f' represents a portion of the derivative function's graph, and its area contributes to the displacement when calculating the function values of f at different x-coordinates.
How does the script use the concept of negative displacement in its calculations?
-The script accounts for negative displacement by considering the direction of integration and the position of the area under the curve relative to the x-axis. When the area is below the x-axis, the displacement is taken as positive because the integration direction is reversed.
What is the relationship between the slope of f' and the concavity of f?
-The slope of f' (the first derivative) is equal to the second derivative (f'') of the original function f, which indicates the concavity of f. A positive second derivative corresponds to positive concavity, and a negative second derivative corresponds to negative concavity.
Why is the change in the direction of the slope of f' significant in the context of the script?
-The change in the direction of the slope of f' is significant because it indicates a change in the concavity of the function f. This change is used to identify points of inflection on the graph of f.
Outlines
📈 Calculating Function Values Using Derivative Graph
This paragraph discusses the process of finding function values using a given derivative graph. The function f is differentiable with an initial condition f(4) = 3. The graph of f' (the derivative of f) is composed of a semicircle and two line segments. The area under the curve represents displacement, and to find f(x), one must start from a fixed point, x = 4, y = 3. The integration from right to left results in the opposite displacement. The calculation of f(0) involves finding the area under the f' graph from x = 4 to x = 0, which is a semicircle with radius 2, resulting in an area of 2π. Since the integration is in the opposite direction, the displacement is positive, leading to f(0) = 3 + 2π. Similarly, f(5) is calculated by starting at y = 3 and adding the displacement from x = 4 to x = 5, resulting in an area of 0.5 and thus f(5) = 3 + 0.5 = 3.5 or 7/2.
📌 Identifying Points of Inflection from Derivative Graph
The second paragraph focuses on identifying points of inflection on the graph of the function f from x = 0 to x = 7. An inflection point is where the concavity of the function changes, which can be determined by examining the slope of f' (the second derivative, f''). The change in direction of the slope of f' indicates a change in concavity. The paragraph identifies two points of inflection: at x = 2, where the slope changes from negative to positive (indicating a change from negative to positive concavity), and at x = 6, where the slope changes from positive to negative (indicating a change from positive to negative concavity). These points are justified by the change in the second derivative's sign, which corresponds to a change in concavity.
Mindmap
Keywords
💡Derivative function
💡Initial condition
💡Displacement
💡Integration
💡Semicircle
💡Inflection point
💡Concavity
💡Second derivative
💡Fixed point
💡Positive and negative displacement
💡Graph
Highlights
The transcript discusses a non-graphing calculator problem involving a differentiable function f with an initial condition f(4) = 3.
The derivative function f' is represented graphically, consisting of a semicircle and two line segments on the interval 0 to 7.
The area under the curve of f' represents the displacement, which is used to find values of f(x) starting from a fixed point.
Integration from right to left results in the opposite displacement, affecting the calculation of f(x) values.
The value of f(0) is calculated by starting at y = 3 and integrating the area under the f' graph from x = 4 to x = 0, resulting in f(0) = 3 + 2π.
For calculating f(5), the starting point is y = 3, and the area under the curve from x = 4 to x = 5 is integrated, yielding f(5) = 3 + 0.5.
Points of inflection on the graph of f are identified by changes in the direction of the slope of f', indicating changes in concavity.
The slope of f' (f'') is used to determine the concavity and potential inflection points on the graph of f.
An inflection point occurs where the second derivative changes from positive to negative or vice versa.
The transcript identifies two points of inflection at x = 2 and x = 6 based on the change in the direction of the slope of f'.
The area under the semicircle from x = 4 to x = 0 is calculated to be 2π, contributing to the displacement calculation for f(0).
The calculation of f(0) involves a positive displacement despite the area being under the x-axis due to the direction of integration.
The area above the curve from x = 4 to x = 5 is calculated to be 0.5, which is used to determine f(5).
The concept of negative concavity and positive concavity is discussed in relation to the slope of f' and the second derivative f''.
The transcript provides a method for calculating function values and identifying inflection points using integration and graphical analysis.
The importance of the initial condition and the direction of integration in calculating function values is emphasized.
The transcript demonstrates the application of calculus concepts to solve a complex problem involving derivatives and integration.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: