Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems
TLDRThis educational video offers a comprehensive guide on proving trigonometric identities, essential for students and learners. It begins by explaining reciprocal identities, moving on to quotient identities, and culminates with the Pythagorean identities. The presenter demonstrates the proofs with step-by-step examples, simplifying complex expressions into more familiar trigonometric functions. The video aims to clarify and solidify understanding of these mathematical concepts, encouraging viewers to engage with the material actively by asking questions in the comments section.
Takeaways
- 📚 The video is a tutorial on proving trigonometric identities, which are fundamental in understanding the relationships between trigonometric functions.
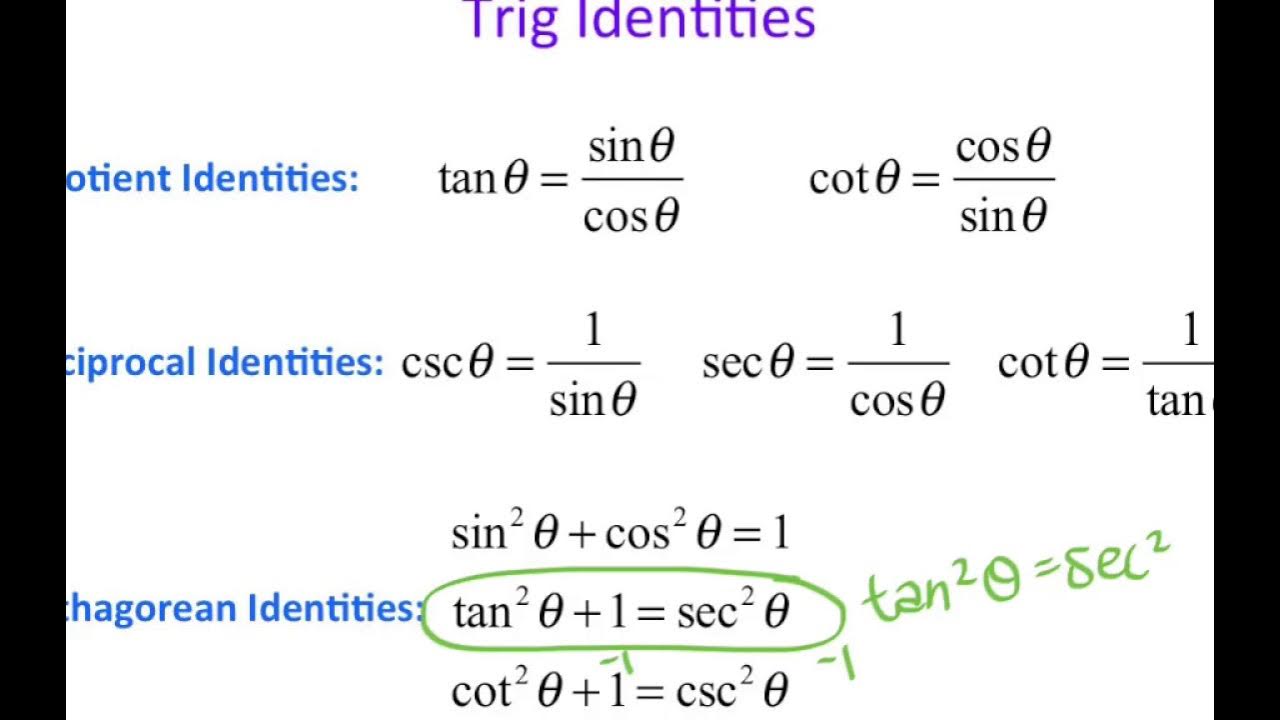
- 🔄 The reciprocal identities are introduced, showing that secant (sec) and cosecant (csc) are the reciprocals of cosine (cos) and sine (sin) respectively.
- 🔄 The video also covers the reciprocal relationship of tangent (tan) and cotangent (cot), where cot is the reciprocal of tan and vice versa.
- 📈 The quotient identities are explained, which relate tan and cot to sin and cos, with tan being sin over cos and cot being cos over sin.
- 🧩 The Pythagorean identities are highlighted, stating that sin squared plus cos squared equals one, and similar relationships for tan and cot with sec and csc.
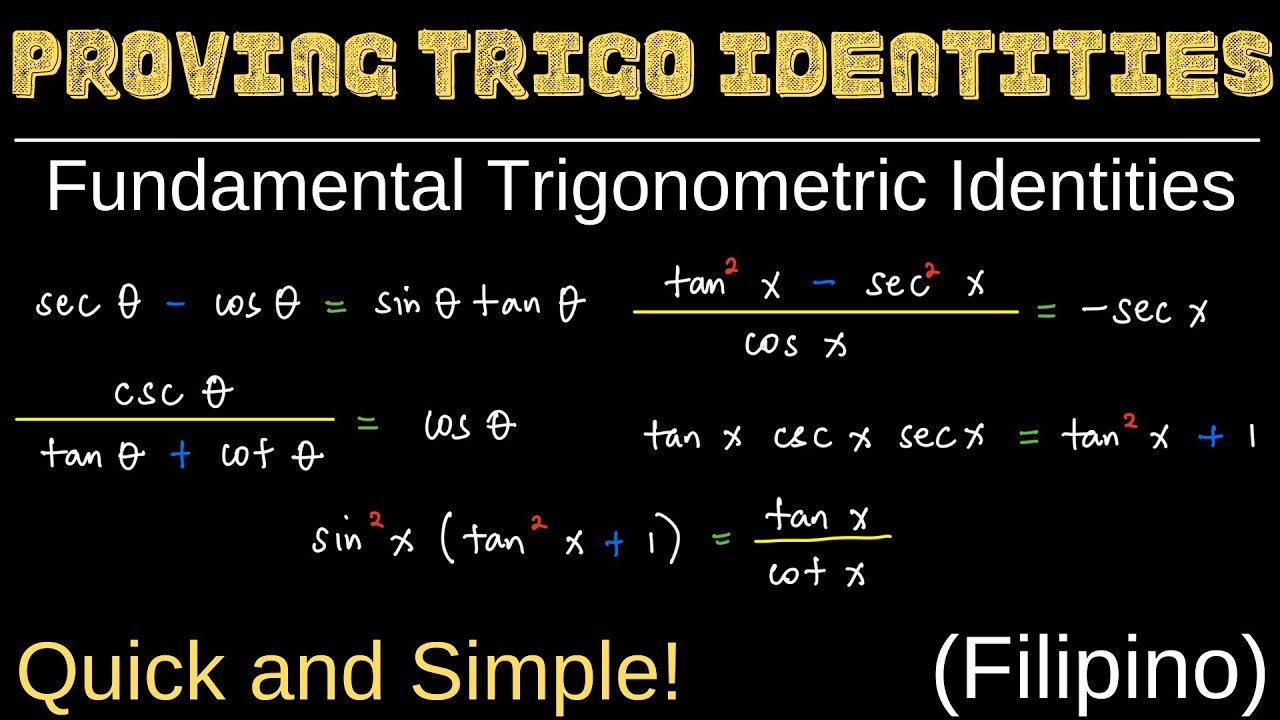
- 📝 An example proof is provided to show that sine times secant equals tangent, demonstrating the process of simplifying and using reciprocal identities.
- 📝 A second example is given to prove that one over sine times cotangent equals one over cosine, illustrating the use of quotient identities.
- 📝 The third example demonstrates proving a more complex identity involving secant squared, cosine squared, tangent squared, and cotangent squared.
- 🔍 The process involves simplifying the left side of the equation to match the right side, using the identities introduced earlier in the video.
- 🤓 The video concludes with an invitation for viewers to ask questions or seek clarifications in the comments section, emphasizing viewer engagement.
- 👋 The presenter signs off, summarizing the content and encouraging further interaction, showing a friendly and approachable demeanor.
Q & A
What is the main topic of the video?
-The main topic of the video is proving trigonometric identities.
What are reciprocal identities in trigonometry?
-Reciprocal identities in trigonometry are the relationships where the reciprocal of one trigonometric function is equal to another function. For example, cosecant(theta) is the reciprocal of sine(theta), and secant(theta) is the reciprocal of cosine(theta).
What is the relationship between secant and cosine according to the video?
-According to the video, secant(theta) is equal to 1 divided by cosine(theta).
What are the quotient identities mentioned in the video?
-The quotient identities mentioned in the video are: tangent(theta) equals sine(theta) over cosine(theta), and cotangent(theta) equals cosine(theta) over sine(theta).
What is the Pythagorean identity for sine and cosine?
-The Pythagorean identity for sine and cosine is that sine squared(theta) plus cosine squared(theta) equals 1.
How does the video demonstrate the proof that sine theta times secant theta equals tangent theta?
-The video demonstrates the proof by converting secant theta into its reciprocal form, which is 1 over cosine(theta), and then multiplying it by sine(theta), resulting in sine(theta) over cosine(theta), which is tangent(theta).
What is the example given in the video to prove that one over sine theta times cotangent theta equals one over cosine theta?
-The video uses the reciprocal identities to express cotangent(theta) as cosine(theta) over sine(theta) and then multiplies it by one over sine(theta), which simplifies to cosine(theta) over sine(theta), and equates it to one over cosine(theta).
What is the identity involving secant squared theta, cosine squared theta, tangent squared theta, and cotangent squared theta that the video discusses?
-The identity discussed in the video is secant squared(theta) minus cosine squared(theta) equals tangent squared(theta) minus cotangent squared(theta).
How does the video simplify the expression secant squared theta minus cotangent squared theta?
-The video simplifies the expression by using the identity secant squared(theta) plus one equals cosecant squared(theta), then substituting and simplifying to get tangent squared(theta) minus cotangent squared(theta).
What does the video suggest for those who have questions or need clarifications on trigonometric identities?
-The video suggests that viewers who have questions or need clarifications should leave them in the comment section below.
Outlines
📚 Introduction to Proving Trigonometric Identities
This paragraph introduces the video's focus on proving trigonometric identities. It begins with a brief mention of the importance of understanding fundamental trigonometric identities and then proceeds to outline the types of identities that will be covered, including reciprocal, quotient, and Pythagorean identities. The speaker emphasizes the need to simplify complex expressions by converting them into simpler forms involving sine and cosine functions, setting the stage for the examples that will follow.
🔍 Detailed Explanation of Reciprocal and Quotient Identities
In this paragraph, the speaker delves into the specifics of reciprocal and quotient trigonometric identities. Reciprocal identities such as secant and cosecant are expressed in terms of sine and cosine, and quotient identities like tangent and cotangent are also defined. The speaker provides a step-by-step approach to simplifying expressions using these identities, illustrating how to transform complex trigonometric functions into more manageable forms. This section is crucial for understanding the foundational concepts that will be applied in the subsequent examples.
📈 Applying Pythagorean Identities and Example Proofs
The final paragraph of the script moves on to the application of Pythagorean identities and provides several example proofs. The speaker demonstrates how to use these identities to simplify expressions and prove relationships between trigonometric functions. The examples include proving that the product of sine and secant equals tangent, showing that the product of the reciprocals of sine and cotangent equals the reciprocal of cosine, and proving a more complex identity involving secant squared, cosine squared, tangent squared, and cotangent squared. Each example is worked through methodically, highlighting the process of simplifying and combining terms to reach the conclusion.
Mindmap
Keywords
💡Trigonometric Identities
💡Reciprocal Identities
💡Quotient Identities
💡Pythagorean Identities
💡Sine Function
💡Cosine Function
💡Tangent Function
💡Cosecant Function
💡Secant Function
💡Cotangent Function
💡Proof
Highlights
Introduction to proving trigonometric identities and reviewing fundamental identities.
Explanation of reciprocal identities: secant and cosecant in terms of sine and cosine.
Secant theta is equal to 1 over cosine theta.
Cotangent theta is equivalent to 1 over tangent theta.
Introduction to quotient identities: tangent and cotangent in terms of sine and cosine.
Pythagorean identities: sine squared plus cosine squared equals one.
Derivation of sine squared equals 1 minus cosine squared theta.
Tangent squared theta plus 1 equals secant squared theta.
1 plus cotangent squared theta equals cosecant squared theta.
Proof example: Sine theta times secant theta equals tangent theta.
Simplification of trigonometric expressions using reciprocal identities.
Proof example: 1 over sine theta times cotangent theta equals 1 over cosine theta.
Conversion of trigonometric functions into sine and cosine for simplification.
Proof example: Secant squared theta minus cosine squared theta equals tangent squared theta minus cotangent squared theta.
Use of Pythagorean identities to simplify trigonometric expressions.
Combining like terms to complete the proof of trigonometric identities.
Conclusion of the video with a summary of the trigonometric identities covered.
Invitation for viewers to ask questions or seek clarifications in the comment section.
Transcripts
Browse More Related Video
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Part 2

Introduction to Using Trigonometric Identities (Precalculus - Trigonometry 23)

How to Prove Trigonometric Identities (Precalculus - Trigonometry 24)

Simplifying Trigonometric Expressions

Ultimate trig identity problem study guide!
Precalc 5.1 Using Fundamental Trig Identities
5.0 / 5 (0 votes)
Thanks for rating: