Ultimate trig identity problem study guide!
TLDRThe video transcript is an engaging walkthrough of 24 different trigonometric identity problems. The presenter guides viewers through the process of solving these problems, emphasizing the importance of practice and understanding of trigonometric concepts. The session begins with simplifying expressions using trigonometric identities, such as converting cotangent to cosine over sine, and applying the Pythagorean identity. The presenter also covers more complex topics like the triple angle identity for cosine and the reduction formulas for sine and cosine powers. Throughout the video, the use of special right triangles, the unit circle, and the angle sum identities for sine and cosine are highlighted. The script is rich with step-by-step solutions, making it an excellent educational resource for those studying trigonometry, pre-calculus, or calculus. The presenter encourages viewers to practice the problems on their own and to apply these identities in further mathematical studies.
Takeaways
- 📚 Start with the basics: When dealing with trigonometric identities, it's crucial to begin with fundamental trigonometric functions like sine and cosine and use them to express other functions like tangent and cotangent.
- 🔢 Pythagorean identity: A key concept used throughout is the Pythagorean identity, which states that sine squared X plus cosine squared X equals one (sin²X + cos²X = 1).
- 📐 Unit circle: Understanding the unit circle and its relation to sine and cosine values is fundamental for visualizing and solving trigonometric problems.
- 🔁 Angle sum identities: The video covers the use of angle sum identities for sine and cosine, which are essential for combining terms involving different angles.
- 📉 Power reduction: Techniques for reducing higher powers of sine and cosine to their first power are discussed, which is particularly useful for integration in calculus.
- 🤔 Practice makes perfect: The video emphasizes the importance of practice in mastering the manipulation of trigonometric identities and fractions.
- 📝 Special right triangles: Knowledge of special right triangles, such as the 45-45-90 triangle, is important for understanding tangent values at specific angles.
- 🔀 Double and triple angle identities: The video demonstrates the application of double and triple angle identities for cosine and tangent, which are useful for simplifying expressions.
- ➗ Complex fractions: Strategies for dealing with complex fractions, such as multiplying by the denominator to simplify, are discussed.
- 🧮 Product-to-sum and sum-to-product: The video covers the conversion between product-to-sum and sum-to-product identities, which are useful for simplifying trigonometric expressions.
- 📌 Memorization tips: While not all identities need to be memorized, the video suggests memorizing key ones and using the board or notes for reference during problem-solving.
Q & A
What is the first trigonometric identity problem discussed in the video?
-The first problem involves simplifying the expression sine X plus cotangent X times cosine X. The video suggests initially rewriting cotangent X as cosine X over sine X and then combining fractions by multiplying the first fraction's top and bottom by sine X.
What is the Pythagorean identity mentioned in the video?
-The Pythagorean identity mentioned is sine Square X plus cosine Square X equals one. This identity is used to simplify the expressions throughout the video.
How is the triple angle identity for cosine derived in the video?
-The triple angle identity for cosine is derived by first expressing cosine of 3x as cosine of (X + 2X) and then applying the angle sum identity for cosine. The double angle identities for sine and cosine are then used to further simplify the expression.
What is the strategy for simplifying expressions involving secant and cosecant as shown in the video?
-The strategy is to express secant and cosecant in terms of sine and cosine. Secant is written as 1 over cosine, and cosecant as 1 over sine. Then, complex fractions are simplified by multiplying the numerator and denominator by the appropriate trigonometric function to create a common denominator.
How does the video approach the problem of adding tangent and cotangent functions?
-The video approaches this by first converting tangent and cotangent to sine and cosine functions, then combining them by finding a common denominator, which often involves multiplying by the missing trigonometric function from the denominator.
What is the purpose of using the unit circle to explain the Pythagorean identity?
-The unit circle is used to provide a geometric interpretation of the Pythagorean identity. By placing a point on the unit circle corresponding to an angle, the x-coordinate corresponds to the cosine value, and the y-coordinate corresponds to the sine value. The Pythagorean theorem then confirms that cosine squared theta plus sine squared theta equals one.
How does the video handle the problem of simplifying an expression with both sine and cosine raised to the fourth power?
-The video handles this by first expressing sine to the fourth power as sine squared X squared and then using the identity sine squared X plus cosine squared X equals one to simplify the expression. For cosine to the fourth power, the video uses the double angle identity for cosine.
What is the key to solving the problem involving the sum of secant X and cosecant X?
-The key is to combine the fractions by finding a common denominator, which involves multiplying the numerator and denominator of each fraction by the respective missing trigonometric function. After combining, the resulting expression can be simplified using the Pythagorean identity.
How does the video use the product-to-sum identities in the problem-solving process?
-The video uses the product-to-sum identities to transform products of cosine functions into sums, which can then be simplified using the angle sum identities. This is particularly useful when dealing with expressions involving cosine of the sum or difference of two angles.
What is the final identity derived for the expression involving secant squared X over 2 in the video?
-The final identity derived for the expression is 2 over 1 plus cosine X. This is achieved by applying the half-angle identity for cosine and simplifying the resulting expression.
How does the video ensure that the identities used are correctly applied throughout the problems?
-The video ensures correct application by first rewriting all trigonometric functions in terms of sine and cosine, then carefully combining fractions and using the appropriate identities, such as the Pythagorean identity, double angle formulas, and sum-to-product identities, to simplify the expressions step by step.
Outlines
📚 Introduction to Trigonometric Identity Problems
The video begins with an introduction to solving 24 different trigonometric identity problems in one take. The presenter encourages viewers to attempt the problems before watching and provides a file with摩托 (motor) portraits format questions for practice. The first tip is to work primarily with sine and cosine functions, and the presenter demonstrates how to manipulate the given identity involving sine, cotangent, and cosine.
🔍 Simplifying Trigonometric Expressions
The presenter simplifies the expression secant x - cosine x over tangent, by converting secant to its reciprocal form and multiplying by the least common denominator. The video then covers the use of the Pythagorean identity to simplify further. The presenter also explains the concept of combining fractions and the use of special right triangles to understand tangent values.
📐 Working with Complex Fractions and Angle Sum Identities
The video tackles complex fractions and the angle sum identities for tangent and cosine. The presenter demonstrates how to combine fractions by finding a common denominator and how to apply the angle sum identities to expressions involving the tangent of the sum of two angles and the cosine of a triple angle.
🧮 Double Angle Identities and Power Reduction
The presenter discusses the double angle identities for cosine and the power reduction formula for sine. The video shows how to use these identities to simplify expressions like cosine of a triple angle and secant of an inverse sine. The process involves squaring angles, applying the Pythagorean identity, and simplifying using the difference of squares formula.
🤔 Solving Trigonometric Identities with Angle Sum and Difference
The video script focuses on solving trigonometric identities using the angle sum and difference formulas. It covers how to rewrite tangent in terms of sine and cosine, combine fractions with a common denominator, and apply the angle sum formula for sine to expressions like tangent of the sum of two angles.
📉 Trigonometric Power Reduction and Factoring
The presenter works through the power reduction of sine and cosine to the fourth power, using the Pythagorean identity and factoring techniques. The video demonstrates how to simplify expressions by factoring the difference of two squares and applying double angle identities to rewrite trigonometric expressions in a more manageable form.
🔁 Applying Trigonometric Identities to Complex Expressions
The video concludes with solving more complex trigonometric identities, including those involving secant and tangent functions. The presenter uses the Pythagorean identity, power reduction formulas, and angle sum identities to simplify and verify the given expressions. The process involves multiplying by the least common denominator, factoring, and canceling out terms.
🎓 Final Thoughts and Upcoming Series
In the final paragraph, the presenter wraps up the session on verifying trigonometric identities and introduces the concept of the half-angle identity. They also express their intention to create a series on finding the domain of functions, inviting feedback from viewers on what topics they would like to see covered next.
Mindmap
Keywords
💡Trigonometric Identity
💡Pythagorean Identity
💡Double Angle Identity
💡Sum and Difference Identities
💡Trigonometric Function
💡Unit Circle
💡Reduction Formula
💡Angle Sum Formula
💡Special Right Triangles
💡Power Reduction
💡Trigonometric Substitution
Highlights
The video covers 24 different trigonometric identity problems, providing a comprehensive guide for students.
Emphasizes the importance of practicing trigonometric identities, especially for those taking pre-calculus classes.
Introduces the concept of working primarily with sine and cosine functions to simplify problems.
Demonstrates the use of the Pythagorean identity (sine^2(x) + cosine^2(x) = 1) as a common tool in solving trigonometric problems.
Explains the transformation of cotangent to sine and cosine ratios, which is a key step in many identity verifications.
Shows how to combine fractions with different denominators by finding a common denominator.
Uses the unit circle and properties of special triangles to explain and verify trigonometric identities.
Teaches the technique of multiplying by 1 in the form of an expression to create a common denominator.
Covers the triple angle identity for cosine, which is a more advanced topic in trigonometry.
Discusses the application of the angle sum identity for cosine in simplifying expressions involving multiple angles.
Introduces the concept of secant and its relationship with cosine, which is crucial for understanding certain identities.
Provides a method for simplifying complex fractions by multiplying by the denominator to clear the fraction.
Demonstrates the use of the difference of squares formula in simplifying trigonometric expressions.
Explains the double angle identity for tangent and how it can be used to simplify expressions involving the tangent of a double angle.
Shows how to use the product-to-sum identities to simplify products of cosine functions with different angles.
Presents the reduction formula for sine to the fourth power, which is useful in calculus integrations.
Concludes with the half-angle identity for secant, which is a less common but still important identity in trigonometry.
Transcripts
Browse More Related Video

Pythagorean Identities for Trigonometric Functions (Precalculus - Trigonometry 10)
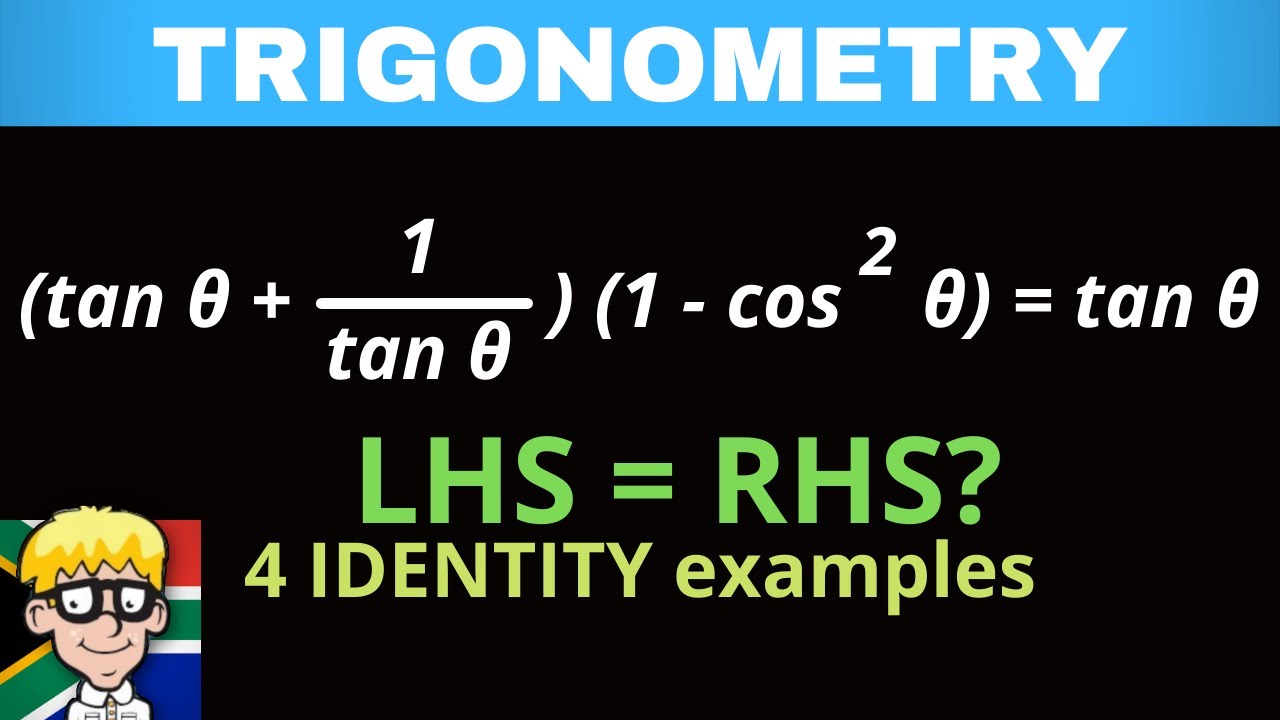
Identities Grade 11: Introduction and practice

Introduction to Sum and Difference Formulas in Trigonometry (Precalculus - Trigonometry 25)

03 - Pythagorean Trig Identities, Part 1
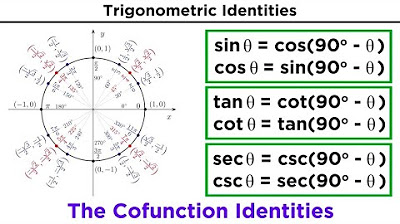
Basic Trigonometric Identities: Pythagorean Identities and Cofunction Identities
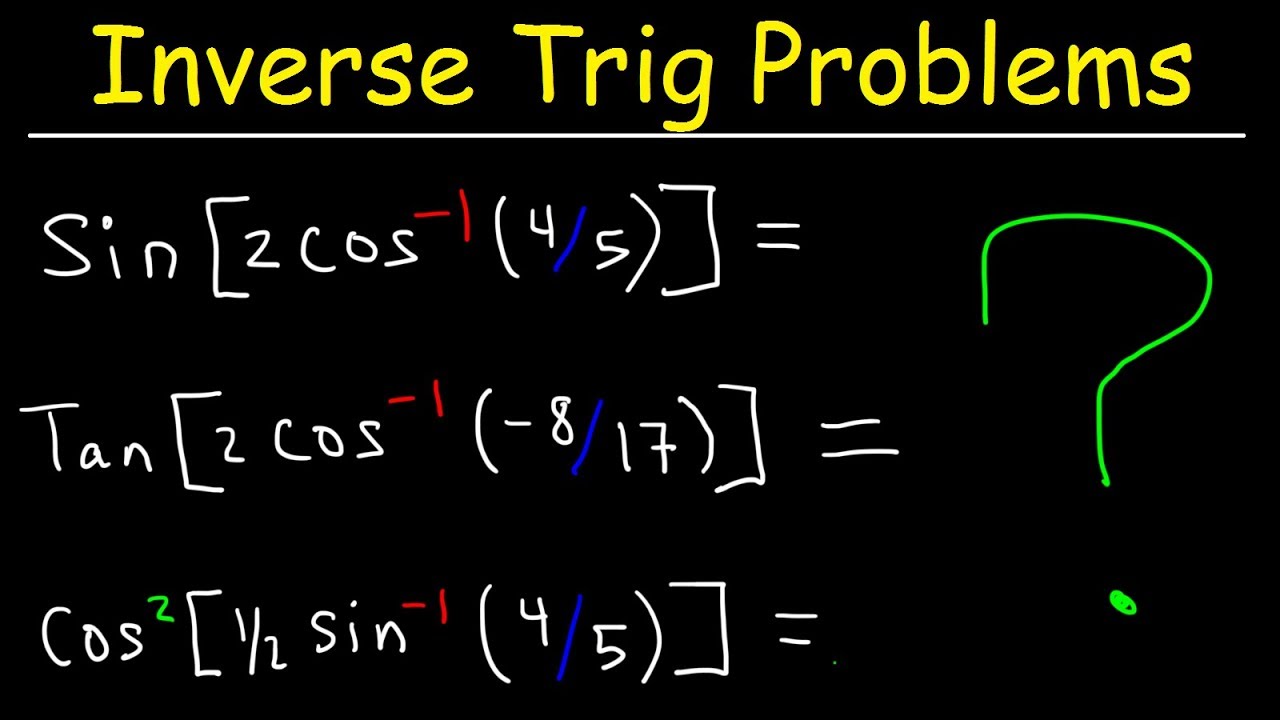
Inverse Trig Functions With Double Angle Formulas and Half Angle Identities - Trigonometry
5.0 / 5 (0 votes)
Thanks for rating: