How To Evaluate Composite Functions Using Function Tables | Precalculus

The Organic Chemistry Tutor
13 Nov 201905:54
EducationalLearning
32 Likes 10 Comments

TLDRThis educational video script guides viewers through the process of evaluating composite functions using tables. It begins by explaining the concept of composite functions, represented as f(g(x)), and demonstrates step-by-step how to find the value of such expressions. The script provides several examples, including f(g(2)), g(f(1)), f(f(2)), and g(g(1)), showing how to evaluate the inner function first and then the outer function. It also covers more complex expressions involving addition and subtraction of composite functions. The video encourages practice by inviting viewers to solve additional problems, such as f(g(4)+g(f(2))) and f(f(5)-g(g(3))), reinforcing the understanding of composite functions and their evaluation.

Takeaways
  • 📚 The video focuses on evaluating composite functions using tables.
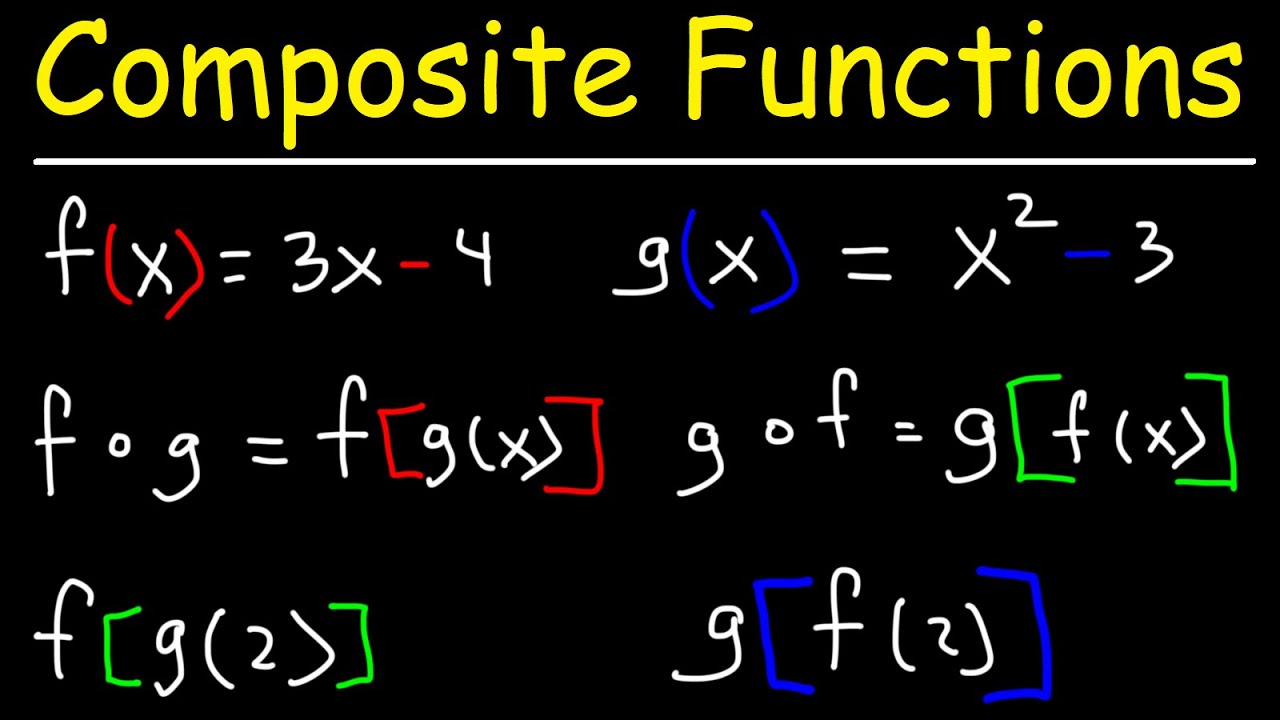
  • 🔍 The expression f(g(x)) is explained, where f is the outer function and g is the inner function.
  • 👉 The first step in evaluation is to determine the value of the inner function (g(x)) for a given x.
  • ✅ Example given: g(2) is found to be 3, then f(g(2)) is calculated as f(3).
  • 📈 The value of f(3) is found to be -4, demonstrating the process of composite function evaluation.
  • 🔄 Another example is provided: g(f(1)) is calculated by first finding f(1) and then g(5).
  • 📝 The result of g(f(1)) is given as -7, reinforcing the evaluation process.
  • 💡 Practice problems are suggested for the viewer to apply the evaluation method to f(f(2)) and g(g(1)).
  • 🎯 The solutions for f(f(2)) and g(g(1)) are provided, showing the process and resulting in 9 and 5, respectively.
  • 🧩 Additional problems are introduced to practice evaluating composite functions with operations like addition and subtraction.
  • 📊 The final examples involve f(g(4) + g(f(2)) and f(f(5) - g(g(3)), with solutions provided to demonstrate the complete process.
Q & A

  • What is the basic concept of evaluating composite functions?

    -The basic concept of evaluating composite functions involves understanding that the function written first (outer function) acts on the function written second (inner function). You evaluate the inner function first and then use its result as the input for the outer function.

  • How do you interpret the expression f(g(2))?

    -The expression f(g(2)) means that you first evaluate the function g at the point x=2, and then take the result of that evaluation and use it as the input for the function f.

  • What is the value of g(2) according to the video?

    -According to the video, when x is 2, the value of g is 3. So, g(2) is equal to 3.

  • What is the value of f(3) as per the given table in the video?

    -Based on the table provided in the video, when x is 3, the value of f is -4. So, f(3) is equal to -4.

  • How do you evaluate the expression g(f(1))?

    -To evaluate g(f(1)), you first find the value of f when x is 1, which is 5 according to the video. Then, you substitute this value into g, so you evaluate g(5), which is -7.

  • What is the result of the expression f(f(2))?

    -Following the steps of evaluating composite functions, f(f(2)) is calculated by first finding f(2) which is 4, and then finding f(4) which is 9. So, f(f(2)) equals 9.

  • How does the video explain the process of evaluating g(g(1))?

    -The video explains that for g(g(1)), you first evaluate g(1) which is 3, and then you evaluate g(3) which is 5. Thus, g(g(1)) equals 5.

  • What is the value of f(g(4)) + g(f(2)) as shown in the video?

    -In the video, it's shown that g(4) is 1 and f(2) is 4. Then, f(1) (since g(4) gave us 1) is 5. So, f(g(4)) + g(f(2)) equals 5 + 1, which is 6.

  • How do you find the result of f(f(5)) - g(g(3)) according to the video?

    -The video demonstrates that f(5) is 0 and g(3) is 5. Then, f(0) is 10 and g(5) is -7. The result is 10 - (-7), which simplifies to 10 + 7, equaling 17.

  • What is the purpose of using a table to evaluate composite functions?

    -Using a table helps in systematically evaluating composite functions by clearly showing the input and output values of each function at each step, making it easier to follow the process and find the correct result.

Outlines
00:00
📚 Evaluating Composite Functions with Tables

This paragraph introduces the concept of evaluating composite functions using tables. It explains the process by first identifying the inner and outer functions, in this case, f(g(x)). The example given walks through finding f(g(2)) step by step: first evaluating g(2) which equals 3, then substituting this value to find f(3) which equals -4. The paragraph also invites the viewer to try evaluating g(f(1)) on their own, providing a brief explanation of how to do so.

05:01
🔍 Solving More Composite Function Problems

The second paragraph continues the theme of evaluating composite functions, but this time it involves more complex expressions such as g(f(1)), f(f(2)), and g(g(1)). It provides the solutions to these problems, showing the step-by-step process of substitution and evaluation. For instance, g(f(1)) is found by first evaluating f(1) to get 5, then finding g(5) which is -7. The paragraph also presents additional practice problems for the viewer to solve, such as f(g(4) + g(f(2))) and f(f(5) - g(g(3))), and provides the solutions to these problems, concluding with the final answer of 17 for the last example.

Mindmap
Keywords
💡Composite Functions
Composite functions are formed by applying one function to the result of another. They are denoted as f(g(x)) and represent the concept where function f is performed after function g on the input x. In the video, the theme revolves around evaluating these composite functions using tables, which is a fundamental concept in understanding function composition in mathematics.
💡Function Evaluation
Function evaluation refers to the process of determining the output of a function for a given input. In the context of the video, it is the step-by-step process of finding the result of a composite function by first evaluating the inner function and then using its result as the input for the outer function.
💡Inner Function
The inner function is the function that is applied first in a composite function. It is the 'g' in the expression f(g(x)). In the video, the inner function is evaluated first to determine its output, which then serves as the input for the outer function.
💡Outer Function
The outer function is the function that is applied second in a composite function. It is represented as 'f' in the expression f(g(x)). After evaluating the inner function, the outer function uses the result from the inner function as its input to produce the final output.
💡Function Table
A function table is a tool used to organize and visualize the input-output pairs of a function. In the video, function tables are used to simplify the process of evaluating composite functions by allowing viewers to look up the values of functions for specific inputs quickly.
💡Input and Output
In the context of functions, input refers to the value(s) that are put into a function, while output is the value that the function produces. The video script uses the terms to describe the process of evaluating functions, where an input to one function becomes the output of another.
💡Rewriting Expressions
Rewriting expressions involves manipulating mathematical expressions to make them easier to understand or to solve. In the video, rewriting is used to clarify the order in which functions are composed and to simplify the process of evaluating composite functions.
💡Example Problems
Example problems are used in educational content to illustrate how to apply concepts or solve for unknowns. The video provides several example problems to demonstrate the process of evaluating composite functions using function tables, helping viewers understand the steps involved.
💡Practice Problems
Practice problems are exercises given to learners to reinforce their understanding of a concept through active engagement. The video encourages viewers to work on practice problems involving composite functions to solidify their grasp of the material.
💡Negative Values
Negative values are numbers that are less than zero. In the video, negative values are part of the function outputs, demonstrating that functions can produce both positive and negative results depending on the input.
💡Arithmetic Operations
Arithmetic operations such as addition and subtraction are used in the video to combine the results of evaluating composite functions. For example, the script mentions adding the results of g(f(1)) and f(g(4)) to find a final answer.
Highlights

Introduction to evaluating composite functions using tables.

Explanation of the composite function notation f(g(x)) as f of g of x.

Step-by-step process to evaluate f(g(2)) where g(2) is found to be 3.

Finding f(3) equals -4 to solve the first example.

Encouragement to try the exercise g(f(1)) using the table.

Solving g(f(1)) by first finding f(1) equals 5, then g(5) equals -7.

Practice exercise: Evaluate f(f(2)) and g(g(1)) using the table.

Solution for f(f(2)) is f(4) which equals 9.

Solution for g(g(1)) is g(3) which equals 5.

Instruction to work on additional problems involving composite functions.

Example problem: f(g(4)) + g(f(2)) equals 6.

Detailed steps to evaluate f(g(4)) and g(f(2)) separately.

Practice exercise: f(f(5)) - g(g(3)) equals 17.

Solution for f(f(5)) is f(0) which equals 10.

Solution for g(g(3)) is g(5) which equals -7.

Final summary on how to evaluate composite functions using a table.

Transcripts

Browse More Related Video

Composite Functions

Composite and Inverse Functions

Composite Function Explained - The Basics You NEED To Know!

Composite Functions

Composition of Functions (Precalculus - College Algebra 48)

Composition of Function - General Mathematics - Grade 11

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Composite FunctionsMathematicsEvaluationTablesEducationalProblem SolvingFunction TablesMath TutorialLearningPractice Problems