Composite Function Explained - The Basics You NEED To Know!

Math Geeks And Science Freaks
2 Oct 202205:09
EducationalLearning
32 Likes 10 Comments

TLDRThis transcript explains the concept of composite functions, where one function's output serves as the input for another. It illustrates two notations for representing composite functions and provides examples of calculating F(G(x)) and G(F(x)). The process involves replacing the x in the inner function with the entire outer function and simplifying the result. The transcript also demonstrates how to evaluate composite functions at specific values, such as F(G(3)) and G(F(3)), emphasizing the importance of understanding the order of operations and function composition for problem-solving in mathematics.

Takeaways
  • 📚 Composite functions are created when one function is used as the input for another function.
  • 🔢 Two common notations for composite functions are F(G(x)) and f(G(x)), where G(x) is the inner function.
  • ⚠️ Be cautious with notation: f(𝓰(x)) represents composition, not f * G(x) which would mean multiplication.
  • 🔍 To find F(G(x)), substitute G(x) into the x value of the F(x) function and simplify.
  • 🔄 For G(F(x)), insert the entire F(x) function into the x value of the G(x) function.
  • 📈 Example: If G(x) = x + 6 and F(x) = 2x + 5, then F(G(x)) = 2(x + 6) + 5 simplifies to 2x + 17.
  • 📊 If F(x) = x^2 + 5 and G(x) = x - 1, then F(G(x)) is (x - 1)^2 + 5 which simplifies to x^2 - x + 1 + 5.
  • 🧩 For G(F(x)), substitute F(x) into the x in G(x), resulting in G(F(x)) = (x^2 + 5) - 1, which simplifies to x^2 + 4.
  • 🎯 To evaluate F(G(3)), first find G(3) = 3 - 1 = 2, then substitute 2 into F(x) to get F(G(3)) = 2^2 + 5 = 9.
  • 🎯 For G(F(3)), first find F(3) = 3^2 + 5 = 14, then substitute 14 into G(x) to get G(F(3)) = 14 - 1 = 13.
  • 📖 Understanding composite functions is a fundamental concept in mathematics that is essential for problem-solving in algebra and calculus.
Q & A

  • What is a composite function?

    -A composite function is created when one function is used as the input for another function.

  • How can a composite function be written?

    -A composite function can be written as F(G(x)) or f(G(x)), where G(x) is the interior function.

  • What does it mean for G(x) to be the interior function?

    -When G(x) is the interior function, it means that the entire G(x) expression (e.g., x + 6) is substituted into the x value of the outer function, F(x).

  • How do you find F(G(x)) when given F(x) = 2x + 5 and G(x) = x + 6?

    -You substitute G(x) into F(x), resulting in F(G(x)) = 2(x + 6) + 5, which simplifies to 2x + 17.

  • How do you find G(f(x)) when given F(x) = 2x + 5 and G(x) = x + 6?

    -You substitute F(x) into G(x), resulting in G(f(x)) = (2x + 5) + 6, which simplifies to 2x + 11.

  • What is the difference between F(G(x)) and G(f(x))?

    -F(G(x)) involves substituting the inner function G(x) into F(x), while G(f(x)) involves substituting the inner function F(x) into G(x).

  • How do you evaluate F(G(3)) with F(x) = 2x + 5 and G(x) = x + 6?

    -First, find G(3) which is 3 + 6 = 2, then substitute 2 into F(x) to get F(2) = 2*2 + 5 = 9.

  • How do you evaluate G(f(3)) with F(x) = 2x + 5 and G(x) = x + 6?

    -First, find f(3) which is 2*3 + 5 = 11, then substitute 11 into G(x) to get G(11) = 11 + 6 = 17.

  • What is the final result of F(G(3)) with the given functions?

    -The final result of F(G(3)) is 9.

  • What is the final result of G(f(3)) with the given functions?

    -The final result of G(f(3)) is 13.

  • How does the order of composition affect the final result?

    -The order of composition affects the final result because it determines which function is the interior function and thus which values are substituted into the other function.

  • What is the significance of understanding composite functions in mathematics?

    -Understanding composite functions is important as it allows for the analysis and manipulation of more complex functions, which is crucial in various mathematical and real-world applications.

Outlines
00:00
📚 Introduction to Composite Functions

This paragraph introduces the concept of composite functions, explaining how they are formed by using one function as the input for another. It outlines two notations for representing composite functions: F(G(x)) and f⊚G(x), emphasizing the difference from the product of functions, F×G(x). The paragraph then walks through the process of finding F(G(x)) by substituting G(x) into the F(x) function, using a specific example where G(x) = x+6. The result of this substitution is simplified to 2x + 12 + 5, leading to the final simplified form of 2x + 17. The explanation continues with finding G(f(x)) by substituting the entire F(x) function into G(x), resulting in 2x + 11. The paragraph concludes with a second example of finding F(G(x)) and G(f(x)) using different functions, and then applies these concepts to evaluate F(G(3)) and G(f(3)) with step-by-step simplifications.

Mindmap
Keywords
💡composite function
A composite function is created when one function is used as the input for another function. It is a fundamental concept in mathematics where functions are combined to create new functions. In the video, composite functions are represented in two ways: F(G(x)) and f∘G(x), which both indicate that the output of function G(x) is used as the input for function f(x). The video provides examples of how to compute composite functions, such as finding F(G(x)) by substituting G(x) into the function f(x).
💡interior function
The interior function in a composite function is the function that is nested inside the other function. It is the function that takes the input first and its output is then used as the input for the exterior function. In the context of the video, the interior function is denoted by G(x), and it is the function that is plugged into the F(x) function to create the composite function F(G(x)).
💡function composition
Function composition, also known as composite function, refers to the process of applying one function to the result of another function. It is a mathematical operation where the output of one function becomes the input of another. The video explains how to perform function composition by substituting the interior function into the exterior function and simplifying the resulting expression.
💡simplifying expressions
Simplifying expressions involves using mathematical rules to reduce complex expressions to their simplest form. This process is essential in mathematics and is particularly important when working with composite functions, as it helps to make the functions easier to understand and compute. In the video, simplifying expressions is demonstrated when calculating composite functions by distributing, combining like terms, and removing unnecessary parentheses.
💡distributing
Distributing is a mathematical operation that involves multiplying a number outside of parentheses by each term inside the parentheses. It is a key step in simplifying expressions, especially when dealing with composite functions that involve multiplication and addition. The video illustrates the process of distributing a number across terms to simplify the composite function.
💡like terms
Like terms are terms in a mathematical expression that contain the same variables raised to the same power. They can be combined or added together to simplify an expression. The concept is crucial when simplifying composite functions, as it allows for the reduction of complex expressions to a more manageable form.
💡evaluating functions
Evaluating a function involves finding the output of the function for a specific input value. It is a fundamental skill in mathematics and is demonstrated in the video through the computation of composite functions at particular values, such as F(G(3)) or G(F(3)).
💡function notation
Function notation is a way of expressing a function using a formula that includes the independent variable and the function's name or a symbol representing the function. It is a standard method for writing mathematical functions and is used throughout the video to describe and compute composite functions.
💡nested functions
Nested functions occur when one function is used as the argument for another function. This is a common scenario in composite functions where the output of one function (the inner or nested function) becomes the input for the outer function. The video explains how to work with nested functions by substituting the inner function into the outer function to find the composite function's value.
💡exponents
Exponents are a mathematical notation that indicate how many times a number (the base) is multiplied by itself. They are used in various mathematical operations, including simplifying expressions and computing functions. In the context of the video, exponents are used when squaring terms, such as in the computation of F(G(x)) where x - 1 is squared.
💡algebraic manipulation
Algebraic manipulation refers to the process of transforming and rearranging algebraic expressions according to mathematical rules to simplify or solve equations. This includes operations such as distributing, combining like terms, and factoring. The video demonstrates algebraic manipulation when simplifying the expressions for composite functions.
Highlights

Composite functions are created when one function is used as the input for another.

Composite functions can be written in two ways: F(G(x)) or f⊗(G(x)).

The interior function (G(x)) is fully substituted into the outer function (F(x)).

An example of finding F(G(x)) involves simplifying 2(G(x)) + 5 to get 2x + 12 + 5, which simplifies to 2x + 17.

For G(f(x)), the process is similar but the roles of the functions F and G are reversed.

When finding G(f(x)), the result is 2x + 11 after simplifying the expression.

For F(G(x)), if the interior function is (x - 1)^2, the result after simplification is x^2 - 2x + 6.

In the case of G(f(x)), with the interior function being x^2 + 5, the final simplified result is x^2 + 4.

To find F(G(3)), first determine G(3) and then substitute that value into the F function.

G(3) equals 2, which when substituted into F, results in F(2) = 2^2 + 5, simplifying to 9.

For G(f(3)), first calculate f(3) which equals 14, then substitute this into G to get G(14) = 13.

The final result for F(G(3)) is 9, showcasing the practical application of composite functions.

The final result for G(f(3)) is 13, demonstrating the reverse application of composite functions.

Understanding composite functions is crucial for mastering algebra and higher-level mathematics.

Composite functions have practical applications in various fields such as physics and engineering.

The process of finding composite functions helps in problem-solving and developing a deeper understanding of mathematical concepts.

The video provides a clear and detailed explanation of composite functions, making it an excellent resource for learners.

The transcript includes examples and step-by-step instructions for finding F(G(x)) and G(f(x)), enhancing comprehension.

The video encourages viewers to subscribe for more educational content on mathematics and related topics.

Transcripts

Browse More Related Video

Composition of Functions (Precalculus - College Algebra 48)

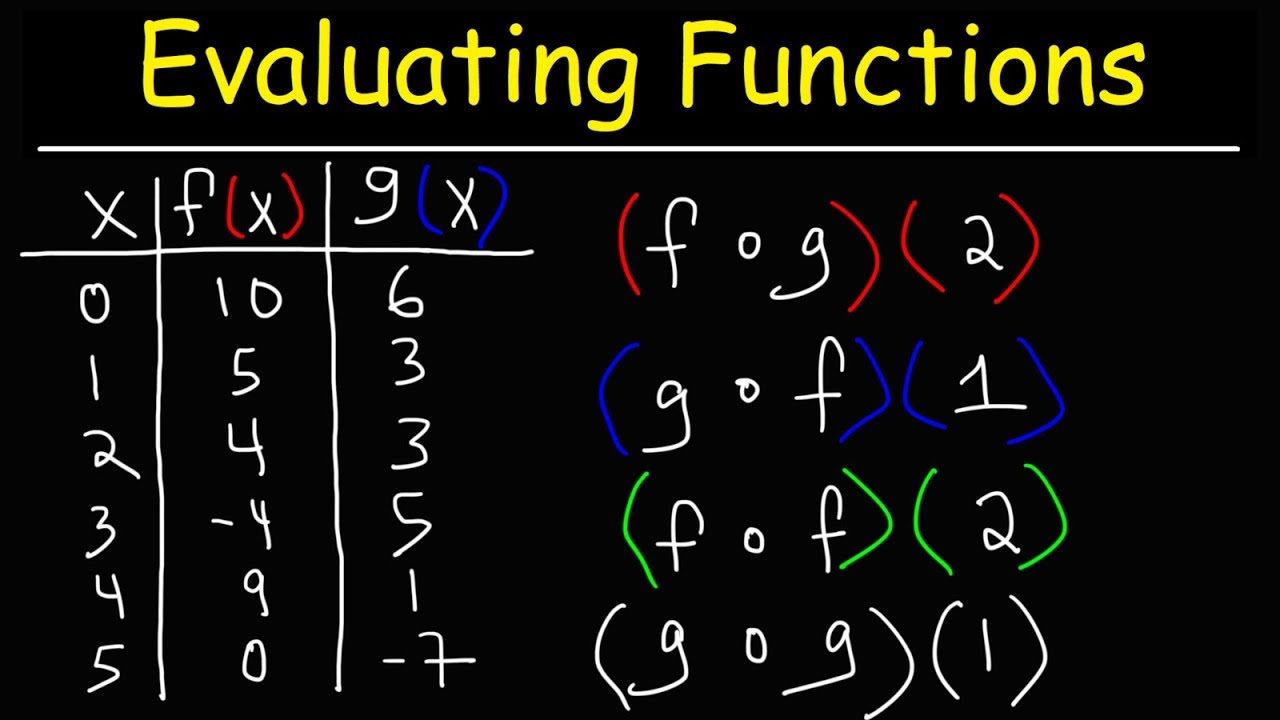
How To Evaluate Composite Functions Using Function Tables | Precalculus

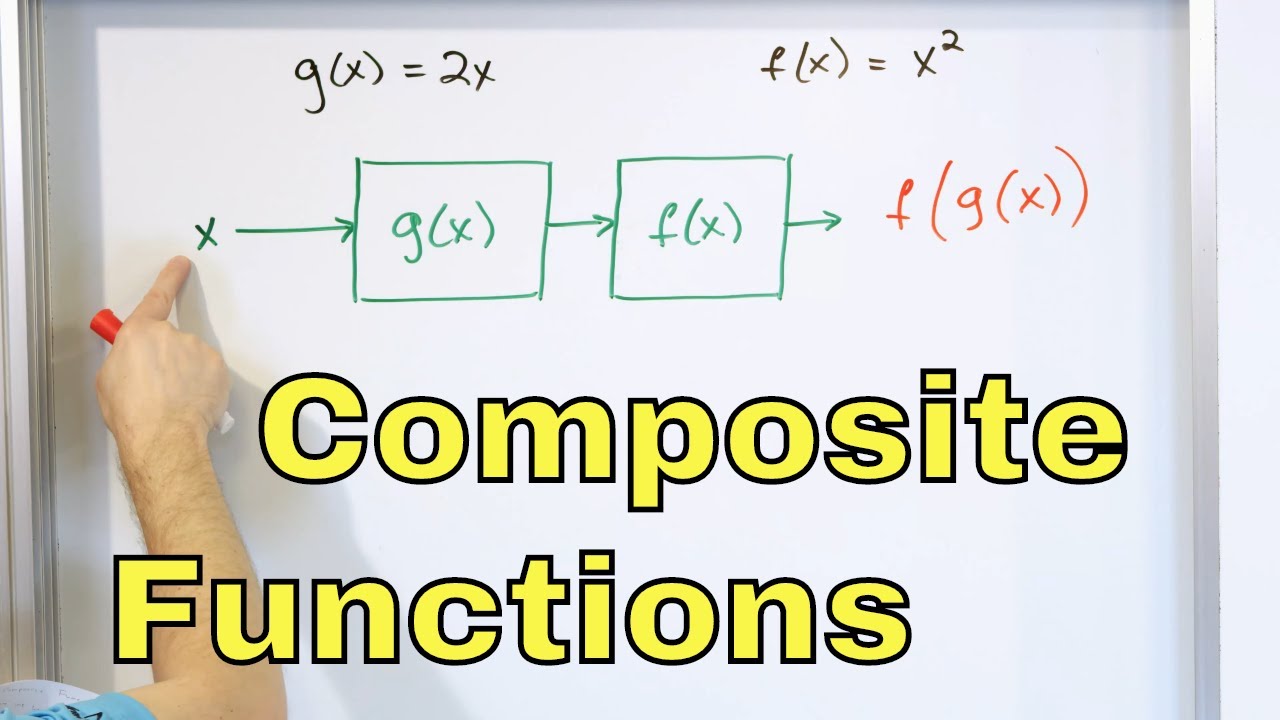
10 - What are Composite Functions? (Part 1) - Evaluating Composition of Functions & Examples

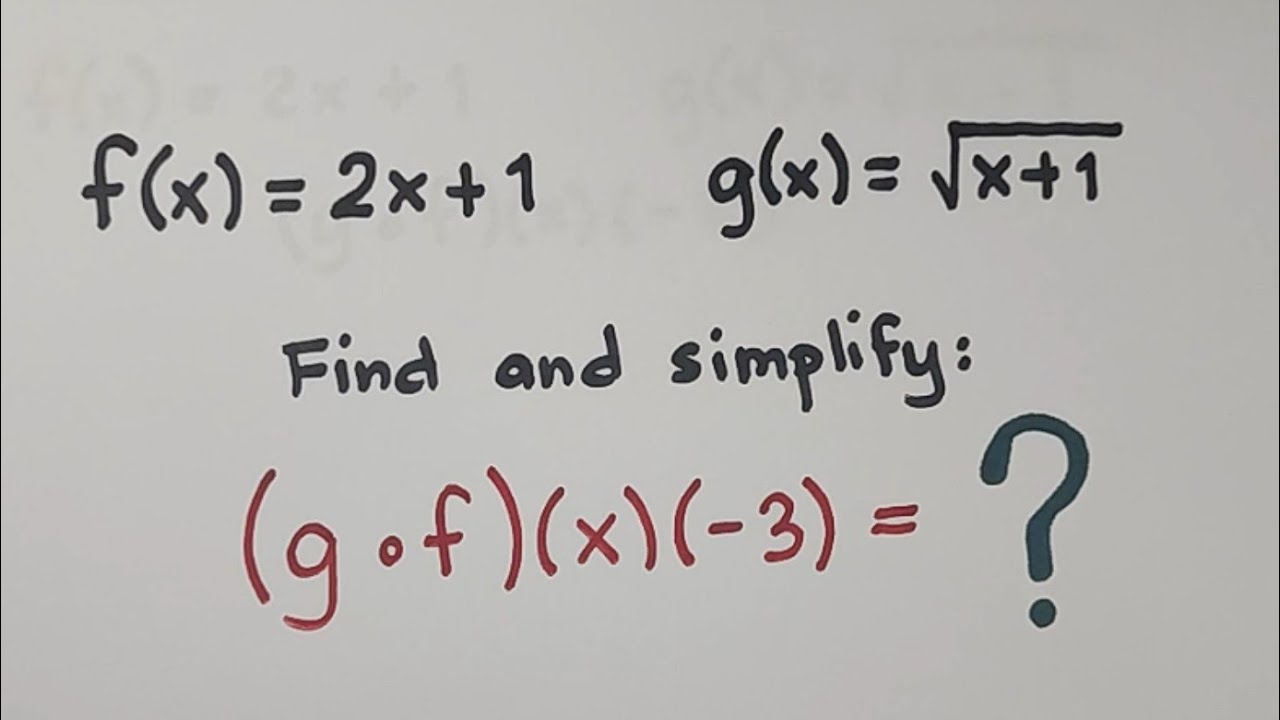
Composition of Function - General Mathematics - Grade 11

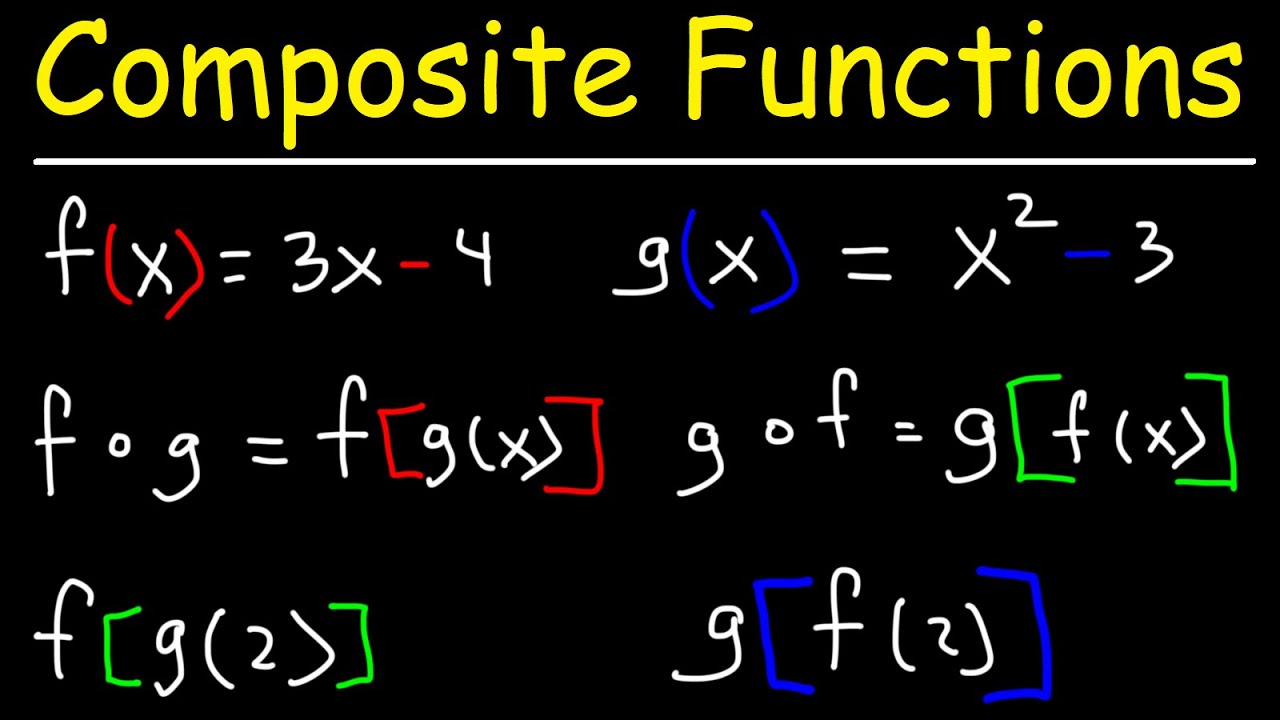
Composite Functions

Manipulating Functions Algebraically and Evaluating Composite Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsComposite FunctionsFunction CompositionAlgebraEducationTutorialMath HelpSimplificationCalculationMathematics Education