Introduction to the t Distribution (non-technical)

jbstatistics
4 May 201308:54
EducationalLearning
32 Likes 10 Comments

TLDRThis video introduces the Student t-distribution, commonly used when estimating population parameters like the mean when the population standard deviation is unknown. It explains that instead of using the population standard deviation, we use the sample standard deviation, which leads to a t-distribution with n-1 degrees of freedom. The t-distribution resembles the standard normal distribution but has heavier tails and a lower peak, indicating more variability. As degrees of freedom increase, the t-distribution approaches the standard normal distribution. The video also discusses the implications for constructing confidence intervals, emphasizing the importance of using t-values instead of z-values when the population standard deviation is unknown, regardless of sample size.

Takeaways
  • πŸ“š The video introduces the Student t distribution, often just called the t distribution, with a focus on its application rather than mathematical derivations.
  • πŸ” The t distribution is used when estimating the population mean from a sample, particularly when the population standard deviation (sigma) is unknown.
  • πŸ“‰ In place of sigma, the sample standard deviation (s) is used, leading to a new statistic, which follows the t distribution rather than the standard normal distribution.
  • πŸ”‘ The t distribution is characterized by its degrees of freedom, which is typically the sample size minus one (n-1).
  • πŸ“Š The t distribution resembles the standard normal distribution but has heavier tails and a lower peak, indicating more variability.
  • πŸ“ˆ As the degrees of freedom increase, the t distribution approaches the standard normal distribution, becoming almost identical at high degrees of freedom.
  • πŸ“ The shape of the t distribution and the values used in statistical inference, such as constructing confidence intervals, depend on the degrees of freedom.
  • πŸ“‰ When constructing a 95% confidence interval and the population standard deviation is unknown, the t distribution must be used instead of the standard normal distribution.
  • πŸ”’ The appropriate t value for a confidence interval is found using a t table or software, and it varies with the degrees of freedom and the desired confidence level.
  • 🚫 Contrary to some opinions, even with large sample sizes, it is important to use the t distribution rather than the standard normal distribution when estimating with sample data.
  • πŸ“‹ The video emphasizes the importance of using the correct statistical methods based on the data's characteristics, such as whether the population standard deviation is known or not.
Q & A

  • What is the t distribution?

    -The t distribution, often shortened to 't', is a type of probability distribution that is used when estimating the mean of a normally distributed population when the population standard deviation is unknown. It is similar to the standard normal distribution but has heavier tails and a lower peak, accounting for greater variability when using sample standard deviation instead of the population standard deviation.

  • Why do we use the sample standard deviation instead of the population standard deviation in practice?

    -In practice, the population standard deviation (sigma) is often unknown, which makes it impossible to use directly in formulas. Therefore, we use the sample standard deviation (s) as an estimate for sigma to construct confidence intervals and perform statistical inference.

  • What is the formula for the t statistic?

    -The t statistic is calculated using the formula: (X bar - mu) / (s / sqrt(n)), where X bar is the sample mean, mu is the population mean, s is the sample standard deviation, and n is the sample size.

  • What are degrees of freedom in the context of the t distribution?

    -Degrees of freedom in the context of the t distribution refer to the number of independent observations that contribute to estimating the variance. For the t distribution, the degrees of freedom are typically the sample size minus one (n-1).

  • How does the shape of the t distribution change with an increase in degrees of freedom?

    -As the degrees of freedom increase, the t distribution tends to resemble the standard normal distribution more closely. It has lighter tails and a higher peak as it approaches the shape of the standard normal distribution.

  • What is the relationship between the t distribution and the standard normal distribution?

    -The t distribution approaches the standard normal distribution as the degrees of freedom increase. At infinite degrees of freedom, the t distribution is identical to the standard normal distribution.

  • How does the t distribution differ from the standard normal distribution in terms of tails and peak?

    -The t distribution has heavier tails and a lower peak compared to the standard normal distribution. This means that there is a higher probability of observing values that are far from the mean in the t distribution.

  • What is the significance of the t distribution in constructing confidence intervals when the population standard deviation is unknown?

    -When the population standard deviation is unknown, using the t distribution to construct confidence intervals accounts for the additional uncertainty introduced by estimating the standard deviation from the sample. This results in a more accurate and wider interval that reflects the greater variability in the sample data.

  • Why is it incorrect to use the standard normal distribution (z-values) when the sample standard deviation is used in the calculation?

    -Using z-values when the sample standard deviation is used can lead to an underestimation of the margin of error because the t distribution, which should be used in this case, has heavier tails and accounts for greater variability than the standard normal distribution.

  • What is the appropriate t value for a 95% confidence interval with five degrees of freedom?

    -With five degrees of freedom, the appropriate t value for a 95% confidence interval is 2.571, which is larger than the z-value of 1.96 used for the standard normal distribution.

  • Is it ever appropriate to use the standard normal distribution instead of the t distribution, regardless of the sample size?

    -It is not appropriate to use the standard normal distribution instead of the t distribution when the population standard deviation is unknown and the sample standard deviation is used, regardless of the sample size. The t distribution should be used to account for the variability introduced by estimating the standard deviation from the sample.

Outlines
00:00
πŸ“š Introduction to the Student t Distribution

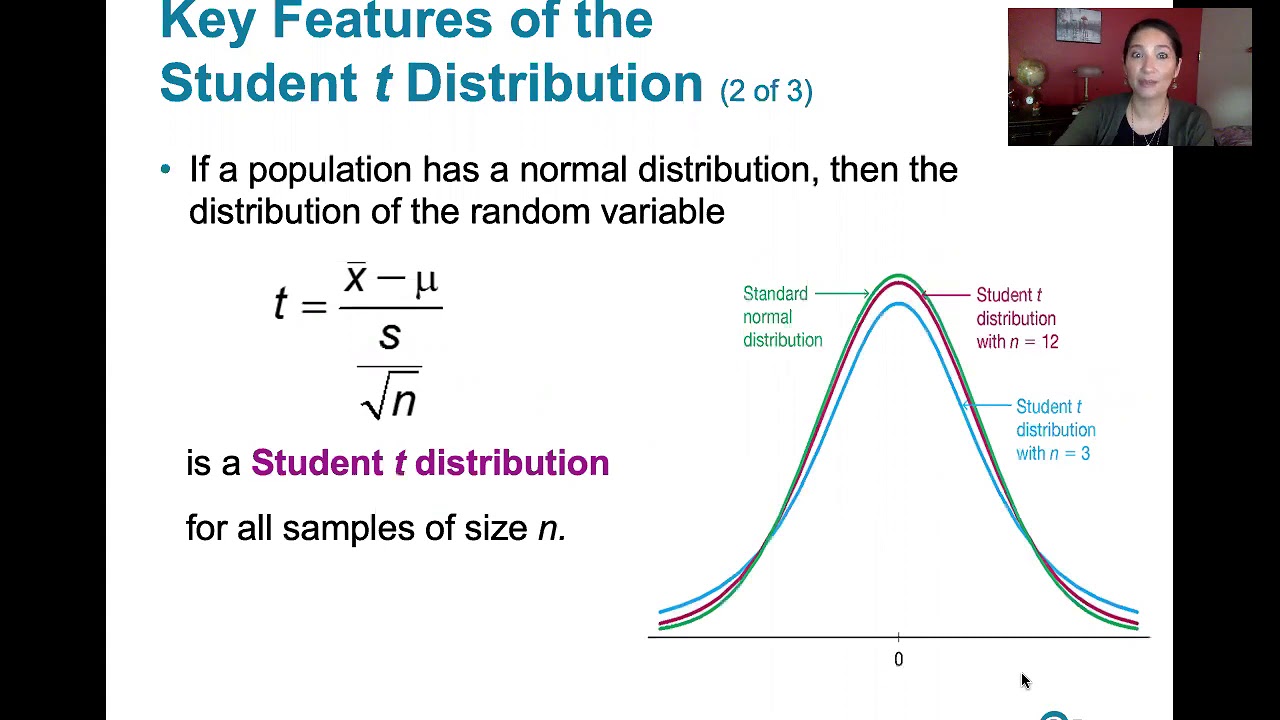
This paragraph introduces the concept of the Student t distribution, often just called the t distribution, which is used when estimating the population mean from a normally distributed population when the population standard deviation is unknown. The video script explains that instead of using the population standard deviation (sigma), we use the sample standard deviation (s) to estimate it. This leads to a new statistic, labeled as t, which has a t distribution with n-1 degrees of freedom. The paragraph also touches on the concept of degrees of freedom and how the t distribution resembles the standard normal distribution but with greater variability, as shown through a comparison of their respective plots. The t distribution's shape varies with degrees of freedom, and as these increase, the t distribution approaches the standard normal distribution.

05:01
πŸ“‰ Comparing t Distribution and Standard Normal Distribution in Confidence Intervals

This paragraph delves into the practical application of the t distribution, particularly in constructing confidence intervals for the population mean when the population standard deviation is unknown. It contrasts the process of using the standard normal distribution (Z distribution) with that of the t distribution. The script explains that when sigma is known, the Z statistic and its corresponding value (z_.025 = 1.96) are used, but when sigma is unknown and replaced with the sample standard deviation, the t statistic and its corresponding t value from the t distribution must be used. The paragraph includes a table showing how the appropriate t value for a 95% confidence interval changes with different degrees of freedom, highlighting that even with large sample sizes, the t distribution should not be disregarded in favor of the standard normal distribution. The importance of using the correct distribution to avoid underestimating the margin of error is emphasized.

Mindmap
Keywords
πŸ’‘Student t distribution
The Student t distribution, often simply referred to as the t distribution, is a probability distribution that is used in inferential statistics when the sample size is relatively small and the population standard deviation is unknown. It is central to the video's theme as it is the main focus of the explanation. The script discusses how the t distribution arises when using the sample standard deviation to estimate the population standard deviation in normally distributed populations.
πŸ’‘Random sample
A random sample is a subset of a population where each member of the population has an equal chance of being selected. In the context of the video, the concept is fundamental as it sets the stage for the introduction of the t distribution, which is used when drawing random samples from a normally distributed population.
πŸ’‘Standard normal distribution
The standard normal distribution, often represented by the letter Z, is a type of normal distribution with a mean of 0 and a standard deviation of 1. The video explains that the standard normal distribution is used when the population standard deviation is known, which contrasts with the situation where the t distribution is necessary due to unknown population standard deviation.
πŸ’‘Population standard deviation (sigma)
Population standard deviation (Οƒ) is a measure of the amount of variation or dispersion in a set of values in a population. The video emphasizes the problem of not knowing the value of sigma in practice, which leads to the use of the t distribution instead of the standard normal distribution for constructing confidence intervals.
πŸ’‘Sample standard deviation (s)
Sample standard deviation (s) is an estimate of the population standard deviation, calculated from a sample of data. The script explains that when the population standard deviation is unknown, s is used as an estimator, which introduces the t distribution into the calculations for confidence intervals and hypothesis testing.
πŸ’‘Degrees of freedom
Degrees of freedom is a term used in statistics that refers to the number of values in the data set that are free to vary. In the context of the t distribution, the degrees of freedom is n-1, where n is the sample size. The script mentions that as the degrees of freedom increase, the t distribution approaches the standard normal distribution.
πŸ’‘Confidence interval
A confidence interval is a range that is likely to contain the value of an unknown population parameter with a certain level of confidence. The video discusses how the t distribution is used to construct confidence intervals when the population standard deviation is unknown, as opposed to using the standard normal distribution when it is known.
πŸ’‘Variance
Variance is a measure of the dispersion of a set of data points. In the script, it is mentioned in the context of sample variance, which is calculated by taking the sum of the squared differences from the mean, dividing by n-1, and is related to the degrees of freedom concept.
πŸ’‘T statistic
The t statistic is a measure used in hypothesis testing and is calculated as the difference between the sample mean and the hypothesized population mean, divided by the standard error of the mean. The script describes how the t statistic follows a t distribution with n-1 degrees of freedom when the population standard deviation is unknown.
πŸ’‘Normal distribution
Normal distribution, also known as Gaussian distribution, is a continuous probability distribution that is symmetric about the mean, showing a bell-shaped curve. The video script uses the normal distribution as a reference point to explain how the t distribution behaves similarly but with heavier tails due to the use of the sample standard deviation.
πŸ’‘Margin of error
Margin of error is the range added to or subtracted from an estimate to account for possible errors in the results. The video script explains that when using the t distribution instead of the standard normal distribution, the margin of error will be larger due to the increased variability represented by the t distribution.
Highlights

Introduction to the Student t distribution, often shortened to t distribution.

The video provides a light approach on mathematical details of the t distribution.

Explanation of the random sample of n observations from a normally distributed population.

Standard normal distribution of X bar minus mu over sigma over the square root of n.

Challenge of not knowing the population standard deviation sigma in practice.

Using sample standard deviation s to estimate unknown population standard deviation.

The statistic X bar minus mu over s over the square root of n follows a t distribution.

t distribution has n-1 degrees of freedom, linked to sample variance calculation.

Visual comparison between the standard normal distribution and t distribution.

t distribution's heavier tails and lower peak compared to the standard normal distribution.

t distribution's shape depends on degrees of freedom and approaches standard normal as degrees of freedom increase.

Demonstration of t distribution converging to standard normal distribution with increasing degrees of freedom.

Implications for statistical inference when constructing a 95% confidence interval.

Difference in using z value (1.96) from standard normal distribution vs. t value from t distribution.

Importance of using t distribution values for constructing confidence intervals when sigma is unknown.

Table of appropriate t values for various degrees of freedom and constructing confidence intervals.

Clarification that even with large sample sizes, t distribution should be used instead of standard normal distribution.

Emphasis on the correct use of t distribution over standard normal distribution regardless of sample size.

Transcripts

Browse More Related Video

Confidence Intervals | Population Mean: Οƒ Unknown

Student's T Distribution - Confidence Intervals & Margin of Error

Elementary Statistics - Chapter 7 - Estimating Parameters and Determining Sample Sizes Part 2

7.2.1 Estimating a Population Mean - Student t Distribution and Finding Critical t Values

Z-statistics vs. T-statistics | Inferential statistics | Probability and Statistics | Khan Academy

Confidence Interval Concept Explained | Statistics Tutorial #7 | MarinStatsLectures

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Student tt-distributionnormal distributionstatistical inferenceconfidence intervalsample standard deviationpopulation meandegrees of freedomstatistical analysisprobability distribution