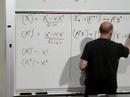Magnetic Force
TLDRIn this AP Physics essentials video, Mr. Andersen explains the concept of magnetic force and its impact on charged particles like protons. He describes how charged particles moving through a magnetic field, such as those from the sun, can spiral into Earth and create phenomena like the Northern Lights. The video delves into the three-dimensional nature of magnetic fields and how to visualize and calculate the magnetic force using the right-hand rule and the cross product of velocity and magnetic field vectors. The formula for magnetic force, F = q(v x B), is introduced, where q is the charge, v is the velocity, and B is the magnetic field. The importance of the angle between the velocity and the magnetic field, denoted by theta, is emphasized, with the sine of this angle determining the magnitude of the force. The video concludes with an example calculation, illustrating how to apply these concepts to understand the force on a proton moving in a magnetic field.
Takeaways
- 🌌 The Northern Lights are caused by charged particles like protons spiraling into Earth's magnetic field, resulting in ionization of colors in the atmosphere.
- 🧲 A magnetic force is only applied to a charged particle when it is moving through a magnetic field; a stationary particle experiences no force.
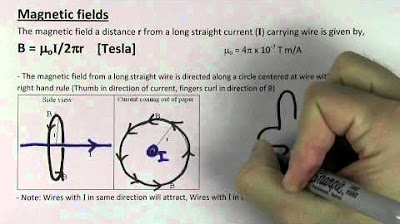
- ➡️ The direction of the magnetic force on a moving charged particle is perpendicular to both the velocity of the particle and the magnetic field.
- 📐 The calculation of magnetic force (F sub n) involves the charge (Q), velocity vector (v), and the magnetic field (B), using a cross product.
- 🤔 The cross product results in a third vector that lies in a different dimension, which can be visualized with an animation.
- ✋ The right-hand rule is used to determine the direction of the magnetic force when a charged particle moves in a magnetic field.
- 🚫 If a charged particle has no velocity in a magnetic field, there is no magnetic force acting on it.
- 🔄 The magnetic force depends on the angle (theta) between the velocity vector and the magnetic field; sine of theta is used to calculate the force's magnitude.
- 🔋 The elementary charge (q) is a key component in the formula for calculating the magnetic force on a charged particle.
- 📐 Units for magnetic force calculation include coulombs for charge, meters per second for velocity, teslas for magnetic field, and newtons for force.
- 📐 Understanding the sine function's role is crucial for determining the magnetic force when the angle between the velocity and magnetic field is not 90 degrees.
Q & A
What is the magnetic force acting on a charged particle?
-The magnetic force, denoted as F_sub_n, is the force applied to a moving charged particle when it passes through a magnetic field. It is perpendicular to both the velocity of the particle and the magnetic field.
How does the Earth's magnetic field influence charged particles from the Sun?
-The Earth's magnetic field acts on charged particles from the Sun, causing them to spiral into the planet, which results in the phenomenon known as the Northern Lights.
What is the significance of the third dimension when dealing with magnetic forces?
-The third dimension is significant because magnetic fields can extend in three-dimensional space, and understanding their effects on charged particles requires considering this three-dimensional aspect.
How can one visualize a magnetic field coming towards or going away from them?
-A magnetic field coming towards you can be visualized as concentric circles or an arrow with the point facing you. Conversely, a field going away from you can be represented by a plus sign or an 'x' inside a circle.
What is the condition for a magnetic force to act on a stationary charged particle?
-A magnetic force will not act on a stationary charged particle. The particle must have a velocity (be moving) for a magnetic force to be applied.
What is the cross product in the context of calculating magnetic force?
-The cross product is a mathematical operation on two vectors that results in a third vector that is perpendicular to the initial two. In the context of magnetic force, it is used to calculate the force vector when a charged particle's velocity vector and the magnetic field vector are given.
What is the right-hand rule used for in the context of magnetic forces?
-The right-hand rule is a mnemonic used to determine the direction of the magnetic force acting on a moving charged particle in a magnetic field. It helps to establish the orientation of the force vector relative to the velocity and magnetic field vectors.
How does the charge of a particle influence the magnetic force it experiences?
-The charge of a particle (Q) is a factor in the magnetic force equation (F = Q * v * sin(theta) * B). The magnitude of the charge directly influences the magnitude of the magnetic force experienced by the particle.
What is the role of the sine function in the magnetic force equation?
-The sine function (sin(theta)) in the magnetic force equation accounts for the angle between the velocity vector of the charged particle and the magnetic field vector. It determines the component of the velocity that is effective in experiencing the magnetic force.
What happens to the magnetic force when the velocity vector and the magnetic field vector are parallel or antiparallel?
-When the velocity vector and the magnetic field vector are parallel or antiparallel (0 degrees or 180 degrees), the sine of the angle between them is zero, resulting in no magnetic force being applied to the charged particle.
How can one calculate the magnetic force acting on a proton moving perpendicular to a magnetic field?
-The magnetic force on a proton moving perpendicular to a magnetic field can be calculated using the equation F = q * v * B, where q is the charge of the proton, v is its velocity, and B is the magnetic field strength. Since the sine of 90 degrees is 1, the sine term is not needed in the calculation.
Outlines
🧲 Magnetic Force on a Charged Particle
In this segment, Mr. Andersen introduces the concept of magnetic force as it applies to charged particles, such as protons. He uses the example of a proton moving through space and explains that a magnetic field, like Earth's, will exert a force on the proton, causing it to spiral into the planet, which contributes to the phenomenon of the Northern Lights. The force is described as acting perpendicularly to both the velocity of the charged particle and the magnetic field itself. The calculation of this force involves the charge (Q), the velocity vector (v), and the magnetic field (B), and is determined through a cross product and the application of the right-hand rule. The segment emphasizes the importance of considering three-dimensional interactions when dealing with magnetic forces.
📐 Calculating Magnetic Force with the Lorentz Force Law
This paragraph delves into the specifics of calculating the magnetic force on a moving charged particle using the Lorentz Force Law. The force is represented by the equation q(v x B), where q is the charge, v is the velocity, and B is the magnetic field, with 'x' indicating a cross product. The force's magnitude is dependent on the angle (theta) between the velocity and the magnetic field, which is addressed using the sine function (sine theta). The right-hand rule is used to determine the direction of the force. The paragraph also discusses the scenarios where the force is zero, such as when the velocity and magnetic field are parallel or antiparallel, and highlights the significance of understanding the sine function in relation to the angle theta. An example problem is provided to illustrate how to calculate the magnetic force on a proton moving perpendicular to a magnetic field.
Mindmap
Keywords
💡Magnetic Force
💡Charged Particle
💡Magnetic Field
💡Northern Lights
💡Ionization
💡
💡Velocity Vector
💡Cross Product
💡Right Hand Rule
💡Sine of Theta (sin θ)
💡Elementary Charge
💡Tesla
Highlights
A charged particle like a proton moving through space will continue in its direction unless acted upon by a force, such as a magnetic field.
The Earth acts as a large magnet, influencing charged particles like protons from the sun, causing them to spiral into the planet and create the Northern Lights.
Protons and electrons hitting the atmosphere cause ionization, which results in the display of colorful Northern Lights.
Magnetic forces are considered in three dimensions, which requires a different approach compared to two-dimensional scenarios.
Magnetic fields can be visualized using concentric circles, with the direction indicating whether the field is coming towards or going away from the observer.
A magnetic force (F sub n) is applied to a charged particle only when it has a velocity vector within a magnetic field.
The magnetic force is perpendicular to both the velocity of the charged particle and the magnetic field itself.
Calculating magnetic force involves the charge (Q), velocity vector, and the cross product with the magnetic field.
The cross product results in a third vector that lies in a different dimension, which can be visualized with an animation.
The right-hand rule is used to determine the direction of the magnetic force acting on a charged particle.
The force on a stationary charged particle in a magnetic field is zero, as there is no velocity to interact with the field.
The magnetic force can be calculated using the equation q(v × B), where q is the charge, v is the velocity, and B is the magnetic field.
The sine of the angle (theta) between the velocity and the magnetic field determines the magnitude of the magnetic force.
The magnetic force is maximized when the angle between the velocity and the magnetic field is 90 degrees, and it is zero at 0 or 180 degrees.
The elementary charge (q) is a necessary component in the calculation of the magnetic force on a charged particle.
An example problem demonstrates the calculation of the magnetic force on a proton moving perpendicular to a magnetic field.
Understanding the mathematical routines and applying them to express the force on a moving charged particle in a magnetic field is crucial for solving physics problems.
The right-hand rule is emphasized as a reliable method for determining the direction of the magnetic force in various scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: