9. Magnetism II
TLDRThe video script offers an in-depth exploration of magnetism, focusing on how objects react to and produce magnetic fields. It begins by explaining the force of magnetism on a moving charge in relation to the magnetic field, denoted by B, and introduces the concept of the cross product to determine the force vector. The script then delves into the technical definition of the cross product and its calculation using the right-hand rule. It proceeds to discuss the unit of magnetic field strength, the Tesla, and its physical meaning in terms of force experienced by a charge moving through a magnetic field. The professor illustrates the computation of magnetic forces on a current-carrying wire segment in a magnetic field, highlighting the cancellation of forces on a loop in a uniform magnetic field and the resulting net torque that tends to align the loop with the field. The concept of magnetic dipole moment is introduced, and the potential energy associated with the orientation of the dipole in a magnetic field is derived. The script further explains the behavior of a magnetic dipole in a magnetic field, comparing it to an electric dipole, and touches on the non-existence of magnetic monopoles. It then presents a practical application in the form of an electric motor, demonstrating how a current loop can be made to rotate within a magnetic field, and the challenges associated with creating a continuous motion. The discussion then shifts to the production of magnetic fields by electric currents, emphasizing the difference between steady currents and individual moving charges. The Biot-Savart law is introduced to calculate the magnetic field produced by a small segment of a current-carrying wire. The script explores the magnetic field of a circular loop and its similarity to that of a bar magnet, leading to the atomic theory explanation of magnetism in solid materials. Finally, the professor covers Ampere's law, which relates the line integral of the magnetic field around a closed loop to the current enclosed by the loop. The law is analogous to Gauss's law for electric fields and is applicable to magnetostatics, providing a powerful tool for calculating magnetic fields in various scenarios. The script concludes with examples of applying Ampere's law to find the magnetic field around an infinite wire and a solid cable carrying current, drawing parallels to similar problems in electrostatics.
Takeaways
- 🧲 The force of magnetism on a charge depends on its velocity and the magnetic field, denoted by B, and is given by the cross product v x B.
- ⚙️ The direction of the cross product can be determined using the right-hand rule, which is a common technique for calculating vector products.
- 📡 The unit for measuring magnetic field strength is the Tesla, which is defined based on the force experienced by a charge moving perpendicular to the field.
- 🔌 For a current-carrying wire segment dl, the force dF due to a magnetic field B is given by dl x B, and this force is perpendicular to both the segment and the field.
- 🏋️♂️ A current loop in a magnetic field experiences no net force but does experience a torque, which tends to align the loop with the field.
- ⚖️ The torque on a current-carrying loop is given by μ x B, where μ is the magnetic moment (area vector times current), and this torque tends to align the loop with the magnetic field.
- 🔁 The principle of the electric motor is based on the torque experienced by a current-carrying loop in a magnetic field, which can cause motion if the current is alternating.
- 🔋 The magnetic field produced by a small segment of current can be calculated using the Biot-Savart law, which involves a cross product and depends on the geometry of the current segment and the point in space where the field is measured.
- ⭕ The magnetic field at the center of a circular loop is μ_0I/2R, where μ_0 is the permeability of free space, I is the current, and R is the radius of the loop.
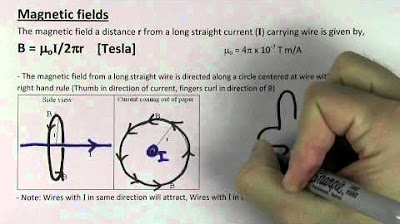
- 🧵 The magnetic field due to an infinite straight wire is μ_0I/2πa, where a is the distance from the wire, and the field is azimuthal around the wire.
- 🔗 Ampere's law states that the line integral of the magnetic field around a closed loop is equal to μ_0 times the total current enclosed by the loop, which is analogous to Gauss's law for electric fields.
Q & A
What are the two fundamental aspects of magnetism?
-The two fundamental aspects of magnetism are how objects react to a magnetic field and how objects produce a magnetic field.
How does the force of magnetism on a moving charge relate to its velocity and the magnetic field?
-The force of magnetism on a moving charge depends on its velocity (it must be moving to experience the force) and the magnetic field denoted by B. The force is given by the cross product of the velocity vector and the magnetic field vector.
What is the right-hand rule for determining the direction of a cross product?
-The right-hand rule for cross products states that if you point your right hand such that the fingers point in the direction of the first vector and then curl them towards the second vector, your thumb will point in the direction of the resulting vector from the cross product.
What is the unit of measurement for magnetic field strength?
-The unit of measurement for magnetic field strength is the Tesla.
How is the force experienced by a small segment of a current-carrying wire in a magnetic field described?
-The force experienced by a small segment of a current-carrying wire in a magnetic field is described as dF = I(dl x B), where I is the current, dl is the infinitesimal segment of the wire, and B is the magnetic field.
What is the relationship between the force on a straight wire segment and a curved wire segment in a uniform magnetic field?
-In a uniform magnetic field, the force on a curved wire segment between two points is the same as the force on a straight wire segment joining those two points.
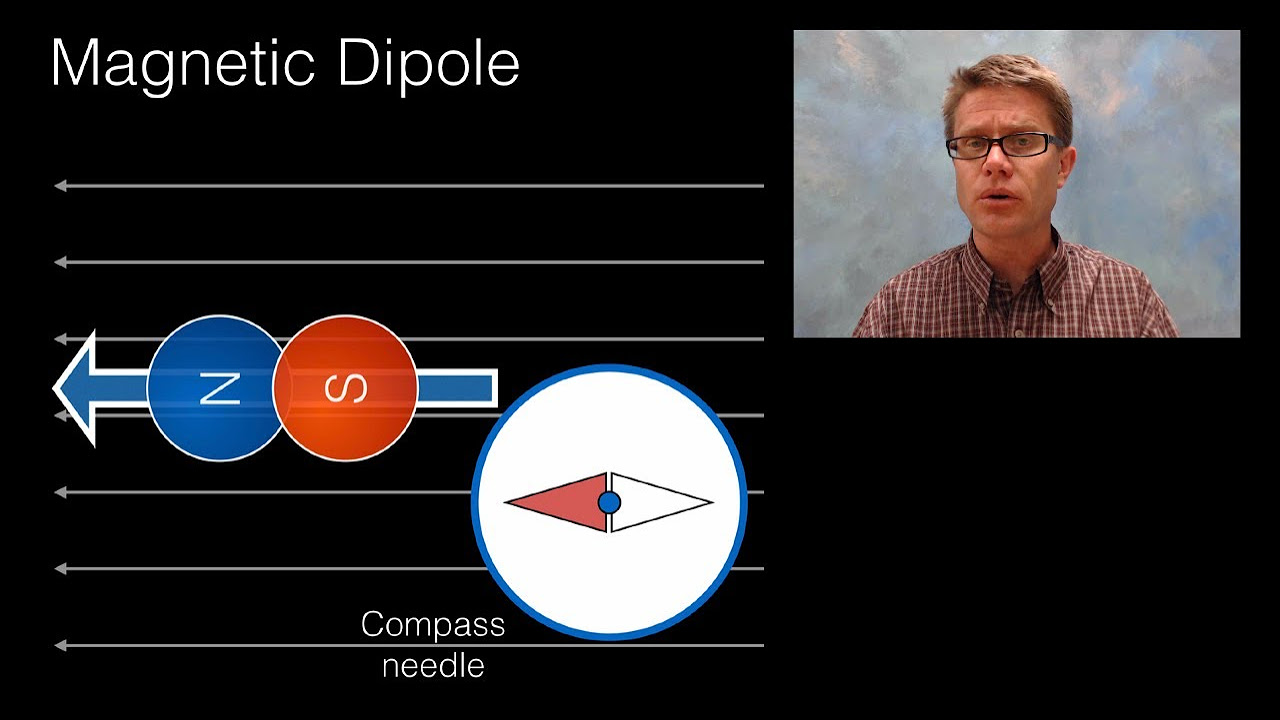
How does a magnetic dipole, like a current loop, align itself in a magnetic field?
-A magnetic dipole, such as a current loop, aligns itself with the magnetic field so that the most amount of magnetic field lines go through it, either in a parallel or anti-parallel configuration.
What is the potential energy of a magnetic dipole in a magnetic field?
-The potential energy of a magnetic dipole in a magnetic field is given by -μ⋅B, where μ is the magnetic moment of the dipole and B is the magnetic field.
How does the presence of thermal agitation affect the alignment of atomic magnetic moments in a material?
-Thermal agitation causes atomic magnetic moments to jiggle and point in random directions, preventing them from lining up and producing a net magnetic moment. When cooled, they can line up, and the material becomes magnetic.
What is the basic principle behind the construction of an electric motor?
-The basic principle behind an electric motor is the use of a current-carrying loop in a magnetic field, which experiences a force causing it to rotate. By continuously reversing the current, the loop can be made to rotate continuously.
What is the law of Biot and Savart, and how is it used to calculate the magnetic field produced by a small segment of current?
-The law of Biot and Savart states that the magnetic field dB produced by a small segment of current I times dl at a point separated by a vector r − r' is given by (μ_0/4Π) * I * dl x e_rr` / (r − r')^2, where μ_0 is a constant and e_rr` is the unit vector from r' to r.
Outlines
🧲 Fundamentals of Magnetism and Magnetic Force
The first paragraph introduces the concept of magnetism, dividing it into two parts: the reaction of objects to a magnetic field and the production of a magnetic field. It explains that the force on a moving charge in a magnetic field depends on its velocity and the magnetic field denoted by B, and is given by the cross product of these vectors. The paragraph also discusses the right-hand rule for determining the direction of the cross product and the unit of magnetic field strength, the Tesla. It concludes with an example of calculating the force on a segment of a current-carrying wire in a magnetic field.
🔍 Calculating Magnetic Force on a Current-Carrying Wire
The second paragraph delves into the calculation of the magnetic force on a segment of a current-carrying wire, using the cross product. It explains the contribution of each segment to the total force and introduces an ancient trick for understanding angles between vectors. The paragraph further illustrates how to calculate the force on a semi-circular wire loop in a magnetic field, emphasizing that the net force on the loop is zero due to symmetry, but there is a net torque that tends to align the loop with the magnetic field.
🗜️ Torque on a Magnetic Dipole
The third paragraph discusses the torque experienced by a magnetic dipole, such as a current loop, in a magnetic field. It explains that the torque tends to align the dipole with the magnetic field and that the potential energy of the system is related to the cosine of the angle between the magnetic moment and the magnetic field. The paragraph also highlights the concept of magnetic dipoles and their alignment with the magnetic field, drawing parallels with electric dipoles.
🔧 Constructing an Electric Motor Based on Magnetic Dipole Principles
The fourth paragraph explores the application of magnetic dipole principles to construct an electric motor. It describes the challenge of keeping the motor running by manually reversing the current direction or switching the magnetic field. The paragraph introduces the concept of a split-ring commutator that automatically reverses the current as the coil spins, allowing the motor to run continuously. It also touches on the moment of inertia and the oscillation of the motor past equilibrium due to this inertia.
⚙️ The Law of Biot and Savart and Magnetic Field Calculations
The fifth paragraph introduces the Law of Biot and Savart, which describes the magnetic field produced by a small segment of current-carrying wire. It explains the formula for calculating the magnetic field, dB, due to an infinitesimally small segment of current, including the factors involved in the calculation. The paragraph also discusses the complexity of the formula due to the presence of multiple vectors and the necessity of using cross products.
📐 Magnetic Field of a Circular Loop and Dipole Moment
The sixth paragraph focuses on calculating the magnetic field at a point on the axis of a circular current loop. It explains the symmetry of the problem and how to use the Law of Biot and Savart to find the magnetic field at the center and at a distance from the loop. The paragraph also discusses the concept of the magnetic dipole moment and how it relates to the magnetic field produced by the loop.
🪜 Magnetic Fields and Atomic Theory
The seventh paragraph ties the concept of magnetic fields to atomic theory, explaining how the magnetic properties of materials arise from the motion of electrons within atoms. It discusses how thermal agitation can disrupt the alignment of atomic magnetic moments and how cooling can lead to a more magnetic material. The paragraph also explains the phenomenon of magnetism in materials beyond the Curie temperature and the concept of a self-consistent solution in which the magnetic dipoles align to produce a stable magnetic field.
🧵 Magnetic Field of an Infinite Wire
The eighth paragraph addresses the calculation of the magnetic field around an infinite wire carrying a current. It uses Ampere's law to determine the magnetic field's dependence on distance from the wire and explains the physical interpretation of the attraction between a moving charge and the wire. The paragraph also discusses the force between two parallel currents and how they attract when parallel and repel when anti-parallel.
🔗 Ampere's Law and Its Applications
The ninth paragraph introduces Ampere's law, which relates the line integral of the magnetic field around a closed loop to the current passing through the loop. It emphasizes the similarities between Ampere's law and Gauss's law for electric fields, particularly in how the line integral is independent of the loop's size or shape. The paragraph also discusses the implications of Ampere's law for calculating magnetic fields in various scenarios, including the field around a solid cable carrying a current.
🛡️ Magnetic Field Inside and Outside a Current-Carrying Cylinder
The tenth paragraph applies Ampere's law to find the magnetic field inside and outside a cylindrical cable carrying a current. It explains how the magnetic field varies with distance from the cable's center, growing linearly inside the cable and decaying as 1/r outside the cable. The paragraph also highlights the analogy between this behavior and the electric field of a charged sphere, as per Gauss's law.
🔬 Miscellaneous Applications of Ampere's Law
The eleventh paragraph briefly mentions that there are a couple of other miscellaneous applications of Ampere's law that will be covered in the next session. It implies that the current discussion is part of a broader exploration of magnetostatics and the integral form of Maxwell's equations.
Mindmap
Keywords
💡Magnetism
💡Magnetic Field
💡Cross Product
💡Right-Hand Rule
💡Tesla
💡Magnetic Dipole
💡Electric Motor
💡Ampere's Law
💡Magnetic Moment
💡Biot-Savart Law
💡Magnetic Materials
Highlights
Magnetism involves two fundamental aspects: the reaction of objects to a magnetic field and the production of a magnetic field by objects.
The force exerted by magnetism on a moving charge is determined by its velocity and the magnetic field, often calculated using the cross product.
The direction of the cross product can be determined using the right-hand rule, which is a common technique in computing cross products.
The unit of magnetic field strength, Tesla, is defined such that a 1 coulomb charge moving at 1 meter per second in a 1 Tesla field experiences a 1 Newton force.
For a current-carrying wire segment, the force exerted by a magnetic field is calculated as the cross product of the segment and the magnetic field.
The force on a curved wire segment between two points is equivalent to the force on a straight segment connecting those points in a uniform magnetic field.
A current-carrying loop in a magnetic field experiences no net force but does experience a net torque, attempting to align the loop with the field.
The torque experienced by a magnetic dipole, such as a current loop, can be calculated using the cross product of the magnetic moment and the magnetic field.
The potential energy of a magnetic dipole in a magnetic field is given by -μ⋅B, indicating the loop tends to align for minimum energy.
A magnetic dipole, unlike an electric dipole, does not have isolated magnetic charges but behaves as if it does when interacting with a magnetic field.
The concept of a magnetic dipole is central to understanding how electric motors function, as they can convert magnetic torque into mechanical motion.
The invention of the electric motor involves a clever mechanical arrangement that allows continuous operation by automatically reversing the current.
The law of Biot and Savart provides the mathematical relationship for the magnetic field produced by a small segment of current in a loop.
The magnetic field produced by a tiny segment of current can be calculated by integrating the contributions from all parts of the current loop.
Ampere's law states that the line integral of the magnetic field around a closed loop is equal to the product of μ_0 and the total current enclosed by the loop.
The surface integral of the magnetic field over any closed surface is zero, as magnetic fields do not originate or terminate at any point.
The integral form of Maxwell's equations provides a relationship between electric and magnetic fields through line and surface integrals, which is crucial for solving problems in magnetostatics.
The magnetic field around an infinite wire can be determined using Ampere's law without performing complex integrals, due to symmetry considerations.
For a solid cable carrying current, the magnetic field outside the cable decays as 1/r, and inside, it grows linearly with the distance from the center of the cable.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: