Physics 15 Torque Example 6 (6 of 7) The Hungry Bear on a Beam; Tension in the Cable

Michel van Biezen
7 Apr 201309:07
EducationalLearning
32 Likes 10 Comments

TLDRIn this intriguing video, the host presents a physics problem involving a bear, a beam, and a picnic basket. The scenario is set with a beam held up by a cable that can withstand a maximum tension of 200 pounds. A 160-pound bear is trying to reach a 20-pound picnic basket, and a 50-pound beam is involved. The beam is 20 feet long, and the challenge is to determine if the bear can reach the basket before the cable breaks. The problem is approached as a torque issue, balancing the forces acting on the pivot point where the beam is attached to the pole. The host calculates the distances and torques due to the basket, the beam's weight, the bear's weight, and the cable's tension. After setting up the equation and substituting the known values, the host solves for the distance 'X' the bear can be away from the pole. The calculation reveals that the bear can reach the basket from 16 feet away, indicating that it's within reach before the cable breaks. This engaging problem illustrates the application of torque principles in a fun and relatable context.

Takeaways
  • 📐 The problem involves a beam supported by a cable with a maximum tension of 200 pounds.
  • 🐻 A 160-pound bear is trying to reach a picnic basket weighing 20 pounds, suspended from the beam.
  • 🌉 The beam is 20 feet long and the forces acting on it include the weight of the bear, the basket, and the beam itself.
  • ⚖️ The sum of all torques around the pivot point (where the beam is attached to the pole) must equal zero for equilibrium.
  • 🔢 The distances from the pivot point to the line of action of the forces are crucial for calculating torques.
  • 📏 Distances D1, D2, and D3 are used to calculate the torques from the picnic basket, the beam's weight, and the bear's weight, respectively.
  • 🧮 Torque contributions are calculated as the product of the force (weight) and the perpendicular distance from the pivot point.
  • 🔄 Clockwise torque is considered positive, and counterclockwise torque is negative, based on the chosen convention.
  • 💡 The equation for X (the distance from the pole to the bear) is derived by isolating the term with X in the torque equation.
  • 🚫 If X is less than 20 feet, the bear cannot reach the picnic basket before the cable breaks.
  • 📐 The distance D3 is calculated using trigonometry, specifically the sine of the 60-degree angle in the triangle formed by the beam, cable, and the pole.
  • 🧮 The final calculation involves substituting known weights and distances into the equation to solve for X, which turns out to be 16 feet.
Q & A

  • What is the maximum tension the cable can withstand?

    -The maximum tension the cable can withstand is 200 pounds.

  • What is the weight of the bear in the problem?

    -The bear weighs 160 pounds.

  • What is the weight of the picnic basket?

    -The picnic basket weighs 20 pounds.

  • How long is the beam?

    -The beam is 20 feet long.

  • What is the pivot point in the torque problem?

    -The pivot point is where the beam is attached to the pole.

  • What is the definition of clockwise and counterclockwise torque in the context of the problem?

    -Clockwise torque is considered positive and counterclockwise torque is considered negative in the problem.

  • What is the formula for calculating the distance from the pivot point to the line of action of the force?

    -The formula is the perpendicular distance from the pivot point to the line of action of the force.

  • What is the role of the tension in the cable in the torque equation?

    -The tension in the cable acts in the opposite direction to the weight of the bear and the picnic basket, creating a negative torque.

  • How is the angle theta related to the distance d3 in the triangle?

    -The distance d3 is equal to the length of the beam times the sine of angle theta.

  • What is the value of sine of 60 degrees?

    -The sine of 60 degrees is equal to the square root of 3 over 2, or approximately 0.866.

  • Can the bear reach the picnic basket before the cable breaks based on the calculations?

    -No, the bear cannot reach the picnic basket before the cable breaks, as the maximum distance it can be away from the pole is 16 feet, and the beam is 20 feet long.

  • What is the final outcome for the bear in the problem?

    -The bear is out of luck and will not be able to reach the goodies in the picnic basket before the cable breaks and the whole system collapses.

Outlines
00:00
🤔 Torque Problem: The Hungry Bear Scenario

The first paragraph introduces a physics problem involving a beam, a cable, a bear, a beam, and a picnic basket. The scenario is set where a 160-pound bear attempts to reach a 20-pound picnic basket attached to the other end of a 50-pound, 20-foot long beam. The cable holding the system has a maximum tension of 200 pounds. The problem is approached by calculating torques around a pivot point, which is the point where the beam is attached to a pole. The forces considered are the weight of the picnic basket, the weight of the beam at its center, the weight of the bear at a variable distance from the pole (X), and the tension in the cable. The paragraph concludes with setting up an equation to solve for X, the distance the bear can be from the pole without the cable breaking.

05:01
📐 Solving for X: The Bear's Reach

The second paragraph delves into solving the torque equation derived in the first paragraph. It begins by substituting known values into the equation, including the weight of the picnic basket (20 pounds), half the length of the beam (10 feet), and the maximum tension in the cable (200 pounds). The paragraph also introduces a trigonometric function to calculate the distance from the pivot point to the line of action of the cable's tension (d3), which is found using the sine of a 60-degree angle, given the cable's angle in relation to the beam. After substituting all known values and performing the calculation, the result is that the bear can be 16 feet away from the pole before the cable breaks. This implies that the bear, being only 4 feet away from the basket, will be unable to reach it before the system collapses.

Mindmap
Keywords
💡Torque
Torque, in the context of the video, refers to the rotational force that can cause an object to rotate around an axis. It is a key concept in the problem-solving process described, as the sum of all torques around a pivot point must equal zero for the system to be in equilibrium. In the script, torque is calculated for different forces acting on the beam to determine if the bear can reach the picnic basket before the cable breaks.
💡Tension
Tension is the force experienced by a cable or a string when it is pulled tight by opposing forces. In the video, the maximum tension the cable can withstand is given as 200 pounds, which is a critical parameter in calculating whether the system will hold or fail under the applied forces, including the weight of the bear and the beam.
💡Pivot Point
The pivot point is the point around which a body or object rotates. In the video, the pivot point is where the beam is attached to the pole, and it is crucial for calculating the torques acting on the system. The pivot point is the reference for determining the direction and magnitude of the torques.
💡Beam
The beam in the video is a horizontal structure that spans a distance and supports the weight of the bear and the picnic basket. It is 20 feet long and weighs 50 pounds. The beam's position and weight are essential in the torque calculation, as they contribute to the overall stability of the system.
💡Picnic Basket
The picnic basket represents the goal the bear is trying to reach. It hangs from the beam and has a mass of 20 pounds. The force exerted by the picnic basket due to gravity is a factor in the torque equation, influencing the balance of the system.
💡Bear
The bear is the main character in the problem, weighing 160 pounds. Its weight and the distance from the pivot point (denoted as X in the script) are critical in determining if the bear can reach the picnic basket without causing the cable to break.
💡Distance
Distance, as used in the script, refers to the perpendicular distance from the line of action of a force to the pivot point. It is a crucial factor in calculating torque. Different distances (D1, D2, D3) are used for the picnic basket, the beam, and the cable, respectively, in the torque equation.
💡Counterclockwise and Clockwise
These terms describe the direction of rotation. In the video, clockwise torque is considered positive, and counterclockwise torque is considered negative. This convention is important for setting up the torque equation correctly and understanding the balance of forces on the beam.
💡Equilibrium
Equilibrium refers to a state where all forces and torques are balanced, resulting in no net movement. The video's problem is about finding the conditions for equilibrium, specifically whether the bear can reach the picnic basket without the cable breaking, which would indicate a loss of equilibrium.
💡Triangle and Trigonometry
The script uses a triangle to determine the distance D3, which is the perpendicular distance from the pivot point to the cable's line of action. Trigonometry, specifically the sine function and a 60-degree angle, is used to calculate D3. This geometric and trigonometric analysis is integral to solving for the unknown distance X.
💡Maximum Tension
The maximum tension is the greatest amount of force that the cable can withstand before breaking, which is given as 200 pounds in the script. This value is used in the torque equation to find the limit of how much weight the system can support before failing.
Highlights

The problem involves a beam, a cable, a bear, a beam, and a picnic basket, with the goal to determine if the bear can reach the basket before the cable breaks.

The maximum tension the cable can withstand is 200 pounds.

The bear weighs 160 pounds, the beam weighs 50 pounds, and the picnic basket weighs 20 pounds.

The beam is 20 feet long.

A torque problem is formulated, where the sum of all torques about a pivot point equals zero.

The pivot point is identified as the point where the beam is attached to the pole.

Torque contributions are calculated for the picnic basket, the beam's weight, the bear's weight, and the cable's tension.

The perpendicular distances from the forces to the pivot point are defined as distances D1, D2, and D3.

Clockwise torque is considered positive and counterclockwise torque is negative by definition.

The equation is set up to solve for the distance X, where the bear is located.

If X is at least 20 feet, the bear can reach the basket; if less, the bear cannot reach before the cable breaks.

A triangle is used to determine the value of D3, involving the length of the beam and a 60-degree angle.

The sine of the 60-degree angle is used to calculate D3 as L times the sine of theta.

All terms in the equation have an L (length of the beam), which is then factored out.

The weight of the bear (160 pounds) is included in the equation, with a negative sign.

The numerical values are plugged in, including the weights of the basket, beam, and bear, and the maximum tension.

The sine of 60 degrees (approximately 0.866) is used in the calculation.

The final calculation shows that the bear can reach the basket from a distance of 16 feet, indicating it can get the goodies before the cable breaks.

Transcripts

Browse More Related Video

Physics 15 Torque Example 3 (3 of 7) Mass on Rod and Cable

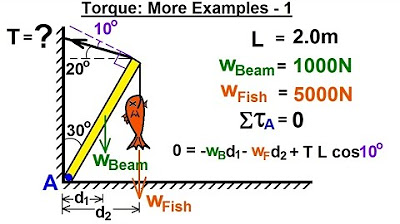
Physics 15 Torque (19 of 25) More Examples: 1 T=? of Cable Holding Fish

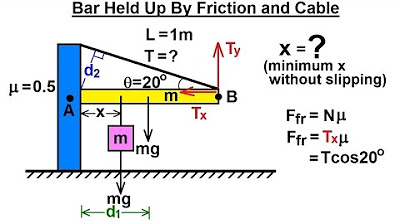
Physics 15 Torque (4 of 27) Bar Held Up by Friction

Physics 15 Torque (3 of 27) Tension=? in the Cable

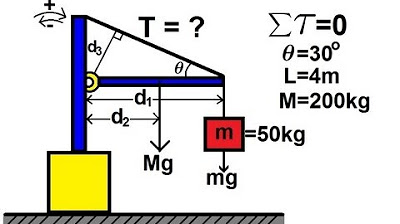
Physics 15 Torque Example 1 (1 of 7) Mass on Rod and Cable

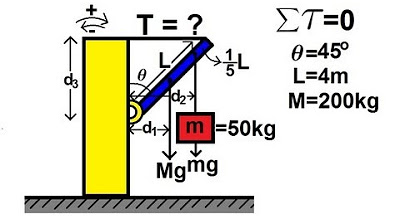
Physics 15 Torque Example 2 (2 of 7) Mass on Rod and Cable

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
PhysicsTorqueBalanceProblem SolvingEngineeringBear ProblemReal-world ApplicationEducationalSTEMFun MathMechanics