Infinite Series Multiple Choice Practice for Calc BC (Part 7)

turksvids

14 Apr 202212:13

EducationalLearning

32 Likes 10 Comments

TLDRThe video script presents a comprehensive review of various series and their convergence/divergence using mathematical reasoning. It covers topics such as infinite series, geometric series, ratio test, alternating series, and more. The presenter navigates through problems, discussing strategies, and providing step-by-step solutions, offering insights into series convergence. This engaging educational content aims to assist viewers in understanding and tackling similar mathematical challenges effectively.

Takeaways

😀 The video is focused on solving multiple-choice questions related to series in mathematics.
🤔 The series discussed include those involving alternating terms, convergence tests, and geometric series.
📚 Concepts such as the limit comparison test, alternating series error bounds, and ratio test are applied.
💡 The speaker provides insights into problem-solving strategies, such as identifying dominant terms and applying convergence tests.
🧠 Rationalization and understanding the behavior of series terms are emphasized for effective analysis.
🔍 The script demonstrates the application of various convergence tests, including the limit comparison test and alternating series test.
📝 Solutions involve calculating partial sums, determining convergence or divergence, and finding error bounds.
🎯 Clear explanations are given for the application of geometric series formulas and the ratio test.
📈 The importance of understanding series properties and utilizing relevant formulas is highlighted for problem-solving.
✅ The script concludes with a summary of the solved problems and encourages viewers to apply the learned techniques.

Q & A

What is the behavior of the series where a sub k equals negative one to the power of k for k equals zero and onward?
-The series diverges because it alternates between 1 and -1, and the limit does not approach zero. It is a well-known non-convergent series.
What is the form of the sequence a sub k in the second problem, and what does it simplify to?
-The sequence a sub k is given as the square root of k plus 1 minus k, which simplifies to 1 over the square root of k plus 1 plus the square root of k.
How does the limit as k approaches infinity of the second series help in determining its convergence?
-The limit as k approaches infinity of the simplified form of a sub k is zero, which suggests that the series might converge. However, further analysis reveals that the series actually diverges due to the non-cancelling terms.
What is the relationship between sine and cosine that is used to solve the series involving sine squared of b to the power of n?
-The relationship used is the Pythagorean identity, which states that sine squared plus cosine squared equals 1. This allows us to express sine squared in terms of cosine.
How is the error bound for an alternating series with 15 terms calculated?
-The error bound is the magnitude of the first term left off, which is the 16th term in this case. It is calculated as 1 over 2 times 16 plus 1, which simplifies to 1 over 33.
What is the ratio test, and how is it applied to determine the convergence of a series?
-The ratio test involves taking the limit of the ratio of consecutive terms of a series as n approaches infinity. If the limit is less than 1, the series converges; if it's greater than 1, the series diverges; if it equals 1, the test is inconclusive.
How does the limit comparison test work, and what is the dominant term in the series n squared over n cubed plus one?
-The limit comparison test involves comparing the limit of a series to the limit of a known series as n approaches infinity. The dominant term in the series n squared over n cubed plus one is 1 over n, as the plus one in the denominator becomes insignificant as n grows large.
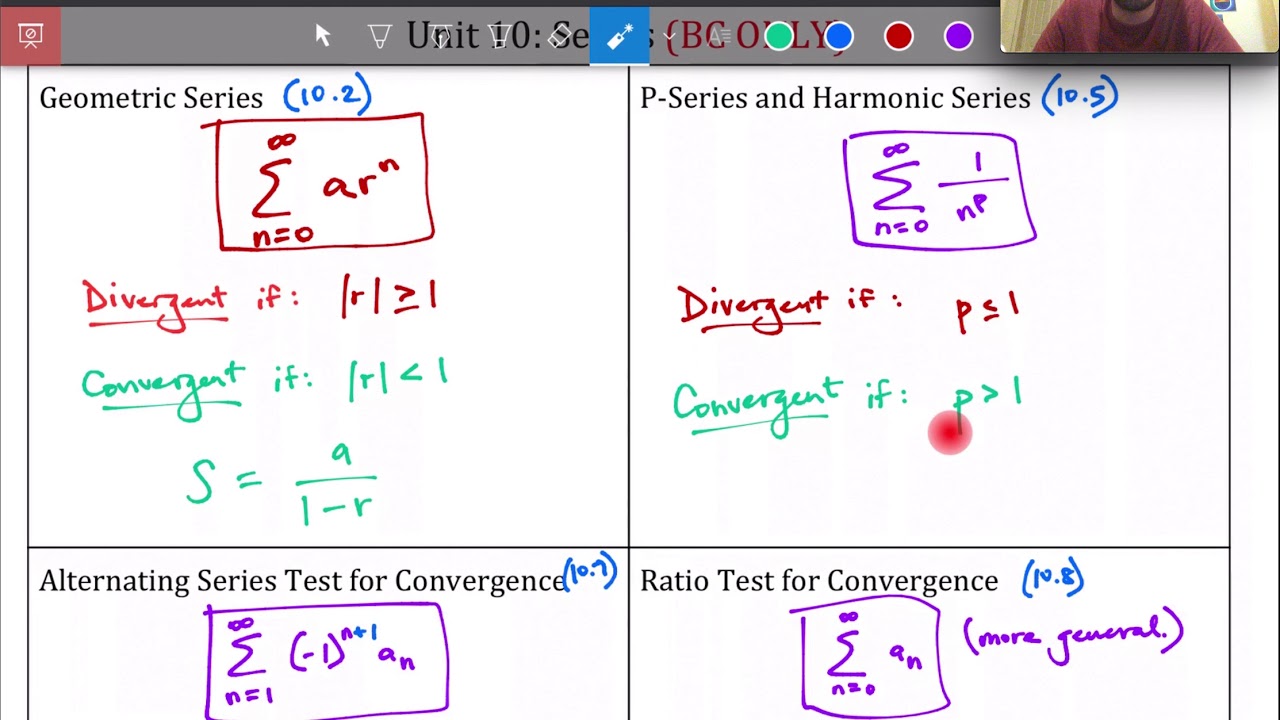
What is the sum of an infinite geometric series with the first term a and common ratio r, and what condition must r satisfy for the series to converge?
-The sum of an infinite geometric series is given by a over 1 minus r. For the series to converge, the common ratio r must be less than 1 in magnitude.
What is the condition for a series to be considered convergent?
-A series is considered convergent if the sum of its terms approaches a finite limit as the number of terms goes to infinity.
How does the partial sum of a series relate to the series sum, and what is its significance?
-The partial sum of a series is the sum of the first n terms of the series. The sum of the series is the limit of the partial sums as n approaches infinity. It signifies the total value to which the series converges if it does so.
What is the significance of the ratio test result in determining the convergence of the series e to the n over n factorial?
-The ratio test result, when the limit as n approaches infinity of the ratio of consecutive terms is less than 1, implies that the series converges. In this case, the limit is e over n plus 1, which is less than 1 for sufficiently large n, indicating convergence.
How does the series sum formula for a geometric series help in solving the problem involving 2 to the n plus 1 over 3 to the n?
-The formula for the sum of a geometric series allows us to find the sum of an infinite series with a common ratio. By rewriting the series to highlight the common ratio and applying the formula, we can easily calculate the sum as 4/3.

Outlines

00:00

😀 Series Divergence due to Alternating Terms

This paragraph discusses the convergence of infinite series by examining alternating terms. It begins by analyzing a series where each term alternates between -1 and 1, explaining that such a series diverges because its limit does not exist. Next, it considers a sequence with alternating terms and rationalizes it to simplify the expression. Through listing out terms and partial sums, it concludes that the series diverges as the limit of the partial sums tends to infinity.

05:00

😀 Convergence of Series: P-Series and Alternating Series Test

The paragraph focuses on determining the convergence of series, particularly considering p-series and alternating series tests. It evaluates three series options, highlighting that a p-series with p=2 converges, the harmonic series diverges, and an alternating series with decreasing terms and limit zero converges. Based on these evaluations, it deduces that the correct option is the one satisfying these convergence criteria.

10:03

😀 Application of Limit Comparison Test

This section deals with the application of the limit comparison test to determine the convergence or divergence of a given series. It simplifies the series expression by focusing on dominant terms and concludes that it can be compared with 1/n. Consequently, it selects the appropriate series that can be used with the limit comparison test, aiding in determining the convergence or divergence of the original series.

😀 Error Bound Calculation for Convergent Alternating Series

In this part, the focus is on computing the error bound for a convergent alternating series. It identifies the alternating series as convergent and proceeds to calculate the error bound by determining the magnitude of the first omitted term. Utilizing the properties of alternating series, it derives the error bound and selects the correct option corresponding to this calculation.

😀 Evaluation of Partial Sum Limits and Series Convergence

This paragraph addresses the convergence of a series by analyzing the limits of partial sums and series terms. It clarifies the relationship between partial sums and the sum of the series, emphasizing that the limit of series terms must approach zero for convergence. By evaluating different statements, it identifies the correct assertion regarding the limits of the series and partial sums, ensuring a deeper understanding of series convergence.

😀 Geometric Series Analysis and Summation

Focusing on geometric series analysis and summation, this part explores the sum of a geometric series involving exponential terms. It rewrites the series to highlight the common ratio, facilitating the application of the geometric series formula. Through careful calculation and application of the formula, it arrives at the final summation, providing a clear solution to the geometric series problem.

😀 Application of Ratio Test for Series Convergence

The paragraph demonstrates the application of the ratio test to determine the convergence of a series. It applies the test to the given series expression, simplifying terms and calculating the limit of the ratio. By analyzing the resulting inequality, it deduces the condition for series convergence and selects the appropriate option indicating convergence based on the ratio test.

😀 Evaluation of Geometric Series Summation

This section focuses on the summation of a geometric series by identifying the common ratio and utilizing the geometric series formula. It demonstrates the process of rewriting the series and applying the formula to compute the sum. Through meticulous calculation and consideration of the series' properties, it arrives at the final summation value, providing a comprehensive solution to the geometric series problem.

Mindmap

Keywords

💡Infinite Series

An infinite series is the sum of the terms in an infinite sequence. It is a fundamental concept in calculus and analysis. In the video, the concept is central to understanding the problems being solved, as the series either converges (sums to a finite value) or diverges (does not sum to a finite value). For example, the series '-1^k' is mentioned as a famous divergent series.

💡Convergence

Convergence in mathematics refers to the property of a sequence or series approaching a definite limit. In the context of the video, determining whether a series converges is a key step in solving the problems. For instance, the series involving 'a_sub_k' is discussed in terms of its convergence or divergence.

💡Divergence

Divergence is the opposite of convergence and describes a sequence or series that does not approach a limit. It is used in the video to describe series that do not sum to a finite value. An example from the script is the series '-1^k', which is stated to diverge because it alternates between -1 and 1 without approaching a limit.

💡Limit

In mathematics, a limit is a value that a function or sequence approaches as the input approaches some value. The concept of limits is essential in calculus. In the video, limits are used to determine the behavior of sequences and series as the number of terms goes to infinity, such as when evaluating 'a_sub_k' as 'k' approaches infinity.

💡Geometric Series

A geometric series is a series with terms that have a constant ratio between successive terms. It is a type of series for which a formula exists to find the sum of the series. In the video, a series involving '2^(n+1) / 3^n' is identified as a geometric series, and the sum is calculated using the formula for the sum of a geometric series.

💡Alternating Series Test

The alternating series test is a method used to determine the convergence of an alternating series, which is a series where the signs of the terms alternate. In the video, the test is mentioned when discussing the convergence of a series with alternating signs, and it is used to confirm that a series converges under certain conditions.

💡Ratio Test

The ratio test is a criterion used to determine the convergence or divergence of an infinite series. It involves comparing the ratio of consecutive terms as the series progresses. In the video, the ratio test is applied to a series involving 'e^n / n!' to determine if the series converges.

💡Partial Sum

A partial sum of a series is the sum of the first 'n' terms of the series. It is used to approximate the sum of an infinite series. In the video, the concept of partial sums is discussed in the context of series convergence, such as when calculating the sum of a series up to the 'kth' term.

💡Error Bound

In the context of series approximation, an error bound is a measure of the difference between the true sum of an infinite series and its partial sum. It is used to quantify the accuracy of the approximation. In the video, the error bound is discussed when approximating a series with 15 terms, and it is calculated using the magnitude of the first omitted term.

💡Harmonic Series

The harmonic series is the infinite series of the reciprocals of the natural numbers. It is an example of a divergent series. In the video, the harmonic series is mentioned as an example of a series that is famously divergent, which means it does not sum to a finite value.

💡p-Series

A p-series is a type of series of the form '1/n^p' where 'n' is a natural number and 'p' is a real number. The convergence of a p-series depends on the value of 'p'. In the video, a p-series with 'p=2' is mentioned and stated to converge because 'p' is greater than 1.

Highlights

The series of multiple-choice questions covers various topics in infinite series and sequences.

The speaker discusses the convergence/divergence of a series defined by a sub k = (-1)^k.

The speaker applies the method of listing out terms to analyze the convergence of a sequence.

The speaker uses the alternating series test to determine convergence.

The speaker simplifies a series using dominant terms to facilitate comparison.

The speaker calculates the alternating series error bound for a given number of terms.

The speaker clarifies the concept of the limit of partial sums in relation to the sum of the series.

The speaker identifies a common misconception regarding the limit of a sub n.

The speaker solves a problem involving the sum of sine squared terms using trigonometric relationships.

The speaker applies the ratio test to determine convergence of a series involving e to the n.

The speaker simplifies a geometric series to find its sum.

The series of videos aims to assist viewers in understanding and solving various types of problems related to infinite series and sequences.

Transcripts

Browse More Related Video

Infinite Series Multiple Choice Practice for Calc BC (Part 3)

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Calculus BC Unit 11 Recap

A Lot of Series Test Practice Problems

Unit 10 Study Guide - AP Calculus (BC Only)

AP Calc BC Series Review Multiple Choice Practice