Infinite Series Multiple Choice Practice for Calc BC (Part 3)
TLDRThis video script presents a detailed exploration of series convergence, focusing on the application of the integral test, ratio test, and alternating series test to determine the conditions under which various mathematical series converge or diverge. The speaker systematically approaches each problem, providing step-by-step reasoning and calculations to reach conclusions about the convergence of series involving functions such as 1/n^(2p), n*3^n/x, and others. The script also delves into conditional convergence, absolute convergence, and the intricacies of series with alternating signs. The speaker's methodical approach and clear explanations make complex mathematical concepts more accessible, offering viewers a comprehensive understanding of series analysis.
Takeaways
- 📚 The video discusses the convergence of integrals and series, specifically using the integral test to determine if a series converges.
- 🔍 For the series to converge, the exponent in the series must be greater than 1, which leads to the conclusion that p > 1/2.
- 🤔 The speaker initially doubts the convergence of a series with a sum involving n * (3^n)/x but uses the ratio test to find the conditions for convergence.
- 🏁 The series converges if the absolute value of x is greater than 3, which is an interesting result that contradicts the speaker's initial instinct.
- 🔢 The concept of conditional convergence is introduced, meaning a series converges when alternating but not when taking the absolute value.
- 🌟 The importance of testing for absolute convergence first is emphasized, as a series that converges absolutely will not be conditionally convergent.
- 📉 The nth term test is used to determine divergence, showing that a series that does not converge absolutely cannot be conditionally convergent.
- 🔄 The video also covers geometric series, noting that all convergent geometric series are absolutely convergent and not conditionally convergent.
- 🧐 The speaker struggles with a problem involving a series with a complex form and suggests that understanding the underlying p-series is crucial.
- 📊 A unique problem involving the sum of an alternating series and its bounds is solved, demonstrating a method for approximating the sum of a series.
- ✅ The alternating series test is confirmed as a valid method for determining the convergence of certain series, provided the terms alternate, decrease in magnitude, and have a limit of zero.
Q & A
What is the integral test used for in the context of series?
-The integral test is used to determine the convergence of an infinite series. If the integral of the series' terms from 1 to infinity converges, then the series itself also converges.
What is the condition for a series to be convergent based on the p-series test?
-A p-series, which is a series of the form 1/n^p, converges if the exponent p is greater than 1.
What does the ratio test involve when analyzing the convergence of a series?
-The ratio test involves comparing the ratio of consecutive terms of a series as n approaches infinity. If the limit of this ratio is less than 1, the series converges.
What is the condition for a series to be absolutely convergent?
-A series is absolutely convergent if the series formed by the absolute values of its terms converges.
What is the definition of a conditionally convergent series?
-A series is conditionally convergent if it converges when it alternates in sign but does not converge when the absolute values of its terms are considered.
How does the geometric series test relate to absolute convergence?
-The geometric series test involves examining the absolute value of the common ratio r. If the series converges, it is absolutely convergent because the test inherently checks for absolute convergence.
What is the conclusion about the series that is the sum from one to infinity of n times 3^n over x?
-The series converges if the absolute value of x is greater than three. This is determined by setting the ratio of the (n+1)th term to the nth term to less than 1 and solving for x.
What is the conclusion for the series involving sine of n over n squared?
-The series converges absolutely because the absolute value of sine is between zero and one, and since 1/n^2 converges, the product of the two also converges.
How does the alternating series test determine the convergence of a series?
-The alternating series test states that a series converges if its terms alternate in sign, decrease in magnitude, and the limit of the nth term is zero.
What is the conclusion for the series involving negative one to the power of (n+1) over the square root of n?
-The series is conditionally convergent because the absolute value of the terms forms a divergent p-series with p equal to 1/2, but the original series converges by the alternating series test.
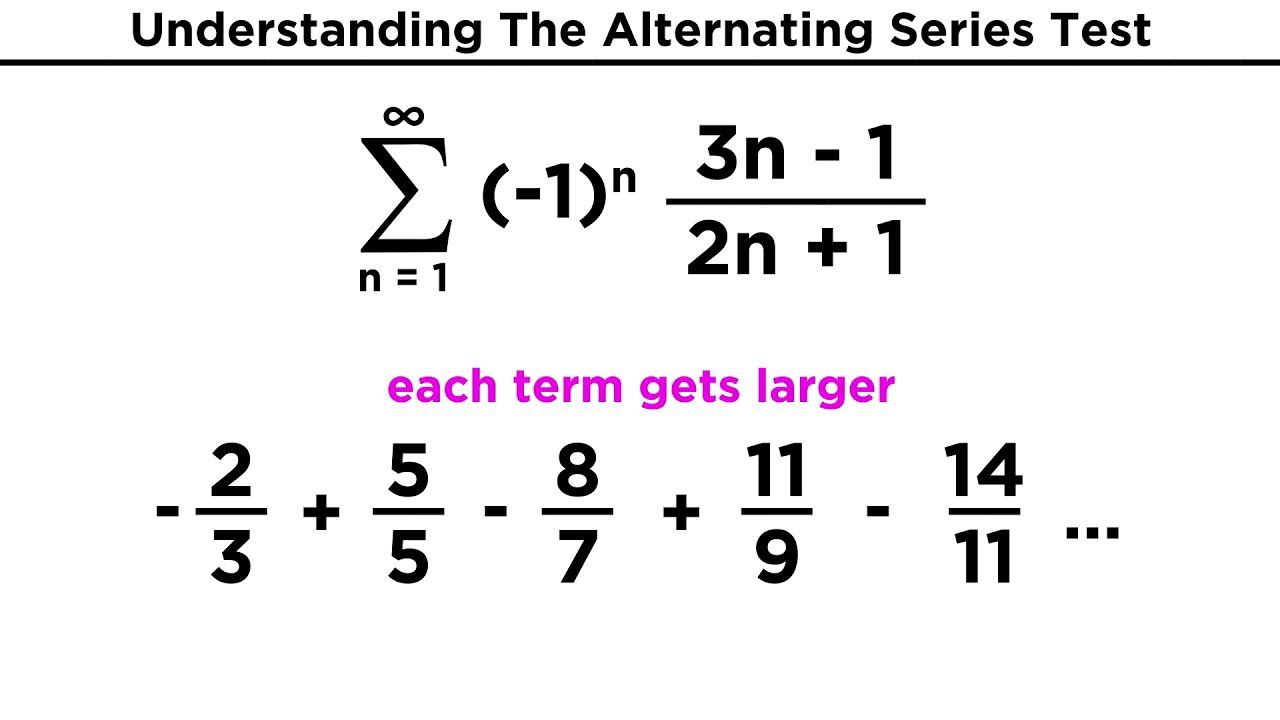
What is the sum of the series from zero to infinity of negative one to the power of n over (2n + 1)?
-The sum of the series is between two-thirds and one. This is determined by estimating the sum with the first few terms and considering the error introduced by each subsequent term.
Outlines
📚 Series Convergence and Divergence Analysis
The video focuses on exploring the convergence and divergence of various mathematical series. It begins with the integral test for series convergence, specifically examining the sum of 1/n^(2p) and determining the values of p for which the series converges. The presenter then tackles a series involving the sum of n*(3^n)/x and uses the ratio test to find the values of x that lead to convergence. The video also discusses conditionally convergent series, absolute convergence, and the application of the alternating series test to determine if a series is conditionally convergent based on whether it converges when alternating and diverges when taking the absolute value.
🔢 Dealing with Geometric and Alternating Series
The video script delves into geometric series, emphasizing their absolute convergence. It also addresses a series involving negative one to the power of n times n over n to the power of p plus two, and through rewriting and analysis, determines the values of p for which the series is conditionally convergent. The presenter further investigates a series with terms involving the cube root of n and ascertains its conditional convergence based on the alternating series test. The video also revisits a previously discussed series and confirms its absolute convergence due to the nature of p-series with p greater than one.
🤔 Summation and Error Estimation in Series
The presenter introduces a novel problem involving the series from zero to infinity of negative one to the power of n over 2n plus one. By examining the pattern of the series and estimating the sum, it is concluded that the series converges and its sum lies between two-thirds and one. This section also includes a discussion on the alternating series test, its requirements, and its applicability to various series, ultimately determining which series pass the test and which do not.
📉 Analyzing Functions and Series Using Graphs
The video concludes with a complex problem involving the function f(x) and its derivative, related to the graph of another function g(x). The presenter defines a series based on the function f and uses the properties of g to determine whether the alternating series test can be applied. After analyzing the behavior of the derivative of f and its relation to g, it is decided that the terms of the series are not decreasing in magnitude, and thus the alternating series test cannot be used to determine convergence.
Mindmap
Keywords
💡Integral Test
💡Series Convergence
💡Ratio Test
💡Conditionally Convergent
💡Geometric Series
💡Alternating Series Test
💡nth Term Test
💡Absolute Convergence
💡P-Series
💡Limit of a Function
💡Error Estimation
Highlights
The video discusses the convergence of an integral series using the reverse integral test, converting it into a series problem to determine the values of 'p' for which the series converges.
The series 1/n^(2p) is shown to converge if 'p' is greater than 1/2, using the integral test.
The sum of n * 3^n / x is explored, with the conclusion that the series converges if the absolute value of x is greater than three.
The concept of conditionally convergent series is introduced, where a series converges when alternating but not when taking the absolute value.
The sine of n over n squared series is shown to converge absolutely, not conditionally, as the absolute value of sine is between zero and one.
A series involving (-1)^(n+1) * n / √n is proven to be conditionally convergent by the alternating series test, as the absolute value diverges but the series itself converges when alternating.
Geometric series are discussed, noting that if they converge, they do so absolutely, not conditionally.
For the series involving (-1)^n * n / (n^p + 2), it is shown that the series converges absolutely if p > 1, and conditionally if p is between 1 and 2.
The series (-1)^n / (∛n) is analyzed and found to be conditionally convergent because its absolute value diverges, but the series itself converges when alternating.
The series from 1 to infinity of (-1)^n / (∛n) is shown to converge conditionally, and (-1)^n / n^3 is shown to converge absolutely due to the p-series test.
An alternating series from 0 to infinity of (-1)^n / (2n + 1) is explored, with the sum determined to be between two-thirds and one.
The alternating series test is applied to several series, with only the first series (-1)^(n+1) / n^2 passing the test for convergence.
The series involving sine functions is shown not to be alternating due to inconsistent signs and thus failing the alternating series test.
A series with terms involving √2 is analyzed, and it is determined that the terms do not decrease in magnitude, failing the alternating series test.
The function f(x) = 2 + cos(x)/x^2 and its derivative are used to analyze the convergence of a related series, concluding that the series does not meet the criteria for the alternating series test due to non-decreasing magnitude.
The video concludes with a reminder that even complex or challenging problems must be addressed and solved step by step.
Transcripts
Browse More Related Video

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test
Alternating Series, Types of Convergence, and the Ratio Test

Infinite Series Multiple Choice Practice for Calc BC (Part 7)

A Lot of Series Test Practice Problems

Conditional and Absolute Convergence for Infinite Series
AP Calculus BC Lesson 10.7
5.0 / 5 (0 votes)
Thanks for rating: