Calculus BC Unit 11 Recap
TLDRThis video offers a comprehensive review of Calculus BC's series of constants, covering infinite series notation, convergence tests including the divergence test, geometric series, and telescoping series. It delves into various convergence tests like direct comparison, integral test, p-series, and limit comparison. The presenter also discusses alternating series, absolute and conditional convergence, and the ratio test. The video is packed with examples and offers links for further study, aiming to prepare viewers for AP Calculus exams.
Takeaways
- 📚 The video covers a review of Calculus BC Unit 11, focusing on series of constants, including Taylor and Maclaurin series.
- 🔍 The instructor begins with the concept of an infinite series, explaining series notation and convergence definitions.
- 📉 The Divergence Test, also known as the nth term test, is highlighted as a method to determine if a series diverges by checking if the limit of its terms is not zero.
- 📈 Geometric series are discussed, with a focus on their recognition and the condition for convergence when the absolute value of the common ratio is less than one.
- 🔢 Examples are provided to illustrate the calculation of the sum of a geometric series, emphasizing the formula involving the first term and the reciprocal of one minus the common ratio.
- 📝 The script mentions various convergence tests such as the Direct Comparison Test, Integral Test, and p-Series Test, which are crucial for determining the convergence of different types of series.
- 🔄 The Limit Comparison Test is introduced for series that are not exactly p-series but are similar, allowing for comparison with known convergent or divergent series.
- 🔁 The video discusses Alternating Series, including the Alternating Series Test for convergence and the difference between conditional and absolute convergence.
- 📊 The Ratio Test is explained as a method to determine the interval of convergence for power series, with examples of when to apply it and its limitations.
- ⚠️ The importance of understanding the difference between conditional and absolute convergence is emphasized, especially in the context of the Alternating Harmonic Series.
- 📚 The script concludes by summarizing all the tests for convergence discussed in the video, preparing viewers for AP Calculus exams.
Q & A
What is the definition of an infinite series?
-An infinite series is the sum of the terms of a sequence that goes on indefinitely. In the script, it is mentioned that a series converges if the limit of the partial sums exists, which is the sum of a finite number of terms added together.
What is the divergence test also known as?
-The divergence test is also known as the nth term test. It states that if the limit of the terms of a series is not zero as n approaches infinity, then the series must diverge.
What is a geometric series and when does it converge?
-A geometric series is a series where each term after the first is found by multiplying the previous one by a constant called the common ratio (r). It converges when the absolute value of r is less than one.
How is the sum of a geometric series calculated if it converges?
-If a geometric series converges, its sum can be calculated using the formula: first term multiplied by (1 / (1 - r)), where r is the common ratio.
What is the direct comparison test for series convergence?
-The direct comparison test states that if the terms of a series are larger than the terms of a known divergent series, then the series in question must diverge. Conversely, if the terms are smaller than those of a known convergent series, then it must converge.
What is the integral test for series convergence?
-The integral test states that if a function is continuous, positive, and decreasing, and its improper integral from 1 to infinity converges, then the related series also converges. If the integral diverges, the series does as well.
What is a p-series and when does it converge?
-A p-series is a series of the form 1/n^p. It converges when p is greater than 1 and diverges when p is less than or equal to 1.
What is the limit comparison test used for?
-The limit comparison test is used to compare two series of positive terms. If the limit of their ratio is positive and finite, then the series have the same convergence or divergence behavior.
What is an alternating series and how is it tested for convergence?
-An alternating series is a series where the signs of the terms alternate between positive and negative. The alternating series test states that if the terms are decreasing in magnitude and the limit of the terms is zero, the series converges.
What is the difference between conditional and absolute convergence for an alternating series?
-A series is conditionally convergent if it converges but the series of absolute values of its terms diverges. It is absolutely convergent if the series of absolute values of its terms also converges.
What is the error bound for an alternating series approximation?
-For an alternating series approximation, the error is no more than the absolute value of the next term after the last term used in the approximation.
What is the ratio test and how is it used to determine the convergence of a series?
-The ratio test involves taking the limit of the ratio of successive terms (or the absolute value of the ratio) of a series. If the limit (l) is less than one, the series converges absolutely. If l is greater than one, the series diverges. If l equals one, the test is inconclusive.
How does the ratio test help determine the interval of convergence for a power series?
-The ratio test can be used to find the values of x for which a power series converges. It helps in determining the radius of convergence and the interval of convergence for the series.
Outlines
📚 Introduction to Series of Constants in Calculus BC
This paragraph introduces the video's focus on the series of constants in the Calculus BC curriculum. It outlines the topics covered in the class, including the concept of infinite series, series notation, convergence definitions, and the divergence test. The instructor also mentions geometric and telescoping series, highlighting that the latter is not part of the AP Calculus topic. Examples and links to further lessons for each topic are provided, setting the stage for a detailed exploration of series in the subsequent paragraphs.
🔍 Exploring Convergence Tests and Series Examples
The second paragraph delves into various tests for series convergence, such as the direct comparison test, integral test, and p-series test. It also touches on the harmonic series and limit comparison test. The instructor emphasizes the importance of recognizing series types and applying the appropriate tests. Additionally, the paragraph includes a brief example of a geometric series and how to determine its convergence, reinforcing the concept with a practical application.
📉 Understanding Alternating Series and Their Convergence
This paragraph discusses alternating series, characterized by their plus-minus pattern, and introduces the alternating series test for convergence. It differentiates between conditional and absolute convergence, providing the alternating harmonic series as an example of conditional convergence. The error bound concept is also introduced, explaining how the absolute value of the next term after a certain number of series terms can serve as an approximation error bound.
📘 Power Series and the Ratio Test for Convergence
The final paragraph covers the ratio test for series convergence, explaining its application for determining absolute divergence and conditional convergence. It also discusses the use of the ratio test in identifying the interval and radius of convergence for power series. The instructor clarifies that while the ratio test is a part of the curriculum, the focus of the unit's exam will be on Taylor and flowing series rather than the interval and radius of convergence. The paragraph concludes with a summary of the convergence tests and a thank you note for watching.
Mindmap
Keywords
💡Infinite Series
💡Convergence
💡Divergence Test
💡Geometric Series
💡Telescoping Series
💡Alternating Series
💡Ratio Test
💡Power Series
💡Radius of Convergence
💡Error Bound
💡Conditional Convergence
💡Absolute Convergence
Highlights
Introduction to the concept of infinite series and series notation.
Definition of convergence and the divergence test, also known as the nth term test.
Explanation of geometric series and the conditions for convergence.
Discussion on telescoping series and its exclusion from AP calculus topics.
Overview of tests for convergence including direct comparison, integral, and p-series tests.
Limit comparison test and its application for series with similar behaviors.
Introduction to alternating series, its test, and the concept of conditional and absolute convergence.
Explanation of the alternating harmonic series and its conditional convergence.
The ratio test for absolute convergence and its application in power series.
Understanding the interval and radius of convergence for power series.
Clarification on the difference between conditional and absolute convergence.
Error bound for alternating series and its calculation based on the next term.
Practice problems and resources for further understanding of series topics.
Emphasis on the importance of the ratio test in determining the interval of convergence for power series.
Explanation of the behavior of series at the endpoints of the interval of convergence.
Insight into the implications of conditional convergence at the edges of the interval.
Conclusion summarizing all the tests for series convergence covered in the video.
Transcripts
Browse More Related Video

A Lot of Series Test Practice Problems

Calculus 2 - Geometric Series, P-Series, Ratio Test, Root Test, Alternating Series, Integral Test

Tests for Convergence
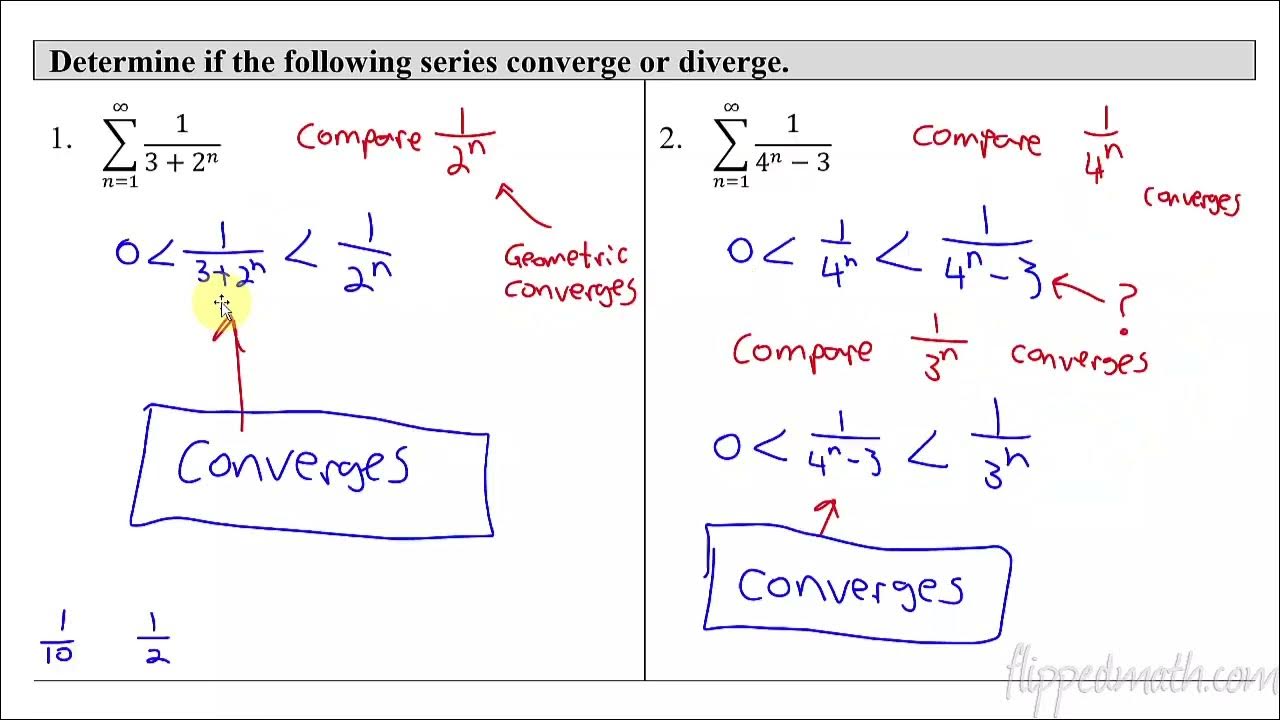
Calculus BC – 10.6 Comparison Tests for Convergence

Choosing Which Convergence Test to Apply to 8 Series
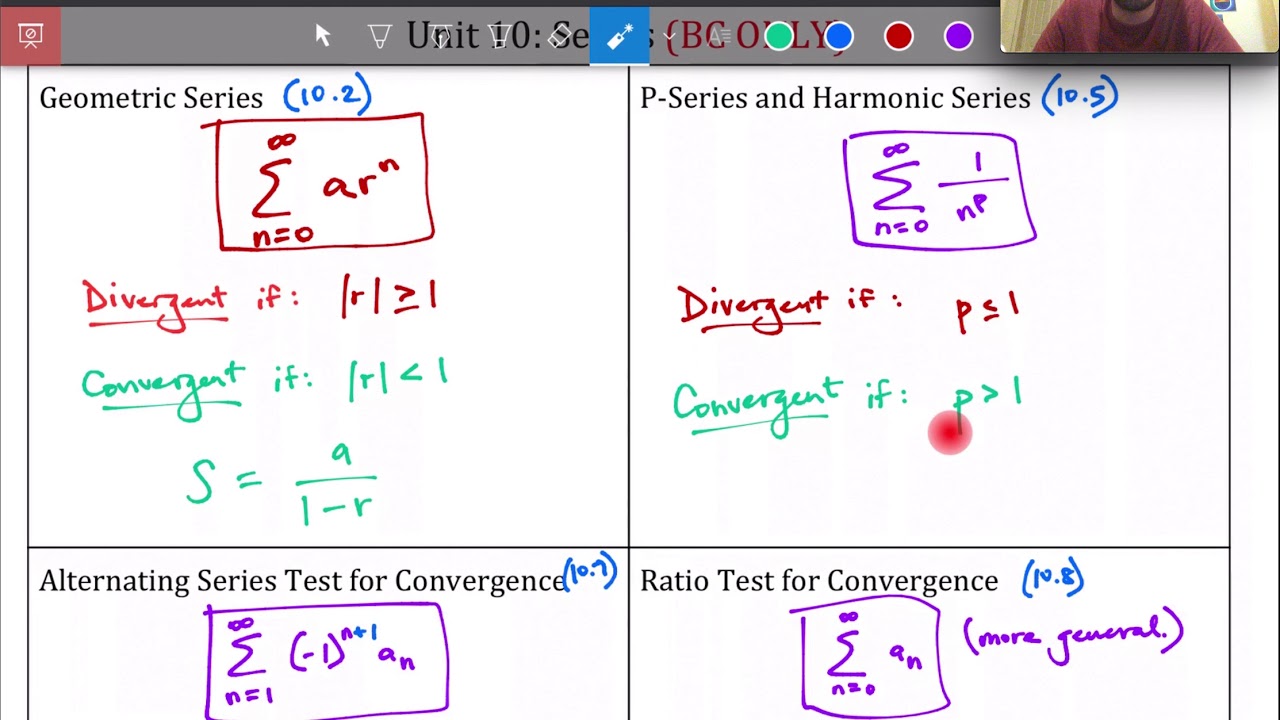
Unit 10 Study Guide - AP Calculus (BC Only)
5.0 / 5 (0 votes)
Thanks for rating: