AP Calculus BC - 10 Minute Recap

Allen Tsao The STEM Coach

6 May 202209:27

EducationalLearning

32 Likes 10 Comments

TLDRThe provided transcript is a comprehensive review of calculus topics typically covered in a BC Calculus course. It emphasizes the importance of understanding parametric and vector equations, including the concepts of position, velocity, and acceleration as functions of a parameter 't'. The review also covers how to calculate the speed of an object using the magnitude of the velocity vector and the application of the chain rule to find the slope of a tangent line. Additionally, the transcript delves into polar equations, explaining the conversion between Cartesian coordinates and polar coordinates, and how to calculate the area enclosed by polar curves. The discussion on series includes the various tests for convergence, such as the integral, limit comparison, and ratio tests, as well as how to determine the interval of convergence and apply error bounds for Taylor and Maclaurin series. The summary also touches on Euler's method for estimating solutions to differential equations, the logistic growth model, and improper integrals. This review serves as a valuable resource for students preparing for BC Calculus exams, providing a structured approach to mastering key calculus concepts.

Takeaways

📌 Remember that in parametric equations, x and y are functions of a parameter t, which is crucial for finding position, velocity, and acceleration.
📐 Use the Pythagorean theorem to find the magnitude of the velocity vector, which is essential for understanding the speed of an object in motion.
🔍 For finding the slope of a tangent line in parametric equations, apply a version of the chain rule: dy/dx = (dy/dt) / (dx/dt).
🛤️ Understand that arc length in parametric form is related to the integral of speed, which is key for calculating distance traveled along a path.
🌐 Know the conversion equations between Cartesian coordinates and polar coordinates, such as x = r*cos(θ) and y = r*sin(θ).
🔵 For polar equations, the area under a curve is found by integrating one half r squared dθ, which is a fundamental concept in polar coordinate systems.
🔍 Finding the bounds for integrals in polar equations often involves finding intersection points of curves, which can be a challenging part of the process.
📊 Series tests are a lengthy topic; ensure you understand how to perform all the tests, including the conditions for the integral test.
🔢 In Calculus BC, the root test is not required, but you should be familiar with the limit comparison and direct comparison tests, as well as the ratio test for series convergence.
🔄 Taylor and Maclaurin series are important; know the general form for a Taylor series and how to calculate error bounds for both alternating and non-alternating series.
📈 Euler's method and the logistic growth model are also topics to be aware of, as they are part of the broader calculus curriculum.
⚠️ Lastly, be familiar with improper integrals and other miscellaneous topics that, while minor, are still part of the AP Calculus BC syllabus.

Q & A

What are the key concepts in parametric equations that students should remember?
-Students should remember that x and y coordinates are functions of a parameter t. They should also understand that position is a function, velocity is the first derivative, acceleration is the second derivative, and the speed is found using the magnitude of the velocity vector through the Pythagorean theorem.
How is the slope of a tangent line found in parametric equations?
-The slope of a tangent line in parametric equations is found by using a version of the chain rule, where dy/dx is calculated as dy/dt divided by dx/dt.
What is the relationship between arc length and distance traveled in parametric equations?
-In parametric equations, arc length is considered the same as distance traveled along a path. It is calculated by integrating the speed, which is the magnitude of the velocity vector.
What are the conversion equations between rectangular and polar coordinates?
-The conversion equations are x = r * cos(θ) and y = r * sin(θ), with x^2 + y^2 = r^2 being another useful relationship.
How is the area under a polar curve calculated?
-The area under a polar curve is calculated by integrating one half r^2 dθ between the angle bounds a and b.
What is the general form of a Taylor series and how does it differ from a Maclaurin series?
-The general form of a Taylor series is f(x) = Σ [f^n(x0) / n!] * (x - x0)^n, where n goes from 0 to infinity. A Maclaurin series is a special case of a Taylor series where the center is at x0 = 0.
How do you determine the radius and interval of convergence for a series?
-The radius of convergence is often determined using the ratio test, setting it less than one to find the bounds for a power series. To determine whether the endpoints converge or diverge, those values are plugged into the series and specifically analyzed.
What are the two main error bounds used in series approximation, and when are they used?
-The two main error bounds are the Alternating Series Error Bound and the Lagrange Error Bound. The Alternating Series Error Bound is used for alternating series and is the absolute value of the term after the truncation point. The Lagrange Error Bound is used for non-alternating Taylor series and involves finding the maximum of the (n+1)th derivative over the interval from the center to the point of estimation.
What is Euler's method, and how is it applied?
-Euler's method is a numerical technique used to estimate the solution to a differential equation. It involves using the derivative of the function at a point and a step size to iteratively approximate the solution.
What is the logistic growth model, and how does it differ from other growth models?
-The logistic growth model is a mathematical model used to describe population growth in which the growth rate slows down as the population size increases. It differs from exponential growth models by including a carrying capacity that limits the population size.
What are the main series tests that students are expected to know for Calculus BC?
-The main series tests for Calculus BC include the nth-term test, the integral test, the ratio test, the root test, and the limit comparison test. Students should understand how to run these tests and the conditions for their use.
Why is it important to find intersection points when dealing with polar curves?
-Finding intersection points is important for determining the angle bounds when calculating areas between two curves or when the problem involves curves that are inside or outside of one another. It helps in setting up the correct integrals to solve the problem.

Outlines

00:00

📐 Parametric and Polar Equations in BC Calculus

This paragraph introduces the specific topics for BC Calculus, emphasizing parametric and vector equations. It explains the concept of x and y coordinates being functions of a parameter t, and how to calculate velocity and acceleration using derivatives. The importance of using the Pythagorean theorem to find the speed of an object and the chain rule to find the slope of a tangent line is highlighted. Arc length is discussed as a measure of distance traveled along a path, with two forms: parametric and non-parametric. The conversion between polar and Cartesian coordinates is covered, along with the calculation of area under a polar curve using the integral of one half r squared d theta. The paragraph also touches on finding intersection points of curves and the process of calculating the slope of a tangent line in polar coordinates.

05:01

🔍 Series Tests and Convergence in BC Calculus

The second paragraph delves into series tests, which are a significant part of BC Calculus. It advises understanding how to perform various tests, such as the integral test, limit comparison test, and direct comparison test, and to be aware of the conditions for each test. The integral test is noted for its requirement that the function be positive and decreasing. The concept of radius and interval of convergence is explained, including the use of the ratio test to determine the bounds for a power series. The method for checking the convergence or divergence of endpoints is described, involving plugging in values and analyzing the endpoints. Taylor and Maclaurin series are introduced, with a focus on their general form and the importance of understanding error bounds, particularly the alternating series error bound and the Lagrange error bound. The paragraph concludes with a brief mention of Euler's method for estimating solutions to differential equations, the logistic growth model, improper integrals, and other miscellaneous topics.

Mindmap

Keywords

💡Parametric equations

Parametric equations are mathematical equations that express the coordinates of a point as functions of a third variable, known as the parameter. In the context of the video, they are used to describe the path of an object where the x and y coordinates are functions of a parameter t. An example from the script is when discussing position, velocity, and acceleration in terms of this parameter t.

💡Vector equations

Vector equations are used to represent the relationship between different vectors in a geometric or physical context. In the video, they are mentioned in conjunction with parametric equations to describe motion and are used to find quantities such as speed and the slope of a tangent line to a path described by parametric equations.

💡Polar equations

Polar equations are a way of representing points in a two-dimensional plane using a distance from a reference point (the pole) and an angle from a reference direction (the polar axis). The video discusses how to convert between polar and Cartesian coordinates using equations like r = sqrt(x^2 + y^2) and how to find areas and slopes using polar coordinates.

💡Chain rule

The chain rule is a fundamental theorem in calculus for finding the derivative of a composite function. In the video, it's used to find the slope of a tangent line to a curve described by parametric equations by applying the chain rule to the derivatives dy/dt and dx/dt.

💡Arc length

Arc length refers to the distance between two points along a curve. In the video, it is discussed in the context of parametric equations, where the arc length is calculated by integrating the speed along the path. It is also mentioned that arc length can be thought of as the distance traveled along a path.

💡Taylor series

A Taylor series is a representation of a function as an infinite sum of terms calculated from the values of its derivatives at a single point. The video explains that the Taylor series is used to approximate functions and involves understanding the error bounds associated with the approximation.

💡Error bounds

Error bounds in the context of Taylor series provide an estimate of the maximum error between the function and its approximation by the series. The video distinguishes between the alternating series error bound and the Lagrange error bound, explaining how they are calculated and when they are used.

💡Series test

A series test is a method used in calculus to determine the convergence or divergence of an infinite series. The video outlines several tests, such as the integral test, the ratio test, and the comparison tests, which are used to analyze the behavior of a series and determine its convergence properties.

💡Radius and interval of convergence

The radius and interval of convergence are parameters that describe the domain in which a power series converges. The video explains how to calculate these using the ratio test and how to determine if the endpoints of the interval converge or diverge by plugging them into the series.

💡Euler's method

Euler's method is a numerical technique used to estimate the solution of a differential equation. The video briefly mentions Euler's method as a topic that should be understood, likely in the context of approximating the solution to a differential equation over a small interval.

💡Logistic growth model

The logistic growth model is a mathematical model used to describe the growth of a population in an environment with limited resources. It is mentioned in the video as a topic within the broader scope of calculus BC that a student should be familiar with.

Highlights

Parametric and vector equations are key topics in BC calculus, often appearing in exam questions.

Understanding that x and y coordinates are functions of a parameter t is crucial for parametric equations.

Velocity and acceleration in parametric equations are derived from the first and second derivatives, respectively.

The magnitude of the velocity vector can be found using the Pythagorean theorem.

The slope of a tangent line in parametric equations is determined using a version of the chain rule.

Arc length is analogous to distance traveled along a path and can be calculated using integral calculus.

Polar equations involve parameterizing by an angle theta and converting between r, x, and y coordinates.

The area under a polar curve is found by integrating one half r squared d theta.

Finding the intersection points of polar curves is a common task, though not overly complex for BC calculus.

Series tests are a lengthy topic, requiring knowledge of various tests and their conditions.

The integral test for series convergence has specific conditions, such as the function being positive and decreasing.

Taylor and Maclaurin series are essential for approximating functions and calculating error bounds.

Euler's method is a technique for estimating solutions to differential equations.

The logistic growth model is a key concept in BC calculus, often related to real-world applications.

Improper integrals are a minor topic but still important for a comprehensive understanding of BC calculus.

Miscellaneous topics like Euler's method and logistic growth models should be understood in addition to the major FRQ topics.

The importance of mastering the major FRQ topics for success in BC calculus exams cannot be overstated.

Transcripts

Browse More Related Video

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 4)

AP Calculus BC Multiple Choice Practice Test (2016 AP CED Problems)

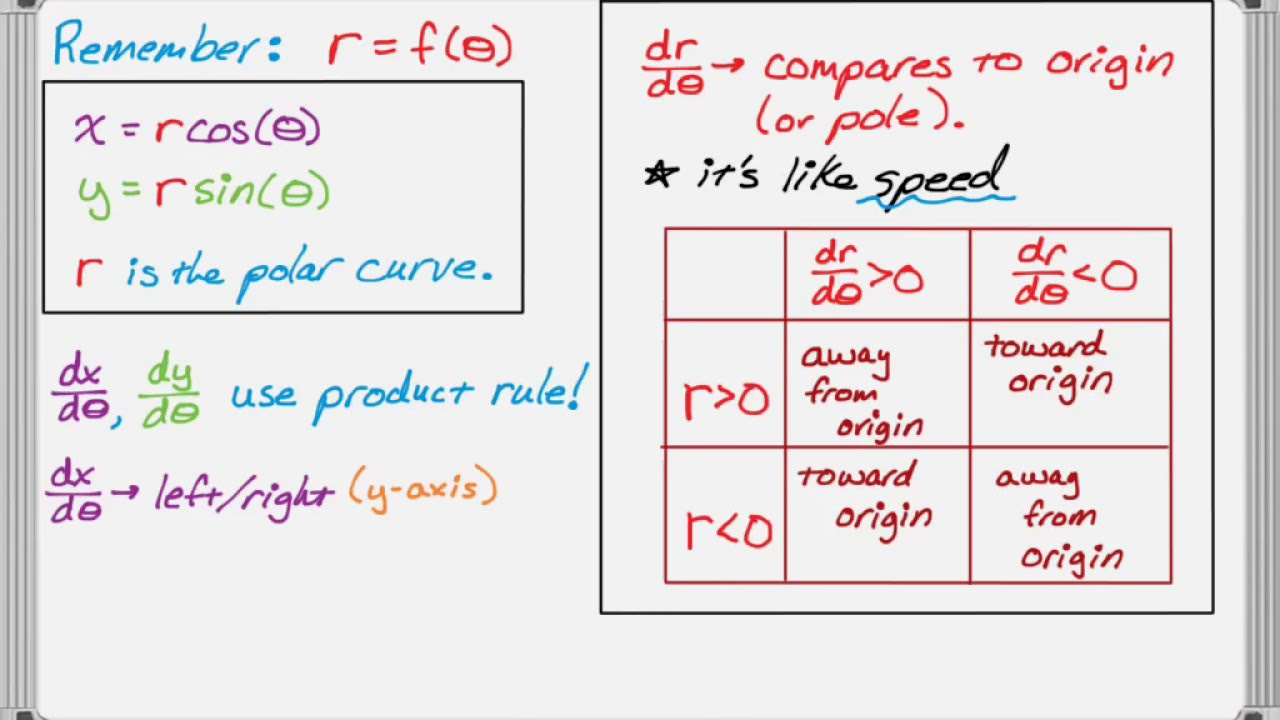
Calc BC Polar Review for AP Calculus BC Exam

AP Calculus BC Practice Exam 2012 - Multiple Choice questions 1-28

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 3)

AP Calculus BC 2003 Multiple Choice (no calculator) - questions 1 - 28

AP Calculus BC - 10 Minute Recap

Takeaways

Q & A

What are the key concepts in parametric equations that students should remember?

How is the slope of a tangent line found in parametric equations?

What is the relationship between arc length and distance traveled in parametric equations?

What are the conversion equations between rectangular and polar coordinates?

How is the area under a polar curve calculated?

What is the general form of a Taylor series and how does it differ from a Maclaurin series?

How do you determine the radius and interval of convergence for a series?

What are the two main error bounds used in series approximation, and when are they used?

What is Euler's method, and how is it applied?

What is the logistic growth model, and how does it differ from other growth models?

What are the main series tests that students are expected to know for Calculus BC?

Why is it important to find intersection points when dealing with polar curves?