Calc BC Polar Review for AP Calculus BC Exam

turksvids
10 Apr 201708:44
EducationalLearning
32 Likes 10 Comments

TLDRThis video offers a comprehensive review of polar coordinates for the BC calculus exam. It covers the basics, including converting polar to rectangular coordinates, understanding rates like dr/dθ, dx/dθ, and dy/dθ using the product rule, and interpreting their meanings. The video also explains how to find the equation of a tangent line, calculate polar area, and determine areas between polar curves. It emphasizes the importance of memorizing formulas and understanding the concepts, and provides tips on using symmetry and estimating answers for problem-solving.

Takeaways
  • 📚 Remember that in polar coordinates, \( R \) is a function of \( \theta \) and can be converted to rectangular coordinates using \( x = R \cdot \cos(\theta) \) and \( y = R \cdot \sin(\theta) \).
  • 🔍 Understand the derivatives in polar coordinates: \( \frac{dR}{d\theta} \), \( \frac{dX}{d\theta} \), \( \frac{dY}{d\theta} \), and their interpretations, especially the direction of movement relative to the origin or pole.
  • 📈 Memorize the relationship between the sign of \( R \) and \( \frac{dR}{d\theta} \) to determine whether you are moving towards or away from the origin.
  • 📉 Use the product rule to find \( \frac{dX}{d\theta} \) and \( \frac{dY}{d\theta} \), which are essential for interpreting movement in the polar coordinate system.
  • 📐 Know how to find the slope of the tangent line, \( \frac{dy}{dx} \), in polar coordinates by dividing \( \frac{dY}{d\theta} \) by \( \frac{dX}{d\theta} \).
  • 📏 Be aware that the equation of a tangent line in polar coordinates is expressed in terms of \( x \) and \( y \), which can involve the unit circle and lead to radical expressions.
  • 🔢 Memorize the formula for calculating polar area, which is \( \frac{1}{2} \int_{A}^{B} R^2 d\theta \), and remember the importance of the 1/2 factor and squaring \( R \).
  • 📐 For finding the area between two polar curves, subtract the area under the smaller curve from the larger one, using the integral \( \frac{1}{2} \int_{A}^{B} (R_{\text{large}}^2 - R_{\text{small}}^2) d\theta \).
  • 🔄 Utilize symmetry in polar curves to simplify calculations, as many polar curves exhibit symmetrical properties that can be leveraged.
  • 📝 When estimating the area of a region in polar coordinates, use rectangles or circles to get a sense of the magnitude of your answer.
  • 🔄 Be familiar with related rates in polar coordinates, such as \( \frac{dX}{dt} \), \( \frac{dY}{dt} \), and \( \frac{dR}{dt} \), and apply the chain rule when solving these problems.
Q & A

  • What is the primary focus of the video?

    -The video focuses on reviewing the key concepts needed for understanding polar coordinates on the BC calculus exam.

  • What is the relationship between R and theta in polar coordinates?

    -In polar coordinates, R is a function of theta, representing the radial distance from the origin to a point on the curve.

  • How do you convert polar coordinates to rectangular coordinates?

    -To convert from polar to rectangular coordinates, use the formulas x = R * cos(theta) and y = R * sin(theta), where R is the radial function and theta is the angle.

  • What does the derivative dR/dθ represent in polar coordinates?

    -The derivative dR/dθ represents the rate at which the radial distance R changes with respect to the angle theta.

  • How can you determine the direction of movement in polar coordinates using dR/dθ?

    -If R is greater than 0 and dR/dθ is greater than 0, the movement is away from the origin. If dR/dθ is less than 0, the movement is toward the origin. Opposite signs indicate movement toward the origin, while the same signs indicate movement away from the origin.

  • What is the significance of the product rule in finding dx/dθ and dy/dθ?

    -The product rule is used to find the derivatives dx/dθ and dy/dθ by plugging in the polar curve for R and differentiating with respect to theta.

  • How does the direction of movement in terms of x and y coordinates relate to dx/dθ and dy/dθ?

    -The sign and value of dx/dθ can indicate whether you are moving left or right, and your position relative to the y-axis. Similarly, dy/dθ indicates whether you are moving up or down, and your position relative to the x-axis.

  • What is the formula for finding the equation of a tangent line in polar coordinates?

    -The equation of a tangent line in polar coordinates is found by using the formula dy/dx = (dy/dθ) / (dx/dθ).

  • How is the area of a polar region calculated?

    -The area of a polar region is calculated by integrating 1/2 * R^2 with respect to theta from the starting angle A to the ending angle B.

  • What is the formula for finding the area between two polar curves?

    -The area between two polar curves is found by integrating 1/2 * (R_big^2 - R_small^2) with respect to theta from angle A to angle B, where R_big and R_small are the larger and smaller polar curves, respectively.

  • Why is it important to consider symmetry when calculating areas or volumes in polar coordinates?

    -Considering symmetry can simplify calculations by allowing you to find the area or volume of a part of the shape and then multiply by the number of symmetrical parts, such as petals in a rose curve.

  • What are related rates in the context of polar coordinates and how are they used?

    -Related rates in polar coordinates involve finding the rate of change of one quantity with respect to time given the rate of change of another, using the chain rule. For example, dx/dt = (dx/dθ) * (dθ/dt).

  • How can you estimate the reasonableness of your answer when calculating areas or volumes in polar coordinates?

    -You can estimate the reasonableness of your answer by circumscribing the region with rectangles or circles and comparing the calculated area or volume to this approximation.

Outlines
00:00
📚 Polar Coordinates Overview for BC Calculus

This paragraph introduces the video's purpose, which is to review key concepts for polar coordinates on the BC calculus exam. The speaker emphasizes that the 'R' in polar coordinates is a function of theta and that converting polar to rectangular coordinates is a common task, using the formulas x = R * cos(theta) and y = R * sin(theta). The importance of memorizing these formulas is stressed, as they are essential for solving problems. The paragraph sets the stage for a deeper dive into polar coordinate concepts.

05:01
🔍 Derivatives and Rates in Polar Coordinates

The second paragraph delves into the specifics of derivatives in polar coordinates, including DRD(theta), DX/D(theta), and DY/D(theta). It explains that DRD(theta) is found by taking the derivative of the polar curve with respect to theta, and its interpretation involves comparing the direction of movement from the origin based on the signs of R and DRD(theta). The paragraph also touches on the product rule for finding DX/D(theta) and DY/D(theta), and how these derivatives can indicate movement left or right, and up or down, respectively. The discussion on rates continues with dy/dx in polar coordinates, which is found by dividing DY/D(theta) by DX/D(theta), and the importance of understanding the tangent line's equation in terms of x and y.

Mindmap
Keywords
💡Polar Coordinates
Polar coordinates are a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. In the context of the video, polar coordinates are essential for understanding the calculus concepts discussed, such as converting between polar and rectangular coordinates using the formulas x = r * cos(θ) and y = r * sin(θ), where r is the radial distance and θ is the angle.
💡BC Calculus Exam
The BC Calculus Exam refers to the AP Calculus BC exam, which is a standardized test in the United States for high school students. The video is specifically tailored to review concepts necessary for this exam, indicating the level of mathematical sophistication expected and the relevance of the topics covered.
💡Polar Curve
A polar curve is a curve represented in polar coordinates where the radial distance r is a function of the angle θ. The video emphasizes that understanding polar curves is crucial for the exam, as they form the basis for many problems involving calculus in polar coordinates.
💡Rectangular Coordinates
Rectangular coordinates, also known as Cartesian coordinates, are a system where points on a plane are defined by ordered pairs (x, y). The video script mentions converting polar coordinates to rectangular coordinates, which is a common task in calculus problems involving polar functions.
💡Derivative
In calculus, a derivative represents the rate at which a function is changing at a given point. The video discusses the derivative with respect to θ, denoted as dr/dθ, which is a key concept for analyzing the behavior of polar curves, such as whether a point on the curve is moving towards or away from the origin.
💡Rates of Change
Rates of change, such as dr/dθ, dx/dθ, and dy/dθ, are discussed in the video as important for understanding the dynamics of polar curves. These rates help in determining the direction and speed of a point on the curve relative to the origin or the pole.
💡Product Rule
The product rule is a fundamental calculus theorem used to find the derivative of a product of two functions. In the context of the video, it is used to find the derivatives dx/dθ and dy/dθ by applying the rule to the polar curve function r(θ).
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. The video explains how to find the equation of a tangent line to a polar curve, which is an important concept for understanding the local behavior of the curve at a specific point.
💡Polar Area
Polar area refers to the area enclosed by a polar curve and is calculated using the integral of (1/2)r²dθ from θ = a to θ = b. The video script highlights the importance of remembering the factor of (1/2) and squaring the radial function when calculating areas in polar coordinates.
💡Area Between Polar Curves
The area between polar curves is the region enclosed by two polar curves, one inside the other. The video script explains how to calculate this area by finding the difference between the areas under the larger and smaller polar curves, which is akin to the method used for finding volumes of revolution in cylindrical shells.
💡Related Rates
Related rates problems involve finding the rate of change of one quantity given the rate of change of another related quantity. The video mentions how to handle such problems in the context of polar coordinates, using chain rule and the relationships between dx/dt, dy/dt, and dr/dθ.
💡Symmetry
Symmetry in polar coordinates often refers to the regular patterns or shapes that polar curves can exhibit, such as a rose curve with multiple petals. The video script suggests using symmetry to simplify calculations, for example, by calculating the area for one petal of a rose curve and then multiplying by the total number of petals.
💡Estimation
Estimation is the process of approximating a value or quantity. In the video, the speaker recommends using estimation techniques to verify the reasonableness of calculated areas or volumes, by comparing them to the areas of circumscribed rectangles or circles.
Highlights

Introduction to the main concepts needed for polar coordinates on the BC calculus exam.

Polar curve R is a function of theta, emphasizing the importance of understanding this relationship.

Conversion from polar to rectangular coordinates using x = R * cos(theta) and y = R * sin(theta).

Explanation of how to find specific x or y coordinates using the polar function R.

Memorization of key formulas for x, y coordinates in relation to theta is crucial for the exam.

Differentiation in polar coordinates: dR/dθ, dX/dθ, and dY/dθ, and their interpretations.

The concept of dr/dθ and its comparison to speed, with a table explaining its implications.

Finding dx/dθ and dy/dθ using the product rule and interpreting their meanings.

Understanding the movement relative to the axes based on the signs of dx/dθ and dy/dθ.

Calculating dy/dx in polar coordinates by dividing dy/dθ by dx/dθ.

Writing the equation of a tangent line in terms of x and y, despite the polar nature of the coordinates.

Calculating polar area using the integral of 1/2 * R^2 * dθ from theta A to theta B.

Common mistakes in calculating polar area, such as forgetting the 1/2 and squaring R.

Finding the area between two polar curves by integrating the difference of their squared functions.

The importance of using symmetry in polar curve problems to simplify calculations.

Estimating the reasonableness of an answer by circumscribing the region with rectangles or circles.

Related rates in polar coordinates, such as dx/dt and dy/dt, and their calculation using chain rule.

A list of free-response questions from 2007 onwards to practice polar coordinate problems.

Transcripts

Browse More Related Video

AP Calculus BC - 10 Minute Recap

AP Calculus BC Lesson 9.7

2022 Live Review 7 | AP Calculus BC | Working with Polar Equations

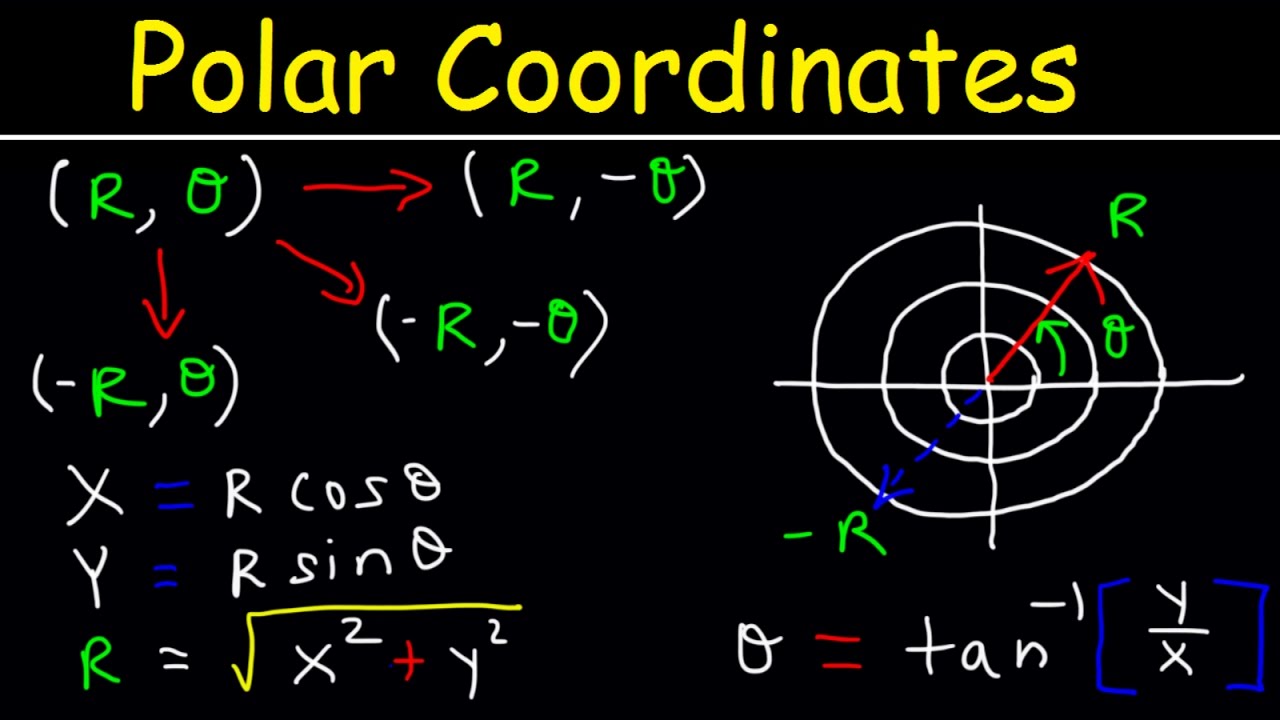
Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu

Polar, Parametric, Vector Multiple Choice Practice for Calc BC (Part 3)

Polar Curves

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polar CoordinatesBC CalculusConcept ReviewExam PrepMathematicsEducationalDerivativesIntegralsTangent LinesArea CalculationRelated Rates