2021 Live Review 7 | AP Calculus AB | Application of Integrals (Part 2)

Advanced Placement

28 Apr 202150:32

EducationalLearning

32 Likes 10 Comments

TLDRThe video transcript features an engaging educational session focused on calculus, specifically area and volume problems. The instructor begins with a review of previously covered material before diving into the day's topic. They tackle a variety of calculus problems, including finding the area of irregular shapes and calculating the volume of solids with rectangular cross-sections. The session emphasizes the importance of understanding calculus concepts and the ability to apply them to different scenarios, such as modeling the rate at which people leave a stadium post-game. The instructor also highlights the necessity of clear communication in mathematical problem-solving, ensuring that the steps and reasoning are well-articulated for the reader's comprehension. Throughout the session, the instructor encourages students to practice and provides guidance on how to approach multiple-choice and free-response questions effectively. The video concludes with a reminder for students to submit feedback and a teaser for the next session, which will include a review of additional problems and a final goodbye to the viewers.

Takeaways

📚 The importance of understanding area and volume calculations, which are highly tested topics in calculus.
∫ When finding volume, you integrate area, and when finding area, you integrate very thin cross-sections.
📈 The concept of using calculus to deal with irregular shapes where standard geometric formulas do not apply.
🔢 The necessity of performing anti-derivatives to find the area under curves, especially when dealing with functions like the square root of x.
📉 Utilizing symmetry and strategic cancellations in integrals to simplify calculations and understand the shape of the region.
📐 The method of setting up integrals for area and volume problems, including using the absolute value where appropriate.
🤔 The need for clear communication in mathematical problem-solving, ensuring that the steps and reasoning are well-explained.
📝 Annotation of work is crucial, especially when the work involves calculus operations like derivatives and integrals.
🕒 Time management is key, as students are encouraged to work slowly and methodically, particularly on AP exams.
📉 The application of calculus in real-world scenarios, such as modeling the rate at which people leave a stadium after an event.
📌 Attention to detail when setting up integrals, ensuring that the correct functions are used as the top and bottom of the integrand.

Q & A

What is the main topic discussed in the video?
-The main topic discussed in the video is the application of calculus to find areas and volumes, specifically focusing on integral calculus and its use in solving problems related to geometric shapes.
What is the first problem presented in the video?
-The first problem involves finding the area of a region bounded by the functions f(x) = √x and g(x) = -1/2x + 4, along with the x-axis (y=0).
What is the method used to calculate the area under a curve between two points?
-The method used to calculate the area under a curve between two points is integration. The definite integral of the top function minus the bottom function over the interval gives the signed area between the curves.
How does the presenter approach the problem of finding the volume of a solid with rectangular cross-sections?
-The presenter approaches the problem by considering the area of the rectangles formed by the cross-sections perpendicular to the x-axis. The volume is found by integrating the product of the height (given by the value of x) and the difference between the top and bottom functions over the given interval.
What is the significance of the feedback form mentioned in the video?
-The feedback form is a way for viewers to provide comments and suggestions about the content of the video. It helps the presenter to understand the audience's needs and to improve the quality of the educational material.
How does the presenter handle the calculation of the area when the functions switch positions?
-The presenter handles the calculation by considering the top and bottom functions relative to the interval being integrated. The integral is set up as the top function minus the bottom function, and the presenter emphasizes the importance of understanding which function is on top or bottom within each interval.
What is the strategy for setting up an integral to find the volume of a solid generated by revolving a region around a line?
-The strategy involves identifying the outer and inner radii of the cross-sections (washer method) or the single radius if it's a disc method. The integral is then set up as the integral of π times the outer radius squared minus the inner radius squared, with respect to the variable along the axis of revolution.
What is the importance of the axis of revolution in the context of finding the volume of a solid of revolution?
-The axis of revolution is crucial as it determines how the region is rotated to form the solid. It affects whether the cross-sections are circles (disc method) or have an inner and outer radius (washer method), which in turn affects the setup and calculation of the integral for the volume.
How does the presenter ensure that the work shown is sufficient for a full response question?
-The presenter ensures that the work is shown by clearly writing out the integral setup, including the limits of integration and the functions involved. They also emphasize the importance of showing each step of the calculation and providing the final numeric answer.
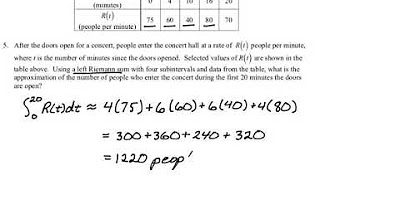
What is the purpose of the trapezoidal sum in the context of approximating an integral?
-The trapezoidal sum is used to approximate the value of a definite integral by averaging the areas of the trapezoids formed between the curve and the x-axis over sub-intervals of the integration domain.
How does the presenter approach the problem of finding the rate at which people are leaving the stadium after a game?
-The presenter uses the given data points to approximate the rate at which people are leaving the stadium by calculating the average rate over sub-intervals (trapezoidal sum) and then uses this information to determine the total number of people who have left by a certain time.

Outlines

00:00

😀 Introduction and Area Calculation Review

The speaker greets the audience and introduces the session, mentioning that they are nearing the end of a review session. The focus is on area and volume calculations, with a mention of a few typos corrected in the practice problems available for download. The first problem involves finding the area of a region bounded by two functions, which is approached using calculus. The speaker outlines the method for solving the problem, which involves integrating one function and adding the area of a triangle formed under the other function.

05:00

📚 No Calculator Multiple Choice Area Problems

The speaker moves on to no calculator multiple choice problems, focusing on area and volume. A problem involving the intersection of a function and a line is discussed, with the goal of finding the enclosed area. The speaker emphasizes the importance of identifying the top and bottom functions and the need for flexibility in approach depending on the shape. The concept of symmetry in area problems is highlighted, and the speaker also mentions the importance of simplifying expressions for clarity.

10:00

🔍 Solids of Revolution and Volume Calculation

The topic shifts to solids of revolution, where the speaker discusses how to calculate the volume of a solid with rectangular cross-sections formed by revolving a region around the x-axis. The importance of identifying the base and height of the rectangles in the integral is stressed. The speaker also touches on the difference between a washer and a disc when it comes to cross-sectional shapes and how this affects the calculation of volume.

15:02

🎨 Geometric Sketching and Volume of Revolution

The speaker attempts to sketch a geometric shape, which is a region bounded by two curves. The problem involves revolving this region around the line y=1 to create a solid object. The distinction between a washer and a disc is clarified, with the speaker deciding that the shape in question is a washer. The process of calculating the volume of this solid involves finding the outer and inner radii and integrating the difference of their squares over the given interval.

20:03

🧮 Final Area and Volume Calculations

The speaker continues with more complex area and volume problems, including a scenario where a region is the base of a solid and cross-sections perpendicular to the x-axis are isosceles right triangles. The calculation involves integrating the square of the difference between the functions defining the top and bottom of the region. The speaker also humorously discusses the need for a new camera and the challenges of teaching while juggling multiple tasks.

25:04

🏟️ Stadium Egress and Volunteer Entry Problem

The final part of the script involves a real-world problem set in a stadium. The scenario describes people leaving the stadium after a game and volunteers entering to clean up. The speaker uses a combination of integral calculus to approximate the number of people in the stadium at a specific time and derivative calculus to find the rate at which the number of people is changing at a particular time interval. The importance of units and clear communication of mathematical processes is emphasized.

30:06

📈 Recap and Final Thoughts

The speaker concludes the session with a recap of the key topics covered, emphasizing the importance of volume and area calculations in the context of calculus. Tips are given for effective communication in mathematical problem-solving, and the speaker encourages students to annotate their work clearly. The session ends with a reminder for viewers to fill out a feedback form and thanks the audience for their participation.

35:07

📝 Closing Remarks

The final paragraph is a brief closing with no substantial content, indicating the end of the video script.

Mindmap

Keywords

💡Calculus

Calculus is a branch of mathematics that deals with the study of change and motion, focusing on the concepts of limits, derivatives, integrals, and infinite series. In the video, calculus is used to find areas and volumes of irregular shapes, which is a core application of the subject. For example, the video discusses using calculus to calculate the area of a region bounded by two functions.

💡Area Under a Curve

The area under a curve, often referred to as the definite integral, is a fundamental concept in calculus that represents the accumulated value under the curve of a function from one point to another. In the video, the concept is used to find the area of regions bounded by curves, such as between a square root function and a line.

💡Volume of a Solid

The volume of a solid is a measure of the three-dimensional space a solid occupies. In the context of the video, the volume of a solid is calculated using integral calculus, specifically by integrating the area of cross-sections over a given interval. This is demonstrated when the video discusses finding the volume of a solid with rectangular cross-sections.

💡Integral

An integral in calculus represents the process of summing infinitesimal data points, which can be used to calculate the area under a curve or the volume of a solid. The video uses integrals to find areas and volumes, emphasizing the importance of setting up the integral correctly and evaluating it over the appropriate limits, as seen when calculating the area of a region bounded by the x-axis and two functions.

💡Derivative

A derivative in calculus measures how a function changes as its input changes. It is the antithesis of an integral and is used to find rates of change. In the video, the concept of derivatives is mentioned in the context of anti-differentiating functions to find their integrals, such as when finding the area under the square root of x.

💡Rate of Change

The rate of change refers to the derivative of a function at a certain point and indicates the instantaneous change in the function's value. In the video, the rate of change is discussed in the context of people leaving a stadium after a game, where the rate is given by a function of time, and the problem involves finding the rate at which the number of people is changing at a specific time.

💡Trapezoidal Sum

The trapezoidal sum is a method used to approximate the definite integral by summing the areas of trapezoids that approximate the shape under the curve of the function. In the video, this method is used to approximate the number of people who have left the stadium within an hour after the game ends.

💡Riemann Sum

A Riemann sum is a way to approximate the definite integral by dividing the area under the curve into rectangles or other shapes and summing their areas. The video briefly mentions the left-hand and right-hand Riemann sums, which are specific methods of approximating integrals by summing the areas of rectangles positioned to the left or right of the partition points of the function's domain.

💡Anti-Derivative

An anti-derivative, also known as an indefinite integral, is a function whose derivative is equal to the original function. In the video, finding the anti-derivative is a step in the process of evaluating definite integrals, such as when calculating the area under the curve of the square root of x.

💡Symmetry in Calculus

Symmetry in calculus can be used to simplify the process of finding areas or volumes, especially when dealing with shapes that are mirror images of each other. The video discusses using symmetry to advantage when setting up integrals to find areas of regions bounded by curves.

💡Free Response

Free response in the context of the video refers to the section of a mathematics exam where students must provide a complete answer to a question, often involving multiple steps and showing work. The video emphasizes the importance of clear communication and proper setup of mathematical problems in a free-response scenario, such as when solving for the area or volume in calculus problems.

Highlights

The session focuses on reviewing area and volume calculations, which are significant topics in calculus.

Students are encouraged to pause the video and work through practice problems independently before checking the solutions.

The presenter discusses a method for calculating the area of an irregular shape using calculus, specifically integrating from 0 to 4 for the function √x.

An explanation is given for how to find the area under a curve that is bounded by two functions, using the concept of 'top function minus bottom function'.

The importance of understanding the order of functions when calculating areas and volumes is emphasized, as it affects whether you subtract or add the function values.

The presenter corrects a mistake and simplifies an integral to find the area under a curve, resulting in a final answer of twenty-eight thirds.

A strategy for solving multiple-choice calculus problems is discussed, including the use of symmetry and absolute values to simplify calculations.

The concept of a 'washer' in calculus is introduced, which is used to calculate the volume of a solid with a cylindrical hole through it.

The session covers how to calculate the volume of a solid with rectangular cross sections, using the formula for the area of a rectangle (base times height).

An example of calculating the volume of a solid generated by revolving a region around a line is provided, using the method of washers.

The presenter emphasizes the need for clear communication in calculus problems, ensuring that the steps taken are clear to the reader.

A problem involving the rate at which people leave a stadium after a game is solved using integral calculus, demonstrating the application of calculus in real-world scenarios.

The use of a trapezoidal sum to approximate the value of an integral is explained, showcasing a common technique in calculus for estimating areas under curves.

The presenter discusses the importance of showing work and including units when calculating rates of change, as it provides clarity and context to the solution.

A comprehensive review of the session's topics is provided, highlighting the importance of understanding calculus concepts for both area and volume calculations.

The session concludes with a reminder for students to fill out the feedback form and a thank you to the viewers for their engagement and participation.

Transcripts

Browse More Related Video

2021 Live Review 1 | AP Calculus AB | Understanding Derivatives & Chain Rule

AP Calc AB & BC Practice MC Review Problems #1

2022 Live Review 1 | AP Calculus AB | Practicing with Derivatives & Chain Rule

AP Calc AB & BC Practice MC Review Problems #7

8 | FRQ (No Calculator) | Practice Sessions | AP Calculus AB

Most Difficult AP Calculus FRQ Parts (Everyone in AB & BC Should Know)

Related Tags

Calculus Integrals Area Volume Review Session Real-world Math AP Exam Problem Solving Education Mathematics Exam Preparation