Implicit Differentiation - Finding Equation of Tangent Line

Sun Surfer Math
27 Feb 202208:46
EducationalLearning
32 Likes 10 Comments

TLDRThe video script presents a detailed walkthrough of implicit differentiation with two examples. The first example focuses on finding the slope of the tangent line to an ellipse at a given point, using implicit differentiation to derive the slope expression. The process involves differentiating both sides of the equation with respect to x, isolating y' (the derivative of y with respect to x), and substituting the given x and y values to find the slope. The second example extends this by finding the equation of the tangent line, which includes finding the slope at a specific point, using the point-slope formula, and solving for y to find the coordinates of the point of tangency. The script concludes with the equation of the tangent line in slope-intercept form, demonstrating a clear understanding of the concept and its application in mathematical problems.

Takeaways
  • 📐 The video discusses examples of implicit differentiation, a method for finding derivatives when the relationship between variables is not explicitly defined.
  • 🔍 To find the slope of the tangent line to an ellipse at a given point, one must differentiate the equation implicitly and solve for the derivative, which represents the slope.
  • 🧮 The process involves applying the power rule to terms involving x and using the chain rule for terms involving the dependent variable y.
  • 📉 After differentiating, isolate y' (the derivative of y with respect to x) to find the expression for the slope of the tangent line.
  • 📌 Substitute the given x and y values into the derivative expression to find the specific slope at that point on the curve.
  • 🔑 For the ellipse example, the slope of the tangent line at the point where x = √2/2 and y = 1 is found to be -√2.
  • 📈 The next example extends the process to find the equation of the tangent line, which requires both the slope and a point on the line.
  • 🧷 The point-slope formula is used to express the equation of the tangent line, which is y - y1 = m(x - x1).
  • 🔍 To find the y-value corresponding to x = 1, the original function is evaluated at x = 1, resulting in y = 2/3.
  • 📝 The final step is to substitute the slope and the point into the point-slope formula and simplify to get the equation of the tangent line in slope-intercept form.
  • 🧘‍♂️ The video emphasizes the importance of understanding implicit differentiation and the application of the point-slope formula in finding tangent lines to curves defined by implicit functions.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is performing examples of implicit differentiation.

  • What is the equation of the ellipse in Example 5?

    -The equation of the ellipse in Example 5 is 2x^2 + y^2 = 2.

  • How does one find the slope of the tangent line to a curve at a given point using implicit differentiation?

    -To find the slope of the tangent line, one must first take the derivative of the given equation implicitly, then solve for y' (the derivative of y with respect to x), and substitute the given point's coordinates into the derivative to find the slope.

  • What is the derivative of y with respect to x for the ellipse equation in Example 5?

    -The derivative of y with respect to x for the ellipse equation is y' = -4x/(2y), which simplifies to y' = -2x/y.

  • What are the coordinates of the given point on the ellipse in Example 5?

    -The coordinates of the given point on the ellipse are x = √2/2 and y = 1.

  • What is the slope of the tangent line to the ellipse at the given point?

    -The slope of the tangent line at the given point is -√2.

  • What is the function in the second example of the video?

    -The function in the second example is 3x - 2y = x^2 + y.

  • How do you find the derivative of the function in the second example?

    -The derivative is found by taking the derivative of each term implicitly, resulting in 3 - 2y' = 2x + y'.

  • What is the slope of the tangent line for the second example when x equals one?

    -The slope of the tangent line when x equals one is 1/3.

  • How do you find the equation of the tangent line using the point-slope formula?

    -You use the point-slope formula y - y1 = m(x - x1), where m is the slope and (x1, y1) is the given point on the line. You substitute the slope and coordinates of the given point into the formula to find the equation of the tangent line.

  • What is the final equation of the tangent line in slope-intercept form for the second example?

    -The final equation of the tangent line in slope-intercept form is y = (1/3)x + (1/3).

  • What is the significance of implicit differentiation in calculus?

    -Implicit differentiation is significant because it allows us to find derivatives of equations where the variable y is not explicitly isolated on one side of the equation, which is often the case with more complex functions or curves.

Outlines
00:00
📐 Implicit Differentiation of an Ellipse

The first paragraph of the video script introduces the topic of implicit differentiation with a focus on finding the slope of the tangent line to an ellipse given by the equation 2x^2 + y^2 = 2. The process involves recognizing the equation as an implicit function, where both x and y are interdependent. The derivative is found by applying the power rule to the terms and then solving for y', which represents the slope of the tangent line. The final expression for the slope at a given point is simplified to -2x/y. To find the actual slope at the point (√2/2, 1), the values of x and y are substituted into the expression, resulting in a slope of -√2.

05:02
🔍 Finding the Equation of a Tangent Line

The second paragraph builds upon the previous one by not only finding the slope of the tangent line but also deriving the full equation of the tangent line to a different implicit function, 3x - 2y = x^2 + y. The process starts by implicitly differentiating the function to find y', then solving for y' to get the slope of the tangent line. At x = 1, the slope is determined to be 1/3. The point-slope form of the line is then used, requiring the knowledge of a specific point on the line. By substituting x = 1 into the original function, the corresponding y-value is found to be 2/3, giving the point (1, 2/3). The final step is to write the equation of the tangent line in slope-intercept form, which after simplification is y = (1/3)x + 1/3.

Mindmap
Keywords
💡Implicit Differentiation
Implicit differentiation is a method used to find the derivatives of equations where the variable y is not explicitly isolated. In the video, it is used to find the slope of the tangent line to an ellipse without first solving for y in terms of x. It is central to the theme of the video as it is the primary technique demonstrated for both examples.
💡Ellipse
An ellipse is a geometric shape defined by the sum of the squares of two linear dimensions, which in the context of the video, is represented by the equation 2x^2 + y^2 = 2. The video focuses on finding the slope of the tangent line to an ellipse at a given point, making the ellipse a key concept in the discussion.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. In the video, finding the slope and equation of the tangent line to an ellipse is the main objective. The tangent line's slope is determined by the derivative of the function at the point of tangency.
💡Derivative
The derivative of a function represents the rate of change of the function with respect to one of its variables. In the video, derivatives are calculated implicitly to find the slope of the tangent line to the ellipse and the curve represented by the equation 3x - 2y = x^2 + y. Derivatives are fundamental to the process of implicit differentiation.
💡Slope
Slope is a measure of the steepness of a line, and in the context of the video, it refers to the slope of the tangent line to the given curves. The slope is found by differentiating the given equations and evaluating at specific points, which is essential for determining the behavior of the curve at those points.
💡Power Rule
The power rule is a basic principle in calculus that allows for the differentiation of monomial terms. In the video, it is used to differentiate the term '2x^2' by applying the rule, which states that the derivative of x^n is n*x^(n-1). The power rule is a fundamental tool in the process of implicit differentiation demonstrated in the video.
💡Substitution
Substitution is a mathematical technique used to replace one expression with another to simplify calculations. In the video, substitution is used to find the actual slope of the tangent line by plugging in the given x and y values into the derived expression. It is also used to find the y-value when the x-value is known, which is necessary for the point-slope form of the tangent line.
💡Point-Slope Form
The point-slope form is a method for writing the equation of a line given a point on the line and the line's slope. In the video, it is used to find the equation of the tangent line to the curve 3x - 2y = x^2 + y after finding the slope and a point on the line. The point-slope form is crucial for transitioning from a conceptual understanding of the tangent line to its explicit algebraic representation.
💡Ordered Pair
An ordered pair is a pair of numbers that represents a point's location in a coordinate system. In the video, the ordered pair (1, 2/3) is found by evaluating the original function at x = 1, and it represents the point of tangency on the curve. This ordered pair is then used in the point-slope form to find the equation of the tangent line.
💡Slope-Intercept Form
The slope-intercept form is a way of writing the equation of a line as y = mx + b, where m is the slope and b is the y-intercept. In the video, the equation of the tangent line is ultimately expressed in this form after simplifying the point-slope form. This is the standard form for representing a linear equation and is useful for graphing and further analysis.
💡Evaluation
Evaluation in the context of the video refers to the process of finding specific values of a function or its derivative at given points. It is used to determine the y-value corresponding to a given x-value in the original function and to find the numerical value of the slope at a particular point on the curve. Evaluation is essential for applying the point-slope form and for finding the explicit equation of the tangent line.
Highlights

The video provides examples of implicit differentiation, focusing on finding the slope of the tangent line to an ellipse at a given point.

The ellipse equation is 2x^2 + y^2 = 2, which is an implicit function where x's and y's are mixed together.

The slope of the tangent line is the derivative of the function at the specific point, which is found implicitly.

The derivative of the ellipse equation is taken with respect to x, resulting in 4x - 2y' = 0.

Solving for y' yields y' = -4x/2y, simplifying to y' = -2x/y.

To find the actual slope at a point, substitute the given x and y values into the derived expression.

For the point (sqrt(2)/2, 1) on the ellipse, the slope of the tangent line is found to be -sqrt(2).

The next example involves finding the equation of the tangent line to the curve 3x - 2y = x^2 + y.

The derivative is taken implicitly, resulting in 3 - 2y' = 2x + y'.

Solving for y' gives y' = (3 - 2x)/3, which simplifies to y' = 1 - (2/3)x.

Evaluating the slope at x = 1 results in a slope of 1/3 for the tangent line.

The point-slope form of the equation is used to find the equation of the tangent line, y - y1 = m(x - x1).

The original function is evaluated at x = 1 to find the corresponding y-value, which is 2/3.

The ordered pair (1, 2/3) is used to find the equation of the tangent line in point-slope form.

The final equation of the tangent line is simplified to y = (1/3)x + 2/3, in slope-intercept form.

The video demonstrates the process of implicit differentiation and its application in finding tangent lines to curves.

The method involves taking derivatives implicitly, solving for the derivative variable, and substituting values to find the slope.

The practical application includes finding the slope and equation of tangent lines to specific points on curves represented by implicit functions.

Transcripts

Browse More Related Video

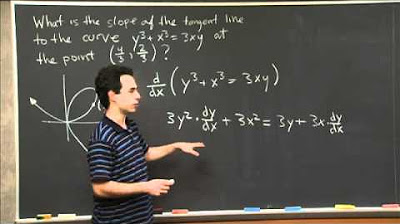
Implicit Differentiation | MIT 18.01SC Single Variable Calculus, Fall 2010

Implicit differentiation | Advanced derivatives | AP Calculus AB | Khan Academy

Implicit Differentiation

How To Find The Equation of a Tangent Line Using Derivatives - Calculus 1

Equation of a tangent line [IB Maths AI SL/HL]

Equation of a normal line [IB Maths AI SL/HL]

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Implicit DifferentiationTangent LineEllipse SlopeCurve EquationMathematicsCalculusDerivativesEducational ContentProblem SolvingAdvanced MathGeometry