Properties of Functions - Even vs Odd (Precalculus - College Algebra 8)
TLDRThe video script delves into the concepts of even and odd functions, which are often misunderstood by students. The presenter clarifies the misconception that even and odd relate solely to the parity of numbers or the exponents in functions. Instead, the focus is on the graphical and algebraic properties of these functions. An even function exhibits symmetry about the y-axis, meaning that for every input, its opposite yields the same output. Conversely, an odd function displays rotational symmetry about the origin, where opposite inputs result in opposite outputs. The script also emphasizes the importance of understanding the domain and range of functions and provides a method to algebraically determine if a function is even, odd, or neither by evaluating the function at negative inputs. The presenter uses various examples to illustrate these concepts and corrects a minor mistake made during the explanation. The video concludes with a teaser for the next topic, which will be about local and absolute extrema.
Takeaways
- 📈 Even and odd functions are a fundamental concept in mathematics, often misunderstood due to a common misconception about the relationship between even numbers and odd numbers.
- 🔍 The term 'even' in the context of functions refers to symmetry about the y-axis, meaning the graph of an even function will be a mirror image of itself when folded over the y-axis.
- 🔍 'Odd' functions exhibit symmetry about the origin, meaning rotating the graph 180 degrees results in the same graph, indicating that odd functions are symmetrical when flipped or rotated.
- 📊 To determine if a function is even, odd, or neither, one can visually inspect the graph for symmetry about the y-axis or the origin, or algebraically by evaluating the function for negative inputs.
- 🤔 A common mistake is assuming that the exponent of a function determines its evenness or oddness; however, this is only true for single-term functions like sine or cosine, and not for polynomials or other functions without exponents.
- 📚 The formal definition of an even function is that it yields the same output for opposite inputs (f(-x) = f(x)), while an odd function yields outputs that are opposite in sign for opposite inputs (f(-x) = -f(x)).
- 📐 When graphing, even functions will show symmetry about the y-axis, and odd functions will show symmetry about the origin, which can help in identifying the function's properties without complex calculations.
- 📈 Understanding the domain and range of a function is crucial; the domain is the set of all possible x-values that will produce a valid y-value, while the range is the set of all possible y-values.
- 🔢 For polynomial functions, the evenness or oddness is determined by the presence of even or odd powers of x. If a polynomial has only even powers, it is even; if it has an odd power term, it is odd.
- ✅ A shortcut for determining if a function is even, odd, or neither is to substitute -x into the function and observe the changes. If the function remains the same, it's even; if it becomes the negative of the original, it's odd; otherwise, it's neither.
- 📚 The properties of even and odd functions are not only important for theoretical understanding but also for practical applications, such as analyzing the behavior of functions around x-intercepts and asymptotes.
Q & A
What is the primary misconception students have when distinguishing between even and odd functions?
-The primary misconception is that students often believe the relationship between even and odd numbers (e.g., 2, 4, 6 for even and 1, 3, 5 for odd) determines the evenness or oddness of a function, which is not true.
How does the graph of an even function appear?
-The graph of an even function appears symmetrical about the y-axis, meaning if you fold it over the y-axis, it would create a mirror image of itself.
What property does the graph of an odd function have?
-The graph of an odd function has symmetry about the origin. If you rotate the graph 180 degrees about the origin, it will coincide with the original graph.
How can one algebraically determine if a function is even, odd, or neither?
-One can algebraically determine this by substituting -x for x in the function and observing the output. If the output is the same as the original function, it's even. If the output is the negative of the original function, it's odd. If neither condition is met, the function is neither.
Why is it incorrect to assume that the exponent of a function determines its evenness or oddness?
-It's incorrect because evenness or oddness is not solely determined by the exponent. For instance, the cosine function is even but does not have an exponent, and there are polynomials and functions without exponents that can still be classified as even or odd.
What is the relationship between a function's domain and range?
-The domain of a function is the set of all possible input values (x-values) for which the function is defined, while the range is the set of all possible output values (y-values) that result from applying the function to its domain.
How does the concept of even and odd functions relate to the behavior of functions around x-intercepts and asymptotes?
-The even and odd properties of functions can inform us about their behavior around x-intercepts and asymptotes. For example, even functions will have symmetrical behavior about the y-axis, which can affect their x-intercepts, while odd functions, having symmetry about the origin, may exhibit different behaviors around these points.
What is a quick way to check if a function is even without graphing?
-A quick way to check if a function is even without graphing is to evaluate the function for a negative input (f(-x)) and see if the result is the same as the original function (f(x)). If it is, the function is even.
What does it mean for a function to have 'neither' symmetry in relation to even or odd functions?
-A function that has 'neither' symmetry does not exhibit symmetry about the y-axis or the origin. This means that when you plug in opposite inputs, you do not get the same or opposite outputs, and the function does not satisfy the conditions for being even or odd.
How do you algebraically determine if a function is odd by plugging in -x?
-To determine if a function is odd by plugging in -x, you evaluate the function with -x and check if the result is the negative of the original function (-f(x)). If this is the case, the function is odd.
What is a common shortcut for determining if a function is even, odd, or neither when evaluating f(-x)?
-A common shortcut is to change the sign of the odd power terms in the function and then evaluate. If the function remains the same, it's even. If the function becomes the negative of the original, it's odd. If there is no symmetry or the function does not match either condition, it's neither.
Outlines
😀 Understanding Even and Odd Functions
This paragraph introduces the concept of even and odd functions, a topic that is often misunderstood. It emphasizes the importance of correctly interpreting what 'even' and 'odd' mean in the context of functions. The speaker clarifies that the relationship between even and odd numbers does not necessarily apply to functions in the same way. The formal definition of even and odd functions is promised, along with a discussion on how to identify these properties both graphically and algebraically. The symmetry properties of even functions about the y-axis and odd functions about the origin are highlighted, along with a quick method to check for even or odd nature of functions.
📈 Graphing and Symmetry in Functions
The second paragraph delves into the graphical representation of functions, focusing on how to determine if a function is even, odd, or neither by observing its symmetry. It explains that even functions will show symmetry about the y-axis, meaning that the graph can be folded along the y-axis to produce a mirror image. On the other hand, odd functions will display symmetry about the origin, which is observed when the graph is rotated 180 degrees and matches the original graph. The speaker also touches on the misconception that the exponent of a function determines its evenness or oddness, using examples from trigonometric functions to illustrate the point.
🔢 Algebraic Evaluation of Even and Odd Functions
This paragraph explains how to algebraically determine if a function is even or odd. It emphasizes that even functions will yield the same output for opposite inputs, thus creating symmetry about the y-axis. Conversely, odd functions will produce outputs that are opposite in sign when opposite inputs are plugged in, leading to symmetry about the origin. The mathematical representation of these properties is also discussed, showing how to plug in negative values for x and observe the resulting outputs to classify the function as even, odd, or neither.
🤔 Evaluating Functions at Negative Inputs
The fourth paragraph provides a method to evaluate the evenness or oddness of functions by substituting negative inputs into the function. It explains that if the function remains the same after the substitution, it is even, and if it is the same but with a negative sign, it is odd. The paragraph also corrects a mistake made in the explanation and stresses the importance of understanding the sign changes when plugging in negative values. It also discusses how to identify even, odd, or neither functions by evaluating them at negative inputs without graphing.
📉 Testing Functions for Even, Odd, or Neither
This paragraph continues the discussion on testing functions by evaluating them at negative inputs. It explains that even functions will yield the same output when a negative input is plugged in, while odd functions will yield outputs that are the negatives of the original function. The concept is further clarified with examples, and a shortcut method for determining the function's classification is introduced. The speaker also addresses situations where functions do not have defined outputs for negative inputs, leading to the classification of 'neither' due to the lack of symmetry.
🔍 Final Thoughts on Even, Odd, and Neither Functions
The final paragraph summarizes the process for determining if a function is even, odd, or neither by evaluating the function at negative inputs and examining the outputs. It reinforces the concepts of symmetry about the y-axis for even functions and about the origin for odd functions. The speaker also previews the next topic of discussion, which will be local and absolute extrema in functions, and concludes the video with a prompt for viewers to join the next session.
Mindmap
Keywords
💡Even function
💡Odd function
💡Symmetry
💡Domain
💡Range
💡Exponents
💡Trigonometric functions
💡Algebraic manipulation
💡Graphs
💡Polynomials
💡Rational functions
Highlights
The video discusses the concept of even and odd functions, which is often misunderstood by students.
Even and odd functions are not determined by the exponents of the functions, contrary to a common misconception.
An even function is symmetric about the y-axis, meaning the graph is a mirror image across the y-axis.
An odd function has symmetry about the origin, which means a 180-degree rotation of the graph results in the same graph.
The video provides a quick method to check if a function is even, odd, or neither by evaluating the function at negative inputs.
Even functions are characterized by equal outputs for opposite inputs, while odd functions produce opposite outputs.
The video clarifies that the domain of a function is the set of all x-values for which the function is defined.
The range of a function is the set of all possible output values (y-values), which can be different from the domain.
The video explains how to identify even and odd functions graphically by looking for symmetry about the y-axis or origin.
Trigonometric functions like sine and cosine are used as examples to illustrate the concept of even and odd functions.
The video emphasizes the importance of understanding the definitions of even and odd functions for algebraic manipulation.
A shortcut method is introduced for checking even, odd, or neither by changing the sign of odd powers and evaluating the function.
The video corrects a common mistake regarding the evaluation of functions at negative inputs for determining even or odd properties.
Functions that do not exhibit symmetry about the y-axis or the origin are classified as 'neither' even nor odd.
The concept of even and odd functions is crucial for understanding the behavior of polynomials and rational functions.
The video concludes with a preview of the next topic, which will be local and absolute extrema in functions.
Transcripts
Browse More Related Video
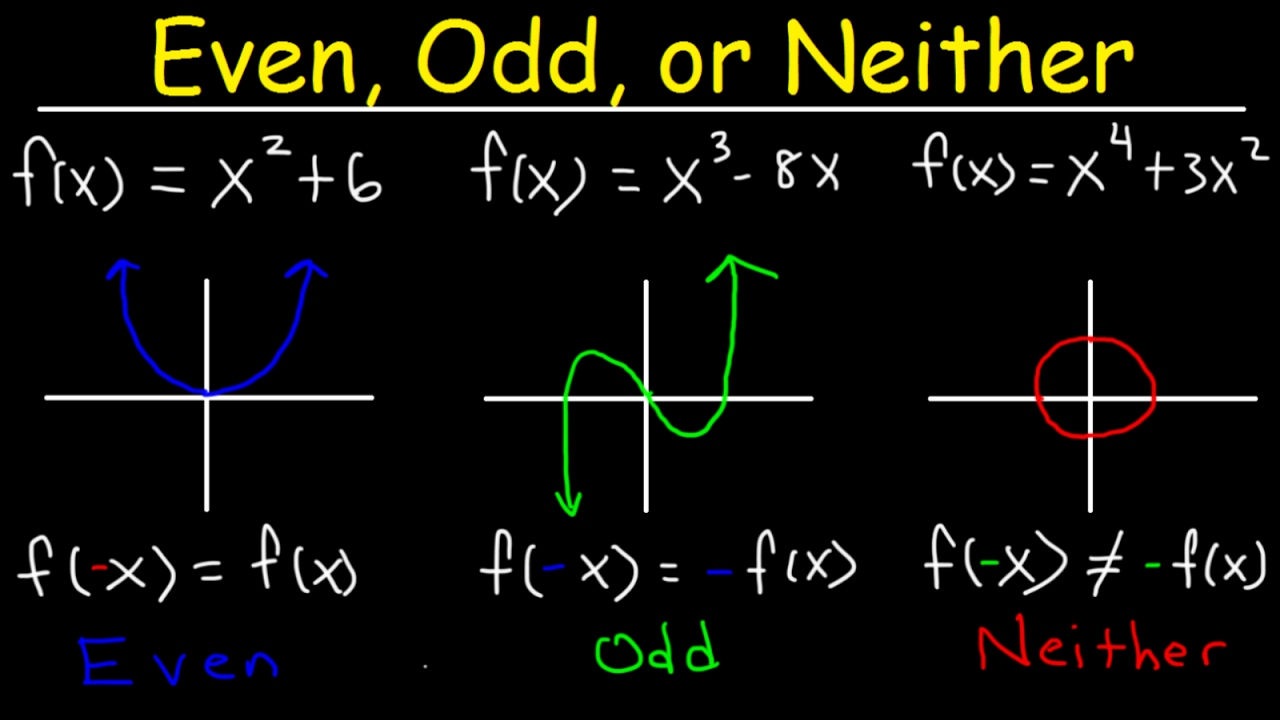
Even, Odd, or Neither Functions The Easy Way! - Graphs & Algebraically, Properties & Symmetry

Trig - 0.5 Periodic and Even and Odd Function Properties
Power Functions (Precalculus - College Algebra 28)
How to Graph Phase Shifts of Trigonometric Functions (Precalculus - Trigonometry 16)

Calculus 1 Lecture 0.2: Introduction to Functions.

Pythagorean Identities for Trigonometric Functions (Precalculus - Trigonometry 10)
5.0 / 5 (0 votes)
Thanks for rating: