Even, Odd, or Neither Functions The Easy Way! - Graphs & Algebraically, Properties & Symmetry
TLDRThis educational video script delves into the characteristics of even, odd, and neither functions. It explains that even functions satisfy f(-x) = f(x), while odd functions meet the condition f(-x) = -f(x). The script provides a simple method to determine function types by examining exponents and offers a step-by-step approach to prove these properties through examples. It also discusses graphical representations, explaining that even functions are symmetric about the y-axis and odd functions about the origin. The script concludes with the clarification that a circle, despite having symmetrical properties, is not a function and thus cannot be classified as even or odd.
Takeaways
- 🔍 A function is considered even if f(-x) = f(x), meaning replacing x with -x yields a function that looks exactly like the original.
- 🎯 A function is odd if f(-x) = -f(x), which implies that replacing x with -x changes the sign of every term in the function.
- 🚫 A function is neither even nor odd if f(-x) does not equal -f(x) or f(x), indicating a mix of sign changes or no sign changes when x is replaced with -x.
- 📚 To determine if a function is even, odd, or neither, one can look at the exponents of the terms; even exponents suggest an even function, while odd exponents suggest an odd function.
- 📈 For a function like f(x) = x^4 + 3x^2, since all exponents are even, the function is even, which is proven by replacing x with -x and showing the resulting function equals the original.
- ✂️ If all exponents in a function are odd, like in f(x) = x^5 + 2x^3, the function is odd, and this can be proven by showing f(-x) equals -f(x) after factoring out a negative one.
- 📉 For functions with a mix of even and odd exponents, such as f(x) = x^3 - 5x^2 + 2, the function is neither even nor odd because it does not satisfy the conditions for either property.
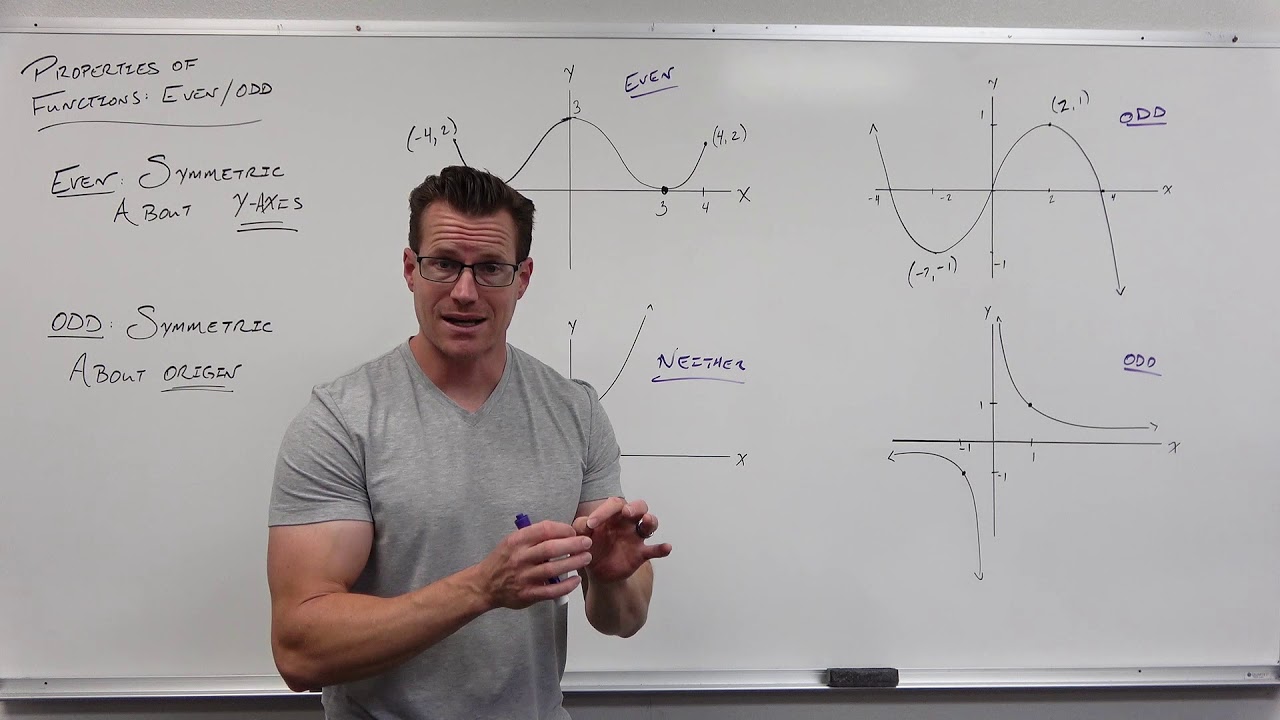
- 📊 Even functions have graphs that are symmetric about the y-axis, while odd functions have graphs that are symmetric about the origin.
- 📐 The graph of a function can help determine if it is even, odd, or neither based on its symmetry; for example, a circle is symmetric about both the y-axis and the origin but is not classified as a function due to not passing the vertical line test.
- 📘 Constants, such as f(x) = 6, are considered even functions because they can be thought of as x^0, which has an even exponent, and their graphs are horizontal lines symmetric about the y-axis.
Q & A
What is the definition of an even function?
-A function is considered even if f(-x) is equal to f(x). This means that replacing x with -x yields a function that looks exactly like the original function.
How can you determine if a function is odd?
-A function is odd if f(-x) equals -f(x). When you replace x with -x, every term in the function must change its sign for the function to be considered odd.
What does it mean for a function to be neither even nor odd?
-A function is neither even nor odd if, upon replacing x with -x, the resulting function does not equal either -f(x) or f(x). This often happens when some terms change sign while others do not.
How can you quickly determine if a polynomial function is even or odd by looking at its exponents?
-If all the exponents in the polynomial are even, the function is even. If all the exponents are odd, the function is odd.
What is the significance of the coefficients in determining if a function is even or odd?
-The coefficients (like the 2 in 2x^3) are unimportant when determining if a function is even or odd. It is the exponents that matter.
Can you provide an example of an even function from the script?
-An example of an even function from the script is f(x) = x^4 + 3x^2. This function is even because all the exponents are even.
How is the function f(x) = x^5 + 2x^3 determined to be odd?
-The function f(x) = x^5 + 2x^3 is odd because all the exponents (5 and 3) are odd. When x is replaced with -x, the signs of all terms change, resulting in -f(x).
What is the significance of the zero exponent in determining the evenness or oddness of a function?
-Any number raised to the zero power is 1, which is an even number. Therefore, terms like 6x^0 are considered even and do not affect the overall evenness of a function.
How can you use symmetry to determine if a function is even, odd, or neither from its graph?
-An even function will be symmetric about the y-axis, an odd function will be symmetric about the origin, and if there is no symmetry about either, the function is neither.
Can you provide an example of a graph that is neither even nor odd from the script?
-An example from the script of a graph that is neither even nor odd is a circle. It has symmetry about the y-axis but does not pass the vertical line test, so it is not a function.
What is the vertical line test, and why is it used to determine if a shape can represent a function?
-The vertical line test is a graphical method used to determine if a curve represents a function. If any vertical line intersects the curve more than once, the curve does not represent a function because a function must pass the vertical line test, meaning each input can only have one output.
Outlines
📚 Introduction to Even, Odd, and Neither Functions
This paragraph introduces the concept of determining whether a function is even, odd, or neither. An even function is defined by the property f(-x) = f(x), meaning replacing x with -x yields an unchanged function. An odd function is characterized by f(-x) = -f(x), where the sign of each term changes upon replacing x with -x. If a function does not meet the criteria for being even or odd, it is classified as neither. The paragraph begins with an example using the function f(x) = x^4 + 3x^2, demonstrating it as an even function by substituting x with -x and showing that the resulting function matches the original.
🔍 Analyzing Functions with Even and Odd Exponents
The second paragraph delves into analyzing functions with various exponents to determine their parity. It explains that a function is even if all its exponents are even, using the example f(x) = x^4 + 3x^2, and odd if all exponents are odd, demonstrated with f(x) = x^5 + 2x^3. The process involves substituting x with -x and simplifying to see if the function remains unchanged (even) or changes sign (odd). The paragraph also covers how to prove these properties algebraically and emphasizes the irrelevance of coefficients in determining parity.
📉 Visualizing Function Parity Through Graphs
This paragraph discusses the graphical representation of even and odd functions. It explains that even functions exhibit symmetry about the y-axis, while odd functions show symmetry about the origin. The paragraph uses the functions x^2 (even), x^3 (odd), and a constant function (even) to illustrate these concepts. It also challenges the viewer to identify the parity of various functions based on their graphs, emphasizing the importance of recognizing symmetry in determining whether a function is even, odd, or neither.
🤔 Determining Parity with Graphs and Special Cases
The final paragraph continues the discussion on function parity using graphs, providing examples and asking the viewer to determine if the functions are even, odd, or neither. It includes a circle, which, despite having symmetrical properties about both the y-axis and the origin, is not classified as a function due to failing the vertical line test. The paragraph reinforces the idea that symmetry about the y-axis indicates an even function, while symmetry about the origin suggests an odd function, and the absence of such symmetry indicates a function that is neither.
Mindmap
Keywords
💡Even function
💡Odd function
💡Neither even nor odd
💡Symmetry
💡Exponents
💡Coefficients
💡Graphs
💡Vertical line test
💡Factoring out
💡Constants
Highlights
A function is even if f(-x) equals f(x), meaning replacing x with -x yields the same function.
A function is odd if f(-x) equals -f(x), where replacing x with -x changes the sign of every term in the function.
A function is neither even nor odd if f(-x) does not equal f(x) or -f(x), and some signs change while others don't.
For an even function, all exponents in the function's expression must be even.
For an odd function, all exponents in the function's expression must be odd.
Coefficients in the function's expression do not affect its even or odd nature.
An even function's graph is symmetric about the y-axis, resembling an 'upward U' shape.
An odd function's graph is symmetric about the origin, with quadrants mirroring each other.
A function that is neither even nor odd will not exhibit symmetry about the y-axis or the origin.
Constants are considered even functions as they are symmetric about the y-axis.
The function f(x) = x^4 + 3x^2 is even, as all exponents are even and the graph is symmetric about the y-axis.
The function f(x) = x^5 + 2x^3 is odd, as all exponents are odd and the graph is symmetric about the origin.
The function f(x) = x^2 + 6 is even, as it contains an even exponent and is symmetric about the y-axis.
The function f(x) = x^3 - 8x is odd, as it contains odd exponents and the graph is symmetric about the origin.
The function f(x) = x^3 - 5x^2 + 2 is neither even nor odd, as it contains both even and odd exponents.
A circle's graph has even properties due to symmetry about the y-axis, but it is not a function and thus not classified as even or odd.
The video explains how to determine if a function is even, odd, or neither using algebraic manipulation and graph analysis.
Transcripts
Browse More Related Video
Properties of Functions - Even vs Odd (Precalculus - College Algebra 8)

Trig - 0.5 Periodic and Even and Odd Function Properties

Functions defined by definite integrals (accumulation functions) | AP Calculus AB | Khan Academy
Power Functions (Precalculus - College Algebra 28)

Graphs You Must Know (Precalculus - College Algebra 13)

Graphing Rational Functions with Transformations (Precalculus - College Algebra 39)
5.0 / 5 (0 votes)
Thanks for rating: