All Of Calculus Explained In 5 Minutes

1red2blue4
14 Mar 202104:56
EducationalLearning
32 Likes 10 Comments

TLDRThe video script introduces calculus as the study of change, focusing on concepts such as limits and instantaneous slope to understand the behavior of functions. It simplifies the process of finding derivatives and explains the intermediate value theorem and the mean value theorem. The script also contrasts differentiation with integration, highlighting the fundamental theorem of calculus, which states that the derivative of an integral returns the original function. The explanation is engaging, making complex concepts accessible and sparking interest in the viewer.

Takeaways
  • 📚 Calculus, also known as 'calc', is the mathematical study of change, focusing on variables such as time, distance, etc.
  • 🚧 The transition to 'pure math' often involves overcoming the barrier of understanding calculus concepts.
  • 📈 Understanding limits is crucial in calculus, as it allows for the handling of expressions that don't follow usual math rules, like dividing by zero.
  • 🔄 The concept of 'infinitesimal' is introduced in calculus, which deals with numbers so small they can be considered zero for practical purposes.
  • 📶 Instantaneous slope represents the slope of a graph at a specific point and is a fundamental concept in understanding the behavior of functions.
  • 🔄 To find the instantaneous slope, the limit of the function evaluated at a point 'x' plus a small 'h' is used.
  • 📊 Continuous functions can be drawn without lifting the pen from the paper, an example being 'y = x^2'.
  • 📝 Derivatives are calculated by applying specific rules to each term, reducing the power by one for variable terms and multiplying by the original power.
  • 📝 The derivative notation uses 'd' to indicate a derived quantity, such as 'dy' for the derivative of 'y'.
  • 🔄 Integration is the inverse process of differentiation, combining infinite small pieces to form a whole, like finding the area under a curve.
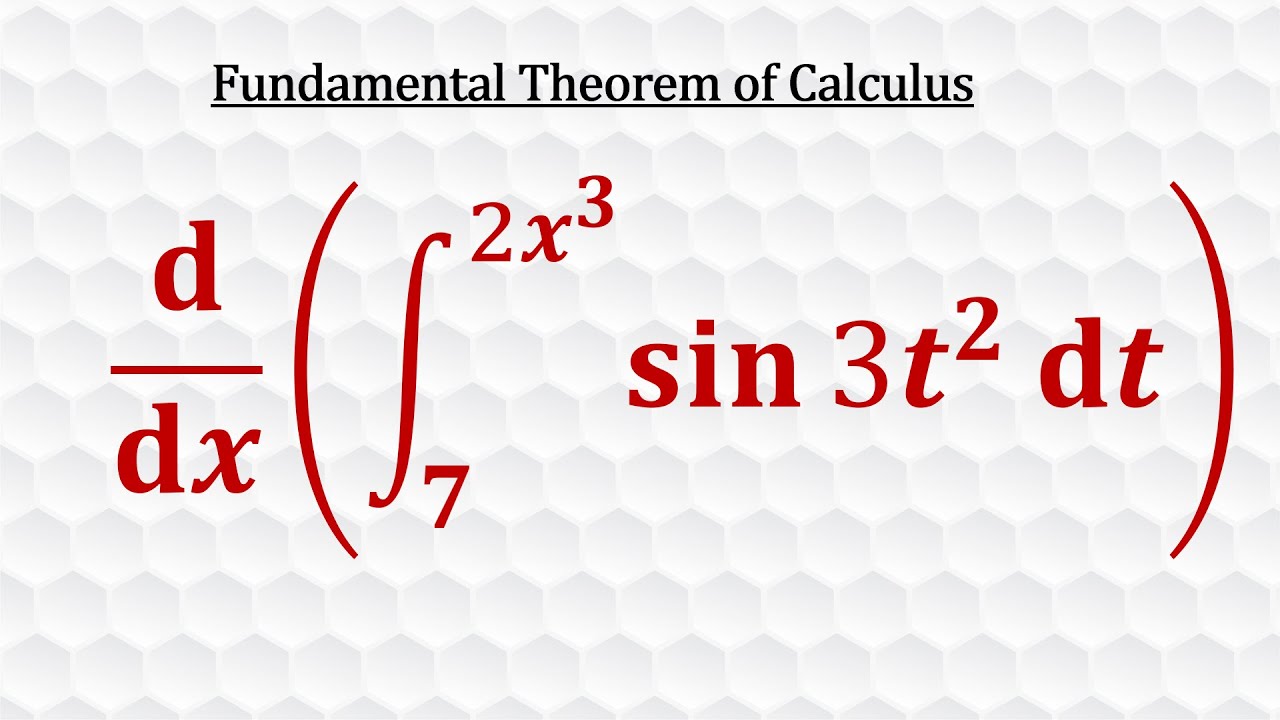
  • 📈 The Fundamental Theorem of Calculus states that the derivative of the integral of a function is the function itself, highlighting the relationship between differentiation and integration.
Q & A

  • What is calculus?

    -Calculus is the mathematical study of change, focusing on rates of change over time, distance, or other variables.

  • Why is calculus considered a barrier to the pure math zone?

    -Calculus is seen as a barrier because it introduces concepts such as limits and infinitesimals, which are more abstract and can be challenging for students transitioning from more concrete mathematical concepts.

  • How are limits used in calculus?

    -Limits are used to make exceptions to usual mathematical rules, such as dividing by zero. They allow us to handle situations where a variable approaches a certain value, like zero, by considering the behavior of a function as the variable gets infinitesimally close to that value.

  • What is the instantaneous slope of a graph?

    -The instantaneous slope of a graph at a given point is the slope of the tangent line at that point, representing the rate of change of the function at that specific location.

  • How can we find the slope of any point on a graph?

    -We can find the slope of any point on a graph by taking the limit as x approaches zero of the function evaluated at x plus a small increment h, divided by h. This gives us the instantaneous slope at x.

  • What is the process of taking the derivative of a function?

    -The process of taking the derivative of a function involves applying the power rule, which states that if a term has a variable, we reduce its power by one and multiply by the original power as a constant factor. For example, the derivative of x^2 is 2x, where the power is reduced from 2 to 1 and then multiplied by 2.

  • What is the intermediate value theorem in calculus?

    -The intermediate value theorem states that if a continuous function has a smallest and largest value, then it must take on every value in between at some point within its domain.

  • What is the mean value theorem?

    -The mean value theorem states that for a continuous curve with a section that is differentiable, the weighted average slope of that section is equal to the slope of the single line connecting the endpoints.

  • How is integration related to differentiation?

    -Integration is the inverse process of differentiation. While differentiation breaks down a function into infinitesimally small parts, integration combines these parts back together to form the original function or a new one.

  • What does the fundamental theorem of calculus state?

    -The fundamental theorem of calculus states that if you take the derivative of the integral of a function, you get back the original function. However, it's important to note that the integral of the derivative of a function does not necessarily equal the original function.

  • How does the concept of a definite integral differ from an indefinite integral?

    -A definite integral calculates the area under a curve over a specific interval, providing a numerical value. An indefinite integral, on the other hand, does not specify an interval and instead returns a function, which includes an unknown constant representing the area up to any point within the function's domain.

Outlines
00:00
📚 Introduction to Calculus and Limits

This paragraph introduces calculus, the study of change, and its application in various contexts such as time, distance, and other variables. It explains the concept of limits, which are used to handle mathematical exceptions like division by zero. The explanation includes an example of a limit as x approaches zero, illustrating the concept of infinitesimals. The paragraph also touches on the idea of instantaneous slope and the continuity of functions, setting the foundation for understanding calculus.

📈 Understanding Derivatives and the Slope of Functions

This section delves into the concept of derivatives, which represent the slope or rate of change of a function at any given point. It explains how to calculate the instantaneous slope using limits and provides a clear example using the function y = x^2. The paragraph outlines the rules for deriving terms with variables, emphasizing the importance of the derivative notation, such as d y and dx. It also explains how to find the slope of a graph at a specific point using derivatives.

🧩 The Intermediate and Mean Value Theorems

This paragraph discusses two important theorems in calculus: the Intermediate Value Theorem and the Mean Value Theorem. The Intermediate Value Theorem states that for a continuous function, if you pick any values within the range of the function, the function will take on all values in between. The Mean Value Theorem asserts that for a differentiable function over an interval, there exists at least one point where the slope of the function equals the average slope between two endpoints of the interval.

🔄 Integration: The Inverse Process of Differentiation

This section introduces integration as the inverse process of differentiation, where the goal is to combine infinitesimally small pieces to form a whole. It explains the process of integration by reversing the steps of differentiation, increasing the power of the variable by one and dividing by the new power. The paragraph also highlights the Fundamental Theorem of Calculus, which states that the derivative of the integral of a function is the function itself. However, it notes that the reverse is not always true without considering the span of integration.

Mindmap
Keywords
💡Calculus
Calculus is the branch of mathematics that studies rates of change and accumulation. It is often considered the gateway to pure mathematics due to its abstract nature and the powerful tools it provides for analyzing complex problems. In the video, calculus is introduced as the mathematical study of change, specifically change over time, but it can also apply to other variables like distance. The main theme of the video revolves around explaining the fundamental concepts of calculus, such as limits, derivatives, and integrals, and how they relate to understanding the behavior of functions and their graphical representations.
💡Limits
Limits are a fundamental concept in calculus that help us understand the behavior of functions as their input approaches a particular value. They are used to define continuity, derivatives, and integrals, among other things. In the context of the video, limits are introduced as a way to handle situations where the usual rules of arithmetic, such as division by zero, do not apply. The concept of infinitesimally small values is also discussed, which allows for the handling of limits as values approach zero.
💡Instantaneous Slope
Instantaneous slope refers to the slope of a curve at a specific point. It is the rate of change of a function at that particular moment and is used to understand the behavior of the function at a precise instant. In the video, the concept is explained by looking at the graph y = x^2 and determining the slope at x = 0 by examining the points just to the left and right of 0. The instantaneous slope is zero at x = 0 because the curve changes from negative to positive slope at that point.
💡Continuous Function
A continuous function is one where it is possible to draw the entire graph without lifting the pen from the paper. In other words, there are no breaks or jumps in the graph. Continuous functions are important in calculus because they allow for the application of calculus techniques, such as differentiation and integration. The video explains that a continuous function, like y = x^2, can be analyzed using calculus to find properties like the slope at any point.
💡Derivative
The derivative of a function is a measure of its rate of change at any given point. It is a fundamental concept in calculus that allows us to find the slope of a curve, which can be thought of as the instantaneous rate of change of the function. The video explains the process of finding the derivative of a function by taking the limit of the average rate of change as the interval size approaches zero. It also introduces the power rule for differentiation, which states that the derivative of x^n (where n is a constant) is n*x^(n-1).
💡Integration
Integration is the process of finding the original function from its derivative, effectively the reverse process of differentiation. It involves summing an infinite number of small pieces to find a whole, which can be thought of as the area under a curve. The video explains integration as the inverse of differentiation, where the process involves increasing the power of the variable by one and dividing by that new power. For example, the integral of 2x with respect to x is x^2, illustrating the fundamental theorem of calculus that the integral of the derivative of a function returns the original function.
💡Intermediate Value Theorem
The Intermediate Value Theorem is a fundamental theorem in calculus that states that if a function is continuous on a closed interval [a, b] and k is any number between f(a) and f(b), then there exists at least one number c in the interval [a, b] such that f(c) = k. This theorem is used to prove the existence of roots for continuous functions and has many applications in various mathematical and real-world problems. In the video, the theorem is humorously referenced in the context of a game, highlighting its importance in ensuring that a continuous curve must contain all values between its maximum and minimum.
💡Mean Value Theorem
The Mean Value Theorem is a key result in calculus that applies to differentiable functions. It states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one number c in the interval (a, b) such that the derivative of the function at c is equal to the average rate of change of the function over the interval [a, b]. This theorem is crucial for understanding the relationship between the average behavior of a function over an interval and its instantaneous behavior at individual points within that interval.
💡Definite Integral
A definite integral is a specific type of integral that calculates the signed area under a curve of a function over a specified interval [a, b]. It is called 'definite' because it deals with a fixed interval, unlike the indefinite integral, which does not have specified limits. The video explains that the definite integral provides a way to find the exact area under the curve, which can be used to solve various real-world problems, such as calculating distances or volumes.
💡Indefinite Integral
The indefinite integral is the process of finding a function whose derivative is a given function. It is called 'indefinite' because it does not involve specific limits, and it results in a family of functions that differ by a constant. The video mentions that the indefinite integral introduces an unknown constant, which is why it is often used in conjunction with the Fundamental Theorem of Calculus to find the particular antiderivative that satisfies given conditions.
💡Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus is a cornerstone result that connects differentiation and integration. It states that if a function is continuous on an interval and has an antiderivative (a function whose derivative is the given function), then the definite integral of the function over any subinterval of that interval is equal to the difference between the antiderivative values at the endpoints of the subinterval. This theorem is crucial for solving many practical problems in calculus and is highlighted in the video as a key concept that demonstrates the relationship between differentiation and integration.
Highlights

Calculus is the mathematical study of change, focusing on change over time, distance, or other variables.

The concept of limits allows for exceptions to usual mathematical rules, such as dividing by zero in certain scenarios.

Instantaneous slope represents the slope of a graph at any given point, which can change from one point to the next.

Continuous functions can be drawn without lifting the pencil, such as y = x^2.

The slope of the graph y = x^2 at x = 0 is found by evaluating the function at points just to the left and right.

The process of finding the slope at any point on a graph involves taking the limit as x approaches zero of the function evaluated at x plus h, divided by x plus h.

Derivatives are calculated by reducing the power of a variable term by one and multiplying by the original power as a constant factor.

The intermediate value theorem states that a continuous curve must contain all y values between its highest and lowest points.

The mean value theorem asserts that the weighted average slope of a differentiable section of a curve is equal to the slope of the line between two points.

Integration is the inverse process of differentiation, combining infinite small pieces to form a whole.

The fundamental theorem of calculus states that the derivative of the integral of a function is the function itself.

Integration requires increasing the power of a variable by one and dividing by the new power, which is the reverse operation of differentiation.

Definite integrals provide the value of the integral over a known span, unlike indefinite integrals which leave an unknown constant.

The integral of the derivative of a function does not necessarily equal the original function, due to the derivative of a constant being zero.

Understanding calculus involves learning about limits, derivatives, instantaneous slope, continuous functions, and the processes of integration and differentiation.

The application of calculus concepts such as instantaneous slope and the mean value theorem are crucial for analyzing the behavior of functions and their graphs.

Transcripts

Browse More Related Video

Calculus - Lesson 15 | Relation between Differentiation and Integration | Don't Memorise

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Fundamental Theorem of Calculus - Part I

Calculus (Version #2) - 9.1 The Second Fundamental Theorem of Calculus

Derivatives of Integrals (w/ Chain Rule)

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Math EducationCalculus ConceptsLimits and InfinitesimalsDerivatives and IntegralsContinuous FunctionsIntermediate Value TheoremMean Value TheoremPolynomial FunctionsFundamental Theorem of Calculus