ALL OF Calculus 2 in a nutshell.
TLDRThe video script provides an insightful overview of Calculus 2, focusing on series and integrals. It introduces power series and Taylor series, highlighting their applications in approximating functions. The concept of convergence and divergence in series is explained using the ratio test. The script delves into advanced integration techniques such as partial fractions, integration by parts, and trigonometric substitution. It also touches on the significant applications of integrals in physics, emphasizing their utility in 3D calculus and real-world problem-solving.
Takeaways
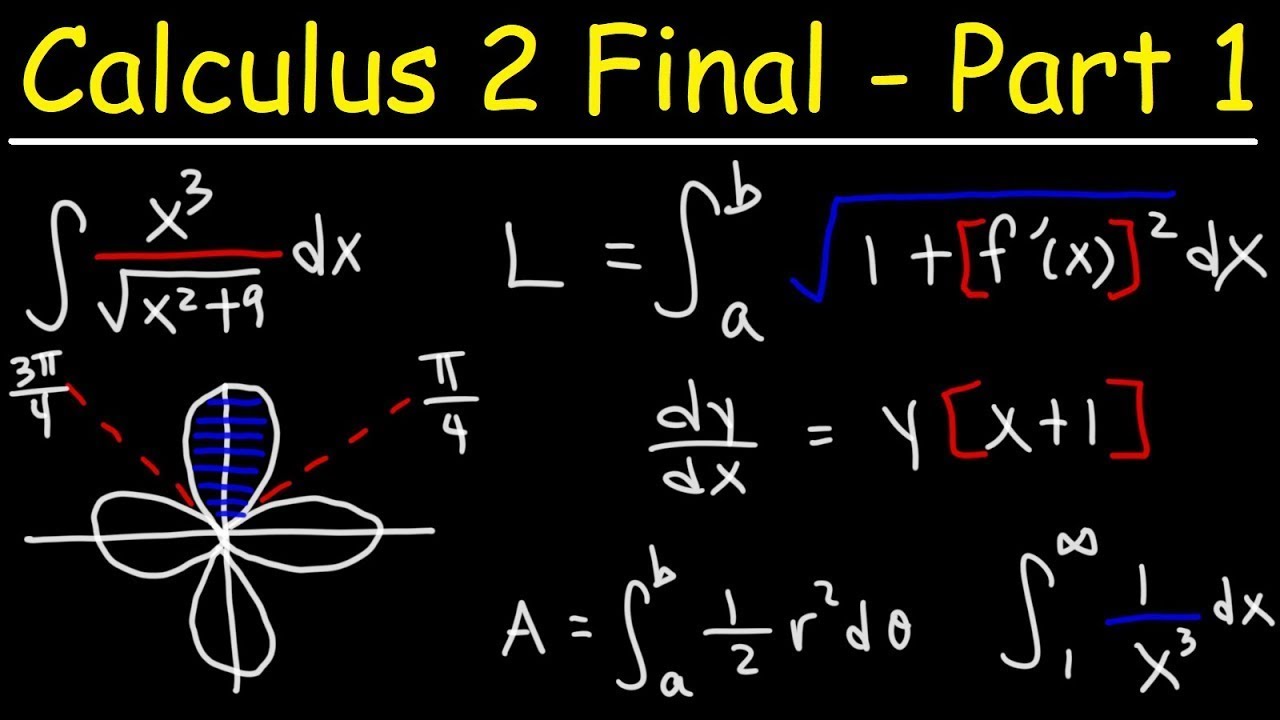
- 📚 Calculus 2 focuses on series and integrals, which are more complex than those in Calculus 1.
- 🔢 A power series is a specific type of series in the form ∑(C_n * x^n) from n=0 to infinity, where C_n are coefficients.
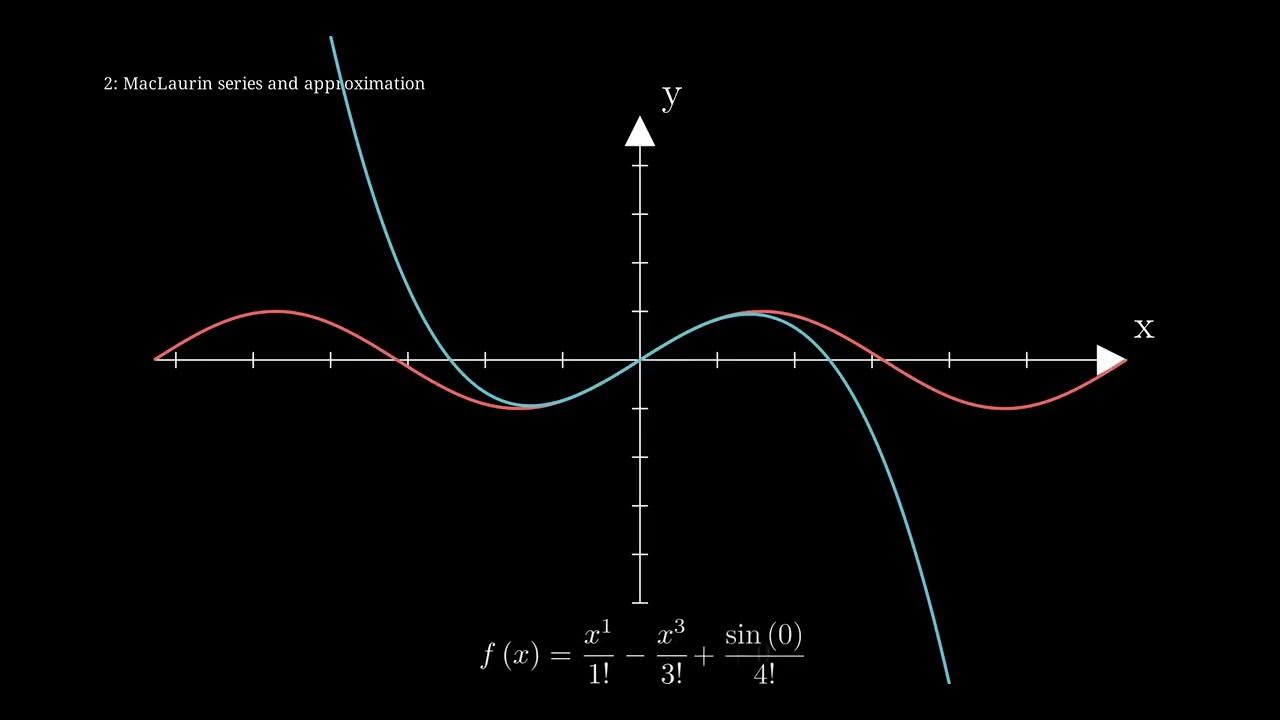
- 📈 The Taylor series is a special power series that represents a function's expansion about a specific point, creating a Taylor polynomial for approximation purposes.
- 🔍 The convergence or divergence of a series is determined by how its partial sums behave, either approaching a certain value or heading towards infinity.
- 📊 The ratio test is a method to assess the convergence of a series by examining the limit of the ratio of consecutive terms.
- 🌟 As the value of n increases in a series expansion, the approximation of the function becomes more accurate.
- 📐 Calculus 2 delves into various integration techniques such as partial fractions, integration by parts, polynomial long division, U-substitution, and trigonometric substitution.
- 🔄 Integration techniques are essential for dealing with complicated functions that cannot be integrated using basic rules.
- 💡 Integrals have significant applications in physics, such as calculating the center of mass, total work done, and magnetic fields.
- 🚀 The applications of integrals expand further in Calculus 3 with the introduction of 3D calculus.
Q & A
What is the primary focus of Calculus 2?
-The primary focus of Calculus 2 is on series and integrals, which builds upon the concepts learned in Calculus 1.
What is a power series in mathematics?
-A power series is a type of series that involves the summation of terms where each term is a coefficient multiplied by a variable raised to a power, and this summation can extend to an infinite number of terms.
How is a Taylor series useful in calculus?
-A Taylor series is a series expansion of a function about a specific point, which can be used to approximate complex functions with a polynomial, and the accuracy of the approximation increases with more terms.
What does the convergence of a series indicate?
-The convergence of a series indicates that the partial sums of the series approach a certain value as more terms are added, signifying a finite result.
How can the ratio test help determine the nature of a series?
-The ratio test involves comparing the limit of the ratio of consecutive terms to the value 1. If the limit is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the series may be conditionally convergent or absolutely convergent.
What are some of the advanced integration techniques covered in Calculus 2?
-Advanced integration techniques in Calculus 2 include integration by partial fractions, integration by parts, polynomial long division, u-substitution, and trigonometric substitution.
How are integrals applied in the field of physics?
-Integrals are applied in physics to calculate the center of mass of rigid bodies, the total work done, and the magnetic field at a point due to an infinite wire, among other applications.
What is the significance of increasing the value of n in a Taylor series?
-Increasing the value of n in a Taylor series leads to a more accurate approximation of the function because more terms of the series are included in the expansion.
What is the role of power series in approximating difficult functions?
-Power series are used to approximate difficult functions by summing the terms of the series, which can closely mimic the behavior of the function over a certain interval.
What happens when a series is divergent?
-When a series is divergent, its partial sums do not approach a specific value; instead, they may tend towards positive or negative infinity or oscillate without converging.
Outlines
📚 Introduction to Calculus 2: Series and Integrals
This paragraph introduces the viewer to the core concepts of Calculus 2, which primarily revolves around series and integrals. It emphasizes that Calculus 2 will be more challenging than the first course and encourages the viewer to prepare accordingly. The paragraph begins by explaining the concept of a power series, a special type of series in calculus, and how it is represented in the form of the summation of the coefficient C_n multiplied by X to the power of n, from n=0 to infinity. It then transitions into discussing the Taylor series, a series expansion of a function about a specific point, and how increasing the value of n leads to a more accurate approximation of the function. The paragraph also touches on the concepts of convergence and divergence of a series, explaining how partial sums approach certain values or go to infinity, and introduces the ratio test as a method to determine the convergence of a series using limits.
🧮 Advanced Integration Techniques and Applications of Integrals
This paragraph delves into the advanced integration techniques covered in Calculus 2, which are essential for solving more complex integrals. It outlines the main integration techniques, including partial fraction decomposition, integration by parts, polynomial long division, U-substitution, and trigonometric substitution, highlighting their importance and complexity. The paragraph also discusses the powerful applications of integrals, especially in the realm of physics, where they can be used to calculate the center of mass of rigid bodies, total work done, and magnetic fields imposed by infinite wires. The summary emphasizes the significance of integrals in higher-level calculus and their practical applications in various fields.
Mindmap
Keywords
💡Calculus 2
💡Power Series
💡Taylor Series
💡Convergence and Divergence
💡Ratio Test
💡Integration Techniques
💡Applications of Integrals
💡Series Expansion
💡Derivatives
💡Polynomial
Highlights
Calculus 2 focuses mainly on series and integrals, which are more complex than those in Calculus 1.
A power series in mathematics is a special type of series in the form of the summation of the coefficient C_n multiplied with x raised to the power of n.
Power series are significant in calculus due to their numerous applications, such as approximating difficult functions.
Taylor series is a series expansion of a function about a specific point and is represented using Sigma notation, which outputs a polynomial known as the Taylor polynomial.
The Taylor polynomial is an approximation of the function at a certain point, and increasing the value of n results in a more accurate approximation.
Convergence and divergence of a series are concepts similar to continuity, with convergent series approaching a certain value and divergent series going to infinity or not approaching a specific value.
The ratio test is a method to determine the convergence of a series by evaluating a specific expression involving limits.
Integration techniques are a major part of Calculus 2, extending beyond the basic concepts introduced in Calculus 1.
Some of the main integration techniques include partial fractions, integration by parts, polynomial long division, U-substitution, and trigonometric substitution.
Integrals are powerful tools in physics, used to find the center of mass of rigid bodies, calculate total work done, and determine magnetic fields.
The applications of integrals become even more significant in Calculus 3 when dealing with 3D calculus.
The video provides a brief overview of Calculus 2, but mastering the subject requires more in-depth study.
Series in mathematics involve adding up numbers based on a pattern, potentially summing an infinite number of quantities.
The summation in a power series involves substituting different values of n to get terms like C_0, C_1 multiplied by x, C_2 multiplied by x squared, and so on.
The Taylor series can be used to approximate functions with increasing accuracy by adding more terms up to infinity.
The convergence or divergence of a series can be determined by the behavior of its partial sums.
Integration of complicated functions sometimes requires advanced techniques that may not be immediately apparent from basic integration rules.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: