Calculus 2 In Less Than 20 Minutes (Complete Overview Of Integral Calculus)
TLDRThis video offers an insightful overview of Calculus 2, also known as integral calculus, focusing on its applications such as finding the area between curves, the volume of solids of revolution, and various integration techniques. It also touches on advanced topics like parametric and polar equations, sequences and series, and power series, culminating in an introduction to Taylor and Maclaurin series. The content is presented in a way to demystify the complexities of Calculus 2 and encourage further exploration.
Takeaways
- 📚 Calculus 2, also known as integral calculus, is often challenging for students and builds upon the concepts learned in Calculus 1, or differential calculus.
- 🔄 In Calculus 1, students focused on differentiation, derivatives, and their applications, with a brief introduction to integration methods like reverse power rule and substitution.
- 📈 Calculus 2 delves deeper into integration techniques, including more advanced methods such as integration by parts, trigonometric integrals, and improper integrals.
- 🏢 Applications of integration in Calculus 2 involve finding the area between curves, the volume of solids of revolution, and surface area, among other practical uses.
- 📊 The course introduces various methods for finding the volume of solids of revolution, which can involve complex cross sections like washers and require integration for calculation.
- 🥂 Techniques like integration by parts allow the solving of integrals involving multiplication of two functions, such as ∫x*cos(x)dx.
- 📐 Partial fraction decomposition is a technique for integrating complex fractions and involves breaking them down into simpler fractions for easier integration.
- 🌐 Parametric and polar equations are introduced as different ways to represent mathematical relationships and are used in calculating derivatives and integrals.
- 🔢 Sequences and series are covered in depth, with a focus on understanding convergence and divergence, and the application of series tests to determine these properties.
- 🔩 Power series are a key concept, where functions can be represented as infinite series, allowing for approximations and analysis of function behavior.
- 📝 Taylor and Maclaurin series are introduced as methods for representing functions as power series, useful for a variety of functions and providing a comprehensive understanding of series behavior.
Q & A
What is the main focus of Calculus 2?
-Calculus 2, also known as integral calculus, focuses on the application of integration techniques, including finding areas between curves, volumes of solids of revolution, and various other advanced integration methods.
What are some key concepts reviewed at the beginning of Calculus 2?
-At the start of Calculus 2, students typically review concepts from Calculus 1, such as differentiation, derivatives, and basic integration techniques like the reverse power rule and integration by substitution.
How is the area between two curves calculated?
-The area between two curves is calculated by integrating the difference between the two functions over the given interval. This involves subtracting the lower function from the upper function and evaluating the integral.
What is the process for finding the volume of a solid of revolution?
-To find the volume of a solid of revolution, one must take cross sections of the solid and sum up the areas of these cross sections. This process is similar to integration and can involve various methods depending on the shape of the solid and the axis of rotation.
What are some advanced integration techniques taught in Calculus 2?
-Some advanced integration techniques taught in Calculus 2 include integration by parts, trigonometric integrals, inverse trigonometric substitution, partial fraction decomposition, and handling improper integrals.
How is arc length calculated for a curve?
-Arc length is calculated using a specific formula that involves integrating the square root of the sum of the squares of the first derivative of x with respect to arc length and the first derivative of y with respect to arc length.
What is the difference between parametric equations and polar equations?
-Parametric equations involve expressing x and y as functions of a third variable, t, whereas polar equations express r as a function of an angle, theta, allowing for the representation of circles, lines, and other shapes in polar coordinates.
What are sequences and series in the context of Calculus 2?
-Sequences are functions that assign a value to each positive integer n, while series are the sum of the terms of a sequence. In Calculus 2, students learn to analyze sequences and series for convergence or divergence, and to represent functions as power series.
How do you determine if a geometric series converges or diverges?
-A geometric series converges if the common ratio, R, is between -1 and 1 (excluding -1 and 1). If R is greater than 1 or less than -1, the series diverges. The convergence of a geometric series can be determined without the need for series tests.
What is a power series and how is it used?
-A power series is an infinite sum of terms where each term is a power of x multiplied by a coefficient. Power series are used to represent functions and to determine the intervals of convergence for these representations, which can help in approximating functions and solving problems in calculus.
What are Taylor and Maclaurin series, and how do they relate to power series?
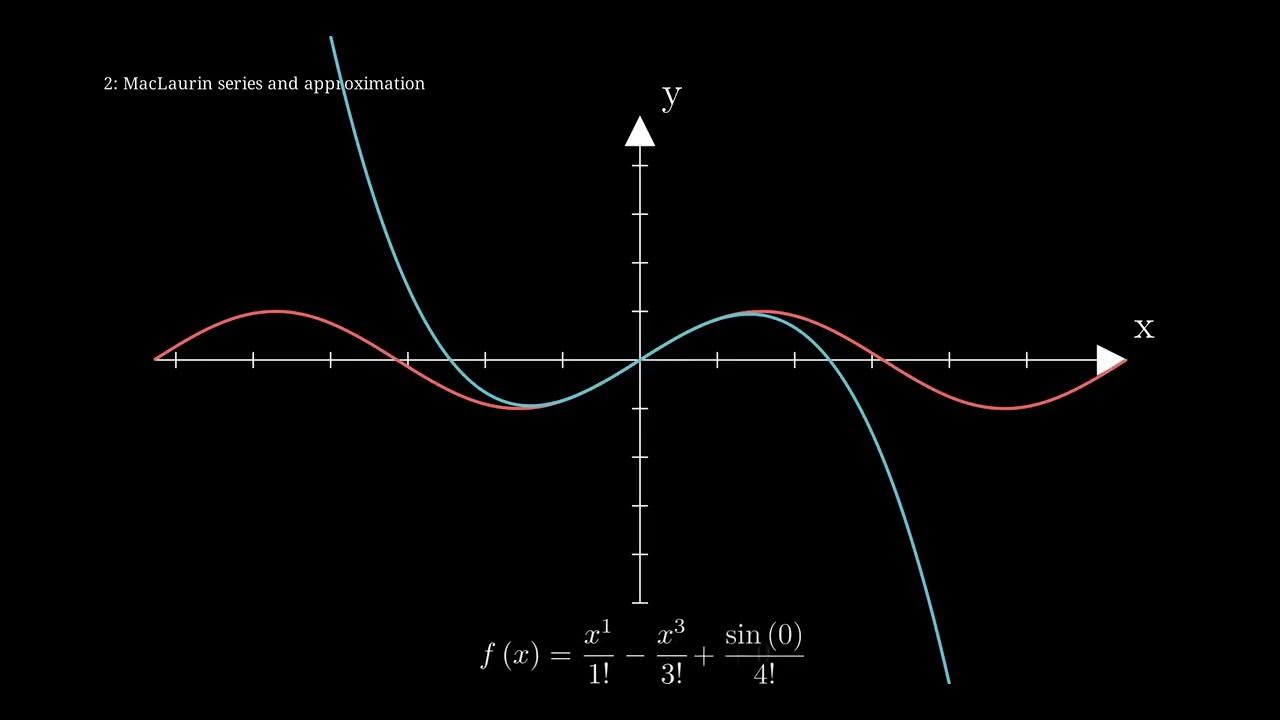
-Taylor and Maclaurin series are special types of power series used to represent functions. Taylor series provides a general representation of a function as a power series around a point, while Maclaurin series is a specific case of the Taylor series centered at 0. These series allow for the approximation of functions and their derivatives using the values of the function's derivatives at a single point.
Outlines
📘 Introduction to Calculus 2 and Review of Calculus 1
This section provides an introductory overview of Calculus 2, emphasizing its challenge for many students and the importance of breaking down its concepts. It begins by recapping Calculus 1, focusing on differentiation techniques like derivatives and integration methods such as the reverse power rule and integration by substitution. The transition to Calculus 2 material is signaled with a promise to explore more advanced integration applications, including finding areas between curves and volumes of solids of revolution, highlighting the continuation and expansion of integration techniques from Calculus 1.
📊 Advanced Integration Techniques and Applications
The focus shifts to advanced integration applications and techniques in Calculus 2, starting with the application of integration to find the area between curves and the volume of solids of revolution, utilizing methods like the washer method for cross sections. It then delves into techniques of integration beyond those introduced in Calculus 1, including integration by parts, trigonometric integrals, and the challenging partial fraction decomposition and improper integrals. The section underscores the importance of selecting the right integration method based on the problem at hand and introduces additional integration applications such as arc length and surface area calculations.
📐 Exploring Parametric, Polar Coordinates, and Their Applications
This segment introduces parametric and polar coordinates, explaining their fundamentals and how they differ from standard Cartesian coordinates. It covers the basics of parametric equations, which involve functions of a parameter 't' leading to XY pairs, and polar coordinates, which are expressed as functions of theta. The discussion includes how to derive and integrate functions within these coordinate systems, as well as how to calculate arc lengths and surface areas for shapes defined in parametric and polar forms. The emphasis is on understanding these concepts to prepare for more complex topics like sequences and series.
🔢 Sequences, Series, and Power Series Analysis
The narrative progresses to sequences and series, fundamental concepts that often challenge students. It explains sequences as ordered lists of numbers generated by a formula, touching upon properties like convergence, boundedness, and monotonicity. The discussion then expands to series, with a focus on geometric series and the introduction to series tests for determining convergence or divergence. Power series are introduced, highlighting their role in representing functions and the importance of determining their convergence based on the value of 'x'. The section sets the stage for exploring Taylor and Maclaurin series, pivotal in representing functions as power series.
🧮 Taylor and Maclaurin Series and Course Summary
The final section covers Taylor and Maclaurin series, essential tools for representing a wide variety of functions as power series. It highlights the versatility of these series in approximating functions beyond the specific cases addressed by earlier series discussions. The narrative emphasizes the importance of understanding preceding topics to grasp Taylor and Maclaurin series effectively. The video concludes by acknowledging the potential for overwhelming information, inviting questions, and directing viewers to further resources, including a comprehensive Calculus 2 course and final review materials on the presenter's website.
Mindmap
Keywords
💡Integral Calculus
💡Applications of Integration
💡Techniques of Integration
💡Improper Integrals
💡Series and Sequences
💡Power Series
💡Taylor and Maclaurin Series
💡Parametric Equations
💡Polar Coordinates
💡Convergence and Divergence
Highlights
Overview of Calculus 2, also known as Integral Calculus, which is often challenging for students.
In Calculus 1, students focused on differentiation, derivatives, and their applications, with some basic integration techniques.
Calculus 2 builds upon the foundation of Calculus 1, with a focus on the applications of integration.
Integration techniques expanded in Calculus 2 include the reverse power rule, integration by substitution, and more.
Calculus 2 introduces finding the area between two curves, which involves subtracting areas to find the difference.
Volume of a solid of revolution is calculated by integrating cross sections of the solid, which can be tricky depending on the axis of rotation.
Integration techniques in Calculus 2 include integration by parts, trig integrals, inverse trig substitution, and partial fraction decomposition.
Improper integrals, which involve discontinuities or infinite bounds, are also covered in Calculus 2.
Applications of integration also extend to finding arc length and surface area of curves.
Parametric and polar equations are introduced as different ways to represent functions, allowing for derivatives and integrals in these forms.
Polar equations convert from Cartesian coordinates (x, y) to polar coordinates (r, θ), with their own methods for derivatives and integrals.
Sequences and series are discussed, including the properties of sequences such as boundedness and monotonicity.
Series tests are used to determine the convergence or divergence of various types of series, beyond just geometric series.
Power series are introduced, which involve the variable x and can represent functions as infinite sums.
Taylor and Maclaurin series are used to represent functions as power series, with Taylor series being more general and Maclaurin series being a special case.
The video provides an overview to help students understand the scope of topics covered in Calculus 2 and to prepare them for the challenges ahead.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: