Drug Calculations Made Ridiculously Easy

Synthesis Med Ed
30 Apr 202007:57
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative video, Dr. M introduces a straightforward method for drug calculations called dimensional analysis, emphasizing its intuitive nature and long-term retention. The method involves keeping units in the equation and using conversion factors to simplify calculations. Through practical examples, including pediatric amoxicillin dosing and norepinephrine infusion rates, the video demonstrates how to apply this technique effectively. Dr. M encourages understanding over memorization for accurate and efficient medication calculations in clinical practice.

Takeaways
  • 📚 The video introduces a simple method for drug calculations called dimensional analysis, designed to be more intuitive and memorable for healthcare professionals.
  • 🧠 The key to dimensional analysis is to keep units in the equation, which helps in setting up the math correctly without the need for constant relearning.
  • 🔢 Understanding that multiplying 1 into a number or expression doesn't change its value is fundamental to the method, as is recognizing equivalent fractions that can be used as conversion factors.
  • 🔄 Conversion factors are used to convert between units, like micrograms to milligrams, and can be flipped into their reciprocals without changing the value, aiding in unit cancellation.
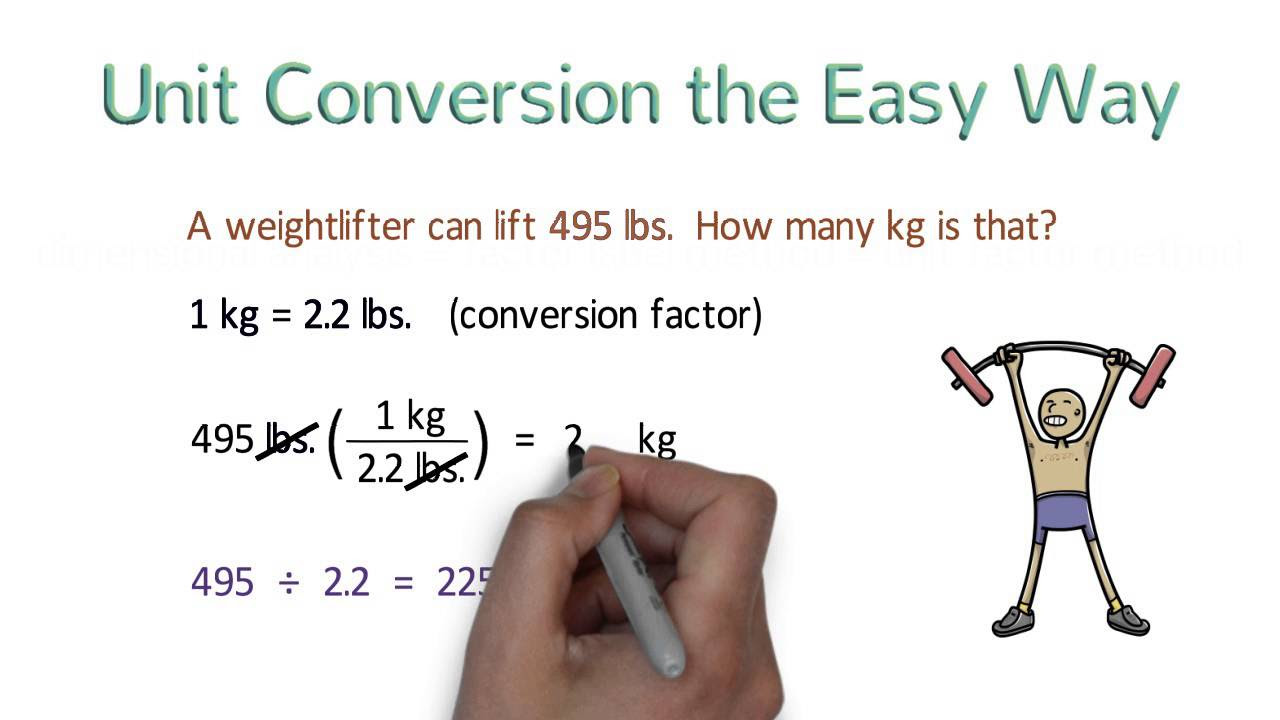
  • 📈 The video demonstrates using dimensional analysis to convert weight from pounds to kilograms, showcasing how to set up and solve the problem step by step.
  • 💊 Dosage calculation examples are provided for pediatric amoxicillin and norepinephrine infusion, emphasizing the importance of using the correct conversion factors and understanding the mathematical process.
  • 📋 When calculating medication dosages, it's crucial to consult drug guides for the most current dosage information, as the examples provided are for illustrative purposes only.
  • 🩺 The method is applicable for various healthcare professionals, including physicians and nurses, for calculating drug dosages and infusion rates at the patient's bedside.
  • 🛠️ Despite the availability of infusion pumps and other tools to perform calculations, it's essential for healthcare professionals to know how to perform these calculations manually.
  • 🎯 The video stresses the importance of setting up the problem correctly using dimensional analysis, ensuring that the final units match the desired outcome.
  • 📖 The ultimate goal is to understand the method so that it can be applied effectively in real-world scenarios, rather than just memorizing formulas.
Q & A

  • What is the main topic of the video?

    -The main topic of the video is teaching a simple and intuitive method for drug calculations called dimensional analysis.

  • Why is it important to learn a method that sticks when it comes to drug calculations?

    -It is important because constantly having to relearn how to perform drug calculations can be inefficient and potentially harmful in a clinical setting. A method that sticks allows healthcare professionals to calculate infusion rates and administer medications accurately and promptly.

  • How does dimensional analysis help in setting up math equations correctly?

    -Dimensional analysis helps by keeping the units in the equation, which assists in ensuring that the mathematical setup is correct and that the units cancel out properly, leading to the desired unit of measurement in the final answer.

  • What is a conversion factor in the context of dimensional analysis?

    -A conversion factor is a fraction that is equivalent to one unit or one thing, used to convert between different units of measurement without changing the overall value of the expression.

  • How can you flip a conversion factor into its reciprocal without changing its value?

    -You can flip a conversion factor into its reciprocal because both the numerator and the denominator are equal, making the fraction equal to 1. This allows for the cancellation of units in the equation without altering the result.

  • In the example given, how is a patient's weight converted from pounds to kilograms?

    -The patient's weight is converted by using the conversion factor that approximately 2.2 pounds is equal to 1 kilogram. The weight in pounds is divided by 2.2 to get the weight in kilograms.

  • How is the dosage of amoxicillin calculated for a pediatric patient in the video example?

    -The dosage is calculated by first determining the daily dose per kilogram (80 mg/kg/day), dividing this by 2 for bid dosing (twice daily), and then using the patient's weight (32 kg) to find the milligrams per dose. The amoxicillin preparation concentration is then used to find the volume in milliliters per dose.

  • What is the purpose of the disclaimer regarding medication dosages in the video?

    -The disclaimer is there to remind viewers that the dosages used in the examples are for illustrative purposes only. Healthcare professionals should always consult their drug guide or current dosage information for accurate and up-to-date recommendations.

  • How does the video demonstrate the use of dimensional analysis for an infusion calculation?

    -The video demonstrates the use of dimensional analysis for an infusion calculation by showing how to set up the problem with the patient's weight, the desired dosage (0.01 mcg/kg/min), and the infusion concentration (8 mg in 250 ml). It then explains how to use conversion factors to cancel out unwanted units and solve for the desired unit (milliliters per hour).

  • What is the key takeaway from the video regarding drug calculations?

    -The key takeaway is to understand and apply the method of dimensional analysis for drug calculations, using conversion factors and cross-cancelling units to set up the problem correctly. This method promotes understanding over memorization, allowing for accurate and efficient calculations in clinical practice.

  • Why is it essential for healthcare professionals to know how to perform drug calculations by hand?

    -It is essential for healthcare professionals to know how to perform drug calculations by hand to ensure they understand the process and can verify the accuracy of calculations, even when using automated systems like infusion pumps.

Outlines
00:00
📚 Introduction to Dimensional Analysis for Drug Calculations

This paragraph introduces the concept of dimensional analysis as a method for drug calculations. It emphasizes the importance of learning a method that sticks, rather than constantly relearning. Dr. M, the speaker, welcomes the audience back to Synthesis Med Ed and explains that dimensional analysis involves keeping units in the equation to set up the math correctly. The speaker reassures the audience of the method's mathematical soundness by discussing the properties of multiplying by 1 and the use of equivalent fractions as conversion factors. The paragraph concludes with an example of using dimensional analysis to convert a patient's weight from pounds to kilograms, demonstrating the method's practical application.

05:02
💊 Applying Dimensional Analysis to Medication Dosages

This paragraph delves into the application of dimensional analysis for calculating medication dosages. It begins with a disclaimer about the illustrative nature of the dosages provided and the necessity of consulting drug guides for current information. The speaker presents two practice problems: the first involves calculating the dosage of high dose amoxicillin for a pediatric patient, and the second focuses on determining the rate of a norepinephrine infusion for a patient in septic shock. Both examples illustrate the step-by-step process of using conversion factors and cross-cancelling units to arrive at the desired units, such as milliliters per dose or per hour. The paragraph concludes with an encouragement to understand the method rather than just memorize it, emphasizing the importance of being able to perform calculations by hand and the intuitive nature of dimensional analysis.

Mindmap
Keywords
💡Dimensional Analysis
Dimensional analysis is a mathematical method used to convert units and solve problems involving physical quantities. In the context of the video, it is introduced as a simpler and more intuitive approach to performing drug calculations, emphasizing the importance of keeping units in the equation to set up the math correctly. The method involves using conversion factors to cancel out unwanted units and solve for the desired unit, such as converting pounds to kilograms or micrograms to milligrams in medical calculations.
💡Conversion Factors
Conversion factors are ratios used to convert one unit of measurement to another. In the video, conversion factors are crucial for dimensional analysis in drug calculations. They are defined as fractions equivalent to one, allowing for the transformation of units without changing the value of the expression. The video provides examples such as 1000 micrograms being equal to 1 milligram, and 2.2 pounds being equal to 1 kilogram, which are used to convert units in the calculations.
💡Cross Canceling
Cross canceling is a technique used in dimensional analysis to simplify the equation by eliminating unwanted units. It involves multiplying the numerator and denominator of a fraction by the reciprocal of another fraction to cancel out common units. This process is essential in solving problems using dimensional analysis, as it helps to isolate the desired unit of measurement. In the video, cross canceling is used to calculate medication dosages and infusion rates by canceling out unnecessary units, leaving behind the required units.
💡Infusion Rate
Infusion rate refers to the speed at which a medication is administered to a patient through an intravenous line. It is typically measured in micrograms per kilogram per minute (mcg/kg/min) or milliliters per hour (mL/h). In the video, calculating the infusion rate for a patient in septic shock is a key example of using dimensional analysis to determine the correct dosage of medication, such as norepinephrine, based on the patient's weight and the required dosing regimen.
💡Amoxicillin
Amoxicillin is a broad-spectrum antibiotic medication used to treat various bacterial infections. In the video, amoxicillin is used as an example to demonstrate how to calculate medication dosages using dimensional analysis. The video provides a scenario where a pediatric patient is diagnosed with otitis media, and the healthcare provider needs to calculate the correct dosage based on the patient's weight and the recommended dosing of 80 mg/kg/day.
💡Norepinephrine
Norepinephrine is a medication used to treat low blood pressure and shock, including septic shock. In the video, norepinephrine is used as an example to explain the process of calculating an infusion rate using dimensional analysis. The video details how to convert the medication's dosing from micrograms per kilogram per minute to a volume of medication to be infused per hour, based on the patient's weight and the medication's concentration.
💡Dosage Calculation
Dosage calculation is the process of determining the correct amount of medication to administer to a patient based on factors such as the patient's weight, the medication's concentration, and the recommended dosing regimen. In the video, dosage calculation is a central theme, with the focus on teaching healthcare providers how to use dimensional analysis to accurately and efficiently calculate medication dosages for their patients.
💡Medical Calculations
Medical calculations are mathematical computations used in healthcare to determine various aspects of patient care, such as drug dosages, fluid management, and monitoring vital signs. In the video, medical calculations are specifically focused on drug calculations, emphasizing the importance of accuracy and understanding the methods used to perform these calculations, such as dimensional analysis.
💡Healthcare Providers
Healthcare providers are professionals who deliver medical care and services to patients. In the video, healthcare providers are the target audience, as the content is designed to educate them on a simpler method for drug calculations that they can apply in their practice. The video aims to equip healthcare providers with the skills to perform accurate and efficient calculations, which are crucial for patient safety and effective treatment.
💡Patient Safety
Patient safety refers to the prevention of harm and ensuring the well-being of patients during the provision of healthcare services. In the video, the emphasis on accurate drug calculations is directly related to patient safety, as incorrect dosages can lead to adverse effects or treatment failures. The video's teaching of dimensional analysis aims to improve healthcare providers' ability to calculate correct dosages, thereby enhancing patient safety.
💡Education
Education in the context of the video refers to the teaching and learning process aimed at improving healthcare providers' knowledge and skills in performing drug calculations. The video serves as an educational resource, providing a detailed explanation of dimensional analysis and its application in medical calculations, with the goal of enhancing understanding and retention of this method among healthcare providers.
Highlights

The introduction of a simple and intuitive method for drug calculations that doesn't require constant relearning.

The method discussed is called dimensional analysis, which keeps units in the equation to set up math correctly.

Dimensional analysis relies on the principle that multiplying 1 into a number or expression doesn't change its value.

A fraction with a numerator and denominator that are the same equals 1 and can be used in equations without altering the value.

Conversion factors are fractions equivalent to one unit that are used to transform units in calculations.

Conversion factors can be flipped into their reciprocal without changing the value, allowing for unit cancellation.

A practical example of using dimensional analysis to convert pounds to kilograms with a patient's weight.

An illustration of calculating medication dosages using dimensional analysis for a pediatric patient with a specific weight.

The importance of using the correct dosage information and consulting drug guides for accurate data.

A detailed example of calculating amoxicillin dosage for a pediatric patient using dimensional analysis.

An explanation of how to set up a prescription using dimensional analysis with a focus on unit cancellation.

A second practice problem involving a patient in septic shock and the calculation of a norepinephrine infusion.

The process of converting complex fractions and units like micrograms to milligrams during drug calculations.

The necessity of understanding how to run drug calculations by hand, even when using infusion pumps.

The emphasis on understanding rather than memorizing to effectively apply the learned method of dimensional analysis.

The conclusion of the session, highlighting the intuitive nature of dimensional analysis and its practical applications.

Transcripts

Browse More Related Video

Unit Conversion the Easy Way (Dimensional Analysis)

Drug Calculations for Nurses Made Easy

IV Infusion Time Calculations Nursing | Dosage Calculations Practice for Nursing Student (Vid 9)

Pediatric Medication Calculations - 4 Step Method Made EASY

Dosage Calculations Nursing Practice Problems & Comprehensive NCLEX Review

Nursing Dosage Calculations - Easier Than You Think! Example Problems 1- 3 | @LevelUpRN

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Dimensional AnalysisDrug CalculationsHealthcare EducationMedication DosageNursing SkillsPharmacy PracticeMedical MathClinical ApplicationEducational VideoHealthcare Professionals