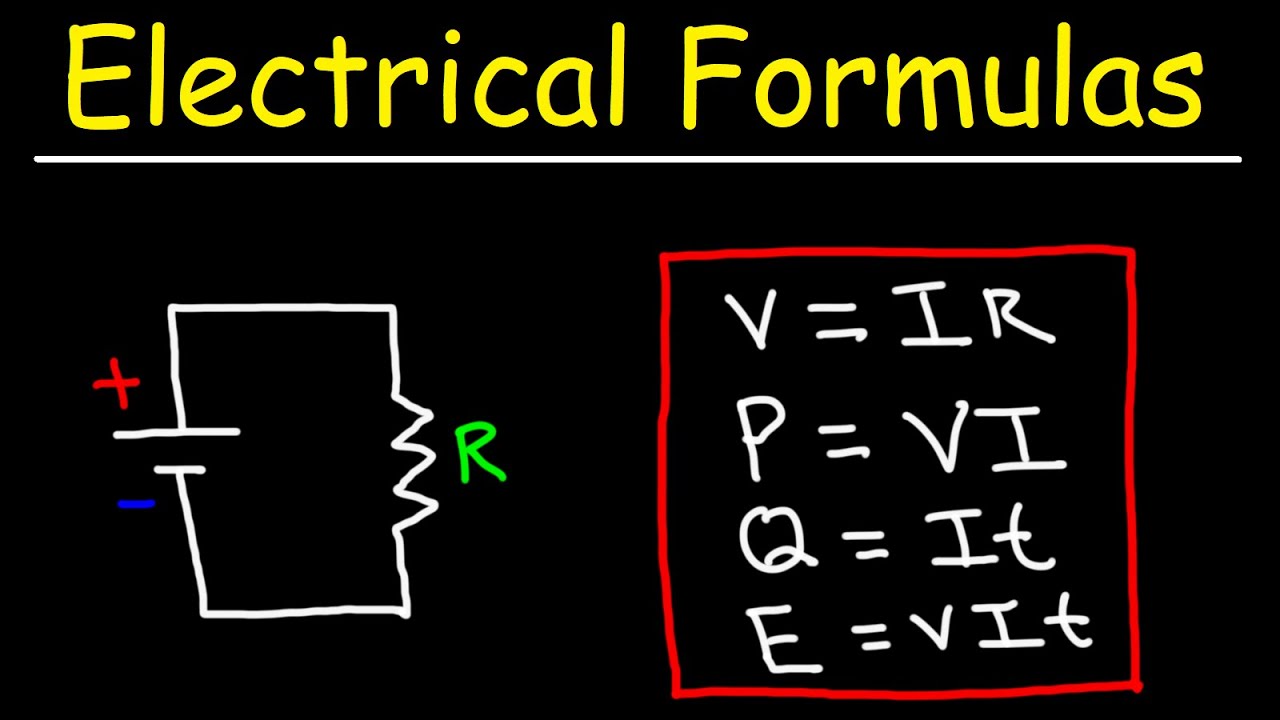
Lesson 1 - Intro To Node Voltage Method (Engineering Circuits)

The paragraph introduces the node voltage method of circuit analysis, emphasizing its importance as a fundamental technique for students studying circuits. It discusses the limitations of relying solely on Kirchhoff's laws, particularly as circuits become more complex with additional branches and resistors. The node voltage method is presented as a more streamlined approach that typically requires fewer equations to solve a circuit, thus making it more efficient and easier to handle. The paragraph also sets the stage for a deeper exploration of the method by providing context from previously learned concepts like voltage, current, resistance, and Ohm's law.

This paragraph delves into the definitions necessary for understanding the node voltage method, such as the concept of a 'node' and the distinction between regular nodes and 'essential nodes.' It uses a simple circuit diagram to illustrate these concepts, clarifying that essential nodes are interconnections of three or more components. The paragraph also explains the process of identifying essential nodes and selecting a reference node, which is crucial for writing node voltage equations. The importance of a structured approach when using the node voltage method is highlighted, contrasting the flexibility of Kirchhoff's laws with the more rigid, defined steps of the node voltage method.

The focus of this paragraph is on the practical steps of the node voltage method, specifically identifying essential nodes within a circuit and choosing a reference node. It explains that essential nodes are the key points of interest when using this method and that the reference node is typically the one with the most connections at the bottom of the circuit diagram. The paragraph reinforces the idea that once the essential nodes are identified and the reference node is chosen, the next step is to write node voltage equations, which will help in solving the circuit. It also touches on the concept of node voltages and how they are measured with respect to the reference node.

This paragraph provides a detailed explanation of how to write node voltage equations for a circuit. It emphasizes the importance of understanding the method's sign conventions and the process of summing currents at a node to zero. The paragraph walks through the process of writing equations for each essential node, considering the currents flowing out of the node and using Ohm's law to express these currents in terms of voltage drops across resistors. It also clarifies that even though the actual current may not flow out from the node, the equations are written assuming that they do, with the understanding that the sign of the resulting values will account for the actual direction of current flow.

The paragraph discusses the process of simplifying and solving the node voltage equations. It explains how to simplify the equations by combining like terms and moving constants to one side to solve for the node voltages. The paragraph then presents the system of equations in matrix form, highlighting the use of matrix methods for solving linear equations, which is particularly useful for circuit analysis. It also mentions the use of calculators or computers to find the inverse of a matrix and solve for the unknown node voltages. The importance of understanding the matrix representation of the equations and the process of solving them is emphasized, as this is a fundamental technique in the field.

Once the node voltages are determined, this paragraph explains how to find other quantities in the circuit, such as currents and voltages across various branches. It emphasizes that with the node voltages known, all other circuit parameters can be calculated. The paragraph also addresses the issue of negative currents, explaining that they indicate the actual direction of current flow is opposite to what was assumed when writing the node voltage equations. The ability to solve the entire circuit after finding the node voltages is highlighted, reinforcing the efficiency of the node voltage method.

The paragraph concludes by encouraging the viewer to understand the node voltage method thoroughly, as it will be applicable even in more complex circuits involving AC, capacitors, and inductors. It reassures that while there may be differences in how the method is applied in these cases, the fundamental concepts will remain the same. The importance of grasping the basics of the node voltage method is stressed, as it is a technique that will be used throughout the study of circuit analysis.