The Ranges of Trigonometric Functions

The Math Sorcerer
15 May 201803:09
EducationalLearning
32 Likes 10 Comments

TLDRThis video delves into the ranges of trigonometric functions, emphasizing their importance in calculus and other math courses. It highlights that sine and cosine are bounded between -1 and 1, while tangent and cotangent can vary across all real numbers. The video also explains the ranges of cosecant and secant based on the reciprocal nature of sine and cosine. Through examples, it demonstrates the possibility or impossibility of certain trig function values, reinforcing the key concept that tangent and cotangent have an unrestricted range, which is particularly crucial for understanding calculus.

Takeaways
  • 📌 The range of the sine function (sin(θ)) is between -1 and 1, which is a crucial identity to remember.
  • 📌 Similarly, the range of the cosine function (cos(θ)) is also between -1 and 1.
  • 📌 The tangent (tan(θ)) and cotangent (cot(θ)) functions can take any real number as their value.
  • 📌 Given that sine's range is between -1 and 1, the cosecant (csc(θ)) function, being the reciprocal of sine, can have values greater than or equal to 1 and less than or equal to -1.
  • 📌 The secant (sec(θ)) function, which is the reciprocal of cosine, follows the same range logic as cosecant, being either greater than or equal to 1 or less than or equal to -1.
  • 📌 The script emphasizes the importance of understanding the ranges of these trigonometric functions, especially for calculus and other math-related studies.
  • 📌 A quick example provided in the script demonstrates that it is impossible for cos(θ) to equal the square root of 3, as this value exceeds the range of cosine.
  • 📌 The script also addresses the possibility of tan(θ) being equal to π/75, confirming that it is possible since tangent's range is all real numbers.
  • 📌 Another example shows that sin(θ) can indeed be equal to 0.02, as it falls within the established range of -1 to 1.
  • 📌 The key takeaway from the video is the versatility of tangent and cotangent functions, whose range encompasses all real numbers, making them particularly useful in calculus.
Q & A

  • What is the range of the sine function?

    -The range of the sine function is between -1 and 1, inclusive.

  • Is it possible for the cosine function to have a value outside the interval [-1, 1]?

    -No, the cosine function also has a range between -1 and 1, inclusive.

  • What is unique about the range of the tangent function compared to sine and cosine?

    -The tangent function can take any real number as its value, unlike sine and cosine which are bounded between -1 and 1.

  • How does the range of the cotangent function differ from that of the tangent?

    -The cotangent function, being the reciprocal of the tangent, can also take any real number as its value.

  • What is the range of the cosecant function considering the range of the sine function?

    -Since cosecant is the reciprocal of sine, its range is all real numbers except for 0, as dividing by zero is undefined.

  • Similarly, what can we infer about the range of the secant function?

    -The secant function, being the reciprocal of the cosine function, will also have a range excluding 0, as the cosine function cannot be zero.

  • Why is the range of the tangent function particularly useful in calculus?

    -The unbounded range of the tangent function makes it especially useful in calculus for analyzing functions that can approach or diverge to infinity.

  • If the cosine of theta is equal to the square root of 3, is this statement possible?

    -No, since the cosine function cannot exceed 1 in absolute value, and the square root of 3 is greater than 1, this statement is impossible.

  • Is it possible for the tangent of theta to equal PI/75?

    -Yes, since the tangent function can take any real number as its value, it is possible for the tangent of theta to equal PI/75.

  • Can the sine of theta be equal to 0.02?

    -Yes, as 0.02 is a number between -1 and 1, it is within the range of possible values for the sine function.

  • What should one remember as a key takeaway from this discussion on trigonometric function ranges?

    -The key takeaway is that the tangent and cotangent functions can take any real number as their values, which is particularly important for understanding their behavior in calculus and other mathematical contexts.

Outlines
00:00
📚 Introduction to Trigonometric Functions' Ranges

This paragraph introduces the concept of ranges for trigonometric functions, emphasizing the importance of understanding these ranges for calculus and other math courses. It highlights the key identity that the sine of theta (sin(θ)) and cosine of theta (cos(θ)) both range between -1 and 1. The tangent (tan(θ)) and cotangent (cot(θ)) functions are noted to have a range of all real numbers. The video then sets up a framework for determining the possible values of related trigonometric functions like cosecant (csc(θ)) and secant (sec(θ)), which are reciprocals of sine and cosine, respectively, and thus have ranges that exclude -1 and 1.

Mindmap
Keywords
💡Trigonometric Functions
Trigonometric functions are mathematical functions that relate the angles of a right triangle to the lengths of its sides. In the context of the video, these functions are crucial for understanding calculus and other mathematical concepts. The video focuses on the sine, cosine, tangent, cotangent, cosecant, and secant functions, discussing their ranges and properties.
💡Ranges
In mathematics, the range of a function is the set of possible output values (y-values) that result from the input values (x-values). The video emphasizes the importance of understanding the ranges of trigonometric functions, especially for those relevant to calculus and other advanced math classes.
💡Sine (sin)
The sine function, often abbreviated as sin, is a fundamental trigonometric function that represents the ratio of the length of the side opposite an angle to the length of the hypotenuse in a right triangle. The video highlights that the sine function's range is between -1 and 1, which is a critical piece of information for solving mathematical problems.
💡Cosine (cos)
The cosine function, denoted as cos, is another fundamental trigonometric function that represents the ratio of the length of the adjacent side to the length of the hypotenuse in a right triangle. The video emphasizes that the cosine function, like sine, has a range between -1 and 1.
💡Tangent (tan)
The tangent function, written as tan, is a trigonometric function that represents the ratio of the length of the side opposite an angle to the length of the adjacent side in a right triangle. Unlike sine and cosine, the tangent function can take any real number as its value, which is a key point discussed in the video.
💡Cotangent (cot)
Cotangent, often denoted as cot or 1/tan, is the reciprocal of the tangent function. It represents the ratio of the length of the adjacent side to the length of the side opposite an angle in a right triangle. The video notes that cotangent, like tangent, can be any real number.
💡Cosecant (csc)
Cosecant, abbreviated as csc or 1/sin, is the reciprocal of the sine function. It is the ratio of the length of the hypotenuse to the length of the side opposite an angle in a right triangle. The video explains that since sine has a range between -1 and 1, the cosecant can be less than or equal to -1 or greater than 1.
💡Secant (sec)
Secant, denoted as sec or 1/cos, is the reciprocal of the cosine function. It represents the ratio of the length of the hypotenuse to the length of the side adjacent to an angle in a right triangle. The video indicates that the secant, like the cosecant, can be less than or equal to -1 or greater than 1, based on the range of the cosine function.
💡Reciprocal
A reciprocal is a mathematical term that refers to the inverse of a number or function. When the video discusses the cosecant and secant as reciprocals of sine and cosine respectively, it means that their values are obtained by taking the reciprocal (1 divided by the function value) of the sine and cosine functions.
💡Key Identities
Key identities in trigonometry are fundamental relationships between trigonometric functions that are essential for solving problems and simplifying expressions. The video script introduces the key identity that the sine of theta is between -1 and 1, which is crucial for understanding the behavior of trigonometric functions in various mathematical contexts.
💡Calculus
Calculus is a branch of mathematics that deals with the study of change and motion, focusing on concepts such as limits, derivatives, and integrals. The video mentions that understanding the ranges of trigonometric functions is particularly important for the study of calculus, as these functions are frequently used in calculus computations.
💡Math Classes
Math classes refer to educational courses that teach various topics in mathematics. The video script implies that the understanding of trigonometric functions and their ranges is not only crucial for calculus but also for other math classes, indicating the broad applicability of these concepts across different areas of mathematics.
Highlights

The video discusses the ranges of trigonometric functions, which is crucial for calculus and other math classes.

A key identity to remember is that the sine of theta (sin(θ)) lies between -1 and 1.

Similarly, the cosine of theta (cos(θ)) also ranges between -1 and 1.

Tangent (tan(θ)) and cotangent (cot(θ)) can take any real number as their value.

The range of tangent and cotangent is all real numbers.

The reciprocal of sine, cosecant (csc(θ)), will be less than or equal to -1 or greater than 1.

Secant (sec(θ)), like cosecant, is either less than or equal to -1 or greater than 1.

The video presents a method to determine whether a trigonometric equation is possible or impossible based on the ranges of the functions.

For example, cosine of theta cannot equal the square root of 3 since it is impossible for cos(θ) to be greater than 1.

Tangent of theta can equal π/75 because tan(θ) can be any real number, making this equation possible.

It is possible for sine of theta to equal 0.02, as it lies within the range of -1 to 1.

The most important takeaway is that tangent and cotangent can vary across the entire spectrum of real numbers.

The video emphasizes the practical applications of these ranges, particularly in calculus.

Understanding the ranges of trig functions is essential for solving mathematical problems and equations.

The video provides a clear and concise explanation of the ranges of sine, cosine, tangent, cotangent, cosecant, and secant.

The reciprocal nature of cosecant and secant in relation to sine and cosine is highlighted.

The video uses the concept of ranges to solve simple questions about trigonometric functions.

The video's content is designed to help viewers understand and apply the ranges of trig functions in various mathematical contexts.

Transcripts

Browse More Related Video

Trig - 0.3 Domains of Trigonometric Functions

Basic Properties of Trigonometric Functions (Precalculus - Trigonometry 8)

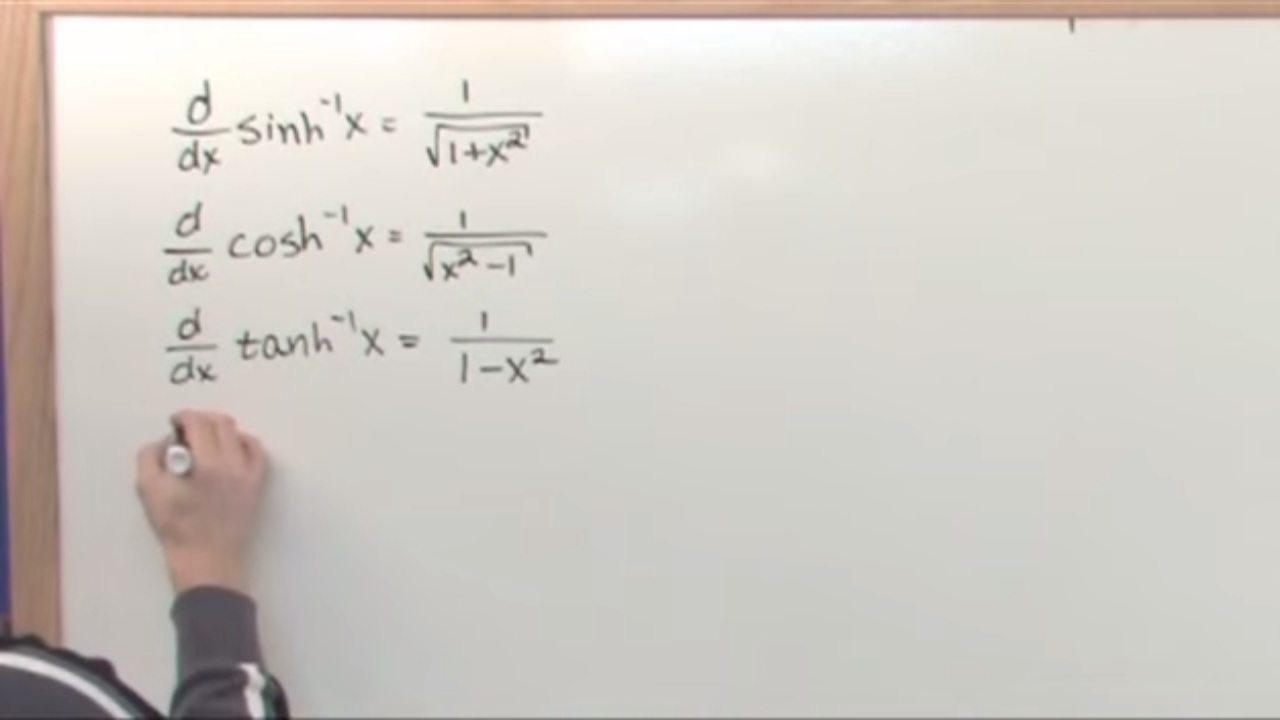
Lesson 4 - Inverse Hyperbolic Functions (Calculus 2 Tutor)

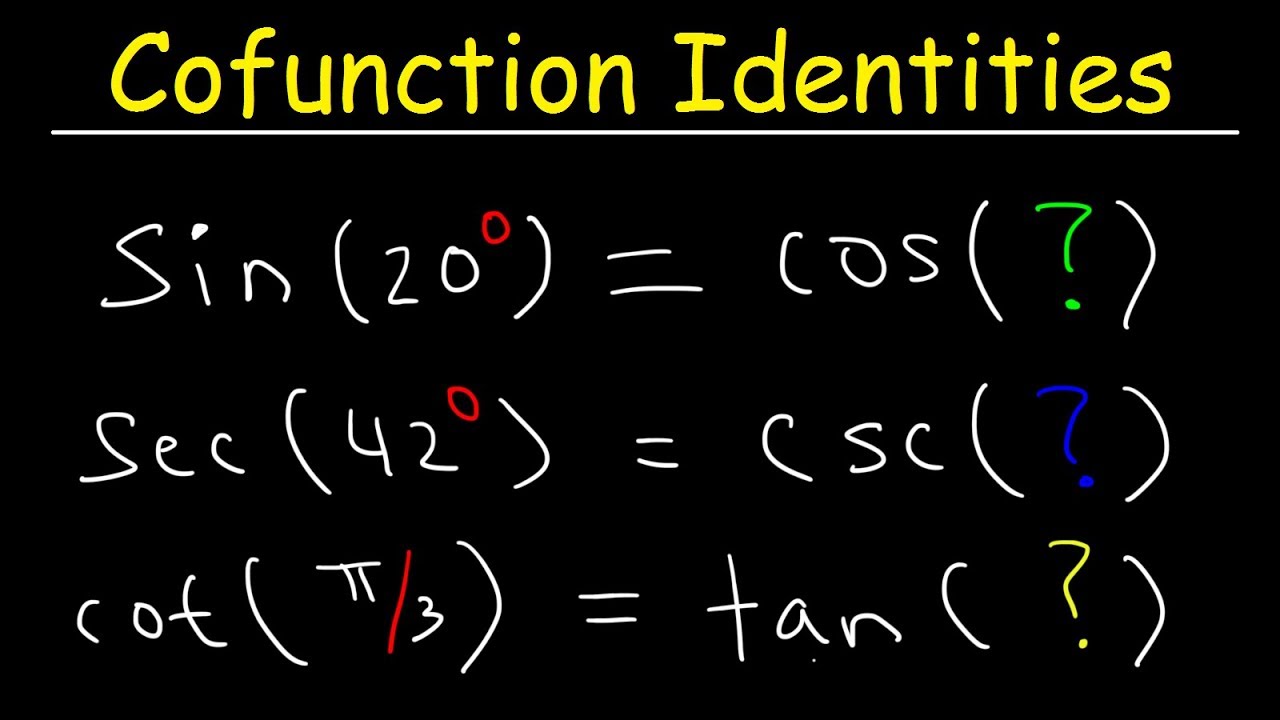
Cofunction Identities Examples & Practice Problems Trigonometry

Trig - 0.6 Trig Functions of Acute Angles

Derivatives of Trigonometric Functions

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
TrigonometryCalculusMathematicsSineFunctionCosineFunctionTangentFunctionCotangentFunctionRangeAnalysisEducationalVideoContent