03 - What is Ohm's Law in Circuit Analysis?
TLDRThis transcript delves into the fundamental concept of Ohm's Law in electric circuit analysis. It explains the relationship between current, voltage, and resistance, and how Ohm's Law serves as the cornerstone for understanding and analyzing circuits. The video uses simple circuits with varying resistances and voltages to illustrate the law's application and emphasizes the importance of recognizing voltage drops across resistors. It also introduces the concept of a voltage divider and its practical applications. The lesson underscores the need to grasp Ohm's Law for tackling more complex circuit analysis methods in the future.
Takeaways
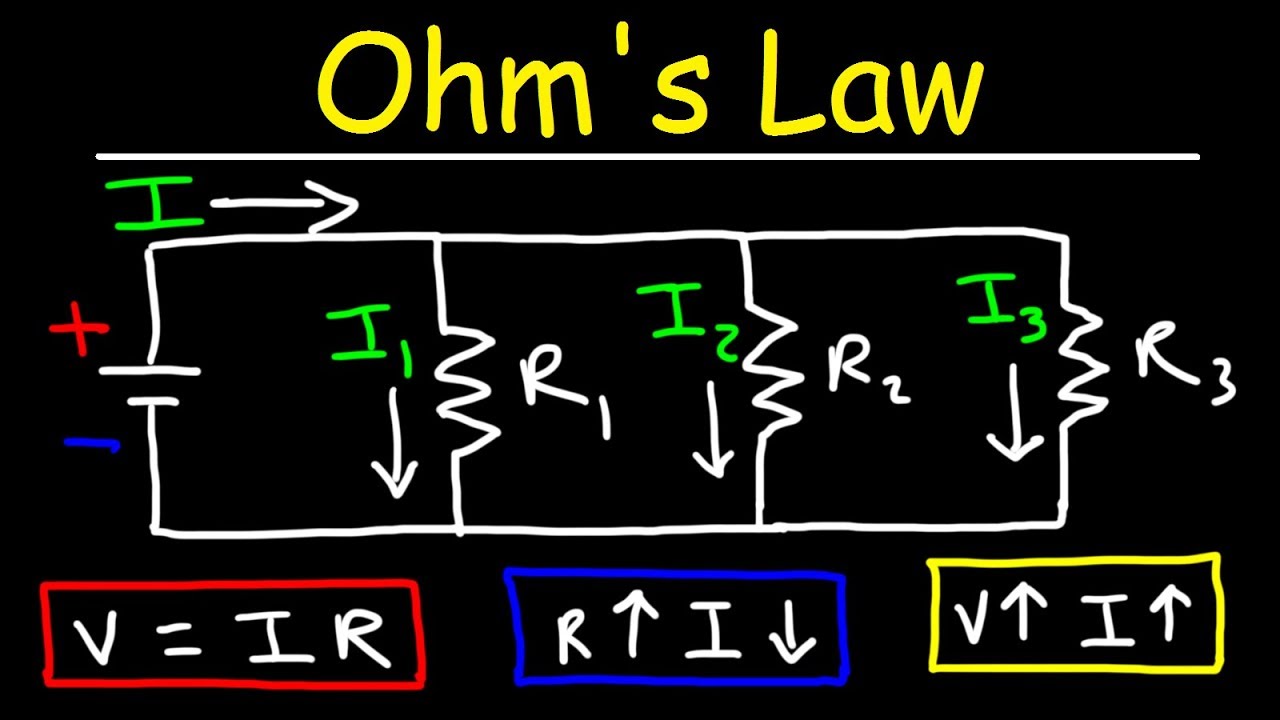
- 📚 Ohm's Law is a fundamental principle in electric circuits, relating current (I), voltage (V), and resistance (R) with the formula V = IR.
- 🔌 The current flowing through a circuit element is the same throughout a series circuit, as it is a continuous path.
- 🏗️ In a series circuit, the total resistance is the sum of the individual resistances (R_total = R1 + R2 + ...).
- 💡 Voltage across a resistor in a series circuit can be calculated using Ohm's Law (V = IR), where V is the voltage drop, I is the current, and R is the resistance.
- 🔄 The sum of all voltage drops across resistors in a series circuit equals the source voltage, following the principle of conservation of energy.
- 📈 The relationship between voltage and current is directly proportional, meaning an increase in voltage results in an increase in current, and vice versa.
- 📉 The relationship between resistance and current is inversely proportional, meaning an increase in resistance results in a decrease in current, and vice versa.
- 🔧 When analyzing circuits, it's essential to consider the specific element of interest and apply Ohm's Law accordingly.
- 🛠️ Unit conversion is crucial when dealing with different units of measurement, such as milliamps (mA) and millivolts (mV), to ensure accurate calculations.
- 🔌 Voltage dividers are practical circuits that split a source voltage across a series of resistors to provide different voltage levels for various parts of a circuit.
- 📊 Understanding and applying Ohm's Law is the foundation for more advanced circuit analysis techniques, such as node voltage and mesh current methods.
Q & A
What is Ohm's Law and how does it relate to electric circuits?
-Ohm's Law is a fundamental principle in circuit analysis that defines the relationship between current (I), voltage (V), and resistance (R) in an electrical circuit. It is given by the formula V = IR, which means that the voltage across a conductor is directly proportional to the current flowing through it and the resistance of the conductor. This law serves as the foundation for understanding how electrical components behave in a circuit and is essential for analyzing more complex circuits.
How does the concept of voltage act as a pushing force in a circuit?
-Voltage acts as a pushing force in a circuit by providing the electrical potential difference needed for electrons to move from a point of higher potential energy to a point of lower potential energy. This movement of electrons constitutes an electric current. The greater the voltage, the stronger the pushing force, resulting in a higher current flow through the circuit. Conversely, if the voltage is reduced, the current flow decreases as there is less force to push the electrons through the circuit.
What is the role of resistance in a circuit and how does it affect the flow of current?
-Resistance is a property of the materials and components in a circuit that opposes or resists the flow of electric current. It acts as a barrier to the movement of electrons, slowing down the current. In Ohm's Law, resistance (R) is the denominator, so an increase in resistance leads to a decrease in current (I) for a given voltage (V), as described by the formula V = IR. Conversely, a decrease in resistance allows for an increase in current flow.
How can you calculate the current flowing through a resistor using Ohm's Law?
-To calculate the current (I) flowing through a resistor using Ohm's Law, you can rearrange the formula to solve for I, which gives I = V/R. Here, V represents the voltage across the resistor, and R is the resistance value of the resistor. By knowing the voltage drop across the resistor and its resistance, you can easily determine the current flowing through it.
What is the significance of voltage drop across a resistor in a circuit?
-The voltage drop across a resistor is the difference in electrical potential that occurs as current flows through it. This voltage drop is a result of the work done by the electric field in moving the electrons against the resistive force of the resistor. It is significant because it represents the energy loss or conversion in the resistor, which can be converted into heat or other forms of energy. The voltage drop also plays a crucial role in analyzing circuits, as it helps in determining the current flow and understanding the distribution of voltage in a circuit.
How does the current behave in a simple series circuit with multiple resistors?
-In a simple series circuit with multiple resistors, the current (I) flows through each resistor one after the other, returning to the source. The same current value flows through all components in series because there is only one path for the current to take. However, the voltage drop (V) across each resistor can be different, depending on the resistance value of each resistor. The sum of the voltage drops across all resistors in series equals the total voltage supplied by the source, in accordance with the principle of conservation of energy.
What is a voltage divider and how is it used in practice?
-A voltage divider is a simple circuit configuration consisting of two or more resistors connected in series across a voltage source. It is used to reduce or divide the source voltage into smaller values. The voltage drop across each resistor in the series is proportional to its resistance value. This configuration is practical when a portion of the circuit requires a voltage lower than the source voltage. By connecting a resistor of appropriate value in series, the voltage divider can provide the necessary lower voltage to the circuit without the need for additional voltage regulation components.
How does the concept of unit conversion apply to Ohm's Law calculations?
-When applying Ohm's Law, it is crucial to use consistent units for voltage (V), current (I), and resistance (R). If the given values are in different units, such as millivolts or milliamps, they must be converted to their base units (volts and amps) before performing calculations. For example, millivolts (mV) can be converted to volts (V) by multiplying by 10^-3, and milliamps (mA) can be converted to amps (A) by the same factor. This ensures that the calculation is accurate and that the resulting values are in the correct units.
What is the significance of the sign convention for voltage drops across resistors?
-The sign convention for voltage drops across resistors is important for understanding the direction of current flow and the potential energy changes within the circuit. By convention, the voltage drop is considered positive to negative across a resistor, following the direction of the current flow. This means that as current flows through a resistor, it loses potential energy, resulting in a voltage drop. This convention helps in visualizing the energy transfer within the circuit and is crucial for circuit analysis and troubleshooting.
How does the resistance value affect the voltage drop across a resistor?
-The resistance value directly influences the voltage drop across a resistor. According to Ohm's Law, the voltage drop (V) across a resistor is the product of the current (I) flowing through it and its resistance (R), as given by V = IR. Therefore, for a given current, a higher resistance value will result in a larger voltage drop, and vice versa. This relationship is useful for designing circuits where specific voltage levels are required or when analyzing the behavior of circuits under varying resistance conditions.
What is the relationship between the voltage supplied by the source and the voltage drops across components in a series circuit?
-In a series circuit, the total voltage supplied by the source is equal to the sum of the voltage drops across all the components connected in series. This is a direct application of the conservation of energy principle, where the energy provided by the source is distributed among the components in proportion to their resistance values. Each resistor in the series will have a voltage drop across it, and when these voltage drops are added together, they should equal the source voltage. This relationship is crucial for circuit analysis and for ensuring that the correct voltage levels are achieved across different parts of the circuit.
Outlines
📚 Introduction to Ohm's Law
This paragraph introduces the fundamental concept of Ohm's Law, which is a critical aspect of electric circuit analysis. It explains the relationship between current, voltage, and resistance, and how Ohm's Law serves as the foundation for understanding these elements in a circuit. The importance of Ohm's Law is emphasized, and the basic formula (V=IR) is introduced, with a discussion on how it applies to every element in a circuit. The paragraph sets the stage for a deeper exploration of circuit analysis and the practical application of Ohm's Law in simple circuits.
🔌 Applying Ohm's Law to Simple Circuits
The second paragraph delves into the practical application of Ohm's Law in simple circuits. It discusses how changes in voltage and resistance affect the current flowing through a circuit, using a 10-volt source and a 5-ohm resistor as an example. The concept of voltage drop across a resistor is introduced, and the importance of understanding this relationship is highlighted for building a strong foundation in circuit analysis. The paragraph emphasizes the direct relationship between voltage and current and the inverse relationship between resistance and current, as dictated by Ohm's Law.
🌟 Understanding Voltage Drops in Circuits
This paragraph focuses on the concept of voltage drops across passive components in a circuit. It explains that every resistor in a circuit will have a voltage drop, which is governed by Ohm's Law. The analogy of potential energy is used to help visualize the concept of voltage drops, comparing the voltage across a resistor to the potential energy at different points on a mountain. The paragraph emphasizes the importance of recognizing that voltage drops are always from positive to negative across a resistor and that these drops add up to the source voltage in a series circuit.
🔄 Exploring Series Circuits and Voltage Division
The fourth paragraph discusses the behavior of voltage in series circuits, introducing the concept of voltage division. It uses the example of a 12-volt source connected in series with a 1-ohm and a 2-ohm resistor, and explains how the total voltage supplied by the source is divided across the resistors. The paragraph highlights the conservation of energy principle, explaining that the sum of the voltage drops across the resistors must equal the source voltage. The practical application of this principle in creating a voltage divider is also discussed, showing how it can be used to supply a specific voltage to a part of a circuit that requires a different voltage than the source.
📈 Ohm's Law in Circuit Analysis
The final paragraph summarizes the key points discussed in the previous sections, reinforcing the importance of Ohm's Law in circuit analysis. It reiterates that Ohm's Law can be applied to individual elements in a circuit to calculate the current, and that the voltage across any element can be determined if the resistance and current are known. The paragraph also emphasizes the necessity of understanding voltage drops and how they add up to the source voltage in series circuits. The analogy of the mountain is used once more to illustrate the concept of voltage drops and the conservation of energy. The paragraph concludes by encouraging the learner to internalize these concepts as they form the basis for more advanced methods of circuit analysis.
Mindmap
Keywords
💡Ohm's Law
💡Voltage
💡Current
💡Resistance
💡Circuit Analysis
💡Series Circuit
💡Voltage Drop
💡Algebraic Equation
💡Passive Components
💡Power Supply
💡Unit Conversion
Highlights
Ohm's Law is introduced as the fundamental relation between current, voltage, and resistance in electric circuits.
Ohm's Law is mathematically expressed as V=IR, where V represents voltage, I represents current, and R represents resistance.
The concept of current is described as the flow of electricity in a circuit.
Voltage is explained as the pushing force that drives the current around the circuit.
Resistance is defined as the opposition to the flow of current in a circuit.
The importance of Ohm's Law as a foundational concept for understanding and analyzing electric circuits is emphasized.
Simple circuits are used to demonstrate the application of Ohm's Law in calculating voltage and current.
The relationship between increasing voltage and increasing current is discussed, as well as the inverse relationship between resistance and current.
The concept of voltage drop across a resistor is introduced, explaining how it is determined by the current flowing through the resistor and its resistance value.
The significance of Ohm's Law in analyzing every component in a circuit is highlighted, with the law being universally applicable.
The transcript provides a clear explanation of how to use Ohm's Law to calculate the current in a circuit, given the voltage and resistance values.
An analogy is used to relate voltage to potential energy from physics, helping to visualize the concept of voltage drop across resistors.
The transcript explains the practical application of voltage dividers, showing how they can be used to control voltage in a circuit.
The importance of understanding voltage drops across resistors and how they add up to the source voltage is emphasized for later analysis of more complex circuits.
Unit conversion is discussed, emphasizing the need to use base units of volts, ohms, and amps when applying Ohm's Law.
The transcript provides a step-by-step guide on how to apply Ohm's Law to individual elements within a circuit to calculate voltage and current.
The concept of series resistors and their impact on voltage distribution is introduced, with a practical example of a 12-volt source split across two resistors.
The lesson concludes with a summary of Ohm's Law's importance in circuit analysis, setting the foundation for more advanced topics.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: