Kirchhoff's Voltage Law - KVL Circuits, Loop Rule & Ohm's Law - Series Circuits, Physics

The Organic Chemistry Tutor

13 Dec 201723:48

EducationalLearning

32 Likes 10 Comments

TLDRThe video script explains Kirchhoff's Voltage Law (KVL), a fundamental principle in electrical circuits stating that the sum of voltages in a closed loop must equal zero. It illustrates how to determine positive and negative voltages across components like resistors and batteries, which either consume or add energy to the circuit. The script provides examples of applying KVL to calculate current and electric potential in various circuit configurations, emphasizing the importance of understanding energy conservation in circuit analysis.

Takeaways

📜 Kirchhoff's Voltage Law (KVL) states that the sum of voltages in a closed circuit must equal zero.
🔋 A battery increases the energy of charges in a circuit when current flows from low potential to high potential, resulting in a positive voltage.
⚡ A resistor consumes energy, causing a voltage drop, and is associated with a negative voltage when current flows from high to low potential.
🔄 In a series circuit, the current is the same through all components, allowing the use of Ohm's Law (V=IR) to calculate current and voltage drops.
🔌 When applying KVL, the direction of current flow is crucial in determining whether a voltage is positive (energy increasing) or negative (energy decreasing).
📊 To calculate the electric potential at various points in a circuit, one must consider the voltage drops across resistors and the potential differences provided by batteries.
🔄 In a circuit with multiple batteries, the overall direction of current flow is determined by the combined effect of all batteries.
🔧 When two batteries have potential differences in the same direction, they support each other; if in opposite directions, they oppose each other.
📈 To solve complex circuits, identify which devices increase and decrease energy, and apply KVL to ensure the sum of all voltages equals zero.
🔩 In a circuit with multiple components, the voltage drop across a resistor can be calculated using the formula V = I * R, where V is the voltage drop, I is the current, and R is the resistance.
🔄 Understanding the relationship between energy and voltage is fundamental to solving problems involving Kirchhoff's Voltage Law.

Q & A

What does Kirchhoff's Voltage Law (KVL) state?
-Kirchhoff's Voltage Law states that in a closed circuit, the voltages around that circuit must add up to zero.
How does a resistor affect the voltage in a circuit?
-A resistor consumes energy from the charges flowing through it, leading to a voltage drop. Therefore, a resistor always has a negative voltage sign assigned to it, indicating the reduction in energy per unit charge.
How does a battery contribute to the voltage in a circuit?
-A battery can either increase or decrease the energy of the circuit depending on the direction of the current flow. If the current flows from low potential to high potential, the battery increases the energy (positive voltage). If the current flows from high potential to low potential, the battery decreases the energy (negative voltage).
What is the relationship between voltage and energy?
-Voltage is essentially the energy change per unit charge. One volt is equivalent to one joule per coulomb, which means it represents the amount of energy transferred to or from the charges in a circuit.
How can you determine the direction of current flow in a circuit?
-Current naturally flows from high potential to low potential in a resistor. This means that it flows from positive to negative, and this direction is used to determine the positive and negative voltages in the circuit.
What is a series circuit and how does it relate to KVL?
-A series circuit is one where there is only one path for the current to flow. In such a circuit, the current is the same through all components. KVL is used to calculate the current in a series circuit by ensuring the sum of all voltages, including voltage drops and increases, equals zero.
How do you calculate the current in a circuit using KVL?
-By setting up an equation based on KVL where the sum of all voltages (including voltage drops across resistors and the voltage supplied by batteries) equals zero, you can solve for the current. This involves knowing the resistances and the voltages of the batteries in the circuit.
How do you calculate the electric potential at different points in a circuit?
-You can calculate the electric potential at different points by using Ohm's Law (V = IR) and considering the voltage drops across resistors and the potential differences provided by batteries. The potential at a point is the sum of the potential differences from the reference point to that point.
What happens when two batteries are connected in a circuit with opposite polarities?
-When two batteries have opposite polarities, they oppose each other. The direction of the current is determined by the battery with the higher voltage. The batteries with the lower voltages will have their current direction countered and will not contribute effectively to the circuit.
In a circuit with multiple batteries and resistors, how does the current direction affect the overall voltage calculation?
-The current direction affects the overall voltage calculation by determining which components are increasing or decreasing the energy in the circuit. A positive current direction indicates an increase in energy (positive voltage for batteries, negative voltage for resistors), while a negative current direction indicates a decrease in energy (negative voltage for batteries, positive voltage for resistors).
What is the key to solving complex circuit problems using KVL?
-The key to solving complex circuit problems using KVL is understanding which devices increase the energy of the circuit (batteries with current flowing from low to high potential) and which devices decrease the energy (resistors and batteries with current flowing from high to low potential). This understanding helps in correctly assigning positive or negative voltage values to each component and setting up the KVL equation to solve for the unknowns.

Outlines

00:00

🔋 Introduction to Kirchhoff's Voltage Law (KVL)

This paragraph introduces Kirchhoff's Voltage Law (KVL), a fundamental principle in electrical circuits stating that the sum of voltages in a closed loop must equal zero. It explains the concept of positive and negative voltages associated with energy transfer in a circuit. The paragraph uses the example of a resistor and a battery to illustrate how voltages are assigned based on the direction of current flow and the role of these components in energy consumption or generation. The resistor is associated with a negative voltage as it consumes energy, while the battery is associated with a positive voltage when it adds energy to the circuit.

05:01

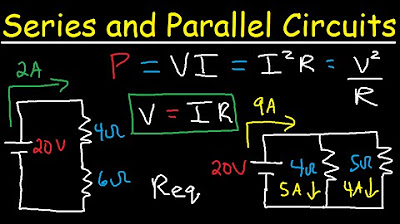
📊 Applying KVL to a Series Circuit with Resistors and Battery

This paragraph delves into the application of KVL in a series circuit consisting of a 12-volt battery and four resistors with values of 8 ohms, 10 ohms, and 12 ohms. It explains how to set up an equation based on KVL to calculate the current flowing through the circuit. The paragraph also details the process of calculating the voltage drop across each resistor using Ohm's Law and how to determine the electric potential at various points in the circuit. The example provided helps to reinforce the understanding of KVL and its practical application in circuit analysis.

10:02

🔄 Working with a Circuit Containing Two Batteries of Different Voltages

This paragraph presents a scenario where a circuit includes two batteries with different voltages, a 12-volt and an 8-volt battery, and two resistors of 50 ohms and 30 ohms. It explains how to determine the direction of the current in the presence of multiple power sources and how to apply KVL to calculate the current in the circuit. The paragraph further illustrates how to calculate the electric potential at different points in the circuit, emphasizing the concept of energy increase or decrease due to the current flow direction relative to the battery terminals.

15:04

🌐 Complex Circuit Analysis with Multiple Batteries and Resistors

The paragraph discusses a more complex circuit with a 50-volt battery, 30-ohms and 70-ohms resistors, and two other batteries with 10 volts and 20 volts each. It explains the process of determining the overall direction of the current based on the voltages and connections of the batteries. The paragraph then applies KVL to calculate the current in the circuit and proceeds to determine the electric potential at various points. The explanation highlights the interplay between the energy contribution of the batteries and the energy consumption by the resistors, reinforcing the understanding of KVL in analyzing complex circuits.

20:05

🎓 Summary and Understanding KVL in Circuit Analysis

In conclusion, this paragraph summarizes the key concepts and techniques discussed in the video script. It reiterates the importance of understanding the energy dynamics in a circuit, where resistors always decrease the energy (negative voltage) and batteries can either increase or decrease the energy based on the current flow direction. The paragraph emphasizes the practical application of KVL in solving a variety of circuit problems, from simple to complex, and encourages the viewer to apply these concepts to further their comprehension of electrical circuits.

Mindmap

Keywords

💡Kirchhoff's Voltage Law (KVL)

KVL is a fundamental principle in electrical circuit analysis which states that the algebraic sum of all the voltages (potential differences) around a closed loop in a circuit is zero. This law is based on the conservation of energy, indicating that the total energy gained in a circuit must equal the total energy lost. In the context of the video, KVL is used to solve for unknown voltages and currents in a circuit, as demonstrated through various examples.

💡Voltage

Voltage is the electrical potential difference between two points in a circuit, often measured in volts (V). It represents the energy per unit charge and is the driving force that pushes electric charge through a circuit. In the video, voltage is used to describe the energy change as current flows through resistors and batteries, with positive voltage indicating an increase in energy and negative voltage indicating a decrease.

💡Resistor

A resistor is a passive component in an electrical circuit that restricts the flow of electric current, converting electrical energy into heat. In the context of the video, resistors always create a voltage drop, meaning they consume energy, and are assigned a negative voltage in the circuit analysis.

💡Current

Current is the flow of electric charge in a circuit, typically measured in amperes (A). It is the rate at which charge flows through a conductor, and its direction is conventionally defined as flowing from a region of higher potential to a region of lower potential. In the video, current is used to calculate the voltage drop across resistors using Ohm's law (V=IR) and to determine the direction of flow in the circuit, which is essential for applying KVL.

💡Ohm's Law

Ohm's Law is a vital relationship in electrical engineering that states that the current through a conductor between two points is directly proportional to the voltage across the two points and inversely proportional to the resistance between them, expressed as V=IR. This law is fundamental for analyzing the behavior of resistors in a circuit.

💡Electric Potential

Electric potential, also known as voltage, is the amount of electric potential energy per unit charge in an electric field. It represents the work done to move a unit charge from a reference point to a specific point in the field without producing any acceleration. In the video, electric potential is used to determine the potential difference across components and to calculate the potential at various points in the circuit.

💡Circuit

An electrical circuit is a path or interconnected network in which electric current can flow. It typically consists of components such as resistors, capacitors, inductors, and power sources like batteries. Circuits can be simple or complex, and they facilitate the flow of current to perform various functions.

💡Energy

In the context of the video, energy refers to electrical energy, which is the capacity to do work through the movement of electric charges in a circuit. Energy is transferred between the charges and circuit components like resistors and batteries, resulting in either an increase or decrease in the energy of the charges.

💡Potential Difference

Potential difference is the change in electric potential between two points in an electric field or circuit. It is the work done per unit charge to move a charge from one point to another and is typically measured in volts (V). In the video, potential difference is used to describe the voltage drop across resistors and the voltage supplied by batteries.

💡Series Circuit

A series circuit is a type of electrical circuit where all the components, such as resistors, are connected end-to-end in a single path so that the same current flows through each component. This configuration means that the total resistance is the sum of the individual resistances, and the voltage drop across each component adds up to the total voltage supplied by the power source.

💡Conservation of Energy

The principle of conservation of energy is a fundamental concept in physics stating that energy cannot be created or destroyed, only transferred or converted from one form to another. In electrical circuits, this principle is reflected in Kirchhoff's Voltage Law, which ensures that the total energy gained in a circuit equals the total energy lost, with the sum of voltages around a closed loop being zero.

Highlights

Kirchhoff's Voltage Law (KVL) states that the sum of voltages in a closed circuit must equal zero.

Voltages can be positive or negative depending on whether they increase or decrease the energy of charges in the circuit.

Resistors consume energy, leading to a voltage drop, and are assigned a negative voltage.

Batteries increase the energy of charges when current flows from low to high potential, resulting in a positive voltage.

In a series circuit, the current is the same throughout, simplifying the application of KVL.

Ohm's Law (V=IR) is used to calculate the voltage drop across resistors and the current in the circuit.

The electric potential at different points in a circuit can be calculated using the voltage drops and gains from the components.

When two batteries have the same polarity direction, they support each other and the current flows in that direction.

If batteries oppose each other, the one with the higher voltage will determine the direction of the current.

KVL ensures that the sum of all voltages in a closed circuit, including those from multiple batteries and resistors, add up to zero.

The direction of current flow is crucial in determining whether a component increases or decreases the energy of the circuit.

The potential difference between two points in a circuit can be found by considering the voltage drops and gains of the components in the path.

This video serves as a basic introduction to understanding and applying Kirchhoff's Voltage Law in circuit analysis.

Practicing with KVL and Ohm's Law allows for the calculation of current, voltage drops, and electric potential across various circuit components.

Understanding the energy transfer within a circuit is key to solving more complex problems in circuit analysis.

The video provides a step-by-step approach to analyzing and calculating the behavior of electrical circuits using KVL.

Transcripts

Browse More Related Video

Kirchhoff's Voltage Law (KVL) Explained

Kirchoff's Loop Rule

03 - What is Ohm's Law in Circuit Analysis?

Series and Parallel Circuits

Capacitors and Kirchhoff: Crash Course Physics #31

Node Voltage Method Circuit Analysis With Current Sources

Related Tags

Kirchhoff's Law Electrical Circuits Voltage Drops Energy Conservation Ohm's Law Resistors Batteries Current Direction Problem Solving Physics Concepts