Introducing Integration (3 of 4: Notation)

Eddie Woo
12 Mar 201812:50
EducationalLearning
32 Likes 10 Comments

TLDRThe video script discusses the concept of integration, the reverse process of differentiation in calculus. It explains how integration allows us to calculate the total change or area by summing up small parts, using rectangles in a Cartesian plane. The script introduces sigma notation and the use of the greek letter sigma (Ξ£) to represent the addition of quantities. It also highlights the connection between the rate of change (differentiation) and the accumulation of change (integration), emphasizing their applications in real-world scenarios such as calculating distances traveled or water flow. The process concludes with a concise definition of integration as finding an area, which, contrary to intuitive expectations, is the opposite of finding a gradient.

Takeaways
  • πŸ”„ The two problems discussed are two perspectives on the same problem, with the ability to solve one leading to the solution of the other.
  • πŸ“ The concept of 'total change' and 'area' are intrinsically linked, where solving for one can lead to solving the other.
  • πŸ€Ήβ€β™‚οΈ The use of auxiliary angles is a common method to transform problems into more manageable forms.
  • 🎯 Sigma notation (Ξ£) is introduced as a way to represent the summation of a series of values, which is crucial in understanding the process of integration.
  • πŸ“ The principle of summing things up from one point to another is highlighted, emphasizing the flexibility in the values that can be summed.
  • πŸ”’ The concept of 'height' and 'width' of rectangles is discussed in the context of calculating areas, with specific attention to the orientation on a Cartesian plane.
  • πŸŒ€ The function 'f' and its derivative 'f dash' are clarified, with the latter renamed as 'f' in the context of the problem to avoid confusion.
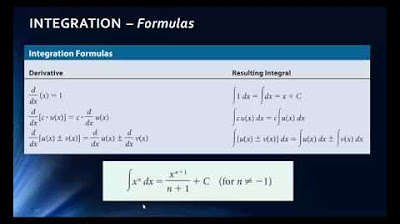
  • πŸ”„ The process of finding a 'primitive' function is introduced, which is the reverse process of differentiation and is denoted by a capital 'F'.
  • πŸ“Š The idea of integration is explained as combining a multitude of small elements into a single entity, represented by the summation of rectangles in the problem.
  • 🎭 The connection between differentiation and integration is emphasized, with the former providing the gradient and the latter providing the area.
  • 🌐 The practical applications of integration are highlighted, particularly in calculating total changes when the rate of change is known.
Q & A

  • What is the main concept discussed in the transcript?

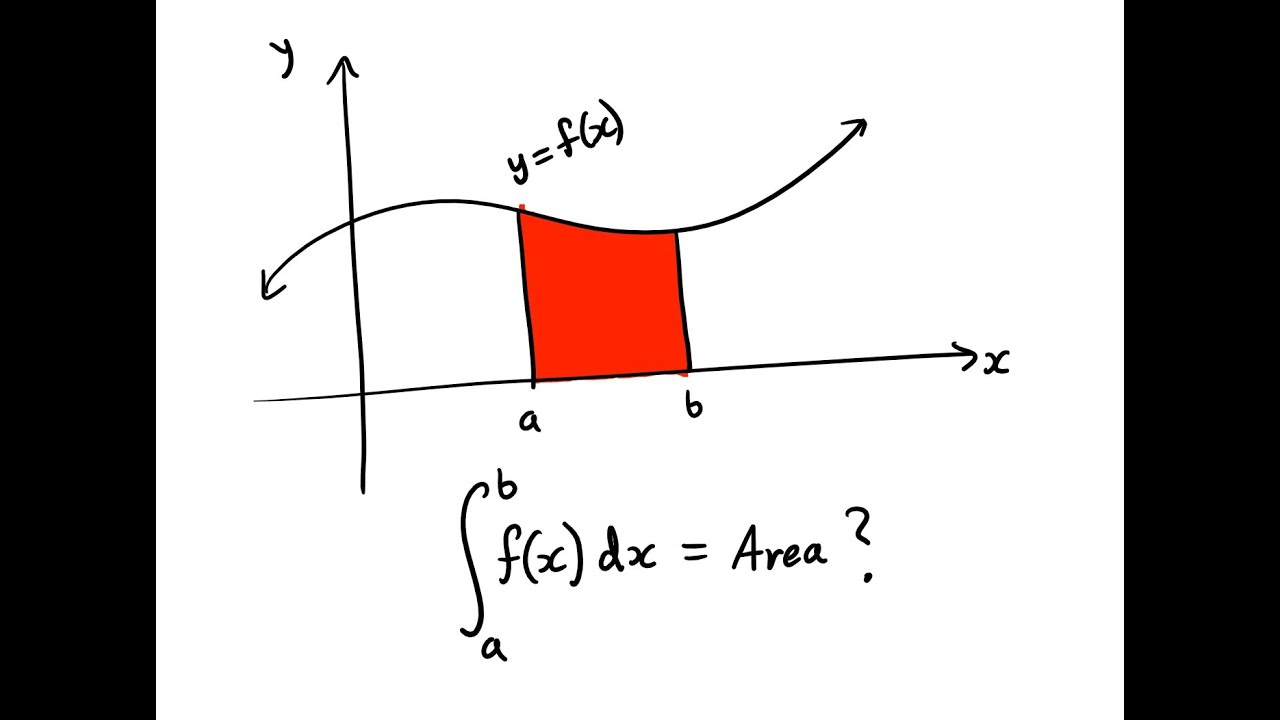
    -The main concept discussed in the transcript is the idea that solving an area problem and finding a total change are two perspectives on the same problem, and how integration is used to find the total change by summing up small areas, represented as rectangles in this context.

  • What is the significance of the sigma notation in the context of the transcript?

    -The sigma notation is significant in the transcript as it represents the summation of a series of quantities, in this case, the areas of rectangles. It is used to express the total distance traveled as the sum of the areas from one point to another.

  • How does the concept of 'primitive' relate to the function in the transcript?

    -In the transcript, a 'primitive' is a function from which the given function (f) is derived. It is the antiderivative of the function, and it is denoted by a capital F to indicate the original function before differentiation.

  • What is the relationship between differentiation and integration as explained in the transcript?

    -Differentiation and integration are presented as opposite processes. Differentiation is the process of finding the rate of change or gradient, while integration is the reverse process that finds the total change or area under a curve.

  • How does the concept of 'area' tie into the problem-solving process described in the transcript?

    -The concept of 'area' is central to the problem-solving process described in the transcript. It is used to calculate the total change by summing up the areas of rectangles, each representing a small change in the function's value over time.

  • What is the role of the Cartesian plane in the explanation provided?

    -The Cartesian plane is used to visually represent the function and the rectangles whose areas are being summed. The height and width of these rectangles are determined by the function's values and the change in the x-value, respectively.

  • How does the transcript explain the transition from dealing with whole objects to dealing with smaller pieces?

    -The transcript explains that while the initial topics like binomial theorem and series and sequences deal with whole objects, the current topic allows for dealing with smaller, irregular pieces of different shapes. This requires a new symbol and a different approach to summation.

  • What is the significance of the height and width of the rectangles in the integration process?

    -The height and width of the rectangles are significant as they determine the area of each rectangle being summed in the integration process. The height represents the function value at a given x-value (f'(x)), and the width represents the change in x (dx).

  • How does the transcript illustrate the concept of integration?

    -The transcript illustrates the concept of integration by describing it as the process of summing up a bunch of small areas (rectangles) to create a total area. This is done by considering each small change in x and the corresponding function value at that point.

  • What is the practical application of the concepts discussed in the transcript?

    -The practical application of the concepts discussed in the transcript is in calculating total changes over time when only the rate of change is known. This is useful in various fields such as physics for calculating displacement, in finance for calculating total profit, and in environmental studies for assessing changes over time.

Outlines
00:00
πŸ“Œ Introduction to Problem Solving via Notation

The paragraph introduces the concept of solving problems by using mathematical notation. It discusses the relationship between two problems that are essentially perspectives on the same issue. The speaker explains how calculating the total change or the area without drawing the figure is possible, and how these two approaches are interconnected. The paragraph emphasizes the use of auxiliary angles and the ease of solving problems through this method. The speaker also introduces the concept of summing areas, represented by rectangles in this context, and explains the use of sigma notation for addition. The discussion touches on the application of this method in various mathematical topics, such as binomial theorem and series and sequences.

05:01
πŸ“ˆ Understanding Area and Change in Functions

This paragraph delves into the specifics of summing rectangles to find an area and how this relates to functions and their derivatives. The speaker clarifies that the height of each rectangle is represented by the function f dash x, and the width is represented by changes in x (denoted as d x). The concept of a function's primitive, or the antiderivative, is introduced to find the original function from its derivative. The speaker renames the function from f dash to f to reflect this understanding and explains how to calculate the total area under the curve for any given interval by summing the areas of rectangles. The paragraph concludes with a brief mention of the flexibility in choosing the interval's start and end points.

10:03
🧩 Integration as the Reverse of Differentiation

The final paragraph wraps up the discussion by defining integration as the process of combining many small parts into one whole. It explains that integration is the reverse of differentiation, which is counterintuitive as their names might suggest. The speaker highlights the importance of understanding integration in various real-world applications, such as calculating total change when the rate of change is known. The paragraph emphasizes the connection between the concepts of gradient and area, and how integration allows us to calculate the total change that is not immediately apparent. The summary underscores the practical significance of integration in problem-solving and its role in calculus.

Mindmap
Keywords
πŸ’‘Notation
In the context of the video, notation refers to the mathematical symbols and expressions used to represent concepts and operations. It is crucial for understanding and solving mathematical problems, as it provides a standardized way to communicate ideas precisely. The video discusses using notation to express the relationship between total change and area, and introduces sigma notation as a tool for summing quantities, exemplified by adding up areas of rectangles without having to draw them.
πŸ’‘Total Change
Total change is the difference in a quantity or value from an initial point to a final point. In the video, it is used to describe the overall change in position or distance traveled by an object over time. The concept is closely related to the idea of differentiation in calculus, where the derivative of a function at a point represents the rate of change at that point. The video emphasizes that understanding total change is essential for various real-world applications, such as calculating the distance traveled by an astronaut over time.
πŸ’‘Area
Area refers to the measure of the extent of a two-dimensional shape or surface. In the video, the concept of area is used to represent the accumulated effect of small changes, such as the total distance traveled by an object when viewed over time. The video explains that by calculating the area under a curve, one can understand the total change in a system, which is a fundamental idea in integration. This concept is also used to illustrate the relationship between the rate of change (differentiation) and the accumulated effect (integration).
πŸ’‘Auxiliary Angle
An auxiliary angle is a supplementary angle used in trigonometry to simplify the analysis of trigonometric functions. In the context of the video, it is mentioned as a method to solve problems by transforming them into a more manageable form. The auxiliary angle is used to demonstrate how changing the perspective or representation of a problem can make it easier to solve, which is analogous to the process of integration where complex areas are broken down into simpler shapes like rectangles.
πŸ’‘Sigma Notation
Sigma notation, represented by the Greek letter sigma (Ξ£), is a symbolic way in mathematics to denote the sum of a sequence of terms. It is a concise representation of addition, where the sigma symbol indicates that you are adding up a series of values. In the video, sigma notation is introduced as a means to calculate the total area under a curve by summing up the areas of rectangles, each representing a small change in the function's value.
πŸ’‘Rectangles
In the context of the video, rectangles are used as a geometric representation of the small changes in a function's value over a given interval. These rectangles, when summed, form an approximation of the area under the curve, which is a fundamental concept in integration. The height and width of each rectangle correspond to the change in the function's value (f'(x)) and the change in the independent variable (dx), respectively.
πŸ’‘Height and Width
In the context of the video, height and width refer to the dimensions of the rectangles used to approximate the area under a curve. The height of each rectangle is determined by the function's derivative (f'(x)), representing the change in the function's value, while the width is determined by the change in the independent variable (dx). Together, these dimensions are used to calculate the area of each small rectangle, which, when summed, approximate the total area under the curve.
πŸ’‘Cartesian Plane
A Cartesian plane, also known as a coordinate plane, is a two-dimensional coordinate system that consists of a horizontal axis (x-axis) and a vertical axis (y-axis). It is named after the French mathematician RenΓ© Descartes. In the video, the Cartesian plane is used as a reference for the orientation of the rectangles, with the height of the rectangles corresponding to the vertical axis and the width to the horizontal axis. This orientation helps in visualizing and calculating the area of the rectangles that represent small changes in the function's value.
πŸ’‘Primitive
In calculus, a primitive or antiderivative of a function is a function whose derivative is the original function. The process of finding a primitive is known as integration. In the video, the term 'primitive' is used to describe the reverse process of differentiation, where one is looking for a function that, when differentiated, yields the original function. This concept is crucial for understanding the relationship between differentiation and integration and their applications in solving problems.
πŸ’‘Integration
Integration is a fundamental concept in calculus that involves finding the area under a curve or the accumulated sum of small changes. It is the reverse process of differentiation, where differentiation finds the rate of change or gradient of a function, integration finds the total change or area. The video emphasizes that integration allows us to calculate the total effect or accumulation from many small parts, which is essential for understanding and solving a wide range of problems in various fields.
πŸ’‘Conclusion
The conclusion section of the video summarizes the main points and provides a final understanding of the concepts discussed. In this case, the conclusion emphasizes that integration is the reverse process of differentiation, allowing us to find the total change or area by combining many small parts. The video highlights that this is not an intuitive concept, as the opposite of finding a gradient is not immediately recognizable as finding an area. However, this concept is powerful because it enables us to calculate the overall effect of many small changes, which is a common requirement in various real-world scenarios.
Highlights

The transcript discusses the concept of solving problems from two different perspectives, namely total change and area, which are essentially two sides of the same coin.

The use of auxiliary angles is mentioned as a common method for solving problems, emphasizing the ease of solving the right-hand side of an equation.

The transcript introduces the concept of sigma notation, a mathematical notation used for summing a series of elements.

The transcript explains the application of sigma notation in the context of summing areas, specifically rectangles, and how it relates to the concept of integration.

The transcript clarifies the importance of understanding the height and width of rectangles in the context of summing areas, and how these dimensions relate to the function and its derivative.

The transcript introduces the concept of a primitive function, which is a function from which the given function is derived, and its notation is represented by a capital letter.

The transcript emphasizes the flexibility of choosing start and end points in the context of summing areas, highlighting the adaptability of the method.

The transcript explains the process of integration, which involves combining a multitude of small elements to form a whole, and its connection to calculus.

The transcript discusses the counterintuitive nature of integration being the opposite of differentiation, as it involves finding an area rather than a gradient.

The transcript highlights the practical applications of integration, such as calculating total changes in various real-world scenarios.

The transcript touches on the concept of differentiating and integrating in the context of an astronaut's velocity and displacement, providing a real-world example.

The transcript explains the use of the letter 'd' to represent a change in a variable, such as x or y, which is a fundamental concept in calculus.

The transcript describes the process of renaming functions and their derivatives to better fit the context of the problem being solved, emphasizing the importance of accurate notation.

The transcript simplifies the notation for expressing the summation of areas by introducing a shorthand method, making the process more efficient and less redundant.

The transcript concludes by reiterating the significance of integration in understanding the total change or area in various contexts, such as travel distance or water flow.

Transcripts

Browse More Related Video

Understand Calculus in 35 Minutes

Calculus - Lesson 12 | Addition as Integration | Don't Memorise

Calculus 1 Lecture 4.3: Area Under a Curve, Limit Approach, Riemann Sums

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Calculus: Why does integrating a function give area under its curve?

Math 1325 Lecture 12 1

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
MathematicsIntegrationDifferentiationTotal ChangeArea CalculationEducationalConceptual UnderstandingInverse ProcessesCartesian PlaneSigma Notation