Physics 101 - Chapter 2 - Motion in One Dimension
TLDRIn this educational video, the presenter delves into the fundamentals of physics, focusing on motion in one dimension as covered in chapter two of Physics 101. The discussion begins with an introduction to the three types of motion: translational, rotational, and vibrational, using everyday examples for clarity. The concept of position is explored, emphasizing its relationship with time and the coordinate system. The video then transitions into a detailed explanation of velocity, differentiating between average and instantaneous velocity, and acceleration. Practice problems are presented to reinforce the concepts, highlighting the relationship between position, velocity, and acceleration. The content is engaging and informative, designed to solidify the viewer's understanding of these fundamental physics concepts.
Takeaways
- 📚 The three types of motion in physics are translational, rotational, and vibrational, each described by different examples like a car traveling (translational), Earth rotating (rotational), and a pendulum swinging (vibrational).
- 🚗 In the study of motion, the particle model is used to simplify objects to points with mass, disregarding their size and internal structure.
- 📍 Position is defined as the location of an object in space with respect to a coordinate system, and it is a fundamental concept in physics.
- 🕒 Position is a function of time, often denoted as x(t), indicating the object's location at different times during its motion.
- 📈 A position vs. time graph can be used to visualize an object's motion, with the x-axis typically representing time and the y-axis representing position.
- 🛣️ Displacement is the change in position (Δx = x_final - x_initial) and is different from distance traveled, as it does not account for the path taken.
- 🏃♂️ Velocity is the rate of change of position and can be both positive and negative, with the sign indicating the direction of motion relative to the chosen coordinate system.
- 🚦 Average velocity is calculated as the displacement divided by the time interval (Δx / Δt), and it can also be positive or negative depending on the direction of motion.
- 🛤️ Average speed, unlike velocity, has no direction and is always positive, calculated as the total distance traveled divided by the time interval (d / Δt).
- 🌀 Instantaneous velocity at a specific point can be found by taking the derivative of the position function or by calculating the slope of the tangent to the position vs. time graph at that point.
- 💨 Acceleration is the rate of change of velocity (dv/dt) and can be positive or negative, indicating whether the speed of an object is increasing or decreasing over time.
Q & A
What are the three types of motion discussed in the script?
-The three types of motion discussed are translational, rotational, and vibrational motion.
What is an example of translational motion?
-An example of translational motion is a car traveling down the highway.
How is rotational motion described in the script?
-Rotational motion is described as the Earth rotating on its axis, causing day and night cycles.
What is the particle model used for in the study of motion?
-The particle model is used to simplify the study of motion by treating the moving object as a point with mass but of infinitesimally small size.
What is the definition of position?
-Position is defined as the location of an object in space with respect to a coordinate system.
How is the position of an object related to time?
-The position of an object is a function of time, often written as x(t), where x represents the position and t represents the time elapsed.
What is displacement and how is it different from distance?
-Displacement is the change in position of an object and is represented by the symbol delta x (Δx). It is different from distance in that displacement considers the shortest path between the initial and final positions, while distance accounts for the actual path length traveled by the object.
How is velocity defined in physics?
-Velocity is defined as the rate of change of position with respect to time. It is a vector quantity that considers both magnitude and direction.
What is the difference between average velocity and instantaneous velocity?
-Average velocity is the displacement divided by the time interval during which the displacement occurs, while instantaneous velocity is the limit of the velocity as the time interval approaches zero. Instantaneous velocity provides the velocity at a specific moment in time, whereas average velocity provides the average velocity over a period of time.
How is acceleration defined and what are its units?
-Acceleration is defined as the rate of change of velocity with respect to time. Its units are meters per second squared (m/s^2).
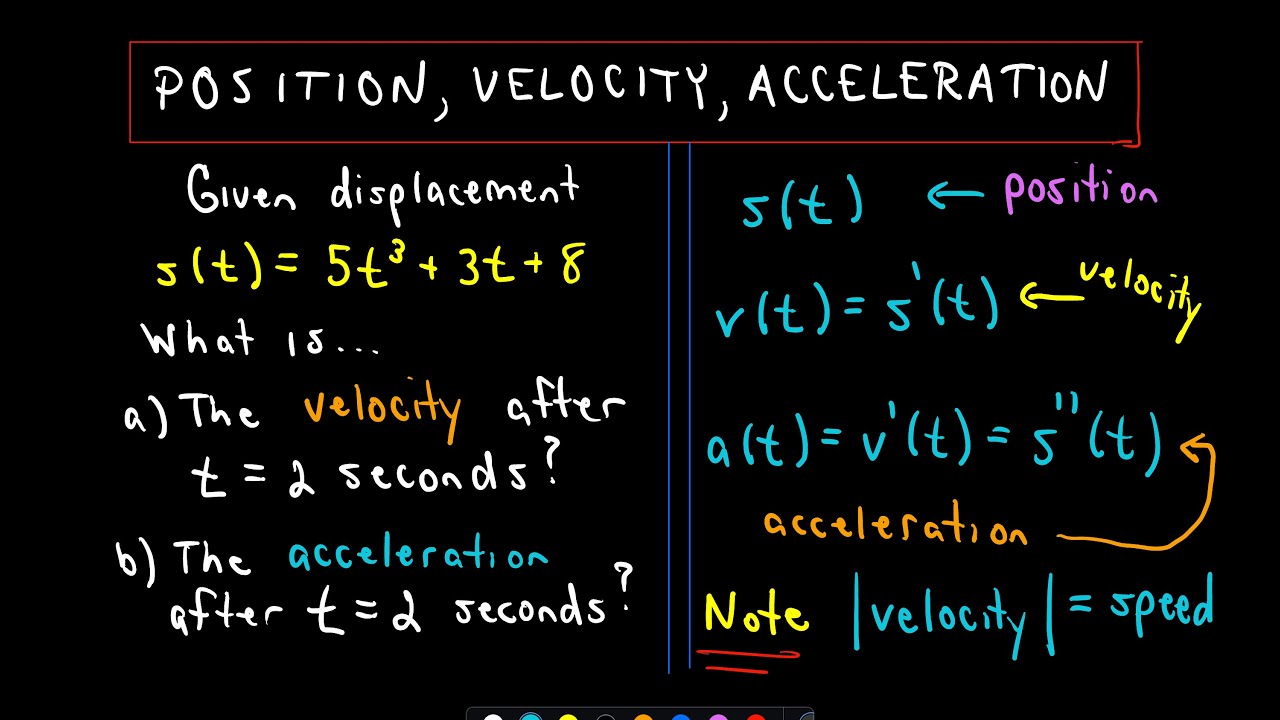
What is the relationship between the position function and the velocity function?
-The velocity function is the derivative of the position function with respect to time. This means that the rate of change of position over time gives us the velocity of the object.
How can you find the total distance traveled by an object?
-The total distance traveled by an object can be found by integrating the absolute value of the velocity function over the given time interval. This gives the total path length covered by the object, regardless of direction.
Outlines
📘 Introduction to Motion in Physics
The script begins with an introduction to chapter two of Physics 101, focusing on motion in one dimension. It emphasizes that the discussion will serve as a quick review for those familiar with the concepts and an introduction for newcomers. The speaker provides context by explaining that motion in physics is categorized into three types: translational, rotational, and vibrational. The example of a car traveling down the highway is used to illustrate translational motion, while the Earth's rotation on its axis is given as an example of rotational motion. Vibration is described as a back-and-forth movement, like that of a pendulum. The speaker clarifies that the upcoming chapters will primarily deal with translational and rotational motion, using the particle model to simplify the study of translational motion.
📐 The Concept of Position in Physics
The speaker delves into the concept of position, defining it as the location of an object in space with respect to a coordinate system. The position is highlighted as a fundamental aspect of studying motion. Using the example of a car on a highway, the speaker explains how to represent the car's position on a one-dimensional coordinate system. The speaker also introduces the idea that position is a function of time, denoted as x(t), and emphasizes the importance of the coordinate system in understanding and solving physics problems. The concept is further illustrated by discussing how different coordinate systems can yield different results.
📊 Position vs. Time Graphs
The speaker introduces the use of position versus time graphs as a tool for visualizing an object's motion. The script describes how different scenarios can be represented graphically, such as an object moving in a straight line, indicating constant velocity, or moving in a manner that changes direction, indicating variable velocity. The speaker explains that the slope of the line on the graph represents the velocity of the object, and how this can be positive or negative depending on the direction of motion. The concept of displacement is also introduced as the change in position (delta x), which is different from distance traveled.
🚗 Examples of Motion and Displacement
This paragraph provides examples to illustrate the concepts of motion and displacement. The speaker uses the example of a car moving on a highway to explain how the car's position changes over time and how this can be represented graphically. The concept of displacement is further clarified by contrasting it with distance traveled. The speaker also introduces the idea of velocity as a measure of how fast an object is moving and how it can be positive or negative depending on the direction of motion. The importance of understanding the coordinate system and the object's initial and final positions is emphasized.
📌 Understanding Velocity and Speed
The speaker clarifies the difference between velocity and speed, explaining that velocity is a vector quantity that includes direction while speed is a scalar quantity with no direction. The concept of average velocity is introduced, defined as the displacement divided by the time interval over which the displacement occurs. The speaker also discusses instantaneous velocity, which is the limit of the average velocity as the time interval approaches zero. The relationship between the slope of a position-time graph and velocity is highlighted, as well as the integral of velocity over time representing displacement.
🔄 Calculating Displacement and Distance
The speaker provides a detailed explanation of how to calculate displacement and distance using the concepts introduced earlier. The difference between the two is emphasized, with displacement being the change in position (denoted as delta x) and distance being the total path length traveled. The speaker uses the example of a person going to school, forgetting their backpack, and then going back home to illustrate the difference. The concept of average speed is also introduced, defined as the total distance traveled divided by the time interval. The speaker notes that average speed does not have a direction and is always a positive value.
📚 Instantaneous Velocity and Acceleration
The speaker discusses instantaneous velocity, which is the velocity of an object at a specific moment in time. It is calculated as the derivative of the position function with respect to time. The concept of acceleration is introduced as the rate of change of velocity, with both average and instantaneous acceleration explained. The speaker emphasizes that acceleration can be positive or negative depending on whether the velocity of the object is increasing or decreasing over time. The relationship between the direction of acceleration and the direction of motion is also discussed, with positive acceleration occurring when the two are in the same direction and negative acceleration (deceleration) when they are in opposite directions.
📈 Practice Problems on Acceleration
The speaker presents two practice problems to solidify the understanding of acceleration. The first problem involves finding the times when the velocity of an object, described by a given position function, is zero. The speaker explains how to find the derivative of the position function to obtain the velocity function and then solve for when the velocity is zero. The second problem asks for the position and total distance traveled by a particle at a given time, based on its velocity function. The speaker demonstrates how to find the position by integrating the velocity function and how to calculate the total distance traveled by considering the absolute value of the velocity function, which represents speed.
Mindmap
Keywords
💡Translational Motion
💡Rotational Motion
💡Vibrational Motion
💡Particle Model
💡Position
💡Displacement
💡Velocity
💡Acceleration
💡Coordinate System
💡Graphs
Highlights
Introduction to chapter two of Physics 101 focusing on motion in one dimension.
Explanation of the three types of motion: translational, rotational, and vibrational.
Discussion on the particle model used to simplify the study of translational motion.
Definition of position as the location of an object in space with respect to a coordinate system.
Explanation of how position is a function of time (x(t)).
Use of the position versus time graph to understand the motion of an object.
Description of the difference between displacement and distance.
Introduction to velocity as the rate of change of position.
Explanation of average velocity and its calculation (delta x / delta t).
Clarification on the difference between velocity and speed.
Definition and calculation of instantaneous velocity.
Discussion on the concept of instantaneous speed as the magnitude of instantaneous velocity.
Introduction to acceleration as the rate of change of velocity.
Explanation of average acceleration and its calculation (delta v / delta t).
Description of the relationship between the direction of acceleration and the direction of motion.
Use of calculus to understand the concept of instantaneous acceleration.
Practice problem involving finding the velocity and position of a particle given its position function.
Solution to a problem calculating the total distance traveled by a particle at a specific time.
Comparison between displacement and distance traveled by a particle, highlighting the importance of understanding their differences.
Transcripts
Browse More Related Video

8.01x - Lect 2 - 1D Kinematics - Speed, Velocity, Acceleration

College Physics 1: Lecture 23 - Rotational Motion
Position, Velocity, Acceleration using Derivatives
Worked example: motion problems (with definite integrals) | AP Calculus AB | Khan Academy

College Physics 1: Lecture 7 - Instantaneous Velocity

Visualizing Motion Using Tape Charts and Motion Graphs | SCIENCE 7 QUARTER 3 MODULE 2 WEEK 3
5.0 / 5 (0 votes)
Thanks for rating: