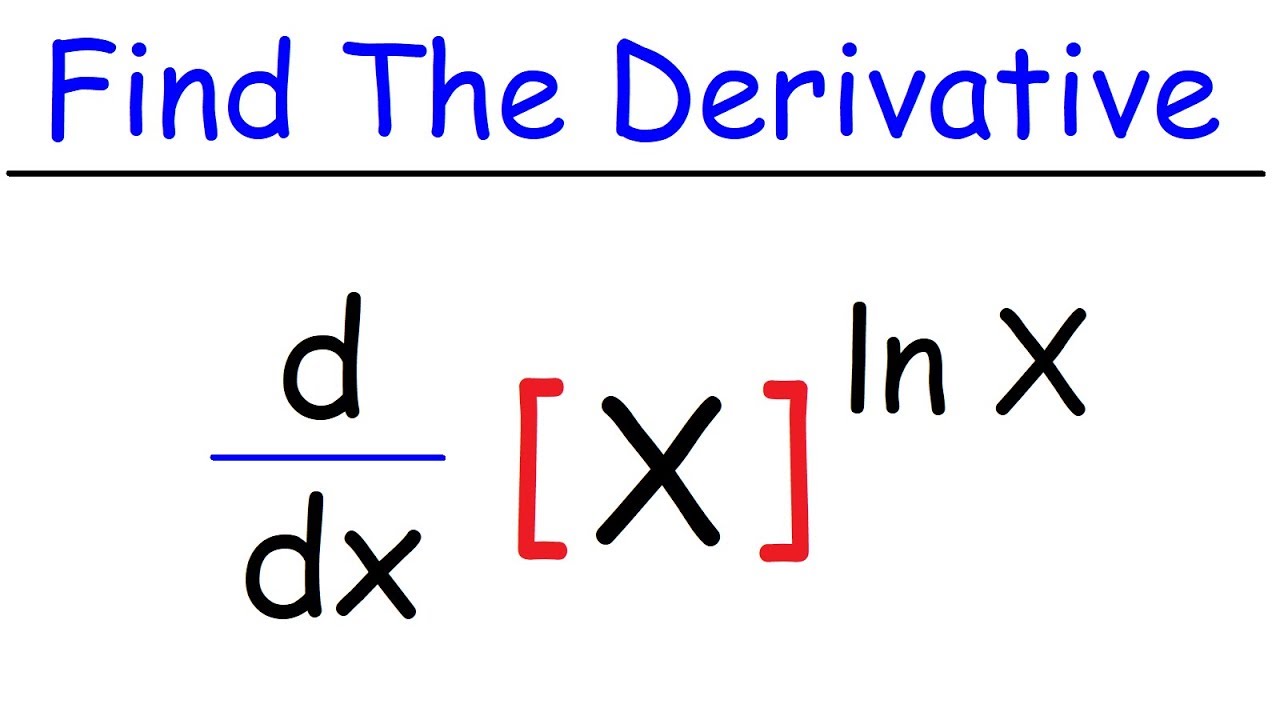
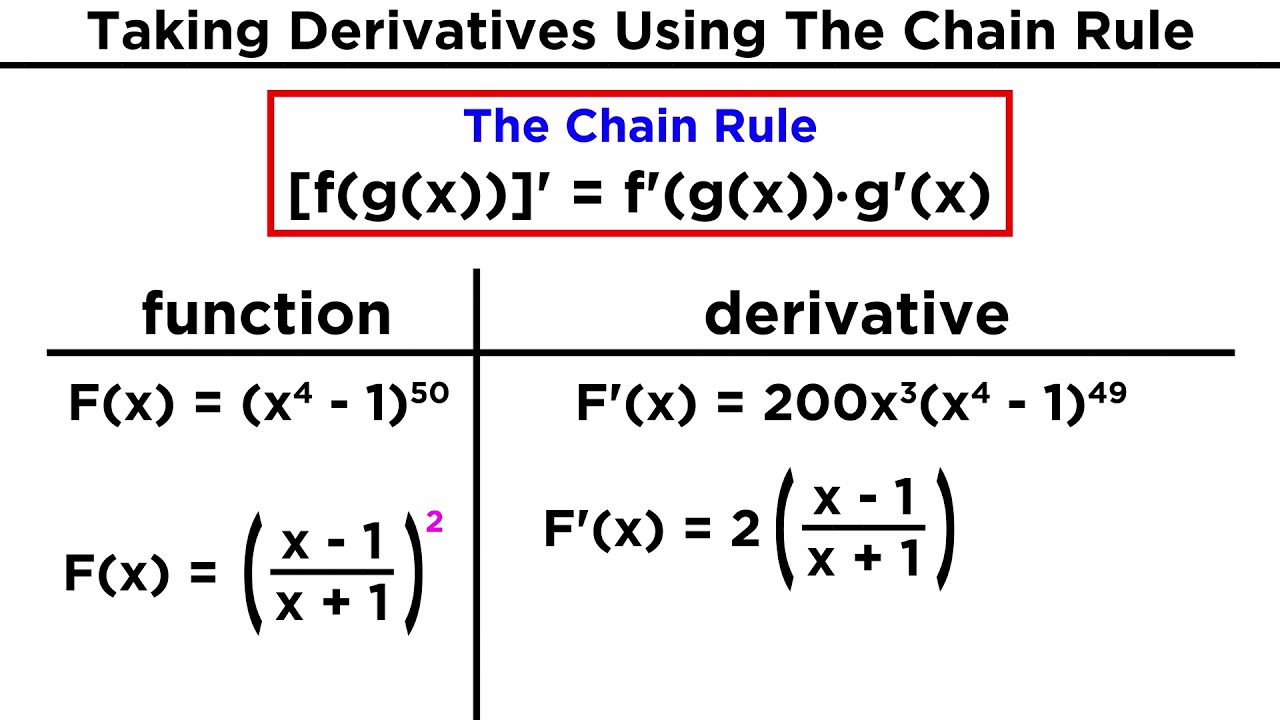
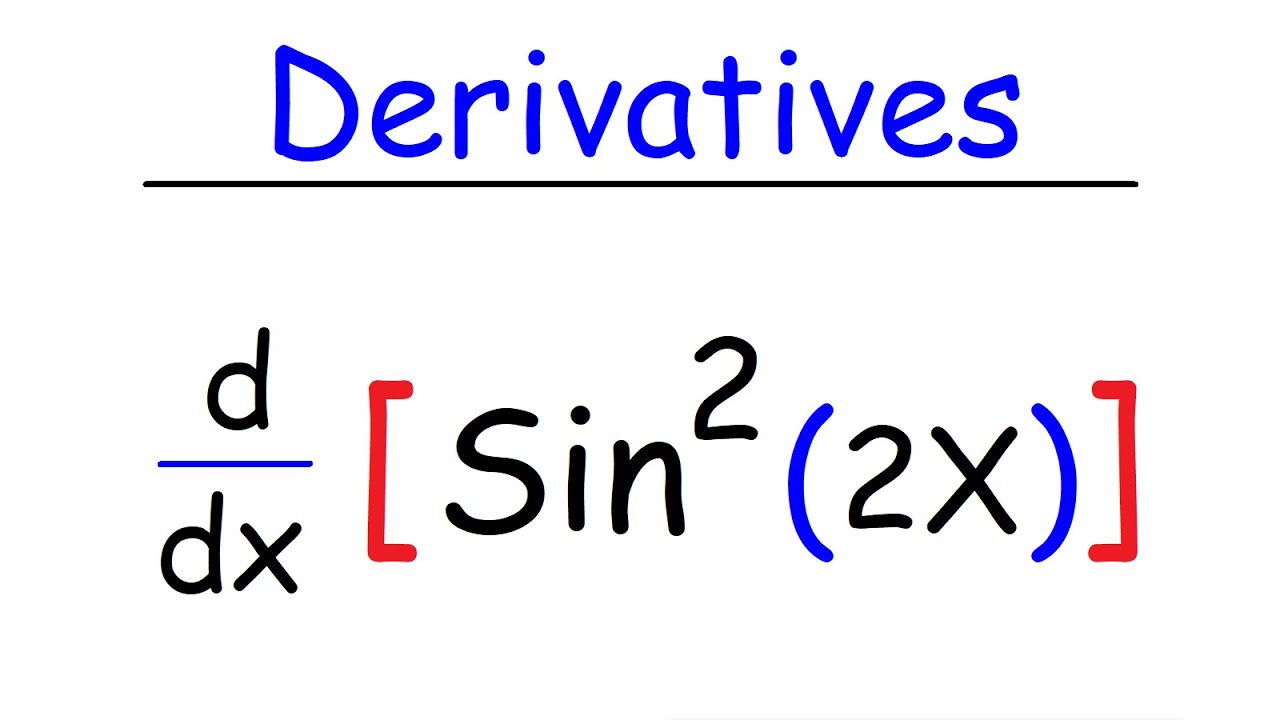Calculus - Chain Rule - Derivatives
TLDRThe video script provides a comprehensive guide on using the chain rule to find derivatives of composite functions. It explains the process step by step, starting with the outermost function and working inwards, and emphasizes the importance of differentiating the outer function while keeping the inner function unchanged. The script illustrates the method with several examples, including finding derivatives of complex expressions like the fifth power of sine of cosine, the square root of tangent, and the natural log of x. The examples are detailed, showing how to simplify the expression and apply the chain rule effectively.
Takeaways
- 📚 The chain rule is essential for finding derivatives of composite functions, where you differentiate the outside function first and then multiply by the derivative of the inside function.
- 🔢 When applying the chain rule, it's helpful to rewrite complex expressions in a more manageable format, such as separating out exponents and roots for clarity.
- 📈 The power rule is used in conjunction with the chain rule to handle expressions like raising a function to a power, such as (u^4)' = 4u^3.
- 🌀 For trigonometric functions within composite functions, like sine or cosine, their derivatives (cosine for sine and negative sine for cosine) are applied with the inner function as the new 'u'.
- 🔢 When differentiating a function like tangent, its derivative (secant squared) is used, and the inner function's derivative is carried through the process.
- 🛠️ The process of differentiating composite functions involves a step-by-step approach, starting from the outermost function and working towards the innermost part.
- 📊 Understanding the derivatives of basic functions like x^2 (2x) and x^3 (3x^2) is crucial for more complex composite function differentiation.
- 🌟 The chain rule allows for the simplification of complex derivative problems by breaking them down into simpler, more manageable parts.
- 📚 The video script provides practical examples of using the chain rule, such as differentiating x^2 + 5x^4, tan(x^2), and sin(tan(x^3)cos(ln(x))^4), to illustrate the concept.
- 🎯 The script emphasizes the importance of practice and applying the chain rule to various examples to solidify understanding and improve problem-solving skills.
- 📈 The final example of differentiating the complex function sin(tan(x^3)cos(ln(x))^4) demonstrates the application of the chain rule in a step-by-step manner, highlighting the process of working from the outside in.
Q & A
What is the main concept discussed in the video?
-The main concept discussed in the video is the chain rule for finding the derivative of composite functions.
How do you apply the chain rule?
-To apply the chain rule, you differentiate the outer function first while keeping the inner function the same, and then multiply by the derivative of the inner function.
What is the first example given in the video to demonstrate the chain rule?
-The first example given is finding the derivative of x squared plus 5x raised to the fourth power.
What are the derivatives of the inner functions used in the example of tangent x squared?
-The derivative of the outer function tangent is secant squared, and the derivative of the inner function x squared is 2x.
How is the problem of finding the derivative of sine tangent x cubed approached?
-The problem is approached by first finding the derivatives of the outer functions (sine and tangent), and then working towards the inner functions (tangent x cubed) using their respective derivatives (secant squared and 3x squared).
What is the recommended strategy for solving complex composite functions?
-The recommended strategy is to start from the outside and work your way in, differentiating each function in the composition one at a time.
How does the video suggest rewriting complex expressions to simplify the process?
-The video suggests rewriting complex expressions by breaking them down into simpler components, such as separating powers and roots, to make the application of the chain rule easier.
What is the final example given to illustrate the use of the chain rule?
-The final example is finding the derivative of the fifth power of sine of cosine to the fourth power of the square root of tangent of the natural log of x.
What is the derivative of ln x?
-The derivative of ln x is 1/x.
How does the video emphasize the importance of the order of operations when using the chain rule?
-The video emphasizes that the order of operations is crucial when using the chain rule, as you must differentiate the outermost function first and then work your way inwards to the inner functions.
Outlines
📚 Introduction to the Chain Rule and Derivative Examples
This paragraph introduces the concept of the chain rule for finding derivatives of composite functions. It explains that when functions are nested, the chain rule is necessary. The process involves taking the derivative of the outer function and multiplying it by the derivative of the inner function. The explanation is supported by examples, such as finding the derivative of x squared plus 5x raised to the fourth power, demonstrating the use of the power rule and chain rule. The paragraph also covers the derivative of tangent x squared and sine tangent x cubed, illustrating the step-by-step application of the chain rule.
📈 Deriving Complex Functions Using the Chain Rule
This paragraph delves into the application of the chain rule for more complex functions. It emphasizes the importance of rewriting expressions to simplify the process, such as expressing cosine to the fourth power as cosine x to the fourth. The paragraph then walks through the derivation of a complex function involving sine, cosine, tangent, and the natural log of x. It details each step, from handling the outermost function to the innermost, and highlights the need to adjust for exponents and work through each layer of the function. The explanation is methodical, ensuring a clear understanding of the chain rule's application in complex scenarios.
🎓 Summary and Conclusion of Chain Rule Application
The final paragraph summarizes the process of using the chain rule to find derivatives of composite functions. It reiterates the steps taken in previous examples and provides the final answer for the complex function discussed. The paragraph concludes by encouraging viewers to practice and apply the chain rule to further their understanding of derivatives. It ends with a prompt for viewers to subscribe to the channel for more educational content.
Mindmap
Keywords
💡Derivative
💡Chain Rule
💡Composite Functions
💡Power Rule
💡Sine, Cosine, Tangent
💡Natural Logarithm (ln x)
💡Square Root
💡Exponents
💡Secant
💡Differentiation
💡Rewriting Expressions
Highlights
The problem involves finding the derivative of a complex function involving multiple composite functions.
The chain rule is necessary for solving this problem due to the nested nature of the functions.
The process of using the chain rule involves differentiating the outer function first and then multiplying by the derivative of the inner function.
An example is provided to illustrate the application of the chain rule, which is finding the derivative of x squared plus 5x raised to the fourth power.
The power rule is used in conjunction with the chain rule to simplify the expression and find the derivative.
Another example demonstrates finding the derivative of tangent x squared, showing the steps to apply the chain rule effectively.
The derivative of sine is found by considering the entire inner function as the argument of cosine.
The derivative of cosine involves dealing with an exponent and is found by using the chain rule and considering the inner functions.
The derivative of tangent is calculated by applying the chain rule to the inner function, which involves the natural log of x.
The process of simplifying the complex function expression is emphasized to make the application of the chain rule more manageable.
The final example involves a complex function with sine, cosine, tangent, and the natural log of x, showcasing the comprehensive use of the chain rule.
The solution to the complex problem is derived step by step, illustrating the power of the chain rule in calculus.
The video provides a clear and detailed explanation of the chain rule, making it accessible for learners at various levels.
The transcript serves as a valuable resource for understanding the chain rule and its application to complex derivative problems.
The examples given in the transcript are practical applications of the chain rule, helping to solidify the concept for the reader.
The transcript emphasizes the importance of starting from the outside and working towards the inner functions when applying the chain rule.
The video concludes with an encouragement for viewers to practice and apply the concepts learned, fostering an environment of continuous learning.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: