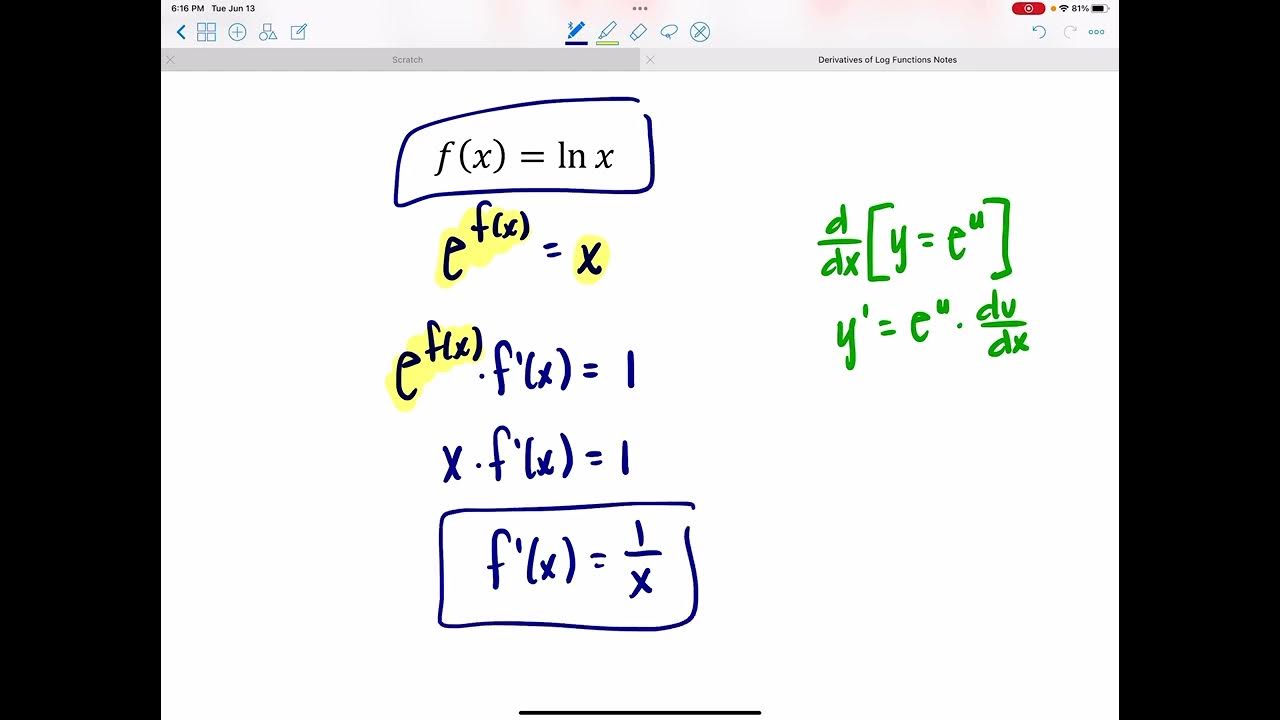
Lesson 14 - Derivatives Of Logarithms (Calculus 1 Tutor)
TLDRThis educational video delves into the concept of logarithms, with a focus on the derivatives of logarithms, exploring functions beyond the usual polynomials and trigonometric functions. The instructor clarifies the integral of x to the power of negative one, explaining why the standard polynomial rule fails and introducing the concept of natural logarithm. The video aims to enhance understanding of calculus, emphasizing the importance of recognizing when standard formulas do not apply.
Takeaways
- 📚 The course section focuses on logarithms, particularly their derivatives, which are more advanced topics in mathematics.
- 🌟 Common functions include polynomials and trigonometric functions, and now logarithms are introduced as another important function type.
- 🔍 Review of basic integrals is provided, such as the integral of x squared being one-third x cubed plus a constant.
- 📈 The process of finding integrals involves manipulating the exponent according to specific rules, such as adding one to the exponent and dividing by the new exponent.
- ⚠️ The standard rule for polynomials does not apply when integrating x to the power of -1, leading to undefined results.
- 💡 In calculus, division by zero is interpreted as infinity, which is a concept that differs from traditional math classes.
- 🌐 The integral of x to the power of -1 (1/x) is defined as the natural logarithm of x, which is a fundamental result in calculus.
- 📝 The properties of integrals and derivatives of logarithmic functions will be used without going into the details of their derivations.
- 🎓 The importance of understanding the limitations of standard rules when dealing with certain function forms, such as x to the power of -1, is emphasized.
- 🔑 The natural logarithm is introduced as a key concept for handling integrals of functions like 1/x, which do not follow the standard polynomial integration rules.
Q & A
What is the main topic of this section of the course?
-The main topic of this section is logarithms, with a specific focus on the derivatives of logarithms.
What is the integral of x squared with respect to x?
-The integral of x squared with respect to x is one-third x cubed plus a constant.
How is the integral of x squared with respect to x calculated?
-It is calculated by using the power rule for integration, which involves taking the exponent, adding 1 to it, writing down x, and then putting the new exponent with a plus 1.
What is the integral of x to the fourth with respect to x?
-The integral of x to the fourth with respect to x is one-fourth x to the fifth plus a constant.
What happens when trying to apply the standard power rule to the integral of x to the minus one?
-Applying the standard power rule to the integral of x to the minus one leads to an undefined result, as it results in one over zero, which is traditionally considered undefined.
How is the integral of x to the minus one with respect to x defined?
-The integral of x to the minus one with respect to x is defined as the natural logarithm of x, denoted as ln(x), plus a constant.
Why can't the standard power rule be used for the integral of x to the minus one?
-The standard power rule cannot be used for the integral of x to the minus one because it results in an undefined expression (one over zero), which is not a valid mathematical result.
What is the significance of the natural logarithm in this context?
-In this context, the natural logarithm serves as the integral of 1 over x, or x to the minus one, providing a way to calculate the integral when the standard power rule fails.
What is the integral of 1 over x with respect to x?
-The integral of 1 over x with respect to x is the natural logarithm of x plus a constant.
How does the concept of infinity relate to the integral of x to the minus one?
-The concept of infinity comes into play because when trying to divide by zero, which happens in the process of applying the standard power rule to x to the minus one, it suggests that zero can be entered an infinite number of times, leading to the definition of the integral as the natural logarithm of x.
What is the condition for the formula of the integral of x to the n with respect to x?
-The formula for the integral of x to the n with respect to x, which is one over n plus 1 x to the n plus 1, is only true when x is not equal to negative one.
Outlines
📚 Introduction to Logarithms and Derivatives
This paragraph introduces the topic of logarithms, focusing on the derivatives of logarithms. It acknowledges the shift to more advanced mathematical functions that are not commonly encountered daily, following previous discussions on polynomials and trigonometric functions. The paragraph begins with a review of basic integrals, such as the integral of x squared, and explains the standard process of integration by raising the exponent by one and adjusting the constant term. It then poses a question about the integral of x to the power of negative one, highlighting the limitations of the standard integration rule when applied to this case. The explanation delves into the concept of infinity and the implications of division by zero in calculus, emphasizing that the standard formula does not apply when integrating x to the power of negative one. The paragraph concludes by noting that the calculus textbooks will provide the necessary definitions and results for dealing with such integrals, specifically mentioning that the integral of 1 over x (or x to the power of negative one) is defined as the natural logarithm of x, with the inclusion of a constant term as with any integral.
Mindmap
Keywords
💡Logarithms
💡Derivatives
💡Polynomials
💡Trigonometric Functions
💡Integrals
💡Exponential Functions
💡Natural Logarithm
💡Limits
💡Infinite
💡Mechanics
💡Definitions
Highlights
The course covers the topic of logarithms, especially derivatives of logarithms, which are more advanced topics in mathematics.
Logarithms are a type of function that is not commonly seen every day, unlike polynomials and trigonometric functions.
The integral of x squared is one-third x cubed plus a constant, which is a basic integral formula.
The integral of x to the fourth is four-fifths x to the fifth plus a constant, demonstrating the straightforward mechanics of integration.
The integral of x to the minus one is a special case that cannot be solved using the standard polynomial integration rule.
When trying to apply the standard rule to x to the minus one, the result is undefined, as anything divided by zero is considered infinite in calculus.
The formula for the integral of x to the n is one over n plus one x to the n plus one, but this is only true when x is not equal to negative one.
The integral of 1 over x, which is the same as x to the minus one, is defined as the natural logarithm of x.
The natural logarithm of x is a fundamental concept in calculus and has its own unique properties and applications.
The transcript emphasizes the importance of understanding the limitations of standard integration formulas when dealing with certain functions.
The concept of infinity in calculus is introduced, which is crucial for understanding the behavior of functions when approaching zero.
The transcript provides a clear explanation of why the standard integration formula fails for x to the minus one, highlighting the need for alternative methods.
The use of natural logarithms in integration is a key topic in advanced mathematics and has practical applications in various fields.
The transcript serves as a guide for learners to understand the transition from basic to more advanced integral formulas and their applications.
The course material is designed to build a strong foundation in understanding the properties and applications of logarithms in calculus.
The discussion on logarithms and their integrals is part of a broader educational effort to enhance understanding of mathematical concepts.
The transcript provides a comprehensive overview of the challenges and solutions in integrating functions like x to the minus one.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: